Skip to main content

Advertisement

Springer Nature Link
Log in

Normalized projection approach to group decision-making with hybrid decision information

  • Original Article
  • Published:
International Journal of Machine Learning and Cybernetics Aims and scope Submit manuscript

Abstract

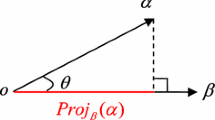
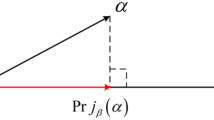
Projection is an important measure in decision science, and it is also often used as a tool for various administrators. However, there are some defects in the existing projection models. To solve this significant scientific problem, this paper intends to establish new projection measures between two real vectors and between two interval vectors. First, the hidden flaws of existing projection measures are pertinently shown, and new projection measures in real number and interval settings are established, which satisfy the condition of normalization. Then new projection measures are applied to group decision-making with hybrid decision information, including real numbers and interval data. Finally, an experimental analysis shows the applicability, feasibility, effectiveness and advantages of the proposed methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

References

  1. Cabrerizo FJ, Chiclana F, Al-Hmouz R, Morfeq A, Balamash AS, Herrera-Viedma E (2015) Fuzzy decision making and consensus: challenges. J Intell Fuzzy Syst 29(3):1109–1118

    Article  MathSciNet  MATH  Google Scholar 

  2. Cabrerizo FJ, Herrera-Viedma E, Pedrycz W (2013) A method based on pso and granular computing of linguistic information to solve group decision making problems defined in heterogeneous contexts. Eur J Oper Res 230(3):624–633

    Article  MathSciNet  MATH  Google Scholar 

  3. Chen T-Y (2013) An interval-valued intuitionistic fuzzy LINMAP method with inclusion comparison possibilities and hybrid averaging operations for multiple criteria group decision making. Knowl Based Syst 45:134–146

    Article  Google Scholar 

  4. Chen T-Y (2015) The inclusion-based TOPSIS method with interval-valued intuitionistic fuzzy sets for multiple criteria group decision making. Appl Soft Comput 26:57–73

    Article  Google Scholar 

  5. Dong Y, Herrera-Viedma E (2015) Consistency-driven automatic methodology to set interval numerical scales of 2-tuple linguistic term sets and its use in the linguistic GDM with preference relation. IEEE Trans Cybern 45(4):780–792

    Article  Google Scholar 

  6. Fu C, Gao X, Liu M, Liu X, Han L, Chen J (2011) GRAP: Grey risk assessment based on projection in ad hoc networks. J Parallel Distrib Comput 71(9):1249–1260

    Article  MATH  Google Scholar 

  7. Ju Y, Wang A (2013) Projection method for multiple criteria group decision making with incomplete weight information in linguistic setting. Appl Math Model 37(20):9031–9040

    Article  MathSciNet  Google Scholar 

  8. Kuo R, Wang Y, Tien F (2010) Integration of artificial neural network and MADA methods for green supplier selection. J Clean Prod 18(12):1161–1170

    Article  Google Scholar 

  9. Liu X, Zhu J, Liu S (2014) Bidirectional projection method with hesitant fuzzy information. Syst Eng Theory Pract 34(10):2637–2644

    Google Scholar 

  10. Sanayei A, Mousavi SF, Abdi M, Mohaghar A (2008) An integrated group decision-making process for supplier selection and order allocation using multi-attribute utility theory and linear programming. J Frankl Inst 345(7):731–747

    Article  MATH  Google Scholar 

  11. Taylor AE, Mann WR (2004) Advanced calculus. Wiley, New York

    MATH  Google Scholar 

  12. Thomas GB, Weir MD, Hass J, Giordano FR (2010) Thomas’ calculus early transcendentals. Pearson, Boston

    Google Scholar 

  13. Tian Z-P, Wang J, Zhang H-Y, Wang J-Q (2016) Multi-criteria decision-making based on generalized prioritized aggregation operators under simplified neutrosophic uncertain linguistic environment. Int J Mach Learn Cybern. doi:10.1007/s13042-016-0552-9

  14. Tsao C-Y, Chen T-Y (2016) A projection-based compromising method for multiple criteria decision analysis with interval-valued intuitionistic fuzzy information. Appl Soft Comput 45:207–223

    Article  Google Scholar 

  15. Verma R (2016) Multiple attribute group decision making based on generalized trapezoid fuzzy linguistic prioritized weighted average operator. Int J Mach Learn Cybern. doi:10.1007/s13042-016-0579-y

  16. Wang J-Q, Li K-J, Zhang H-Y (2012) Interval-valued intuitionistic fuzzy multi-criteria decision-making approach based on prospect score function. Knowl Based Syst 27:119–125

    Article  Google Scholar 

  17. Wang Q, Fang Z, Zhang N, Shi H (2015) A two-tuple linguistics muti-attribute group decision making based on bi-directional projection operator. Math Pract Theory 20:44–51

    MATH  Google Scholar 

  18. Wei G (2009a) Decision-making based on projection for intuitionistic fuzzy multiple attributes. Chin J Manag 6(9):1154–1156

    Google Scholar 

  19. Wei G (2009b) Project method for multiple attribute group decision making in two-tuple linguistic setting. Oper Res Manag Sci 18(5):59–63

    Google Scholar 

  20. Xu G, Liu F (2013) An approach to group decision making based on interval multiplicative and fuzzy preference relations by using projection. Appl Math Model 37(6):3929–3943

    Article  MathSciNet  MATH  Google Scholar 

  21. Xu Z (2005) On method for uncertain multiple attribute decision making problems with uncertain multiplicative preference information on alternatives. Fuzzy Optim Decis Mak 4(2):131–139

    Article  MathSciNet  MATH  Google Scholar 

  22. Xu Z (2008) Dependent uncertain ordered weighted aggregation operators. Inf Fusion 9(2):310–316

    Article  Google Scholar 

  23. Xu Z, Hu H (2010) Projection models for intuitionistic fuzzy multiple attribute decision making. Int J Info Tech Dec Mak 9(2):267–280

  24. Xu Z, Da Q (2004) Projection method for uncertain multi-attribute decision making with preference information on alternatives. Int J Inf Technol Decis Mak 3(03):429–434

    Article  Google Scholar 

  25. Xu Z, Hu H (2010) Projection models for intuitionistic fuzzy multiple attribute decision making. Int J Inf Technol Decis Mak 9(2):267–280

    Article  MATH  Google Scholar 

  26. Ye J (2016) Bidirectional projection method for multiple attribute group decision making with neutrosophic numbers. Neural Comput Appl 1–9. doi:10.1007/s00521-015-2123-5

  27. Yue C (2016) A geometric approach for ranking interval-valued intuitionistic fuzzy numbers with an application to group decision-making. Comput Ind Eng 102:233–245

    Article  Google Scholar 

  28. Yue C (2016) A model for evaluating software quality based on symbol information. J Guangdong Ocean Univ 36(1):85–92

    Google Scholar 

  29. Yue Z (2012) Application of the projection method to determine weights of decision makers for group decision making. Sci Iran 19(3):872–878

    Article  Google Scholar 

  30. Yue Z (2012) Approach to group decision making based on determining the weights of experts by using projection method. Appl Math Model 36(7):2900–2910

    Article  MathSciNet  MATH  Google Scholar 

  31. Yue Z (2013) An intuitionistic fuzzy projection-based approach for partner selection. Appl Math Model 37(23):9538–9551

    Article  MathSciNet  Google Scholar 

  32. Yue Z, Jia Y (2015) A direct projection-based group decision-making methodology with crisp values and interval data. Soft Comput. doi:10.1007/s00500-015-1953-5

  33. Yue Z, Jia Y (2015) A group decision making model with hybrid intuitionistic fuzzy information. Comput Ind Eng 87:202–212

    Article  Google Scholar 

  34. Zeng S, Baležentis T, Chen J, Luo G (2013) A projection method for multiple attribute group decision making with intuitionistic fuzzy information. Informatica 24(3):485–503

    MathSciNet  MATH  Google Scholar 

  35. Zhang J, Wu D, Olson D (2005) The method of grey related analysis to multiple attribute decision making problems with interval numbers. Math Comput Model 42(9–10):991–998

    Article  MathSciNet  MATH  Google Scholar 

  36. Zhang X, Jin F, Liu P (2013) A grey relational projection method for multi-attribute decision making based on intuitionistic trapezoidal fuzzy number. Appl Math Model 37(5):3467–3477

    Article  MathSciNet  MATH  Google Scholar 

  37. Zheng G, Jing Y, Huang H, Gao Y (2010) Application of improved grey relational projection method to evaluate sustainable building envelope performance. Appl Energy 87(2):710–720

    Article  Google Scholar 

Download references

Acknowledgements

The author would like to thank the editors and the anonymous reviewers for their insightful and constructive comments and suggestions that have led to this improved version of the paper. This work was partially supported by the Education and Teaching Reform Program of Guangdong Ocean University (XJG201644).

Author information

Authors and Affiliations

  1. College of Mathematics and Computer Science, Guangdong Ocean University, Zhanjiang, 524088, China

    Chuan Yue

Authors
  1. Chuan Yue
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Chuan Yue.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yue, C. Normalized projection approach to group decision-making with hybrid decision information. Int. J. Mach. Learn. & Cyber. 9, 1365–1375 (2018). https://doi.org/10.1007/s13042-017-0650-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13042-017-0650-3

Keywords