Abstract
In a continuous-time model with uncertain market development, two potential entrants detect a nascent demand only if it reaches a firm-specific threshold. Entry occurs by investing irreversibly before competing in quantities. When leadership in the investment stage implies a first-mover advantage in the market stage, we examine how the firms’ relative “alertness” drives the equilibrium outcomes. If the firms detect the new demand relatively late, the entry strategies and resulting firm values differ qualitatively from those in standard real option games: (1) In case of symmetric detection, the probability of simultaneous entry is nonzero, and can be one, although demand is still nascent. When sequential entry occurs, there is no rent equalization, with the post-entry market advantage, resulting in higher equilibrium expected value to the leader; (2) in case of asymmetric detection, entry is always sequential, and the more alert firm maximizes value by delaying its investment to enter exactly when its short-sighted rival detects demand. The marginal effect of the market advantage on the leader’s equilibrium value increases in the inter-firm alertness differential; and (3) more demand volatility reduces the value differential across firms and makes less likely the impact of imperfect alertness on entry decisions.




Similar content being viewed by others
Notes
Although in the late nineties independent pioneers started supplying commercial music services online, a large majority of downloads were sourced for free, including from unauthorized sites and file-sharing services (see [33]).
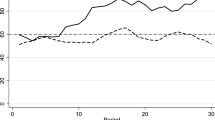
Source: [34, p. 3].
Figure 1 is constructed by using two sources from the Recording Industry Association of America (RIAA) that describe year-end US revenue statistics. The data estimate the retail dollar value of music downloads as a percentage of the total value of all formats, including on-demand streaming from 2011 onwards.
See the transcript of the press conference from Steve Jobs concerning the first anniversary of the iTunes Music Store, dated April 29, 2004, available from: http://www.macobserver.com.
Biglaiser et al. [4] also refer to the market for music downloads to motivate their formal investigation of the role of heterogeneous switching costs.
The geometric Brownian motion is derived from \(Y_{t}=Y_{0}\exp \left[ \left( \alpha -\frac{1}{2}\sigma ^{2}\right) t+\sigma Z_{t}\right] \) by using Itô’s lemma. For the expected first-hitting time by the state variable of a given threshold to be finite, it is assumed that \(\alpha >\frac{\sigma ^{2} }{2}\).
I am grateful to Francisco Ruiz-Aliseda for hinting at this specification.
Toward more realism, with no consequence for the analysis that follows, \( \delta \) could also be defined as a decreasing function of \(\underline{y}\), with \( \delta (Y_{0})\ge \delta (\bar{Y})=0\), so that detection is symmetric if it occurs at \(\bar{Y}\) when demand is relatively high. Alternatively, \(\delta \) could be specified as non-deterministic and be chosen by nature, provided that firm f, as soon as it is aware of the new demand, continuously monitors its less alert rival and thus can observe accurately when \(-f\) detects as well.
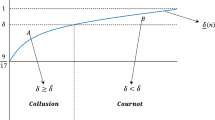
See “Appendix 1” for a formal definition.
The parameters \(\phi \), \(\gamma \), and \(\lambda \) refer (phonetically) to “follower,” “Cournot,” and “leader,” respectively.
The ranking of instantaneous profits is rooted in the quantity-setting firms assumption. Indeed (3) does not occur in a price-setting duopoly with standard specifications, where the slope of best-reply functions is positive, resulting in a second-mover advantage, as first demonstrated by [27]. For a real options investment model with price competition in the market stage, see [10].
In real-world circumstances, a first-mover advantage is likely to erode over time (see [16] for historical evidence). Therefore, the firms’ equilibrium values, as derived in the present setting, should be seen as reference levels vis-à-vis more realistic situations in which the first investor’s superiority is only temporary.
In [14] a condition is established on the inverse demand function for the Stackelberg and Cournot equilibria to coincide, in which case \(\phi =\gamma =\lambda \) so there is no advantage for a firm to take the lead.
If \(r\le \alpha \), value is maximized by postponing entry forever. In (4), the term \(\left( \frac{y}{y_{F}^{*}}\right) ^{\beta }\) reads as the expected discounted value, measured when \(Y_{t}=y\), of receiving one monetary unit at the first-hitting time \(\tau _{F}^{*}\doteq \min \{t\ge 0:Y_{t}\ge y_{F}^{*}\}\). As \(\beta \) is the positive root of the “fundamental quadratic” \(\alpha \beta +\frac{1}{2}\sigma ^{2}\beta (\beta -1)-r=0\), if \(\sigma =0\) (no uncertainty) \(\beta =\frac{r}{\alpha }\), hence \(\left( \frac{y}{y_{F}^{*} }\right) ^{\beta }=e^{-r\left( \tau _{F}^{*}-t\right) }\), the usual discounting term. For a detailed exposition of the steps that lead to the expression of \(\beta \), see [18, pp. 140–144].
The function \(F_{y_{F}}(y)\doteq \left( \frac{y}{y_{F}}\right) ^{\beta }\left( \frac{\pi _{F}}{r-\alpha }y_{F}-I\right) \) is concave in \(y_{F}\) if and only if \(y_{F}<\left( 1+\frac{1}{\beta }\right) y_{F}^{*}\), a condition satisfied at \(y_{F}^{*}\), and is monotone decreasing in \( y_{F}>y_{F}^{*}\), with \(\lim _{y_{F}\rightarrow \infty }F_{y_{F}}(y)=0\), all \(\beta >1\), \(r>\alpha \). Whenever \(y>y_{F}^{*}\), the follower thus maximizes value by entering at y.
In Sect. 5, the property that \(L_{y_{L}}(y)\) is concave in \(y_{L}\) for all \(y_{L}<\left( 1+\frac{1}{\beta }\right) y_{L}^{*}\) is useful to identify the maximizer in case of asymmetric detection.
The subscript p refers to “preemption”.
Here \(\tau _{F}^{*}\doteq \min \{t\ge 0:Y_{t}\ge y_{F}^{*}\}\), and we have \(y_{F}^{*} = \hat{y}_{F}^{*}\), hence \(\tau _{F}^{*} = \hat{\tau }_{F}^{*}\), if and only if \(\phi = \gamma \), all \(\lambda \ge \phi \).
To compare, see [11, Chapter 12] for a similar analysis of the probability of sequential or simultaneous entry by Cournot competitors, though with ex ante asymmetric marginal costs, for any initial state of the stochastic process.
See, in particular, the illustrative investment model in Section 4 of [50], where uncertainty is driven by a Lévy process, and duopoly profits are symmetric.
With asymmetric instantaneous profits as in the present model, it is shown in [5] that simultaneous entry is jointly optimal for the two firms relative to sequential investment if \(Y_{0}\) is above a threshold \(Y^{*}(>\tilde{y})\).
The assumption that \(\Delta t\) is positive (detection precedes entry), though arbitrarily close to zero (no time to build), here works as a tie-breaking device. This is reminiscent of [28] who “divide each time t into two ‘moments’, \(t^{-}\) and \( t^{+} \)” (in the words of [24], p. 396). Here everything happens as if the less alert firm \(-f\) detects demand at \(\bar{\tau }^{-}\), and thus can possibly enter only at \(\bar{\tau }^{+}\), or later. The more alert firm f, which detected demand earlier, remains protected from preemption when it enters at \(\bar{\tau }^{-}\). However, at each other time beyond \(\bar{\tau }\), the firms are both alert and able to move simultaneously, so we need considering mixed strategies (see “Appendix 1”).
As the derivations in this section are relatively straightforward, they are left aside for space limitation. They are available from the author upon request.
The negative sign of (11) and consequently the claim that \( y_{p}\) increases with \(\sigma \) hold for \(\lambda \le 1\), as assumed in (3). When \(\lambda >1\), which may occur when the follower’s investment is assumed to be highly beneficial to the leader (positive externality), in [41] it is found that more uncertainty can lower the leader’s trigger point.
An increase in \(\sigma \) does not change the level of \(Y_{t}\) at which the leader enters until \(y_{p}\) (which does depend on \(\sigma \)) reaches \(\bar{y} \) from below, in which case one gets back to a non-constrained preemption equilibrium.
Here the specification that dt approaches zero is necessary for \(Y_{t}\), and the associated payoffs of \(\Gamma \), to remain almost constant in each of the repeated rounds until one of the two firms invests. Following [7], for any \(\epsilon ,\eta >0\), there exists \(\nu >0\) such that the probability of having \(\left| Y_{t+\mathrm{d}t}-Y_{t}\right| >\epsilon \) is lower than \(\eta \) for d\(t<\) \(\nu \). In that case, \(Y_{s}\) is assumed to remain in the \(\epsilon \)-neighborhood of \( Y_{t}\) for all s in \([t+\mathrm{d}t]\).
\(V^{f}(0,0)\) results from \(\lim _{\alpha _{t}^{f}=\alpha _{t}^{-f}\rightarrow 0}\frac{\alpha _{t}^{f}\alpha _{t}^{-f}S(y)+\alpha _{t}^{f}(1-\alpha _{t}^{-f})L(y)+(1-\alpha _{t}^{f})\alpha _{t}^{-f}F^{*}\left( y\right) }{ \alpha _{t}^{f}+\alpha _{t}^{-f}-\alpha _{t}^{f}\alpha _{t}^{-f}}=\frac{ L(y)+F^{*}\left( y\right) }{2}.\)
References
Arewa OB, Sharpe NF (2007) Is apple playing fair? Navigating the iPod FairPlay DRM controversy. Northwest J Technol Intell Prop 5(2):331–349
Azevedo A, Paxson D (2014) Developing real option game models. Eur J Oper Res 237(3):909–920
Barney J, Wright M, Ketchen D (2001) The resource-based view of the firm: ten Years after 1991. J Manag 27(6):625–641
Biglaiser G, Crémer J, Dobos G (2010) The Value of Switching Costs. IDEI Working Paper 596
Billette de Villemeur E, Ruble R, Versaevel B (2013) Caveat preemptor: coordination failure and success in a duopoly investment game. Econ Lett 118(2):250–254
Bouis R, Huisman KJM, Kort PM (2009) Investment in oligopoly under uncertainty: the Accordion effect. Int J Ind Organ 27(2):320–331
Boyarchenko S, Levendorskii S (2011) Preemption Games under Lévy Uncertainty. mimeo
Boyer M, Gravel E, Lasserre P (2010) Real options and strategic competition: a survey of the different modeling strategies. mimeo
Boyer M, Lasserre P, Moreaux M (2012) A dynamic duopoly investment game without commitment under uncertain market expansion. Int J Ind Organ 30(6):663–681
Boyer M, Lasserre P, Mariotti T, Moreaux M (2004) Preemption and rent dissipation under price competition. Int J Ind Organ 22(3):309–328
Chevalier-Roignant B, Trigeorgis L (2011) Competitive strategy: options and games. The MIT Press, Cambridge
Chevalier-Roignant B, Huchzermeier A, Trigeorgis L (2010) Market entry sequencing under uncertainty. mimeo
Chevalier-Roignant B, Flath Ch, Huchzermeier A, Trigeorgis L (2011) Strategic investment under uncertainty: a synthesis. Eur J Oper Res 215(3):639–650
Colombo L, Labrecciosa P (2008) When Stackelberg and Cournot Equilibria Coincide. BE J Theor Econ (Topics) 8(1), Article 1
Cottrell TJ, Sick GA (2002) Real options and follower strategies: the loss of real option value to first-mover advantage. Eng Econ 47(3):232–263
Cottrell TJ, Sick GA (2005) First-mover (Dis)advantage and real options. J Appl Corp Finance 14(2):41–51
Décamps J-P, Mariotti T (2004) Investment timing and learning externalities. J Econ Theory 118(1):80–102
Dixit AK, Pindyck RS (1994) Investment under uncertainty. Princeton University Press, Princeton
Driver C, Temple P, Urga G (2008) Real options—delay vs. pre-emption: Do Industrial Characteristics Matter? Int J Ind Organ 26(3):532–545
Folta TB, Miller KD (2002) Real options in strategic management. Strateg Manag J 23(7):655–665
Folta TB, O’Brien JP (2004) Entry in the presence of Dueling options. Strateg Manag J 25(2):121–138
Folta TB, Johnson DR, O’Brien JP (2006) Uncertainty, irreversibility, and the likelihood of entry: an empirical assessment of the option to defer. J Econ Behav Organ 61(3):432–452
Foss NJ, Klein PG (2009) Entrepreneurial alertness and opportunity discovery: origins, attributes, critique. SMG Working Paper 2–09, Copenhagen Business School, Denmark
Fudenberg D, Tirole J (1985) Preemption and rent equalization in the adoption of a new technology. Rev Econ Stud 52(170):383–401
Fudenberg D, Tirole J (1987) Understanding rent dissipation: on the use of game theory in industrial organization. Am Econ Rev 77(2):176–183
Fudenberg D, Tirole J (1991) Game theory. The MIT Press, Cambridge
Gal-Or E (1985) First-mover and second-mover advantages. Int Econ Rev 26(3):649–653
Gilbert RJ, Harris RG (1984) Competition with lumpy investment. RAND J Econ 15(2):197–212
Grenadier SR (1996) The strategic exercise of options: development cascades and overbuilding in real estate markets. J Finance 51(5):1653–1679
Helfat CE, Lieberman MB (2002) The birth of capabilities: market entry and the importance of pre-history. Ind Corp Change 11(4):725–760
Huisman KJM (2001) Technology investment: a game theoretic real options approach. Kluwer, Dortrecht
Huisman KJM, Kort PM (1999) Effects of Strategic Interactions on the Option Value of Waiting. CentER DP No. 9992, Tilburg University
IFPI (2002) The Recording Industry World Sales 2001. http://www.ifpi.org/content/library/worldsales2001
IFPI (2005) Digital Music Report. http://www.ifpi.cz/wp-content/uploads/2013/03/Digital-Music-Report-2005
Kim J, Lee C-Y (2011) Technological regimes and the persistence of first-mover advantages. Ind Corp Change 20(5):1305–1333
Kirzner IM (1973) Competition and entrepreneurship. University of Chicago Press, Chicago
Kirzner IM (1979) Discovery and the capitalist process. University of Chicago Press, Chicago
Kirzner IM (1997) Entrepreneurial discovery and the competitive market process: an Austrian approach. J Econ Lit 35(1):60–85
Koski H, Kretschmer T (2004) Survey on competing in network industries: firm strategies, market outcomes, and policy implications. J Ind Compet Trade 4(1):5–31
Lambrecht B, Perraudin W (2003) Real options and preemption under incomplete information. J Econ Dyn Control 27(4):619–643
Mason R, Weeds H (2010) Investment, uncertainty, and pre-emption. Int J Ind Organ 28(3):278–287
Nerkar A, Roberts PW (2004) Technological and product-market experience and the success of new product introductions in the pharmaceutical industry. Strateg Manag J 25(8–9):779–799
Pawlina G, Kort P (2006) Real options in an asymmetric duopoly: Who benefits from your competitive disadvantage? J Econ Manag Strategy 15(1):1–35
Posner RA (1975) The social costs of monopoly and regulation. J Polit Econ 83(4):807–827
Prescott EC, Visscher M (1977) Sequential location among firms with foresight. Bell J Econ 8(2):378–393
Stone B, Vance A (2009) Apple’s obsession with secrecy grows stronger. The New York Times, June 22
Smit HTJ, Trigeorgis L (2004) Strategic investment: real options and games. Princeton University Press, Princeton
Thijssen JJJ (2012) Preemption in a real option game with first mover advantage and player-specific uncertainty. J Econ Theory 145(6):2448–2462
Thijssen JJJ, Huisman KJM, Kort PM (2002) Symmetric equilibrium strategies in game theoretic real option models. CentER DP No. 81, Tilburg University
Thijssen JJJ, Huisman KJM, Kort PM (2012) Symmetric equilibrium strategies in game theoretic real option models. J Math Econ 48:219–225
Zaheer A, Zaheer S (1997) Catching the wave: alertness, responsiveness, and market influence in global electronic networks. Manage Sci 43(11):1493–1509
Author information
Authors and Affiliations
Corresponding author
Additional information
This paper was partly written while the author was visiting ESMT (Berlin), whose hospitality is warmly acknowledged. I am grateful to two anonymous referees for their most useful suggestions, and to Francis Bidault, Marcel Boyer, Michèle Breton, Jean-Paul Décamps, Emmanuel Dechenaux, Vianney Dequiedt, Timothy Folta, Tobias Kretschmer, François Larmande, Pierre Lasserre, Leonard Mirman, Jean-Pierre Ponssard, Francisco Ruiz-Aliseda, Lenos Trigeorgis, Jean-Philippe Tropeano, and Mike Wright for comments or help in various other forms. The paper benefited from presentations in London (Real Options Group), Lyon (French Finance Association), Paris (PSE IO seminar), Toronto (Canadian Economics Association), and Toulouse (European Association for Research in Industrial Economics). Special thanks are due to Etienne Billette de Villemeur, Benoit Chevalier-Roignant, Olivier Le Courtois, and Richard Ruble for numerous discussions and feedbacks, and to Karim Aroussi for research assistance. All remaining errors are mine.
Electronic supplementary material
Below is the link to the electronic supplementary material.
Appendices
The first formal treatment of preemption appears in [24] in a deterministic framework, and extended in [32] to a stochastic environment. This appendix is adapted from the recent contribution by [12]. For a more general approach, see [50].
Appendix 1: Strategies
The first formal treatment of preemption appears in [24] in a deterministic framework, and extended in [32] to a stochastic environment. This appendix is adapted from the recent contribution by [12]. For a more general approach, see [50].
The information set for each firm is \(h_{t}\doteq \left( Y_{t},(i,j)_{t}\right) \). The state variables \(Y_{t}\ge 0\) and \( (i,j)_{t}\in \{0,1\}^{2}\) describe the industrywide shock and the firms’ entry status, respectively (\(i=0\) if firm f has not entered, and \(i=1\) otherwise, while \(j=0\) if \(-f\) has not entered, and \(j=1\) otherwise).
Given a history \(h_{t}\) of state variables, denote by \(A^{f}\left( h_{t}\right) \), the set of firm f’s pure actions available at any date t that follows detection by both firms (that is, after \(\min \{\tau ^{f},\tau _{L}^{*},\bar{\tau }\}\)). As a firm faces only two possible actions in the investment stage (it may invest or wait), for firm f, we have \( A^{f}\left( h_{t}\right) =\{\)invest, wait\(\}\) if \(i=0\), all j, and \(A^{f}\left( h_{t}\right) =\{\)wait\(\}\) otherwise. The investment process stops as soon as both firms have entered. Then, denote by \(\left( \alpha ^{f}\left( h_{t}\right) ,1-\alpha ^{f}\left( h_{t}\right) \right) \in [0,1]^{2}\), a probability distribution over the elements of \( A^{f}\left( h_{t}\right) \), with \(\alpha ^{f}\left( h_{t}\right) \) defined as the probability of investing at time t (with symmetric notations for firm \(-f\)).
At any t, firm f chooses a behavioral investment strategy:
Definition 1
A behavioral investment strategy of firm f at time t is a map which assigns to each history \(h_{t}\) of state variables a probability distribution \(\left( \alpha ^{f}\left( h_{t}\right) ,1-\alpha ^{f}\left( h_{t}\right) \right) \) over the elements of \(A^{f}\left( h_{t}\right) \).
For simplicity, in what follows, we slightly abuse terminology by referring to \(\alpha _{t}^{f}\doteq \alpha ^{f}\left( h_{t}\right) \) as firm f’s strategy at time t.
To characterize the chosen strategies, suppose that no firm has invested yet at t and consider a \(2\times 2\) game \(\Gamma \) in which each firm can choose either to invest or to wait.
In strategic form:

In the strategic-form representation of \(\Gamma \), the function \( V^{f}:[0,1]^{2}\rightarrow R_{+}^{1}\) describes firm f’s present expected value of replaying the same game as a function of the two firms’ respective probabilities of investing, \(\alpha _{t}^{f}\) and \(\alpha _{t}^{-f}\). With continuous time, the game can be repeated infinitely many times over the interval \([t+\mathrm{d}t]\), with d\(t\rightarrow 0\).Footnote 30 It follows that, whenever \((\alpha _{t}^{f},\alpha _{t}^{-f})\ne (0,0)\), one of the two firms invests with probability 1.
In this game, firm f’s expected payoff is given by the recursive expression
where \(F^{*}(y)\), L(y), and S(y) are defined in (4), (5), and (6). Here the displayed expression of \(V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})\) is firm f’s value for a history \(h_{t}\), with a symmetric expression for \(-f\). With a Markov refinement, only the current state levels are used, for any information set, in the definition of the value functions \(F^{*}(y)\), L(y), and S(y). This leads to the following solution concept:
Definition 2
At any \(t\ge 0\) , given \(h_{t}\), a pair of behavioral strategies \((\hat{\alpha } _{t}^{f},\hat{\alpha }_{t}^{-f})\) is a Markov Nash equilibrium of \( \Gamma \) if \(V^{f}(\hat{\alpha }_{t}^{f},\hat{\alpha }_{t}^{-f})\ge V^{f}(\alpha _{t}^{f},\hat{\alpha }_{t}^{-f})\) and \(V^{-f}(\hat{ \alpha }_{t}^{f},\hat{\alpha }_{t}^{-f})\ge V^{-f}(\hat{\alpha } _{t}^{f},\alpha _{t}^{-f})\), all \(\alpha _{t}^{f},\alpha _{t}^{-f}\) .
For subgame perfection, the condition must hold at each point in time.
Definition 3
A collection of behavioral strategies \(\{(\hat{\alpha }_{t}^{f},\hat{\alpha } _{t}^{-f})\}_{t\ge 0}\) is a subgame perfect Markov Nash equilibrium if for every \(t\in [0,\infty )\) the pair of behavioral strategies \((\hat{\alpha }_{t}^{f},\hat{\alpha }_{t}^{-f})\) is a Markov Nash equilibrium of \(\Gamma \).
The next step is to compute equilibrium strategies. After a reorganization of terms in the expression of firm f’s expected payoff, define
which describes firm f’s objective for a given \(h_{t}\) as a function of its own strategy \(\alpha _{t}^{f}\) and of the rival’s strategy \(\alpha _{t}^{-f}\).Footnote 31
The first-order derivative of \(V^{f}\) in \(\alpha _{t}^{f}\) is
with a symmetric expression for \(-f\). The sign of the derivative in (15) is the same as the sign of the numerator, which depends on the comparison of S(y) with L(y), and of L(y) with \(F^{*}(y)\). If the numerator is zero, the value \(V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})\) does not depend on \(\alpha _{t}^{f}\), and there is no cost to specify that firm f plays the same strategy as what is obtained when the numerator is either negative or positive.
We already know from Lemma 2 that \(S(y)<(=)F^{*}(y)\) if and only \(y<(=) \tilde{y}\in \left( y_{p},y_{F}^{*}\right) \), and from the definitions in (5, 6) that \(S(y)\le L(y)\) for all y (the market profit to the leader is \(\pi _{10}\ge \hat{\pi }\) when \(y<y_{F}^{*}\) and is followed by \(\pi _{L}\ge \hat{\pi }\) when \(y\ge y_{F}^{*}\), while the profit is \(\hat{\pi }\) all along in case of simultaneous entry). It remains to consider the partition of the support of y in three intervals, as follows.
- Case 1: :
-
\(y<y_{p}\). We know from above that \(S(y)\le L(y)\), and from (5) and (6) that \(L\left( y\right) <F^{*}\left( y\right) \). It follows from (15) that \(\frac{\partial V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}<0\) and \(\frac{\partial V^{-f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}<0\), for all \((\alpha _{t}^{f},\alpha _{t}^{-f})\), implying that f and \(-f\) maximize \(V^{f}\) and \(V^{-f}\), respectively, by choosing the lowest possible probability of investing. Therefore, the unique equilibrium is \(\hat{\alpha } _{t}^{f}=\hat{\alpha }_{t}^{-f}=0\).
- Case 2: :
-
\(y_{p}\le y<y_{F}^{*}\). Again \(S(y)\le L(y)\) for all \( y\le y_{F}^{*}\), and we know from (5-6) that \(L\left( y\right) >\left( =\right) F^{*}\left( y\right) \) if \(y_{p}<\left( =\right) y<y_{F}^{*}\). Therefore, the sign of the derivative in (15) is a priori indeterminate. There are now two situations that depend on the level of y relative to \(\tilde{y}\).
First, if \(y<\left( =\right) \tilde{y}\), we have \(S\left( y\right) <\left( =\right) F^{*}\left( y\right) \) from Lemma 2. Moreover, either \( y_{p}\le y<\tilde{y}<y_{F}^{*}\) and \(S\left( y\right) <L\left( y\right) \), or \(y_{p}<y=\tilde{y}<y_{F}^{*}\) and \(S\left( y\right) =F^{*}\left( y\right) <L\left( y\right) \), so in both cases \(S\left( y\right) <L\left( y\right) \). This leads to three possibilities that depend on the level of \(\alpha _{t}^{-f}\):
-
(i)
Suppose that \(0\le \alpha _{t}^{-f}<\frac{L(y)-F^{*}\left( y\right) }{L(y)-S(y)}\), implying that \(\frac{\partial V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}\ge 0\), for all \( \alpha _{t}^{f}\). Then firm f maximizes \(V^{f}\) by choosing \(\alpha _{t}^{f}=1\). Hence \(\frac{\partial V^{-f}(1,\alpha _{t}^{-f})}{\partial \alpha _{t}^{-f}}=S(y)-F^{*}\left( y\right) <\left( =\right) 0\) for all \( y<\left( =\right) \tilde{y}\), so that \(-f\) maximizes \(V^{-f}\) with \(\alpha _{t}^{-f}=0\), and the solution is asymmetric.
-
(ii)
Suppose that \(\frac{L(y)-F^{*}\left( y\right) }{L(y)-S(y)} <\alpha _{t}^{-f}\le 1\), implying that \(\frac{\partial V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}<0\), for all \(\alpha _{t}^{f}\). Then firm f maximizes \(V^{f}\) by choosing \(\alpha _{t}^{f}=0\). This implies in turn that \(\frac{\partial V^{-f}(0,\alpha _{t}^{-f})}{ \partial \alpha _{t}^{-f}}=0\), for all \(\alpha _{t}^{-f}>0\) sufficiently high to satisfy the initial supposition, leading to an asymmetric solution.
-
(iii)
The last possibility is \(\alpha _{t}^{-f}=\frac{L(y)-F^{*}\left( y\right) }{L(y)-S(y)}\), implying that \(\frac{\partial V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}=0\), all \(\alpha _{t}^{f}\in [0,1]\). The latter continuum renders admissible the unique symmetric strategy
$$\begin{aligned} \hat{\alpha }_{t}^{f}=\hat{\alpha }_{t}^{-f}=\frac{L(y)-F^{*}(y)}{L(y)-S(y) }, \end{aligned}$$(16)so that firm values are \(V^{f}(\hat{\alpha }_{t}^{f},\hat{\alpha } _{t}^{-f})=V^{-f}(\hat{\alpha }_{t}^{f},\hat{\alpha }_{t}^{-f})=L(y)=F^{*}(y)>S(y)\).
Only in (iii) we have symmetry. From here on, we focus on this case. Then (16) implies that \(\hat{\alpha }_{t}^{f}=\hat{\alpha }_{t}^{-f}\) describes a nonzero instantaneous probability of simultaneous entry for all \( y\in (y_{p},y_{F}^{*})\), with \(\lim _{y\rightarrow y_{p}}\hat{\alpha } _{t}^{f}=\lim _{y\rightarrow y_{p}}\hat{\alpha }_{t}^{-f}=0\), and \(\left. \hat{ \alpha }_{t}^{f}\right| _{y=\tilde{y}}=\left. \hat{\alpha }_{t}^{-f}\right| _{y=\tilde{y}}=1\).
Second, if \(y>\tilde{y}\), \(S(y)>F^{*}\left( y\right) \) implies \(\alpha _{t}^{-f}\left( S(y)-L(y)\right) +L(y)-F^{*}\left( y\right) >0\), so that \(\frac{\partial V^{f}(\alpha _{t}^{f},\alpha _{t}^{-f})}{\partial \alpha _{t}^{f}}\ge 0\) from (15), all \(\alpha _{t}^{-f}\ge 0\). Then firm f maximizes \(V^{f}\) with \(\alpha _{t}^{f}=1\). Hence, \(\frac{ \partial V^{-f}(1,\alpha _{t}^{-f})}{\partial \alpha _{t}^{-f}}=S(y)-F^{*}\left( y\right) >0\), so that \(-f\) maximizes \(V^{-f}\) with \(\alpha _{t}^{-f}=1\), leading to a symmetric solution:
- Case 3: :
-
\(y_{F}^{*}\le y\). From (4), the follower enters at y “immediately after” the leader (hence the flow profit term remains \(\pi _{F}\le \pi _{L}\)), and \(F^{*}(y)\le S(y)\le L(y)\), with equality only if instantaneous profits are symmetric (\(\phi =\gamma =\lambda \)). Therefore, as in the previous case (with \(y>\tilde{y}\)), the symmetric solution is (17).
Apeendix 2: Proof of Lemma 1
The value function \(F^{*}(y)\), which is strictly increasing with y, is also strictly convex as
all \(y>0\), while L(y) is strictly (weakly) concave if and only if \(\pi _{L}<(=)\pi _{10}\) because
all \(y>0\). It follows that \(F^{*}(y)-L(y)=0\) may admit up to two roots. Consider first the benchmark case \(\phi =\lambda \) (Cournot), for which \( \hat{y}_{F}^{*}\doteq \left. y_{F}^{*}\right| _{\phi =\lambda }\) is a root. Then \(\hat{F}^{*}(0)=0>\hat{L}(0)=-I\), and
together are sufficient to conclude that there exists another positive root \( \hat{y}_{p}<(=)\hat{y}_{F}^{*}\) for any \(\gamma <(=)1\). Then, consider the more general case \(\phi \le \lambda \). Define \({\mathcal {F}}\doteq F^{*}-\hat{F}^{*}\) and \({\mathcal {L}}\doteq L-\hat{L}\), to compare the slopes of \(F^{*}(y)\) and L(y) with the slopes of \(\hat{F}^{*}(y)\) and \(\hat{L}(y)\), respectively. As \(\beta >1\), we find
if \(\phi <\gamma \), and
if \(\gamma <\lambda \), all \(y>0\). In the Stackelberg scenario, the slope of the second entrant’s value function is strictly lower than in Cournot, whereas the slope of the first entrant’s value function is higher. Together with \(F^{*}\left( 0\right) =\hat{F}^{*}\left( 0\right) =0\) and \( L\left( 0\right) =\hat{L}\left( 0\right) =-I\), this leads to the conclusion that \(F^{*}\) and L intersect at a lower y than \(\hat{F}^{*}\) and \(\hat{L}\). This establishes that \(y_{p}<\hat{y}_{p}\). In addition, it is sufficient to check that \(F^{*}(y_{F}^{*})<\lim _{y\uparrow y_{F}^{*}}L(y)\) when \(\phi <\lambda \) to conclude that \(F^{*}\) and L cannot intersect for any \(y>y_{p}\) on which the value functions are defined. \(\square \)
Appendix 3: Proof of Lemma 2
Set \(\phi =\gamma \). From (4) and (6), the slope of \(F^{*}(y)\) is (weakly) lower than the slope of S(y) for all \(y<\left( =\right) y_{F}^{*}\) (with \(\phi /\gamma =1\) implying \(y_{F}^{*}=\hat{y} _{F}^{*}\) here). Moreover, \(F^{*}(0)=0>S(0)=-I\) and \(F^{*}(y_{F}^{*})=S(y_{F}^{*})\) imply that \(F^{*}(y)>\left( =\right) S(y)\) for all \(y<\left( =\right) y_{F}^{*}\). It is immediate to check that for all \(y\le y_{F}^{*}\), (i) the slope of \(F^{*}(y)\) is monotone decreasing when \(\phi \) departs from \(\gamma \) toward 0, while S(y) is unchanged, and (ii) the slope of S(y) is monotone increasing when \(\gamma \) departs from \(\phi \) toward 1, while \(F^{*}(y)\) is unchanged. It follows that there exists a unique \(\tilde{y}\) in \( (0,y_{F}^{*}]\) such that \(F^{*}(y)>\left( =\right) S(y)\) if and only if \(y<\left( =\right) \tilde{y}\), where \(\tilde{y}\) is strictly increasing in \(\phi \) and decreasing in \(\gamma \), and with \(\lim _{\phi /\gamma \uparrow 1}\tilde{y}=\hat{y}_{F}^{*}\).
Next, observe from (5) that \(L^{*}(y)\) is strictly concave, and from (6) that S(y) is linear, for all \(y<\left( =\right) y_{F}^{*}\), so that \(L^{*}(y)-S(y)=0\) has at most two roots. We know that \(L^{*}(0)=S(0)\), and from (4–6) that \(L^{*}(y_{F}^{*})>(=)S(y_{F}^{*})\) if and only if \(\lambda /\gamma >(=)1\) . Therefore, \(L^{*}(y)>S(y)\), all \(y\in (0,y_{F}^{*})\), including \( \tilde{y}\), hence \(L^{*}(\tilde{y})>S(\tilde{y})=F^{*}(\tilde{y})\). Then on the same interval \((0,y_{F}^{*})\), it is sufficient to recall that \(L^{*}(y)>F^{*}(y)\) if and only if \(y>y_{p}\) to obtain \(\tilde{y }>y_{p}\). Finally, \(F^{*}(y)\) is monotone in \(\phi \) with \(\lim _{\phi \downarrow 0}F^{*}(y)=0\), and \(S\left( y\right) \) remains constant, so when \(\phi \) approaches 0 the root \(\tilde{y}\) converges to the solution to \(S\left( y\right) =0\), that is \(\frac{r-\alpha }{\hat{\pi }}I\). \(\square \)
Appendix 4: Proof of Proposition 3
The probability that only one firm, say f, enters as a leader, while \(-f\) waits and enters as a follower, is given by \(p_{L}^{f}=\alpha _{t}^{f}\left( 1-\alpha _{t}^{-f}\right) +\left( 1-\alpha _{t}^{f}\right) \left( 1-\alpha _{t}^{-f}\right) p_{L}^{f}\). For all \((\alpha _{t}^{f},\alpha _{t}^{-f})\ne (0,0)\), a reorganization of terms gives
Next, the probability of simultaneous entry is \(p_{S}=\alpha _{t}^{f}\alpha _{t}^{-f}+\left( 1-\alpha _{t}^{f}\right) \left( 1-\alpha _{t}^{-f}\right) p_{S}\). For all \((\alpha _{t}^{f},\alpha _{t}^{-f})\ne (0,0)\), a reorganization of terms gives
\(\square \)
In a symmetric equilibrium \(\alpha _{t}=\alpha _{t}^{f}=\alpha _{t}^{-f}\), (23) and (24) simplify to \(p_{L}^{f}=\frac{ 1-\alpha _{t}}{2-\alpha _{t}}\) and \(p_{S}=\frac{\alpha _{t}}{2-\alpha _{t}}\) . It remains to plug in the latter expressions the equilibrium strategies \( \left( \hat{\alpha }_{t}^{f},\hat{\alpha }_{t}^{-f}\right) \) derived in Appendix 1. Again there are two cases that depend on the level of y relative to \(\tilde{y}:\)
-
If \(y_{p}\le y<\tilde{y}\), the symmetric equilibrium strategies \(\hat{\alpha }_{t}^{f}=\hat{\alpha }_{t}^{-f}=\frac{ L(y)-F^{*}(y)}{L(y)-S(y)}\) in (16) give
$$\begin{aligned} p_{L}^{f}(y)=\frac{F^{*}(y)-S(y)}{L(y)+F^{*}(y)-2S(y)}\qquad \text {and}\qquad p_{S}(y)=\frac{L(y)-F^{*}(y)}{L(y)+F^{*}(y)-2S(y)}. \end{aligned}$$(25)Then \(L(y_{p})=F^{*}(y_{p})\Rightarrow p_{L}^{f}(y_{p})=\frac{1}{2} >p_{S}(y_{p})=0\), and \(L(y)>F^{*}(y)\Rightarrow p_{S}(y)>0\) for all \( y\in (y_{p},\tilde{y})\).
-
If \(\tilde{y}\le y<y_{F}^{*}\), the symmetric equilibrium strategies \(\hat{\alpha }_{t}^{f}=\hat{\alpha }_{t}^{-f}=1\) in (17) lead to
$$\begin{aligned} p_{L}^{f}(y)=p_{F}^{-f}(y)=0\qquad \text {and}\qquad p_{S}(y)=1. \end{aligned}$$(26)
Next, suppose that \(Y_{t}\) reaches \(y_{S}\), with \(y_{p}<y_{S}<\tilde{y}\), at a given date \(t>0\). Immediately after detection, by continuity \( y_{p}<\lim _{\Delta t\rightarrow 0}Y_{t+\Delta t}=y_{S}<\tilde{y}\). Then from (25), we have \(\lim _{\Delta t\rightarrow 0}p_{L}^{f}(Y_{t+\Delta t})=p_{L}^{f}(y_{S})=\frac{F^{*}(y_{S})-S(y_{S}) }{L(y_{S})+F^{*}(y_{S})-2S(y_{S})}\), and \(\lim _{\Delta t\rightarrow 0}p_{S}(Y_{t+\Delta t})=p_{S}(y_{S})=\frac{L(y_{S})-F^{*}(y_{S})}{ L(y_{S})+F^{*}(y_{S})-2S(y_{S})}\), which together give (8a) and (8b) in Proposition 3. By the same token (26) leads to (9).\(\square \)
Rights and permissions
About this article
Cite this article
Versaevel, B. Alertness, Leadership, and Nascent Market Dynamics. Dyn Games Appl 5, 440–466 (2015). https://doi.org/10.1007/s13235-015-0163-1
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13235-015-0163-1