Skip to main content
SpringerLink
Log in

A new effective coherent numerical technique based on shifted Vieta–Fibonacci polynomials for solving stochastic fractional integro-differential equation

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

Abstract

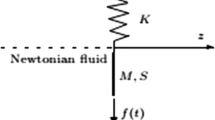
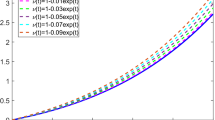
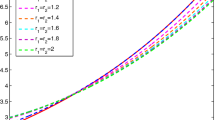
In this article, an operational matrix method based on shifted Vieta–Fibonacci polynomials is utilised to find the numerical solution of fractional order stochastic integro-differential equations. In this method, the operational matrices are developed by using the shifted Vieta–Fibonacci polynomials for the fractional order Caputo differential operator in order to solve the present concerned problem. Using Newton cotes nodes as collocation points, operational matrices are employed to convert the above-mentioned equation into a system of linear algebraic equations. The coherent procedure for the appropriate numerical technique is described in this article. Additionally, the convergence analysis and error bound of the suggested method are well established. In order to illustrate the effectiveness, consistency, plausibility, and reliability of the proposed technique, three numerical examples are given. Moreover, the results obtained by the proposed method have been compared with those obtained by the Chelyshkov operational matrix method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data Availability

This article includes all the data that were generated or analyzed during this research.

References

  • Agarwal P, El-Sayed AA, Tariboon J (2021) Vieta–Fibonacci operational matrices for spectral solutions of variable-order fractional integro-differential equations. J Comput Appl Math 382:113063

    Article  MathSciNet  MATH  Google Scholar 

  • Ahmad B, Nieto JJ (2011) Riemann-Liouville fractional integro-differential equations with fractional nonlocal integral boundary conditions. Bound Value Probl 1:1–9

    MathSciNet  MATH  Google Scholar 

  • Arikoglu A, Ozkol I (2009) Solution of fractional integro-differential equations by using fractional differential transform method. Chaos Solitons Fractals 40(2):521–529

    Article  MathSciNet  MATH  Google Scholar 

  • Atman KG, Şirin H (2020) Nonlocal phenomena in quantum mechanics with fractional calculus. Rep Math Phys 86(2):263–270

    Article  MathSciNet  MATH  Google Scholar 

  • Behera S, Saha Ray S (2022) An efficient numerical method based on Euler wavelets for solving fractional order pantograph Volterra delay-integro-differential equations. J. Comput. Appl. Math. 406:113825

    Article  MathSciNet  MATH  Google Scholar 

  • El-Misiery AEM, Ahmed E (2006) On a fractional model for earthquakes. Appl Math Comput 178(2):207–211

    MathSciNet  MATH  Google Scholar 

  • Kamrani M (2016) Convergence of Galerkin method for the solution of stochastic fractional integro differential equations. Optik 127(20):10049–10057

    Article  Google Scholar 

  • Lodge AS, McLeod JB, Nohel JA (1978) A nonlinear singularly perturbed Volterra integrodifferential equation occurring in polymer rheology. Proc R Soc Edinb Sect A Math 80(1–2):99–137

    Article  MATH  Google Scholar 

  • Machado JT, Lopes AM (2015) Analysis of natural and artificial phenomena using signal processing and fractional calculus. Fract Calc Appl Anal 18:459–478

    Article  MathSciNet  MATH  Google Scholar 

  • Meral FC, Royston TJ, Magin R (2010) Fractional calculus in viscoelasticity: an experimental study. Commun Nonlinear Sci Numer Simul 15(4):939–945

    Article  MathSciNet  MATH  Google Scholar 

  • Mirzaee F, Alipour S (2020) Cubic B-spline approximation for linear stochastic integro-differential equation of fractional order. J Comput Appl Math 366:112440

    Article  MathSciNet  MATH  Google Scholar 

  • Mirzaee F, Samadyar N (2017) Application of orthonormal Bernstein polynomials to construct a efficient scheme for solving fractional stochastic integro-differential equation. Optik 132:262–273

    Article  Google Scholar 

  • Mirzaee F, Samadyar N (2019) On the numerical solution of fractional stochastic integro-differential equations via meshless discrete collocation method based on radial basis functions. Eng Anal Bound Elem 100:246–255

    Article  MathSciNet  MATH  Google Scholar 

  • Mirzaee F, Alipour S, Samadyar N (2019) Numerical solution based on hybrid of block-pulse and parabolic functions for solving a system of nonlinear stochastic Itô-Volterra integral equations of fractional order. J Comput Appl Math 349:157–171

    Article  MathSciNet  MATH  Google Scholar 

  • Momani S, Odibat Z (2006) Analytical approach to linear fractional partial differential equations arising in fluid mechanics. Phys Lett A 355(4–5):271–279

    Article  MATH  Google Scholar 

  • Øksendal B (2003) Stochastic differential equations. Springer, Berlin, pp 65–84

    Google Scholar 

  • Sadri K, Hosseini K, Baleanu D, Salahshour S, Park C (2022) Designing a matrix collocation method for fractional delay integro-differential equations with weakly singular kernels based on Vieta–Fibonacci polynomials. Fractal Fract 6(1):2

    Article  Google Scholar 

  • Saha Ray S (2015) Fractional calculus with applications for nuclear reactor dynamics. CRC Press, Boca Raton

    MATH  Google Scholar 

  • Saha Ray S, Patra A (2013) Numerical solution of fractional stochastic neutron point kinetic equation for nuclear reactor dynamics. Ann Nucl Energy 54:154–161

    Article  Google Scholar 

  • Saha Ray S, Singh P (2021) Numerical solution of stochastic Itô-Volterra integral equation by using Shifted Jacobi operational matrix method. Appl Math Comput 410:126440

    MATH  Google Scholar 

  • Sahu PK, Saha Ray S (2018) Comparison on wavelets techniques for solving fractional optimal control problems. J Vib Control 24(6):1185–1201

    Article  MathSciNet  MATH  Google Scholar 

  • Scalas E, Gorenflo R, Mainardi F (2000) Fractional calculus and continuous-time finance. Physica A Stat Mech Appl 284(1–4):376–384

    Article  MathSciNet  Google Scholar 

  • Sun H, Zhang Y, Baleanu D, Chen W, Chen Y (2018) A new collection of real world applications of fractional calculus in science and engineering. Commun Nonlinear Sci Numer Simul 64:213–231

    Article  MATH  Google Scholar 

  • Taheri Z, Javadi S, Babolian E (2017) Numerical solution of stochastic fractional integro-differential equation by the spectral collocation method. J Comput Appl Math 321:336–347

    Article  MathSciNet  MATH  Google Scholar 

  • Yzbaı S, Sezer M, Kemancı B (2013) Numerical solutions of integro-differential equations and application of a population model with an improved Legendre method. Appl Math Model 37(4):2086–2101

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This research work was financially supported by NBHM, Mumbai, under Department of Atomic Energy, Government of India vide Grant Ref. no. 02011/4/2021 NBHM(R.P.)/R &D II/6975 dated 17/06/2021.

Author information

Authors and Affiliations

  1. Department of Mathematics, National Institute of Technology, Rourkela, 769008, India

    Reema Gupta & S. Saha Ray

Authors
  1. Reema Gupta
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Saha Ray
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Saha Ray.

Additional information

Communicated by Vasily E. Tarasov.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gupta, R., Saha Ray, S. A new effective coherent numerical technique based on shifted Vieta–Fibonacci polynomials for solving stochastic fractional integro-differential equation. Comp. Appl. Math. 42, 256 (2023). https://doi.org/10.1007/s40314-023-02398-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40314-023-02398-4

Keywords

Mathematics Subject Classification

Search

Navigation