Abstract
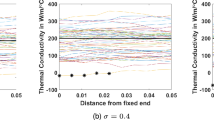
This study is devoted to find the numerical solution of the surface heat flux history and temperature distribution in a non linear source term inverse heat conduction problem (IHCP). This type of inverse problem is investigated either with a temperature over specification condition at a specific point or with an energy over specification condition over the computational domain. A combination of the meshless local radial point interpolation and the finite difference method are used to solve the IHCP. The proposed method does not require any mesh generation and since this method is local at each time step, a system with a sparse coefficient matrix is solved. Hence, the computational cost will be much low. This non linear inverse problem has a unique solution, but it is still ill-posed since small errors in the input data cause large errors in the output solution. Consequently, when the input data is contaminated with noise, we use the Tikhonov regularization method in order to obtain a stable solution. Three different kinds of schemes are applied to choose the regularization parameter which are in agreement with each other. Numerical results show that the solution is accurate for exact data and stable for noisy data.





















Similar content being viewed by others
Data availability
The data that support the findings of this study are available from the corresponding author, [Ahmad Jafarabadi], upon reasonable request.
References
Arora S, Dabas J (2019) Inverse heat conduction problem in two-dimensional anisotropic medium. Int J Appl Comput Math 5(6):161
Cannon JR (1984) The one-dimensional heat equation, vol 23. Cambridge University Press, Cambridge
Cannon JR, Du Chateau P (1998) Structural identification of an unknown source term in a heat equation. Inverse Problems 14(3):535
Dehghan M, Ghesmati A (2010) Numerical simulation of two-dimensional sine-Gordon solitons via a local weak meshless technique based on the radial point interpolation method (RPIM). Comput Phys Commun 181(4):772–786
Dehghan M, Yousefi SA, Rashedi K (2013) Ritz–Galerkin method for solving an inverse heat conduction problem with a nonlinear source term via Bernstein multi-scaling functions and cubic B-spline functions. Inverse Problems Sci Eng 21(3):500–523
Deng C, Zheng H, Fu M, Xiong J, Chen CS (2020) An efficient method of approximate particular solutions using polynomial basis functions. Eng Anal Boundary Elem 111:1–8
Fernandes AP, dos Santos MB, Guimarães G (2015) An analytical transfer function method to solve inverse heat conduction problems. Appl Math Model 39(22):6897–6914
Frankel JI, Keyhani M (1997) A global time treatment for inverse heat conduction problems. J Heat Trans 119:673–683
Grabski JK (2019) Numerical solution of non-Newtonian fluid flow and heat transfer problems in ducts with sharp corners by the modified method of fundamental solutions and radial basis function collocation. Eng Anal Boundary Elem 109:143–152
Hansen PC (1999) The L-curve and its use in the numerical treatment of inverse problems
Hao DN, Reinhardt H-J (1996) Recent contributions to linear inverse heat conduction problems. J Inverse Ill-Posed Problems 4:23–32
Hon YC, Wei T (2004) A fundamental solution method for inverse heat conduction problem. Eng Anal Boundary Elem 28(5):489–495
Hussen G et al (2022) Meshless and homotopy perturbation methods for one dimensional inverse heat conduction problem with Neumann and robin boundary conditions. J Appl Math Inf 40(3–4):675–694
Ismail S et al (2017) Meshless collocation procedures for time-dependent inverse heat problems. Int J Heat Mass Transf 113:1152–1167
Jonas P, Louis AK (2000) Approximate inverse for a one-dimensional inverse heat conduction problem. Inverse Prob 16(1):175
Kanca F, Ismailov MI (2012) The inverse problem of finding the time-dependent diffusion coefficient of the heat equation from integral overdetermination data. Inverse Problems Sci Eng 20(4):463–476
Krawczyk-Stańdo D, Rudnicki M (2007) Regularization parameter selection in discrete ill-posed problems—the use of the U-curve. Int J Appl Math Comput Sci 17(2):157–164
Ku C-Y, Liu C-Y, Xiao J-E, Hsu S-M, Yeih W (2021) A collocation method with space–time radial polynomials for inverse heat conduction problems. Eng Anal Boundary Elem 122:117–131
Lesnic D, Elliott L (1999) The decomposition approach to inverse heat conduction. J Math Anal Appl 232(1):82–98
Lesnic D, Elliott L, Ingham DB (1996) Application of the boundary element method to inverse heat conduction problems. Int J Heat Mass Transf 39(7):1503–1517
Liu J (1996) A stability analysis on Beck’s procedure for inverse heat conduction problems. J Comput Phys 123(1):65–73
Morozov VA (1966) On the solution of functional equations by the method of regularization. Dokl Akad Nauk 167:510–512
Shen S-Y (1999) A numerical study of inverse heat conduction problems. Comp Math Appl 38(7–8):173–188
Shidfar A, Karamali GR, Damirchi J (2006) An inverse heat conduction problem with a nonlinear source term. Nonlinear Anal Theory Methods Appl 65(3):615–621
Shivanian E, Jafarabadi A (2017a) Inverse Cauchy problem of annulus domains in the framework of spectral meshless radial point interpolation. Eng Comput 33(3):431–442
Shivanian E, Jafarabadi A (2017b) Numerical solution of two-dimensional inverse force function in the wave equation with nonlocal boundary conditions. Inverse Problems Sci Eng 25(12):1743–1767
Shivanian E, Jafarabadi A (2018a) The numerical solution for the time-fractional inverse problem of diffusion equation. Eng Anal Boundary Elem 91:50–59
Shivanian E, Jafarabadi A (2018b) An inverse problem of identifying the control function in two and three-dimensional parabolic equations through the spectral meshless radial point interpolation. Appl Math Comput 325:82–101
Smith GD (1985) Numerical solution of partial differential equations: finite difference methods. Oxford University Press, Oxford
Wang JG, Liu GR (2002) A point interpolation meshless method based on radial basis functions. Int J Numer Methods Eng 54(11):1623–1648
Xiong P, Deng J, Tao L, Qi L, Liu Y, Zhang Y (2020) A sequential conjugate gradient method to estimate heat flux for nonlinear inverse heat conduction problem. Ann Nucl Energy 149:107798
Yaparova N (2014) Numerical methods for solving a boundary-value inverse heat conduction problem. Inverse Problems Sci Eng 22(5):832–847
Yousefi SA, Lesnic D, Barikbin Z (2012) Satisfier function in Ritz–Galerkin method for the identification of a time-dependent diffusivity. J Inverse Ill-Posed Problems 20(5–6):701–722
Acknowledgements
The authors would like to thank the anonymous referees for their valuable comments and helpful suggestions that improve the quality of our paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Antonio José Silva Neto.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Dinmohammadi, A., Jafarabadi, A. Inverse heat conduction problem with a nonlinear source term by a local strong form of meshless technique based on radial point interpolation method. Comp. Appl. Math. 42, 284 (2023). https://doi.org/10.1007/s40314-023-02414-7
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s40314-023-02414-7
Keywords
- Meshless local radial point interpolation method
- Radial basis function
- Inverse heat conduction problem
- Surface heat flux