Abstract
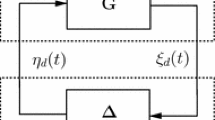
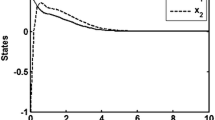
The decentralized \({\mathscr{H}}_{\infty }\) sampled-data control problem is investigated for a class of continuous-time large-scale networked nonlinear systems with interconnection. Each nonlinear subsystem in the considered large-scale system is represented by a Takagi–Sugeno model and is closed by a communication channel with transformed time delay. Our objective is to design a decentralized sampled-data fuzzy controller such that the resulting fuzzy control system is asymptotically stable with an \({\mathscr{H}}_{\infty }\) performance. Firstly, using an input delay approach, the sampled-data control system is formulated into the system with time-varying delay, and a two-term approximation method is proposed such that the delayed system is reformulated into an interconnected framework with input and output. Then, we introduce a Lyapunov–Krasovskii functional that all Lyapunov matrices are no longer required to be positive definite. Combined with the scaled small gain theorem, the less conservative solutions to the decentralized \({\mathscr{H}}_{\infty }\) sampled-data control problem for the considered system are derived in the form of linear matrix inequalities. Finally, the effectiveness of the proposed methods is illustrated by two numerical examples.







Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
Tanaka, T., Wang, H.: Fuzzy Control Systems Design and Analysis: A Linear Matrix Inequality Approach. Wiley, New York (2001)
Li, Y., Tong, S., Li, T.: Composite adaptive fuzzy output feedback control design for uncertain nonlinear strict-feedback systems with input saturation. IEEE Trans. Cybern. 45(10), 2299–2308 (2015)
Wang, Y., Zhang, H., Zhang, J., Wang, Y.: An SOS-based observer design for discrete-time polynomial fuzzy systems. Int. J. Fuzzy Syst. 17(1), 94–104 (2015)
Qiu, J., Ding, S.X., Gao, H., Yin, S.: Fuzzy-model-based reliable static output feedback \(\fancyscript {H}_{\infty }\) control of nonlinear hyperbolic PDE systems. IEEE Transactions on Fuzzy Systems. IEEE Trans. Fuzzy Syst. (2015). doi: 10.1109/TFUZZ.2015.2457934
Tong, S., Li, Y.: Adaptive fuzzy output feedback control of MIMO nonlinear systems with unknown dead-zone inputs. IEEE Trans. Fuzzy Syst. 21(1), 134–146 (2013)
Qiu, J., Feng, G., Gao, H.: Observer-based piecewise affine output feedback controller synthesis of continuous-time T–S fuzzy affine dynamic systems using quantized measurements. IEEE Trans. Fuzzy Syst. 20(6), 1046–1062 (2012)
Siljak, D.: Large-Scale Dynamic Systems: Stability and Structure. Elsevier/North-Holland, New York (1978)
Bakule, L.: Decentralized control: status and outlook. Annu. Rev. Control 38(1), 71–80 (2014)
Mahmoud, M., Qureshi, A.: Decentralized sliding-mode output-feedback control of interconnected discrete-delay systems. Automatica 48(5), 808–814 (2012)
Yu, G., Zhang, H., Huang, Q., Dang, C.: New delay-dependent stability analysis for fuzzy time-delay interconnected systems. Int. J. Gen. Syst. 42(7), 739–753 (2013)
Li, Y., Tong, S., Li, T.: Adaptive fuzzy output feedback dynamic surface control of interconnected nonlinear pure-feedback systems. IEEE Trans. Cybern. 45(1), 138–149 (2015)
Li, Y., Tong, S., Liu, Y., Li, T.: Adaptive fuzzy robust output feedback control of nonlinear systems with unknown dead zones based on a small-gain approach. IEEE Trans. Cybern. 22(1), 164–176 (2014)
Tong, S., Li, Y., Zhang, H.: Adaptive neural network decentralized backstepping output-feedback control for nonlinear large-scale systems with time delays. IEEE Trans. Neural Netw. 22(7), 1073–1086 (2011)
Wu, H.: Decentralized adaptive robust control for a class of large-scale systems including delayed state perturbations in the interconnections. IEEE Trans. Autom. Control 47(10), 1745–1751 (2002)
Zhang, H., Yu, G., Zhou, C., Dang, C.: Delay-dependent decentralized \(\fancyscript {H}_{\infty }\) filtering for fuzzy interconnected systems with time-varying delay based on Takagi–Sugeno fuzzy model. IET Control Appl. 7(5), 720–729 (2013)
Zhong, Z., Fu, S., Hayat, T., Alsaadi, F., Sun, G.: Decentralized piecewise \(\fancyscript {H}_{\infty }\) fuzzy filtering design for discrete-time large-scale nonlinear systems with time-varying delay. J. Frankl. Inst. 352(9), 3782–3807 (2015)
Zhang, H., Zhong, H., Dang, C.: Delay-dependent decentralized \(\fancyscript {H}_{\infty }\) filtering for discrete-time nonlinear interconnected systems with time-varying delay based on the T–S fuzzy model. IEEE Trans. Fuzzy Syst. 20(3), 431–443 (2012)
Tong, S., Huo, B., Li, Y.: Observer-based adaptive decentralized fuzzy fault-tolerant control of nonlinear large-scale systems with actuator failures. IEEE Trans. Fuzzy Syst. 22(1), 1–15 (2014)
Tseng, C.: A novel approach to \(\fancyscript {H}_{\infty }\) decentralized fuzzy-observer-based fuzzy control design for nonlinear interconnected systems. IEEE Trans. Fuzzy Syst. 16(5), 1337–1350 (2008)
Zhu, Y., Zhang, L., Zheng, W.: Distributed \(\fancyscript {H}_{\infty }\) filtering for a class of discrete-time Markov jump Lure systems with redundant channels. IEEE Trans. Ind. Electron. (2015) doi: 10.1109/TIE.2015.2499169
Zhu, Y., Zhang, L., Basin, M.: Nonstationary \(\fancyscript {H}_{\infty }\) dynamic output feedback control for discrete-time Markov iump linear systems with actuator and sensor saturations. Int. J. Robust Nonlinear Control (2015). doi: 10.1002/rnc.3348
Fridman, E.: A refined input delay approach to sampled-data control. Automatica 46(2), 421–427 (2010)
Zhang, J., Knopse, C., Tsiotras, P.: Stability of time-delay systems: equivalence between Lyapunov and scaled small-gain conditions. IEEE Trans. Autom. Control 46(3), 482–486 (2001)
Gu, K., Kharitonov, V., Chen, J.: Stability of Time-Delay Systems. Birkhauser, Boston (2003)
Jiang, X., Han, Q., Yu, X.: Stability criteria for linear discrete-time systems with interval-like time-varying delay. In: Proceedings of the 2005 IEEE conference on American control, vol. 4, pp. 2817–2822 (2005)
Gu, K., Zhang, Y., Xu, S.: Small gain problem in coupled differential-difference equations, time-varying delays, and direct Lyapunov method. Int. J. Robust Nonlinear Control 21(4), 429–451 (2011)
Acknowledgments
The authors are grateful to the Editor-in-Chief, the Associate Editor, and anonymous reviewers for their constructive comments based on which the presentation of this paper has been greatly improved. This work was supported in part by the Natural Science Foundation (2015J01275) of the Science and Technology Agency, Fujian, and the National Science Council of Taiwan under Grant NSC98-2221-E-155-059-MY3, and the Science and Technology-Planned Project (3502Z20143034) of Xiamen, and the Foreign Science and Technology Special Cooperation (E201401400) and (E201400900) of Xiamen University of Technology, and the Natural Foundation Pre-Research Project (XYK201402) of Xiamen University of Technology, China.
Author information
Authors and Affiliations
Corresponding authors
Rights and permissions
About this article
Cite this article
Zhao, J., Lin, CM. & Huang, J. Decentralized \(\mathscr{H}_{\infty }\) Sampled-Data Control for Continuous-Time Large-Scale Networked Nonlinear Systems. Int. J. Fuzzy Syst. 19, 504–515 (2017). https://doi.org/10.1007/s40815-016-0140-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40815-016-0140-x