Skip to main content

Advertisement

Springer Nature Link
Log in

Comprehensive Decision Making with Fuzzy Compromised Variable Weights and its Application on Maintenance Order for Entries in Underground Coal Mine

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

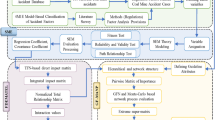
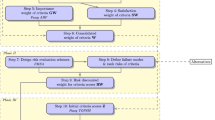
Abstract

Development of fuzzy compromised variable weight vector (VWV) and its application on fuzzy comprehensive decision making (FCDM) are studied specifically in this paper. Based on the definition of variable weight state vector (VWSV), a compromised VWSV is proposed and then further developed to obtain the fuzzy compromised VWSV. According to the algorithm of Hardarmard product, fuzzy compromised VWV is developed. A FCDM model with fuzzy compromised variable weights is developed accordingly. Fuzzy numbers and fuzzy operations are determined by using the fuzzy structured element, which is the essential part for the analytical algorithm of the FCDM model. The developed model was then applied as decision-making method on maintenance order for entries in underground coal mine. The research finding could also provide an alternative way for determination of weights and fuzzy operations in other fuzzy analysis models.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Dalkey, N., Helmer, O.: An experimental application of the DELPHI method to the use of experts. Manag. Sci. 9, 458–467 (1963). https://doi.org/10.1287/mnsc.9.3.458

    Article  Google Scholar 

  2. Milholland, A.V., Wheeler, S.G., Heieck, J.J.: Medical assessment by a Delphi group opinion technic. N. Engl. J. Med. 288, 1272–1275 (1973). https://doi.org/10.1056/NEJM197306142882405

    Article  Google Scholar 

  3. Saaty, T.L.: The Analytic Hierarchy Process. McGraw-Hill, New York (1980)

    MATH  Google Scholar 

  4. Dadeviren, M., Yuksel, I.: Developing a fuzzy analytic hierarchy process (AHP) model for behavior-based safety management. Inf. Sci. (Ny) 178, 1717–1733 (2008). https://doi.org/10.1016/j.ins.2007.10.016

    Article  Google Scholar 

  5. Wang, Y.Y., Xu, Z.S.: Evaluation of the human settlement in Lhasa with intuitionistic fuzzy analytic hierarchy process. Int. J. Fuzzy Syst. 20, 1–16 (2018). https://doi.org/10.1007/s40815-017-0422-y

    Article  MathSciNet  Google Scholar 

  6. Juang, C.H., Huang, X.H., Schiff, S.D.: Determination of weights of criteria for decision making by the fuzzy eigenvector method. Civ. Eng. Syst. 9, 1–16 (1992). https://doi.org/10.1080/02630259208970636

    Article  Google Scholar 

  7. Xu, Y.J., Wang, H.M.: Eigenvector method, consistency test and inconsistency repairing for an incomplete fuzzy preference relation. Appl. Math. Model. 37, 5171–5183 (2013). https://doi.org/10.1016/j.apm.2012.10.008

    Article  MathSciNet  MATH  Google Scholar 

  8. Xu, Y.J., Chen, L., Rodríguez, R.M., Herrera, F., Wang, H.M.: Deriving the priority weights from incomplete hesitant fuzzy preference relations in group decision making. Knowl. Based Syst. 99, 71–78 (2016). https://doi.org/10.1016/j.knosys.2016.01.047

    Article  Google Scholar 

  9. Saaty, T.L.: The analytic network process. International 195, 1–26 (1996)

    Google Scholar 

  10. Saaty, T.L.: Decision making with dependence and feedback: The analytic network process. In: RWS Publications, 1996, ISBN 0-9620317-9-8. p. 370 (1996)

  11. Erdoğmuş, Ş., Aras, H., Koç, E.: Evaluation of alternative fuels for residential heating in Turkey using analytic ne-twork process (ANP) with group decision-making. Renew. Sustain. Energy Rev. 10, 269–279 (2006). https://doi.org/10.1016/j.rser.2004.09.003

    Article  Google Scholar 

  12. Moore, J.R., Baker, N.R.: An analytical approach to scoring model design—application to research and development project selection. IEEE Trans. Eng. Manag. EM-16, pp. 90–98 (1969). https://doi.org/10.1109/tem.1969.6447060

  13. Nelson, C.A.: A scoring model for flexible manufacturing systems project selection. Eur. J. Oper. Res. 24, 346–359 (1986). https://doi.org/10.1016/0377-2217(86)90028-7

    Article  Google Scholar 

  14. Huang, M.L., Xu, X.J., Tashnev, D.: A weighted linear quantile regression. J. Stat. Comput. Simul. 85, 2596–2618 (2015). https://doi.org/10.1080/00949655.2014.938240

    Article  MathSciNet  Google Scholar 

  15. Wu, C., Yu, J.Z.: Evaluation of linear regression techniques for atmospheric applications: the importance of appropriate weighting. Atmos. Meas. Tech. 11, 1233–1250 (2018). https://doi.org/10.5194/amt-11-1233-2018

    Article  Google Scholar 

  16. Aduol, F.W.O.: Robust geodetic parameter estimation under least squares through weighting on the basis of the mean square error. In Geodesy the Challenge of the 3rd Millennium. pp. 269–276 (2003)

  17. Shannon, C.E.: A mathematical theory of communication. Bell Syst. Tech. J. 27, 379–423 (1948). https://doi.org/10.1145/584091.584093

    Article  MathSciNet  MATH  Google Scholar 

  18. Soofi, E.: Generalized entropy-based weights for multiattribute value models. Oper. Res. 38, 362–363 (1990). https://doi.org/10.1287/opre.38.2.362

    Article  MATH  Google Scholar 

  19. Ng, D.K.W., Deng, J.: Contrasting grey system theory to probability and fuzzy. ACM Sigice Bull. 20, 3–9 (1995). https://doi.org/10.1145/202081.202082

    Article  Google Scholar 

  20. Kao, P.S., Hocheng, H.: Optimization of electrochemical polishing of stainless steel by grey relational analysis. J. Mater. Process. Technol. 140, 255–259 (2003). https://doi.org/10.1016/S0924-0136(03)00747-7

    Article  Google Scholar 

  21. Liang, D.C., Kobina, A.: Quan W (2017) Grey relational analysis method for probabilistic linguistic multi-criteria group decision-making based on geometric Bonferroni mean. Int. J. Fuzzy Syst. 20, 1–11 (2017). https://doi.org/10.1007/s40815-017-0374-2

    Google Scholar 

  22. Pawlak, Z.: Rough sets. Int. J. Comput. Inf. Sci. 11, 341–356 (1982). https://doi.org/10.1007/BF01001956

    Article  MATH  Google Scholar 

  23. Son, C.S., Kim, Y.N., Kim, H.S., Park, H.S., Kim, M.S.: Decision-making model for early diagnosis of congestive heart failure using rough set and decision tree approach-es. J. Biomed. Inform. 45, 999–1008 (2012). https://doi.org/10.1016/j.jbi.2012.04.013

    Article  Google Scholar 

  24. Oztaysi, B.: A decision model for information technology selection using AHP integrated TOPSIS-Grey: the case of content management systems. Knowl. Based Syst. 70, 44–54 (2014). https://doi.org/10.1016/j.knosys.2014.02.010

    Article  Google Scholar 

  25. Wang, Y.S., Jin, Z.X., Deng, C.B., Wang, X.Y.: Comprehensive decision-making with fuzzy combined weighting and its application on the order of gob management. J. Intell. Fuzzy Syst. 34, 2641–2649 (2018). https://doi.org/10.3233/JIFS-17700

    Article  Google Scholar 

  26. Liu, B.S., Huo, T.F., Liao, P.C., Yuan, J.F., Sun, J., Hu, X.: A special Partial Least Squares (PLS) path decision modeling for bid evaluation of large construction projects. KSCE J. Civ. Eng. 21, 579–592 (2017). https://doi.org/10.1007/s12205-016-0702-3

    Article  Google Scholar 

  27. Liu, B.S., Shen, Y.H., Chen, X.H., Sun, H., Chen, Y.: A complex multi-attribute large-group PLS decision-making method in the interval-valued intuitionistic fuzzy environment. Appl. Math. Model. 38, 4512–4527 (2014). https://doi.org/10.1016/j.apm.2014.02.023

    Article  MathSciNet  MATH  Google Scholar 

  28. Xu, Y.J., Patnayakuni, R., Wang, H.M.: Logarithmic least squares method to priority for group decision making with incomplete fuzzy preference relations. Appl. Math. Model. 37, 2139–2152 (2013). https://doi.org/10.1016/j.apm.2012.05.010

    Article  MathSciNet  MATH  Google Scholar 

  29. Li, H.X.: Multifactorial functions in fuzzy sets theory. Fuzzy Sets Syst. 35, 69–84 (1990). https://doi.org/10.1016/0165-0114(90)90019-3

    Article  MathSciNet  MATH  Google Scholar 

  30. Wang, P.Z.: Fuzzy sets and the falling shadow of random sets. Beijing Normal University Press, Beijing (1985)

    Google Scholar 

  31. Li, H.X., Yen, V.C.: Fuzzy sets and fuzzy decision-making. Crc Press. (1995)

  32. Li, H.X., Li, L.X., Wang, J.Y., Mo, Z.W., Li, Y.D.: Fuzzy decision making based on variable weights. Math. Comput. Model. 39, 163–179 (2004). https://doi.org/10.1016/S0895-7177(04)90005-2

    Article  MathSciNet  MATH  Google Scholar 

  33. Zeng, W.Y., Li, D.Q., Wang, P.Z.: Variable weight decision making and balance function analysis based on factor space. Int. J. Inf. Technol. Decis. Mak. 15, 999–1014 (2016). https://doi.org/10.1142/S021962201650022X

    Article  Google Scholar 

  34. Li, D.Q., Zeng, W.Y., Li, J.H.: Balance function analysis in variable weight decision making. In: Proceedings—2014 10th International Conference on Computational Intelligence and Security, CIS 2014 (2015). https://doi.org/10.1109/cis.2014.61

  35. Li, D.Q., Hao, F.L.: Weights transferring effect of state variable weight vector. Syst. Eng. Pract. 29, 127–131 (2009). https://doi.org/10.1016/S1874-8651(10)60054-3

    Article  Google Scholar 

  36. Wu, Q., Li, B., Chen, Y.L.: Vulnerability assessment of groundwater inrush from underlying aquifers based on variable weight model and its application. Water Resour. Manag. 30, 3331–3345 (2016). https://doi.org/10.1007/s11269-016-1352-4

    Article  Google Scholar 

  37. Nagurney, A., Ke, K.: Financial networks with intermediation: risk management with variable weights. Eur. J. Oper. Res. 172, 40–63 (2006). https://doi.org/10.1016/j.ejor.2004.09.035

    Article  MathSciNet  MATH  Google Scholar 

  38. Zeng, X.Z., Fang, Z.F.: Comprehensive evaluation of blasting effects based on variable weight method and research on fuzzy decision. Adv. Mater. Res. 402, 610–616 (2012). https://doi.org/10.4028/www.scientific.net/AMR.402.610

    Article  Google Scholar 

  39. Zhang, J.H., Tian, C.F., Fang, W., Zhang, N.N.: Assessment on operational risk in power grid enterprises based on variable weight fuzzy evaluation. In: 2009 International Conference on Energy and Environment Technology, ICEET 2009. pp. 92–95 (2009). https://doi.org/10.1109/iceet.2009.259

  40. Li, L., Xie, L.J., Zhang, D., Yu, B., Ge, Y.F., Lin, F.C.: Condition assessment of power transformers using a synthetic analysis method based on association rule and variable weight coefficients. IEEE Trans. Dielectr. Electr. Insul. 20, 2052–2060 (2013). https://doi.org/10.1109/TDEI.2013.6678853

    Article  Google Scholar 

  41. Liu, C.: Research on safety assessment method for bridge structure based on variable weight synthesis method. Perspect. Sci. 7, 200–203 (2016). https://doi.org/10.1016/j.pisc.2015.11.033

    Article  Google Scholar 

  42. Zhang, R.F., Huang, L.F., Xiao, M.B.: Security evaluation for wireless network based on fuzzy-AHP with variable weight. In: 2010 Second International Conference on Networks Security, Wireless Communications and Trusted Computing. pp. 490–493 (2010). https://doi.org/10.1109/nswctc.2010.122

  43. Zhang, S.Z., Zeng, Q.D., Zhang, G.: A new approach for prioritization of failure mode in FMECA using encouragement variable weight AHP. Appl. Mech. Mater. 289, 93–98 (2013). https://doi.org/10.4028/www.scientific.net/AMM.289.93

    Article  Google Scholar 

  44. Hu, J.H., Yang, Y., Guo, S.Z.: Fuzzy number intuitionist-ic fuzzy set and its representation of structured element. Presented at the (2008). https://doi.org/10.1109/iita.workshops.2008.131

  45. Guo, S.Z.: Comparison and ordering of fuzzy numbers b-ased on method of structured element. Syst. Eng. Theory Pract. 29, 106–111 (2009). https://doi.org/10.1016/S1874-8651(10)60013-0

    Article  Google Scholar 

  46. Guo, S.C., Zhao, Y.: Transform group of monotonic functions with the same monotonicity on [− 1, 1] and operations of fuzzy numbers. Ann. Data Sci. 2, 281–291 (2015). https://doi.org/10.1007/s40745-015-0055-7

    Article  Google Scholar 

  47. Wang, H.D., Guo, S.C., Bamakan, S.M.H., Shi, Y.: Homeomorphism problems of fuzzy real number space and the space of bounded functions with same monotonicity on [− 1,1]. Int. J. Comput. Commun. Control 10, 889–903 (2015). https://doi.org/10.1007/978-3-319-24474-7_11

    Google Scholar 

Download references

Acknowledgements

The authors would like to appreciate editors and reviewers for their constructive comments and suggestions. The research described in this paper was financially supported by National Natural Science Foundation of China (51704145 and 51604144). The authors would like also to thank for all the supports to publish this paper.

Author information

Authors and Affiliations

  1. College of Mining Engineering, Liaoning Technical University, Fuxin, 123000, Liaoning, China

    Yansheng Wang, Zhixin Jin, Xinyang Wang & Xun Zhang

  2. Shanxi Coking Coal Refco Group Ltd, Taiyuan, 030024, Shanxi, China

    Zhixin Jin

  3. College of Safety Science and Engineering, Liaoning Technical University, Fuxin, 123000, Liaoning, China

    Cunbao Deng & Xi Chen

  4. Institute of Safety Engineering and Technology, Liaoning Technical University, Fuxin, 123000, Liaoning, China

    Cunbao Deng & Xi Chen

Authors
  1. Yansheng Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Zhixin Jin
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Cunbao Deng
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Xinyang Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. Xi Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

  6. Xun Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yansheng Wang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, Y., Jin, Z., Deng, C. et al. Comprehensive Decision Making with Fuzzy Compromised Variable Weights and its Application on Maintenance Order for Entries in Underground Coal Mine. Int. J. Fuzzy Syst. 21, 1379–1388 (2019). https://doi.org/10.1007/s40815-019-00638-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-019-00638-6

Keywords