Abstract
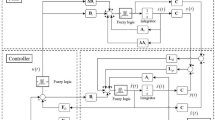
This paper investigates the design problem of the proportional-plus-derivative state feedback fuzzy controller for the Takagi–Sugeno fuzzy singular systems, which considering the influence of internal perturbations and external noises. To solve the perturbation problem in the control system simply and concisely, the benefit of employing a robust control approach is proposed. Considering the perturbed Takagi–Sugeno fuzzy singular systems with external noises, the Lyapunov stability theory is chosen to derive the stability conditions with passivity constraints. To bring better transient performance for the controlled systems, the decay rate constraint is also adopted. These sufficient stability conditions can be effectively transferred into the linear matrix inequality problem. Finally, an example is used to verify the proposed robust fuzzy controller design method’s applicability and effectivity.





Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Takagi, T., Takayuki, L., Wang, H.O.: Fuzzy regulators and fuzzy observers: relaxed stability conditions and LMI-based designs. IEEE Trans. Fuzzy Syst. 6(2), 250–265 (1998)
Yeh, J., Su, S.F.: Efficient approach for RLS type learning in TSK neural fuzzy systems. IEEE Trans. Cybern. 47(9), 2343–2352 (2017)
Chang, W.J., Ku, C.C.: Robust fuzzy control for uncertain stochastic time-delay Takagi-Sugeno fuzzy models for achieving passivity. Fuzzy Sets Syst. 161(15), 2012–2032 (2010)
Uang, H.J.: On the dissipativity of nonlinear systems: fuzzy control approach. Fuzzy Sets Syst. 156(2), 185–207 (2005)
Liu, X., Zhang, Q.: Approaches to quadratic stability conditions and H∞ control designs for TS fuzzy systems. IEEE Trans. Fuzzy Syst. 11(6), 830–839 (2003)
Chiang, W.L., Chen, T.W., Liu, M.Y., Hsu, C.J.: Application and robust H∞ control of PDC fuzzy controller for nonlinear systems with external disturbance. J. Mar. Sci. Technol. 9(2), 84–90 (2001)
K. R. Lee, T. J. Eun, and B. P. Hong, “Robust fuzzy H∞ control for uncertain nonlinear systems via state feedback: an LMI approach,” Fuzzy sets and systems, Vol. 120, Vo. 1, pp. 123-134, 2001
Chang, X.H., Liu, Y.: Robust H∞ filtering for vehicle sideslip angle with quantization and data dropouts. IEEE Trans. Veh. Technol. 69(10), 10435–10445 (2020)
Chang, X.H., Yang, G.: Nonfragile H∞ filter design for T-S fuzzy systems in standard form. IEEE Trans. Industr. Electron. 61(7), 3448–3458 (2014)
Tanaka, K., Wang, H.O.: Fuzzy Control Systems Design and Analysis: A Linear Matrix Inequality Approach. Wiley, New York (2001)
Chang, W.J., Hsu, F.L.: Sliding mode fuzzy control for Takagi-Sugeno fuzzy systems with bilinear consequent part subject to multiple constraints. Inf. Sci. 327, 258–271 (2016)
Rongchang, L., Yang, Y.: Fault detection for TS fuzzy singular systems via integral sliding modes. J. Franklin Inst. 357(17), 13125–13143 (2020)
Zhenghong, J., Zhang, Q., Ren, J.: The approximation of the T-S fuzzy model for a class of nonlinear singular systems with impulses. Neural Comput. Appl. 32(14), 10387–10401 (2020)
Lu, G., Ho, D.W.C.: Generalized quadratic stability for continuous-time singular systems with nonlinear perturbation. IEEE Trans. Autom. Control 51(5), 818–823 (2006)
Huang, C.P.: Stability analysis of discrete singular fuzzy systems. Fuzzy Sets Syst. 151(1), 155–165 (2005)
Xu, S., Song, B., Lu, J., Lam, J.: Robust stability of uncertain discrete-time singular fuzzy systems. Fuzzy Sets Syst. 158(20), 2306–2316 (2007)
Liu, P., Yang, W.T., Yang, C.E.: Robust observer-based output feedback control for fuzzy descriptor systems. Expert Syst. Appl. 40(11), 4503–4510 (2013)
Huang, C.P.: Stability analysis and controller synthesis for fuzzy descriptor systems. Int. J. Syst. Sci. 44(1), 23–33 (2013)
Ren, J., Zhang, Q.: Simultaneous robust normalization and delay-dependent robust H∞ stabilization for singular time-delay systems with uncertainties in the derivative matrices. Int. J. Robust Nonlinear Control 25(18), 3528–3545 (2015)
Ding, Y., Weng, F.: Robust decentralized stabilization of large-scale singular systems via proportional-plus-derivative state feedback. Int. Conf. Adv. Comput. Theory Eng. (ICACTE) 4, 320–324 (2010)
Kwon, W., Kang, D., Park J., Won, S.: Decentralized guaranteed cost control for uncertain large-scale singular systems via proportional-plus-derivative state feedback. Asian Control Conference (ASCC), pp. 1-6, 2015
Lozano, R., Brogliato, B., Egeland, O., Maschke, B.: Dissipative Systems Analysis and Control Theory and Application. Springer, London (2000)
Chang, W.J., Chang, Y.C., Ku, C.C.: Passive fuzzy control via fuzzy integral Lyapunov function for nonlinear ship drum-boiler systems. ASME 137(4), 15 (2015)
Chang, W.J., Chen, P.H., Ku, C.C.: Mixed sliding mode fuzzy control for discrete-time nonlinear stochastic systems subject to variance and passivity constraints. IET Control Theory Appl. 9(16), 2369–2376 (2015)
Chang, W.J., Chen, P.H., Ku, C.C.: Variance and passivity constrained sliding mode fuzzy control for continuous stochastic nonlinear systems. Neurocomputing 201, 29–39 (2016)
Li, Q., Zhang, Q., Yi, N., Yuan, Y.: Robust passive control for uncertain time-delay singular systems. IEEE Trans. Circuits Syst. I Regul. Pap. 56(3), 653–663 (2008)
Sun, Y.G., Xu, J.Q., Chen, C., Lin, G.B.: Fuzzy H∞ robust control for magnetic levitation system of maglev vehicles based on TS fuzzy model: design and experiments. J. Intell. Fuzzy Syst. 36(2), 911–922 (2019)
Xu, J., Fang, H., Zhou, T., Chen, Y.H., Guo, H., Zeng, F.: Optimal robust position control with input shaping for flexible solar array drive system: a fuzzy-set theoretic approach. IEEE Trans. Fuzzy Syst. 27(9), 1807–1817 (2019)
Nguyen, N.T.: Lyapunov Stability Theory. Springer, Cham (2018)
Mu, Y., Zhang, H., Sun, S., Ren, J.: Robust non-fragile proportional plus derivative state feedback control for a class of uncertain Takagi-Sugeno fuzzy singular systems. J. Franklin Inst. 356(12), 6208–6225 (2019)
Mu, Y., Zhang, H., Sun, J., Ren, J.: Proportional plus derivative state feedback controller design for a class of fuzzy descriptor systems. Int. J. Syst. Sci. 50(12), 2249–2260 (2019)
Zhang, G., Xia, J., Zhang, B., Sun, W.: Robust normalisation and P-D state feedback control for uncertain singular Markovian jump systems with time-varying delays. IET Control Theory Appl. 12(3), 419–427 (2018)
Acknowledgements
This work was supported by the National Science Council of the Republic of China under Contract MOST109-2221-E-019-049. It was also supported by the University System of Taipei Joint Research Program under Contract USTP-NTUT-NTOU-109-03.
Author information
Authors and Affiliations
Corresponding authors
Rights and permissions
About this article
Cite this article
Chang, WJ., Lian, KY., Su, CL. et al. Multi-constrained Fuzzy Control for Perturbed T–S Fuzzy Singular Systems by Proportional-Plus-Derivative State Feedback Method. Int. J. Fuzzy Syst. 23, 1972–1985 (2021). https://doi.org/10.1007/s40815-021-01096-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40815-021-01096-9