Skip to main content

Advertisement

Springer Nature Link
Log in

Interval Type-3 Fuzzy Differential Evolution for Parameterization of Fuzzy Controllers

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

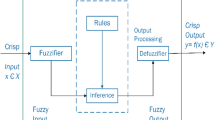
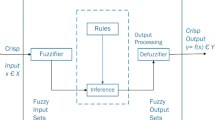
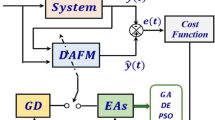
Fuzzy logic has been successfully utilized in a plethora of disciplines, and this article is presenting, for the first time, the utilization of interval type-3 fuzzy sets in the differential evolution (DE) algorithm. Type-3 fuzzy theory is a recently new proposal in the literature, and for this work, a study is carried out by varying an important element of interval type-3 fuzzy sets, called the LowerScale (λ) parameter, which is varied to create different fuzzy systems that are used to dynamically move a parameter of differential evolution during execution with the goal of improving its convergence. Experiments with benchmark functions and motor control optimization were undertaken to test the proposed type-3 differential evolution approach. This work aims to find out how the variation of the LowerScale (λ) influences the results on the two different presented case studies. Simulation results demonstrate that interval type-3 in combination with DE outperforms the type-1 and interval type-2 variants of DE, previously proposed in the literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Mendel, J.M.: On computing the similarity of trapezoidal fuzzy sets using an automated area method. Inf. Sci. (2022)

  2. Zhao, Y., Zhang, Q., Li, Z., Xin, X., Gao, R., Chai, F., Yang, M.: Dynamic routing, modulation, and spectrum assignment based on fuzzy logic control in elastic optical networks. Appl. Opt. 61(1), 223–230 (2022)

    Article  Google Scholar 

  3. Jovanović, A., Teodorović, D.: Type-2 fuzzy logic based transit priority strategy. Expert Syst. Appl. 187, 115875 (2022)

    Article  Google Scholar 

  4. Wang, J.W., Zhang, Q.Y.: Using parallel algorithm to speedup the rules learning process of a type-2 fuzzy logic system. In: Proceedings of 2021 Chinese Intelligent Systems Conference, pp. 141–151. Springer, Singapore (2022)

  5. Peinado, J., Ochoa, A., Paiva, S.: Implementation of an intelligent model based on big data and decision-making using fuzzy logic type-2 for the car assembly industry in an industrial estate in Northern Mexico. In: Immersive Technology in Smart Cities, pp. 107–122. Springer, Cham (2022)

  6. Cao, Y., Mohammadzadeh, A., Tavoosi, J., Mobayen, S., Safdar, R., Fekih, A.: A new predictive energy management system: deep learned type-2 fuzzy system based on singular value decommission. Energy Rep. 8, 722–734 (2022)

    Article  Google Scholar 

  7. Ma, B., Wang, Y., Chen, G.: Event-triggered type-2 fuzzy-based sliding mode control for steer-by-wire systems. Mechatronics 82, 102704 (2022)

    Article  Google Scholar 

  8. Santhosh, R., Mohanapriya, M.: Generalized fuzzy logic based performance prediction in data mining. Mater. Today 45, 1770–1774 (2021)

    Google Scholar 

  9. Amini, M.R., Azar, A., Eskandari, H., Wanke, P.F.: A generalized fuzzy Multiple-Layer NDEA: an application to performance-based budgeting. Appl. Soft Comput. 100, 106984 (2021)

    Article  Google Scholar 

  10. Saraswat, R.N., Khatod, N.: Generalized fuzzy divergence measure, pattern recognition, and inequalities. Int. J. Fuzzy Syst. Appl. 11(1), 1–22 (2022)

    Google Scholar 

  11. Castillo, O., Ochoa, P., Soria, J.: Fuzzy logic systems. In: Differential Evolution Algorithm with Type-2 Fuzzy Logic for Dynamic Parameter Adaptation with Application to Intelligent Control, pp. 5–8. Springer, Cham (2021)

  12. Tian, M.W., Mohammadzadeh, A., Tavoosi, J., Mobayen, S., Asad, J.H., Castillo, O., Várkonyi-Kóczy, A.R.: A deep-learned type-3 fuzzy system and its application in modeling problems. Acta Polytechnica Hungarica 19(2), 151–172 (2022)

    Article  Google Scholar 

  13. Liu, Z., Mohammadzadeh, A., Turabieh, H., Mafarja, M., Band, S.S., Mosavi, A.: A new online learned interval type-3 fuzzy control system for solar energy management systems. IEEE Access 9, 10498–10508 (2021)

    Article  Google Scholar 

  14. Ganthia, B.P., Barik, S.K., Nayak, B.: Hardware in loop (THIL 402) validated type-I fuzzy logic control of type-III wind turbine system under transients. J. Electr. Syst. 17(1), 28–51 (2021)

    Google Scholar 

  15. Mohammadzadeh, A., Castillo, O., Band, S.S., Mosavi, A.: A novel fractional-order multiple-model type-3 fuzzy control for nonlinear systems with unmodeled dynamics. Int. J. Fuzzy Syst. 1–19 (2021)

  16. Aly, A.A., Felemban, B.F., Mohammadzadeh, A., Castillo, O., Bartoszewicz, A.: Frequency regulation system: a deep learning identification, type-3 fuzzy control and LMI stability analysis. Energies 14(22), 7801 (2021)

    Article  Google Scholar 

  17. Samala, R.K., Rosalina, K.M.: Optimal allocation of multiple photo-voltaic and/or wind-turbine based distributed generations in radial distribution system using hybrid technique with fuzzy logic controller. J. Electr. Eng. Technol. 16(1), 101–113 (2021)

    Article  Google Scholar 

  18. Kabat, S.R., Panigrahi, C.K., Ganthia, B.P.: Implementation and analysis of mathematical modeled drive train system in type III wind turbines using computational fluid dynamics. Adv. Sci. Technol. Res. J. (2022)

  19. Selvachandran, G., Quek, S.G., Thong, P.H., Vo, B., Hawari, T.A.A., Salleh, A.R.: Relations and compositions between interval-valued complex fuzzy sets and applications for analysis of customers’ online shopping preferences and behavior. Appl. Soft Comput. 114, 108082 (2022)

    Article  Google Scholar 

  20. Deveci, M., Simic, V., Karagoz, S., Antucheviciene, J.: An interval type-2 fuzzy sets based Delphi approach to evaluate site selection indicators of sustainable vehicle shredding facilities. Appl. Soft Comput. 118, 108465 (2022)

    Article  Google Scholar 

  21. Mesiar, R., Kolesárová, A., Senapati, T.: Aggregation on lattices isomorphic to the lattice of closed subintervals of the real unit interval. Fuzzy Sets Syst (2022)

  22. Rani, P., Mishra, A.R.: Interval-valued fermatean fuzzy sets with multi-criteria weighted aggregated sum product assessment-based decision analysis framework. Neural Comput. Appl. 1–17 (2022)

  23. Pant, M., Kumar, S.: Particle swarm optimization and intuitionistic fuzzy set-based novel method for fuzzy time series forecasting. Granul. Comput. 7(2), 285–303 (2022)

    Article  Google Scholar 

  24. Huidobro, P., Alonso, P., Janiš, V., Montes, S.: Convexity and level sets for interval-valued fuzzy sets. Fuzzy Optim. Decis. Making 1–28 (2022)

  25. Bernal, E., Lagunes, M.L., Castillo, O., Soria, J., Valdez, F.: Optimization of type-2 fuzzy logic controller design using the GSO and FA algorithms. Int. J. Fuzzy Syst. 23(1), 42–57 (2021). https://doi.org/10.1007/s40815-020-00976-w

    Article  Google Scholar 

  26. Khaparde, A.R., Alassery, F., Kumar, A., Alotaibi, Y., Khalaf, O.I., Pillai, S., Alghamdi, S.: Differential evolution algorithm with hierarchical fair competition model. Intell. Autom. Soft Comput. 33(2), 1045–1062 (2022)

    Article  Google Scholar 

  27. Abdelkader, E.M., Moselhi, O., Marzouk, M., Zayed, T.: An exponential chaotic differential evolution algorithm for optimizing bridge maintenance plans. Autom. Constr. 134, 104107 (2022)

    Article  Google Scholar 

  28. Peng, Y., He, S., Sun, K.: Parameter identification for discrete memristive chaotic map using adaptive differential evolution algorithm. Nonlinear Dyn. 107(1), 1263–1275 (2022)

    Article  Google Scholar 

  29. He, Y., Zhang, F., Mirjalili, S., Zhang, T.: Novel binary differential evolution algorithm based on Taper-shaped transfer functions for binary optimization problems. Swarm Evol. Comput. 69, 101022 (2022)

    Article  Google Scholar 

  30. He, L., Cao, Y., Li, W., Cao, J., Zhong, L.: Optimization of energy-efficient open shop scheduling with an adaptive multi-objective differential evolution algorithm. Appl. Soft Comput. 118, 108459 (2022)

    Article  Google Scholar 

  31. Ma, X., Zhang, K., Zhang, L., Wang, Y., Wang, H., Wang, J., Yao, J.: A distributed surrogate system assisted differential evolutionary algorithm for computationally expensive history matching problems. J. Petrol. Sci. Eng. 210, 110029 (2022)

    Article  Google Scholar 

  32. Castillo, O., Melin, P., Ontiveros, E., Peraza, C., Ochoa, P., Valdez, F., Soria, J.: A high-speed interval type 2 fuzzy system approach for dynamic parameter adaptation in metaheuristics. Eng. Appl. Artif. Intell. 85, 666–680 (2019)

    Article  Google Scholar 

  33. Castillo, O., Valdez, F., Peraza, C., Yoon, J.H., Geem, Z.W.: High-speed interval type-2 fuzzy systems for dynamic parameter adaptation in harmony search for optimal design of fuzzy controllers. Mathematics 9(7), 758 (2021)

    Article  Google Scholar 

  34. Mancilla, A., García-Valdez, M., Castillo, O., Merelo-Guervós, J.J.: Optimal fuzzy controller design for autonomous robot path tracking using population-based metaheuristics. Symmetry 14(2), 202 (2022). https://doi.org/10.3390/sym14020202

    Article  Google Scholar 

  35. Melin, P., Miramontes, I., Carvajal, O., Prado-Arechiga, G.: Fuzzy dynamic parameter adaptation in the bird swarm algorithm for neural network optimization. Soft Comput. (2022). https://doi.org/10.1007/s00500-021-06729-7

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Tijuana Institute of Technology, Tijuana, Mexico

    Patricia Ochoa, Oscar Castillo & Patricia Melin

  2. UABC University, Tijuana, Mexico

    Juan R. Castro

Authors
  1. Patricia Ochoa
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Oscar Castillo
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Patricia Melin
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Juan R. Castro
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Oscar Castillo.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ochoa, P., Castillo, O., Melin, P. et al. Interval Type-3 Fuzzy Differential Evolution for Parameterization of Fuzzy Controllers. Int. J. Fuzzy Syst. 25, 1360–1376 (2023). https://doi.org/10.1007/s40815-022-01451-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-022-01451-4

Keywords