Cross-Platform Distributed Product Online Ratings Aggregation Approach for Decision Making with Basic Uncertain Linguistic Information

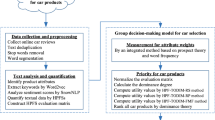
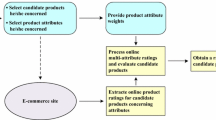
Abstract

The research on decision making driven by product rankings faces challenges due to the rise of extensive positive reviews and the widespread distribution of electronic word of mouth (eWOM) across multiple platforms. There is a limited body of research that examines the impact of platform credibility on the quality of product rankings. Hence, based on the basic uncertain linguistic information (BULI), which enables simultaneous representation of information and its credibility, we investigate the development of a ratings aggregation approach for cross-platform distribution (CPD) with the aim of facilitating decision-making processes, focusing specifically on the aspect of credibility. To begin with, this paper introduces the concept of BULI as a means to effectively represent both product ratings and their corresponding levels of credibility. Subsequently, we proceeded to devise the BULI-based aggregation functions that are well suited for the aggregation of CPD ratings and that can be degraded to the existing operator. In addition, we develop a credibility evaluation index system and credibility calculation model for the platform in order to derive a product BULI matrix consisting of ratings and their corresponding levels of credibility. In this study, we propose two models, namely the feature information-based user weighting model and the BULI distance measure-based technique for order preference by similarity to an ideal solution (BULI-TOPSIS) model, to enhance the product ratings aggregation approach for decision-making purposes. The utilization of the proposed method is exemplified through the case study of passenger car ranking, showcasing its practicality and efficiency.

Appendices

Appendix A

The following presents the BULI matrix for Step 4.5 within subsection 6.1.

$$\begin{aligned} \begin{array}{l} BULI - UT = \\ \left[ {\begin{array}{*{20}{c}} {\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 5 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 1 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 1 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 2 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle } \end{array}} \right. \\ \left. {\begin{array}{*{20}{c}} {\left\langle {{\Delta _S}\left( 4 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.2} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,0.4} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 3 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.8} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.6} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 4 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,1} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,1} \right\rangle }\\ {\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 3 \right) ,0.6} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 4 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,0.2} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 2 \right) ,0.4} \right\rangle }&{}{\left\langle {{\Delta _S}\left( 1 \right) ,0.8} \right\rangle } \end{array}} \right] \end{array} \end{aligned}$$

Appendix B

The following presents the BULI matrices for Step 6.3 within subsection 6.1.

$$\begin{aligned}\begin{aligned}&BULI-{{{\bar{S}}}_{1}}= \\&\left[ \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.35 \right) ;0.43 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.79 \right) ;0.55 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.50 \right) ;0.44 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.91 \right) ;0.63 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.64 \right) ;0.49 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.70 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.52 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.54 \right) ;0.45 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.77 \right) ;0.54 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.56 \right) ;0.46 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.86 \right) ;0.59 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.54 \right) ;0.42 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.653 \right) ;0.48 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.77 \right) ;0.53 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.42 \right) ;0.41 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.84 \right) ;0.58 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.44 \right) ;0.43 \right\rangle \\ \end{matrix} \right. \\&\left. \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.54 \right) ;0.46 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.59 \right) ;0.46 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.58 \right) ;0.46 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.65 \right) ;0.49 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.70 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.67 \right) ;0.50 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.78 \right) ;0.54 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.68 \right) ;0.49 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.62 \right) ;0.47 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.58 \right) ;0.46 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.76 \right) ;0.53 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.66 \right) ;0.48 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.80 \right) ;0.55 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.28 \right) ;0.39 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.51 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.53 \right) ;0.44 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.59 \right) ;0.45 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.56 \right) ;0.43 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.61 \right) ;0.45 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \end{matrix} \right] \\ \end{aligned}\\\begin{aligned}&BULI-{{{\bar{S}}}_{2}}= \\&\left[ \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.80 \right) ;0.68 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.06 \right) ;0.63 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.93 \right) ;0.85 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.23 \right) ;0.60 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.92 \right) ;0.84 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.61 \right) ;0.67 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.74 \right) ;0.71 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.78 \right) ;0.74 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.70 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.26 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.84 \right) ;0.77 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.13 \right) ;0.60 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.82 \right) ;0.75 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.16 \right) ;0.60 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.55 \right) ;0.64 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.53 \right) ;0.63 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.71 \right) ;0.69 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.17 \right) ;0.58 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.82 \right) ;0.76 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.16 \right) ;0.60 \right\rangle \\ \end{matrix} \right. \\&\left. \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.46 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.54 \right) ;0.63 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.46 \right) ;0.60 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.70 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.57 \right) ;0.66 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.71 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.62 \right) ;0.67 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.85 \right) ;0.78 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.21 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.24 \right) ;0.60 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.15 \right) ;0.58 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.52 \right) ;0.61 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.60 \right) ;0.65 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.70 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.84 \right) ;0.54 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.56 \right) ;0.63 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.38 \right) ;0.60 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.46 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.55 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.40 \right) ;0.58 \right\rangle &{} \left\langle 0;0 \right\rangle \\ \end{matrix} \right] \\ \end{aligned}\\\begin{aligned}&BULI-{{{\bar{S}}}_{3}}= \\&\left[ \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.83 \right) ;0.57 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.13 \right) ;0.41 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.86 \right) ;0.59 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.28 \right) ;0.43 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.91 \right) ;0.64 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.66 \right) ;0.49 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.75 \right) ;0.53 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.77 \right) ;0.57 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.69 \right) ;0.48 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.28 \right) ;0.36 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.76 \right) ;0.52 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.27 \right) ;0.37 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.86 \right) ;0.60 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.28 \right) ;0.43 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.75 \right) ;0.52 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.63 \right) ;0.47 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.60 \right) ;0.46 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.30 \right) ;0.40 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.80 \right) ;0.55 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.20 \right) ;0.42 \right\rangle \\ \end{matrix} \right. \\&\text { }\left. \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.38 \right) ;0.41 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.57 \right) ;0.45 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.43 \right) ;0.42 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.64 \right) ;0.46 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.58 \right) ;0.47 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.67 \right) ;0.50 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.62 \right) ;0.47 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.85 \right) ;0.59 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.47 \right) ;0.40 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.33 \right) ;0.39 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.25 \right) ;0.37 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.49 \right) ;0.41 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.65 \right) ;0.48 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.72 \right) ;0.51 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.37 \right) ;0.41 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.71 \right) ;0.49 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.30 \right) ;0.41 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.49 \right) ;0.43 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.50 \right) ;0.42 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.43 \right) ;0.41 \right\rangle \\ \end{matrix} \right] \\ \end{aligned}\\\begin{aligned}&BULI-{{{\bar{S}}}_{4}}= \\&\left[ \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.00 \right) ;0.69 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.58 \right) ;0.71 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.30 \right) ;0.67 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.77 \right) ;0.71 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.73 \right) ;0.73 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.33 \right) ;0.69 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.30 \right) ;0.68 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.30 \right) ;0.64 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.10 \right) ;0.66 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.98 \right) ;0.71 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.19 \right) ;0.67 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.97 \right) ;0.78 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.48 \right) ;0.68 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.98 \right) ;0.63 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.24 \right) ;0.62 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.95 \right) ;0.70 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.57 \right) ;0.67 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.63 \right) ;0.61 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.57 \right) ;0.68 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.96 \right) ;0.66 \right\rangle \\ \end{matrix} \right. \\&\text { }\left. \begin{matrix} \left\langle {{\Delta }_{S}}\left( 3.90 \right) ;0.72 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.87 \right) ;0.70 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.82 \right) ;0.68 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.10 \right) ;0.69 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.20 \right) ;0.65 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.33 \right) ;0.68 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.98 \right) ;0.68 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.78 \right) ;0.69 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.11 \right) ;0.68 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.94 \right) ;0.69 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.01 \right) ;0.68 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.03 \right) ;0.66 \right\rangle \\ \left\langle {{\Delta }_{S}}\left( 3.08 \right) ;0.66 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.07 \right) ;0.66 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle 0;0 \right\rangle &{} \left\langle {{\Delta }_{S}}\left( 3.53 \right) ;0.61 \right\rangle \\ \end{matrix} \right] \\ \end{aligned}\end{aligned}$$