Skip to main content

Advertisement

Springer Nature Link
Log in

A Hybrid Framework for Selecting Food Waste Treatment Techniques Using q-Rung Orthopair Fuzzy CRITIC-Generalized TODIM Method

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

Abstract

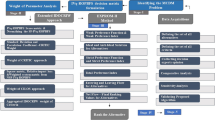
Evaluation and prioritization are indispensable for selecting the appropriate FWTT (food waste treatment technique). Previous research shows that the decision methods are suitable for selecting FWTTs. Nevertheless, current literature on choosing FWTTs seldom considers the impact of experts’ bounded rational behavior and interactive criteria, especially with uncertain information. This article proposes a q-ROFS (q-rung orthopair fuzzy set)-generalized TODIM (an acronym in Portuguese for interactive and multi-criteria decision-making method)-based hybrid framework for coping with the selection problem of FWTTs. In the information fusion process, the fuzziness and ambiguity of information are expressed by the q-ROFS. Then, the q-ROFS-CRITIC (criteria importance through inter-criteria correlation) method is introduced to calculate the criteria weights considering the interactive criteria. Next, the q-ROFS-generalized TODIM framework is presented to rank the alternative techniques considering the experts’ bounded rational behavior. Finally, a real case of selecting FWTTs with q-ROFSs is performed to examine the applicability and feasibility of this framework. The result shows that the alternative \(f_{2}\) (anaerobic digestion) has the highest priority for food waste treatment with the largest overall dominance degree value. After that, a sensitivity study of different parameters and comparative analysis with similar selection methods are organized to elaborate on the reasonability of the provided framework.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Abbreviations

AD:

Anaerobic digestion

CP:

Composting

CRITIC:

Criteria importance through inter-criteria correlation

EDAS:

Distance from average solution

FAO:

Food and agriculture organization

FFS:

Fermatean fuzzy set

FW:

Food waste

FWTT:

Food waste treatment technique

HD:

Degree of hesitancy

IFS:

Intuitionistic fuzzy set

IN:

Incineration

LF:

Landfill

MCDM:

Multi-criteria decision-making

MULTIMOORA:

Multi-attribute multi-objective optimization with the ratio analysis

PFS:

Pythagorean fuzzy set

q-ROFS:

Q-rung orthopair fuzzy set

TODIM:

Acronym in Portuguese for interactive and multi-criteria decision-making

WA:

Weighted averaging

\(\tilde{P}\) :

A q-ROFS

\(\gamma_{{\tilde{P}}} \left( x \right)\) :

Degree of membership

\(\delta_{{\widetilde{P}}} \left( x \right)\) :

Degree of non-membership

\(\mu_{{\widetilde{P}}} \left( x \right)\) :

Degree of hesitancy

\(S\left( {\tilde{g}} \right)\) :

Score function

\(H\left( {\tilde{g}} \right)\) :

Accuracy function

\(\widehat{d}\left( {g_{1} ,g_{2} } \right)\) :

Distance between two fuzzy numbers

\(v^{t}\) :

A group of experts

\(f_{i}\) :

Each alternative

\(f_{1}\) :

CP

\(f_{2}\) :

AD

\(f_{3}\) :

IN

\(f_{4}\) :

LF

\(z_{j}\) :

Each criterion

\(\widetilde{{A^{t} }}\) :

Q-ROFS evaluation matrix

\(\overline{{\widetilde{{a_{{_{ij} }}^{t} }}}}\) :

Mean values of the individual matrix

\(B\) :

Similarity measure

\(w_{t}\) :

Weights of experts

\(\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{A}\) :

Group decision matrix

\(a^{\prime}_{ij}\) :

Score matrix

\(\sigma_{j}\) :

Standard deviation value

\(r_{{jj^{\prime}}}\) :

Correlation coefficient

\(w_{j}\) :

Criteria weights

\(l_{j}\) :

Quantity of information on criteria

\(\Phi_{j} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\) :

Dominance grade

\(\Phi \left( {f_{i} } \right)\) :

Overall dominance degree

References

  1. Wang W., Cao, Y., Deveci, M., Wu, Q.: An extensible complex spherical fuzzy decision making model based selection framework for the food waste treatment method. Appl. Soft Comput. 150 111068 (2024)

    Google Scholar 

  2. Xue, L., Liu, X., Lu, S., Cheng, G., Hu, Y., Liu, J., Dou, Z., Cheng, S., Liu, G.: China’s food loss and waste embodies increasing environmental impacts. Nat. Food. 2(7), 519–528 (2021)

    MATH  Google Scholar 

  3. Minnuse, T.J., Fangninou, F.F., Bowen, A.M., Cao, J., Wu, B., Moleli, I.A.: Food waste: waste or resource? Current practices and status. Int. J. Sci. Res. Publ. 10(1), 735–739 (2020)

    Google Scholar 

  4. Bernstad Saraiva Schott, A., Wenzel, H., la Cour-Jansen, J.: Identification of decisive factors for greenhouse gas emissions in comparative life cycle assessments of food waste management: an analytical review. J. Clean. Prod. 119, 13–24 (2016)

    Google Scholar 

  5. Rani, P., Mishra, A.R., Krishankumar, R., Ravichandran, K.S., Kar, S.: Multi-criteria food waste treatment method selection using single-valued neutrosophic-CRITIC-MULTIMOORA framework. Appl. Soft Comput. 111, 107657–107670 (2021)

    MATH  Google Scholar 

  6. Wang, W., Chen, Y., Wang, Y., Deveci, M., Moslem S., Coffman, D.M.: Unveiling the implementation barriers to the digital transformation in the energy sector using the Fermatean cubic fuzzy method. Appl. Energy. 360 122756 (2024)

    Google Scholar 

  7. Wang, W., Han, X., Ding, W., Wu, Q., Chen, X., Deveci, M.: A Fermatean fuzzy Fine–Kinney for occupational risk evaluation using extensible MARCOS with prospect theory. Eng. Appl. Artif. Intell. 117, 105518–105530 (2023)

    MATH  Google Scholar 

  8. Tripathi, D., Nigam, S.K., Mishra, A.R., Shah, A.R.: A novel intuitionistic fuzzy distance measure-SWARA-COPRAS method for multi-criteria food waste treatment technology selection. Oper. Res. Eng. Sci. Theory Appl. (2022). https://doi.org/10.31181/oresta111022106t

    Article  MATH  Google Scholar 

  9. Fetanat, A., Tayebi, M., Mofid, H.: Water-energy-food security nexus based selection of energy recovery from wastewater treatment technologies: an extended decision making framework under intuitionistic fuzzy environment. Sustain. Energy Technol. Assess. 43, 100937–100955 (2021)

    Google Scholar 

  10. Chaurasiya, R., Jain, D.: Pythagorean fuzzy entropy measure-based complex proportional assessment technique for solving multi-criteria healthcare waste treatment problem. Granul. Comput. 7(4), 917–930 (2022)

    MATH  Google Scholar 

  11. Ramya, L., Narayanamoorthy, S., Manirathinam, T., Kalaiselvan, S., Kang, D.: An extension of the hesitant Pythagorean fuzzy ELECTRE III: techniques for disposing of e-waste without any harm. Appl. Nanosci. 13, 1939–1957 (2022)

    Google Scholar 

  12. Rani, P., Mishra, A.R., Saha, A., Hezam, I.M., Pamucar, D.: Fermatean fuzzy Heronian mean operators and MEREC-based additive ratio assessment method: an application to food waste treatment technology selection. Int. J. Intell. Syst. 37(3), 2612–2647 (2021)

    MATH  Google Scholar 

  13. Yager, R.R.: Generalized orthopair fuzzy sets. IEEE Trans. Fuzzy Syst. 25(5), 1222–1230 (2017)

    MATH  Google Scholar 

  14. Deveci, M., Pamucar, D., Gokasar, I., Koppen, M., Gupta, B.B.: Personal mobility in metaverse with autonomous vehicles using q-rung orthopair fuzzy sets based OPA-RAFSI model. IEEE Trans. Intell. Transp. Syst. (2022). https://doi.org/10.1109/TITS.2022.3186294

    Article  MATH  Google Scholar 

  15. Xiao, L., Huang, G., Pedrycz, W., Pamucar, D., Martínez, L., Zhang, G.: A q-rung orthopair fuzzy decision-making model with new score function and best-worst method for manufacturer selection. Inf. Sci. 608, 153–177 (2022)

    MATH  Google Scholar 

  16. Kumar, K., Chen, S.-M.: Group decision making based on q-rung orthopair fuzzy weighted averaging aggregation operator of q-rung orthopair fuzzy numbers. Inf. Sci. 598, 598–616 (2022)

    MATH  Google Scholar 

  17. Deveci, M., Gokasar, I., Pamucar, D., Coffman, D.M., Papadonikolaki, E.: Safe E-scooter operation alternative prioritization using a q-rung orthopair Fuzzy Einstein based WASPAS approach. J. Clean. Prod. 347, 131239–131257 (2022)

    MATH  Google Scholar 

  18. Saha, A., Mishra, A.R., Rani, P., Hezam, I.M., Cavallaro, F.: A q-rung orthopair fuzzy FUCOM double normalization-based multi-aggregation method for healthcare waste treatment method selection. Sustainability 14(7), 4171–4199 (2022)

    MATH  Google Scholar 

  19. Mishra, A.R., Rani, P., Pamucar, D., Hezam, I.M., Saha, A.: Entropy and discrimination measures based q-rung orthopair fuzzy MULTIMOORA framework for selecting solid waste disposal method. Environ. Sci. Pollut. Res. 30, 12988–13011 (2023)

    MATH  Google Scholar 

  20. Soni, A., Das, P.K., Kumar, S.: Application of q-rung orthopair fuzzy based SWARA-COPRAS model for municipal waste treatment technology selection. Environ. Sci. Pollut. Res. 30(37), 88111–88131 (2023)

    MATH  Google Scholar 

  21. Narayanamoorthy, S., Anuja, A., Pragathi, S., Sandra, M., Ferrara, M., Ahmadian, A., Kang, D.: Assessment of inorganic solid waste management techniques using full consistency and extended MABAC method. Environ. Sci. Pollut. Res. (2023). https://doi.org/10.1007/s11356-11023-29195-11350

    Article  Google Scholar 

  22. Naz, S., Akram, M., Naseer, I., Saeid, A.B., Fatima, A.: 2-Tuple linguistic q-rung orthopair fuzzy power MSM approach for choosing sustainable waste disposal technology. Sci. Iran. (2023). https://doi.org/10.24200/SCI.22023.60804.27006

    Article  MATH  Google Scholar 

  23. Khan, A., Rehman, N., Al-Duais, F.S., Al-Bossly, A., Al-Essa, L.A., Tag-eldin, E.M.: A novel decision model with Einstein aggregation approach for garbage disposal plant site selection under q-rung orthopair hesitant fuzzy rough information. AIMS Math. 8(10), 22830–22874 (2023)

    MATH  Google Scholar 

  24. Kang, D., Anuja, A., Ahmadian, A., Manirathinam, T., Shanmugam, P., Narayanamoorthy, S.: Sustainable assessment of plastic and mixed waste disposal problem during COVID-19 pandemic: an integrated multi-criteria decision-making approach. Environ. Dev. Sustain. (2023). https://doi.org/10.1007/s10668-10023-03175-10663

    Article  Google Scholar 

  25. Narayanamoorthy, S., Anuja, A., Brainy, J.V., Manirathinam, T., Pragathi, S., Nallasivan Parthasarathy, T., Kang, D.: Assessment of the solid waste disposal method during COVID-19 period using the ELECTRE III method in an interval-valued q-rung orthopair fuzzy approach. Comput. Model. Eng. Sci. 131(3), 1229–1261 (2022)

    Google Scholar 

  26. Seker, S.: IoT based sustainable smart waste management system evaluation using MCDM model under interval-valued q-rung orthopair fuzzy environment. Technol. Soc. 71, 102100–102113 (2022)

    MATH  Google Scholar 

  27. Ali, J.: A q-rung orthopair fuzzy MARCOS method using novel score function and its application to solid waste management. Appl. Intell. 52(8), 8770–8792 (2021)

    MATH  Google Scholar 

  28. Ling, J., Li, X., Lin, M.: Medical waste treatment station selection based on linguistic q-rung orthopair fuzzy numbers. Comput. Model. Eng. Sci. 129(1), 117–148 (2021)

    MATH  Google Scholar 

  29. Buyuk, A.M., Temur, G.T.: Food waste treatment option selection through spherical fuzzy AHP. J. Intell. Fuzzy Syst. 42(1), 97–107 (2022)

    MATH  Google Scholar 

  30. Abu, R., Aziz, M.A.A., Sapuan, N., Abdullah, T.A.T., Hassan, C.H.C., Noor, Z.Z.: Multi-criteria decision approach with stakeholders for food waste management. IOP Conf. Ser. Earth Environ. Sci. 756(1), 012005–012014 (2021)

    Google Scholar 

  31. Wang, W., Liu, X., Chen, X., Qin, Y.: Risk assessment based on hybrid FMEA framework by considering decision maker’s psychological behavior character. Comput. Ind. Eng. 136, 516–527 (2019)

    MATH  Google Scholar 

  32. Llamazares, B.: An analysis of the generalized TODIM method. Eur. J. Oper. Res. 269, 1041–1049 (2018)

    MathSciNet  MATH  Google Scholar 

  33. Wu, Q., Liu, X., Qin, J., Wang, W., Zhou, L.: A linguistic distribution behavioral multi-criteria group decision making model integrating extended generalized TODIM and quantum decision theory. Appl. Soft Comput. 98, 106757–106777 (2021)

    MATH  Google Scholar 

  34. Tang, J., Liu, X., Wang, W.: Consensus-based generalized TODIM approach for occupational health and safety risk analysis with opinion interactions. Appl. Soft Comput. 150, 111093–111122 (2023)

    MATH  Google Scholar 

  35. Huang, G., Xiao, L., Zhang, G.: An integrated design concept evaluation method based on best–worst entropy and generalized TODIM considering multiple factors of uncertainty. Appl. Soft Comput. 140, 110165–110203 (2023)

    Google Scholar 

  36. Wen, H., Chen, Y., Wang, W., Ding, L.: A q-rung orthopair fuzzy generalized TODIM method for prioritizing barriers to sustainable food consumption and production. J. Intell. Fuzzy Syst. 45(3), 5063–5074 (2023)

    MATH  Google Scholar 

  37. Wu, X., Zhu, Z., Chen, G., Pedrycz, W., Liu, L., Aggarwal, M.: Generalized TODIM method based on symmetric intuitionistic fuzzy Jensen–Shannon divergence. Expert Syst. Appl. 237, 121554–121572 (2024)

    MATH  Google Scholar 

  38. Garg, A., Das, S., Maiti, J., Pal, S.K.: Granulized Z-VIKOR model for failure mode and effect analysis. IEEE Trans. Fuzzy Syst. 30(2), 297–309 (2022)

    MATH  Google Scholar 

  39. Wang, W., Liu, X., Qin, J., Shuli, L.: An extended generalized TODIM for risk evaluation and prioritization of failure modes considering risk indicators interaction. IISE Trans. 11, 1236–1250 (2019)

    MATH  Google Scholar 

  40. Zhang, H., Wang, H., Wei, G.: Spherical fuzzy TODIM method for MAGDM integrating cumulative prospect theory and CRITIC method and its application to commercial insurance selection. Artif. Intell. Rev. 56(9), 10275–10296 (2023)

    MATH  Google Scholar 

  41. Aytekin, A., Okoth, B.O., Korucuk, S., Mishra, A.R., Memiş, S., Karamaşa, Ç., Tirkolaee, E.B.: Critical success factors of lean six sigma to select the most ideal critical business process using q-ROF CRITIC-ARAS technique: case study of food business. Expert Syst. Appl. 224, 120057–120073 (2023)

    MATH  Google Scholar 

  42. Mishra, A.R., Chen, S.-M., Rani, P.: Multicriteria decision making based on novel score function of Fermatean fuzzy numbers, the CRITIC method, and the GLDS method. Inf. Sci. 623, 915–931 (2023)

    MATH  Google Scholar 

  43. Haktanır, E., Kahraman, C.: A novel picture fuzzy CRITIC & REGIME methodology: wearable health technology application. Eng. Appl. Artif. Intell. 113, 104942–104956 (2022)

    MATH  Google Scholar 

  44. Peng, X., Garg, H.: Intuitionistic fuzzy soft decision making method based on CoCoSo and CRITIC for CCN cache placement strategy selection. Artif. Intell. Rev. 55(2), 1567–1604 (2021)

    MATH  Google Scholar 

  45. Garg, H., Chen, S.-M.: Multiattribute group decision making based on neutrality aggregation operators of q-rung orthopair fuzzy sets. Inf. Sci. 517, 427–447 (2020)

    MathSciNet  MATH  Google Scholar 

  46. Liu, P., Wang, P.: Some q-rung orthopair fuzzy aggregation operators and their applications to multiple-attribute decision making. Int. J. Intell. Syst. 33(2), 259–280 (2018)

    MATH  Google Scholar 

  47. Peng, X., Liu, L.: Information measures for q-rung orthopair fuzzy sets. Int. J. Intell. Syst. 34(8), 1795–1834 (2019)

    MathSciNet  MATH  Google Scholar 

  48. Verma, R., Álvarez-Miranda, E.: Group decision-making method based on advanced aggregation operators with entropy and divergence measures under 2-tuple linguistic Pythagorean fuzzy environment. Expert Syst. Appl. 231, 120584–120616 (2023)

    MATH  Google Scholar 

  49. Liu, P., Rani, P., Mishra, A.R.: A novel Pythagorean fuzzy combined compromise solution framework for the assessment of medical waste treatment technology. J. Clean. Prod. 292, 126047–126424 (2021)

    MATH  Google Scholar 

  50. Komal: Archimedean t-norm and t-conorm based intuitionistic fuzzy WASPAS method to evaluate health-care waste disposal alternatives with unknown weight information. Appl. Soft Comput. 146, 110751–110774 (2023)

    MATH  Google Scholar 

  51. Alkan, N., Kahraman, C.: An intuitionistic fuzzy multi-distance based evaluation for aggregated dynamic decision analysis (IF-DEVADA): its application to waste disposal location selection. Eng. Appl. Artif. Intell. 111, 104809–104834 (2022)

    MATH  Google Scholar 

  52. Chakraborty, S., Saha, A.K.: Novel Fermatean Fuzzy Bonferroni Mean aggregation operators for selecting optimal health care waste treatment technology. Eng. Appl. Artif. Intell. 119, 105752–105768 (2023)

    MATH  Google Scholar 

  53. Patel, A., Jana, S., Mahanta, J.: Intuitionistic fuzzy EM-SWARA-TOPSIS approach based on new distance measure to assess the medical waste treatment techniques. Appl. Soft Comput. 144, 110521–110543 (2023)

    Google Scholar 

  54. Alkan, N., Kahraman, C.: Evaluation of government strategies against COVID-19 pandemic using q-rung orthopair fuzzy TOPSIS method. Appl. Soft Comput. 110, 107653 (2021)

    MATH  Google Scholar 

  55. Du, W.S.: Concise representations and limiting cases of q-rung orthopair fuzzy Hamacher-Bonferroni mean aggregations. Comput. Appl. Math. 42(8), 357–390 (2023)

    MathSciNet  MATH  Google Scholar 

  56. Ashraf, A., Ullah, K., Hussain, A., Bari, M.: Interval-valued picture fuzzy maclaurin symmetric mean operator with application in multiple attribute decision-making. Rep. Mech. Eng. 3(1), 301–317 (2022)

    MATH  Google Scholar 

  57. Wang, W., Cao, Y., Deveci, M., Wu, Q.: An extensible complex spherical fuzzy decision making model based selection framework for the food waste treatment method. Appl. Soft Comput. 150, 111068–111114 (2024)

    Google Scholar 

  58. Peng, X., Krishankumar, R., Ravichandran, K.S.: A novel interval-valued fuzzy soft decision-making method based on CoCoSo and CRITIC for intelligent healthcare management evaluation. Soft. Comput. 25(6), 4213–4241 (2021)

    MATH  Google Scholar 

  59. Torkayesh, A.E., Malmir, B., Rajabi Asadabadi, M.: Sustainable waste disposal technology selection: the stratified best-worst multi-criteria decision-making method. Waste Manag. 122, 100–112 (2021)

    MATH  Google Scholar 

  60. Palaniveloo, K., Amran, M.A., Norhashim, N.A., Mohamad-Fauzi, N., Peng-Hui, F., Hui-Wen, L., Kai-Lin, Y., Jiale, L., Chian-Yee, M.G., Jing-Yi, L., Gunasekaran, B., Razak, S.A.: Food waste composting and microbial community structure profiling. Processes 8(6), 723–753 (2020)

    Google Scholar 

  61. Meng, Q., Liu, H., Zhang, H., Xu, S., Lichtfouse, E., Yun, Y.: Anaerobic digestion and recycling of kitchen waste: a review. Environ. Chem. Lett. 20(3), 1745–1762 (2022)

    MATH  Google Scholar 

  62. Llamazares, B.: An analysis of the generalized TODIM method. Eur. J. Oper. Res. 269(3), 1041–1049 (2018)

    MathSciNet  MATH  Google Scholar 

  63. Wang, W., Liu, X., Qin, Y., Fu, Y.: A risk evaluation and prioritization method for FMEA with prospect theory and Choquet integral. Saf. Sci. 110, 152–163 (2018)

    MATH  Google Scholar 

  64. Alattas, K., Wu, Q.: A framework to evaluate the barriers for adopting the internet of medical things using the extended generalized TODIM method under the hesitant fuzzy environment. Appl. Intell. 52(12), 13345–13363 (2022)

    Google Scholar 

Download references

Acknowledgements

This work was supported by the Social Science Planning Project of Anhui province (AHSKQ2021D56).

Author information

Authors and Affiliations

  1. School of Economics and Management, Anhui Normal University, Wuhu, 241002, Anhui, China

    Yushuo Cao, Xuzhong Wu, Ling Ding & Weizhong Wang

Authors
  1. Yushuo Cao
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Xuzhong Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Ling Ding
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Weizhong Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Ling Ding.

Appendix

Appendix

See Tables 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, and 28.

Table 17 The dominance degree \(\Phi_{2} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 18 The dominance degree \(\Phi_{3} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 19 The dominance degree \(\Phi_{4} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 20 The dominance degree \(\Phi_{5} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 21 The dominance degree \(\Phi_{6} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 22 The dominance degree \(\Phi_{7} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 23 The dominance degree \(\Phi_{8} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 24 The dominance degree \(\Phi_{9} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 25 The dominance degree \(\Phi_{10} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 26 The dominance degree \(\Phi_{11} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 27 The dominance degree \(\Phi_{12} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table
Table 28 The dominance degree \(\Phi_{13} \left( {f_{i} ,f_{{i^{\prime}}} } \right)\)
Full size table

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cao, Y., Wu, X., Ding, L. et al. A Hybrid Framework for Selecting Food Waste Treatment Techniques Using q-Rung Orthopair Fuzzy CRITIC-Generalized TODIM Method. Int. J. Fuzzy Syst. 26, 1916–1935 (2024). https://doi.org/10.1007/s40815-024-01714-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-024-01714-2

Keywords