Skip to main content

Advertisement

Springer Nature Link
Log in

Predefined-time Fuzzy Output Feedback Control for Nonlinear Systems with Multiple Actuator Constraints

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

Abstract

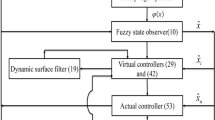
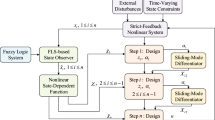
In this paper, a predefined-time adaptive fuzzy tracking control method is proposed for uncertain nonlinear systems with multiple actuator constraints and external disturbances. The unknown dynamic part of the system is dealt with by means of the fuzzy approximation theory, and the unmeasured state in the system is approximated by the constructed fuzzy state observer. On the basis of the observer, a novel predefined-time control scheme is developed to ensure that the system can achieve the practical predefined time stable (PPTS). Combined with the stability analysis, the virtual control input with predefined time can be obtained, and its derivative can be estimated by the first-order filter. Theoretical analysis shows that the proposed controller achieves a small residual set of error convergence to the origin in a predefined time. Finally, the feasibility of the theoretical results is demonstrated through simulation examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

References

  1. Ren, C.-E., Chen, C.L.P., Du, T., Guan, Y.: Fuzzy adaptive leader-following consensus control for nonlinear multi-agent systems with unknown control directions. Int. J. Fuzzy Syst. 22, 748 (2020)

    Article  MATH  Google Scholar 

  2. Shi, J., Cao, J., Liu, H., Zhang, X.: Compound adaptive fuzzy output feedback control for uncertain fractional-order nonlinear systems with fuzzy dead-zone input. Int. J. Fuzzy Syst. 25, 2439–2452 (2023)

    Article  MATH  Google Scholar 

  3. Wang, H., Liu, P.X., Niu, Ben: Robust fuzzy adaptive tracking control for nonaffine stochastic nonlinear switching systems. IEEE Trans. Cybernet. 48(8), 2462–2471 (2018)

    Article  MATH  Google Scholar 

  4. Ochoa, P., Castillo, O., Melin, P.: Castro, J R: Interval type-3 fuzzy differential evolution for parameterization of fuzzy controllers. Int. J. Fuzzy Syst. 25(4), 1360–1376 (2023)

    Article  MATH  Google Scholar 

  5. Precup, R.-E., Preitl, S., Petriu, E., Bojan-Dragos, C.-A., Szedlak-Stinean, A.-I., Roman, R.-C., Hedrea, E.-L.: Model-based fuzzy control results for networked control systems. Reports Mech. Eng. 1(1), 10–25 (2020)

    Article  MATH  Google Scholar 

  6. Bojan-Dragos, C.-A., Precup, R.-E., Preitl, S., Roman, R.-C., Hedrea, E.-L., Szedlak-Stinean, A.-I.: GWO-based optimal tuning of type-1 and type-2 fuzzy controllers for electromagnetic actuated clutch systems. IFAC-PapersOnLine. 54(4), 189–194 (2021)

    Article  MATH  Google Scholar 

  7. Precup, R.-E., Nguyen, A.-T., Blažič, S.: A survey on fuzzy control for mechatronics applications. Int. J. Syst. Sci. 55(4), 771–813 (2024)

    Article  MATH  Google Scholar 

  8. Yu, J., Shi, P., Dong, W., Yu, H.: Observer and command-filter-based adaptive fuzzy output feedback control of uncertain nonlinear systems. IEEE Trans. Ind. Electron. 62(9), 5962–5970 (2015)

    Article  MATH  Google Scholar 

  9. Zhang, K., Hao, W.-N., Yu, X.-H., Jin, D.-W., Yu, K.: A fuzzy neural network classifier and its dual network for adaptive learning of structure and parameters. Int. J. Fuzzy Syst. 25, 1034–1054 (2023)

    Article  MATH  Google Scholar 

  10. Sui, S., Li, Y., Tong, S.: Observer-based adaptive fuzzy control for switched stochastic nonlinear systems with partial tracking errors constrained. IEEE Trans. Syst. Man Cybernet. Syst. 46(12), 1605–1617 (2016)

    Article  MATH  Google Scholar 

  11. Xu, H., Yu, D., Sui, S., Chen, C.L.P.: An Event-Triggered Predefined Time Decentralized Output Feedback Fuzzy Adaptive Control Method for Interconnected Systems. IEEE Trans. Fuzzy Syst. (2022). https://doi.org/10.1109/TFUZZ.2022.3184834

    Article  MATH  Google Scholar 

  12. Wang, H., Liu, K., Liu, X., Chen, B., Lin, C.: Neural-Based Adaptive Output-Feedback Control for a Class of Nonstrict-Feedback Stochastic Nonlinear Systems. IEEE Trans. Cybernet. 45(9), 1977–1987 (2015)

    Article  MATH  Google Scholar 

  13. Xu, H.,Yu, ., Sui, S., Chen, C.L.P., Wang, Z.:Nonsingular practical fixed-time adaptive output feedback control of MIMO nonlinear. IEEE Transactions on Neural Networks and Learning Systems. https://doi.org/10.1109/TNNLS.2021.3139230.(2022)

  14. Zuo, Z., Tie, L.: A new class of finite-time nonlinear consensus protocols for multi-agent systems. Int. J. Control. 87(2), 363–370 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  15. Huang, A.-C., Chen, Y.-C.: Adaptive sliding control for single-link flexible-joint robot with mismatched uncertainties. IEEE Trans. Control Syst. Technol. 12(5), 770–775 (2004)

    Article  MATH  Google Scholar 

  16. Wang, L., Wang, H., Liu, P.X., Ling, S., Liu, S.: Fuzzy finite-time command filtering output feedback control of nonlinear systems. IEEE Trans. Fuzzy Syst. 30(1), 97–107 (2020)

    Article  MATH  Google Scholar 

  17. Chen, B., Liu, X., Ge, S.S., Lin, C.: Adaptive fuzzy control of a class of nonlinear systems by fuzzy approximation approach. IEEE Trans. Fuzzy Syst. 20(6), 1012–1021 (2012)

    Article  MATH  Google Scholar 

  18. Liang, Y., Li, Y.-X., Che, W.-W., Hou, Z.: Adaptive fuzzy asymptotic tracking for nonlinear systems with nonstrict-feedback structure. IEEE Trans. Cybernet. 51(2), 853–861 (2020)

    Article  MATH  Google Scholar 

  19. Wang, L., Liu, P.X., Wang, H.: Fast finite-time control for nonaffine stochastic nonlinear systems against multiple actuator constraints via output feedback. IEEE Trans. Cybernet. 53(5), 3253–3262 (2023)

    Article  MATH  Google Scholar 

  20. Bai, W., Liu, P.X., Wang, H.: Neural-network-based adaptive fixed-time control for nonlinear multiagent non-affine systems. IEEE Trans. Neural Netw. Learn. Syst. (2022). https://doi.org/10.1109/TNNLS.2022.3175929

    Article  MATH  Google Scholar 

  21. Tong, S., Li, Y., Sui, S.: Adaptive fuzzy tracking control design for SISO uncertain nonstrict feedback nonlinear systems. IEEE Trans. Fuzzy Syst. 24(6), 1441–1454 (2016)

    Article  MATH  Google Scholar 

  22. Chen, M., Wang, H., Liu, X.: Adaptive fuzzy practical fixed-time tracking control of nonlinear systems. IEEE Trans. Fuzzy Syst. 29(3), 664–673 (2019)

    Article  MATH  Google Scholar 

  23. Wang, L., Wang, H., Liu, P.X.: Adaptive fuzzy finite-time control of stochastic nonlinear systems with actuator faults. Nonlinear Dyn. 104(1), 523–536 (2021)

    Article  MATH  Google Scholar 

  24. Wang, L., Wang, H., Liu, P X.: Finite-time Compensation Control for State-Variable-Unmeasurable Nonlinear Systems with Sensor and Actuator Faults. IEEE Transactions on Instrumentation and Measurement. 71, (2022)

  25. Sun, Y., Chen, B., Lin, C., Wang, H.: Finite-time adaptive control for a class of nonlinear systems with nonstrict feedback structure. IEEE Trans Cybernet. 48(10), 2774–2782 (2017)

    Article  MATH  Google Scholar 

  26. Zou, X., Zhao, T., Dian, S.: Finite-time adaptive interval type-2 fuzzy tracking control for Mecanum-Wheel mobile robots. Int. J. Fuzzy Syst. 24, 1570–1585 (2022)

    Article  MATH  Google Scholar 

  27. Wang, N., Fu, Z., Tao, F., Song, S., Wang, T.: Observer-based adaptive fuzzy finite-time fault-tolerant control for stochastic nonlinear systems with state Constraint. Int. J. Fuzzy Syst. 24, 3265–3276 (2022)

    Article  MATH  Google Scholar 

  28. Zhang, Y., Xie, L., Zhang, K.: Fast finite-time fuzzy control for a class of nonstrict feedback systems with input quantization. Int. J. Fuzzy Syst. 25, 1213–1226 (2023)

    Article  MATH  Google Scholar 

  29. Lu, K., Liu, Z., Wang, Y., Chen, C.L.P.: Fixed-time adaptive fuzzy control for uncertain nonlinear systems. IEEE Trans. Fuzzy Syst. 29(12), 3769–3781 (2020)

    Article  MATH  Google Scholar 

  30. Xia, J., Zhang, J., Sun, W., Zhang, B., Wang, Z.: Finite-time adaptive fuzzy control for nonlinear systems with full state constraints. IEEE Trans. Syst. Man Cybernet. 49(7), 1541–1548 (2018)

    Article  MATH  Google Scholar 

  31. Shen, Y., Huang, Y.: Global finite-time stabilisation for a class of nonlinear systems. Int. J. Syst. Sci. 43(1), 73–78 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  32. Liu, J., Zhang, Y., Yu, Y., Sun, C.: Fixed-time leader-follower consensus of networked nonlinear systems via event/self-triggered control. IEEE Trans. Neural Netw. Learn. Syst. 31(11), 5029–5037 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  33. Jin, X.: Adaptive fixed-time control for MIMO nonlinear systems with asymmetric output constraints using universal barrier functions. IEEE Trans. Automatic Control. 64(7), 3046–3053 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  34. Qiang, J., Liu, L., Xu, M., Fang, Y.: Fixed-time backstepping control based on adaptive super-twisting disturbance observers for a class of nonlinear systems. Int. J. Control. 95(9), 2294–2306 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  35. Liu, R., Liu, M., Ye, D., Yu, Y.: Event-triggered adaptive fixed-time fuzzy control for uncertain nonlinear systems with unknown actuator faults. Inform. Sci. 612, 344–360 (2022)

    Article  MATH  Google Scholar 

  36. Shi, Y., Wang, C., Li, S.: Observer-based fixed-time fuzzy adaptive output feedback tracking control for a class of nonlinear systems. Int. J. Control Auto. Syst. 20(8), 2569–2582 (2022)

    Article  MATH  Google Scholar 

  37. Cao, L., Xiao, B., Golestani, M.: Robust fixed-time attitude stabilization control of flexible spacecraft with actuator uncertainty. Nonlinear Dyn. 100(3), 2505–2519 (2020)

    Article  MATH  Google Scholar 

  38. Jiang, B., Hu, Q., Friswell, M.I.: Fixed-time attitude control for rigid spacecraft with actuator saturation and faults. IEEE Trans. Control Syst. Technol. 24(5), 1892–1898 (2016)

    Article  MATH  Google Scholar 

  39. Zhang, J., Niu, B., Duan, P., Zhao, P., Wang, H.: Event-triggered adaptive tracking control for nonlinear systems with predefined time-varying output constraints based on Nussbaum design. Int. J. Robust Nonlinear Control. 31(15), 7513–7527 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  40. Garza-Alonso, A., Basin, M., Rodriguez-Ramirez, P.: Predefined-Time Backstepping Stabilization of Autonomous Nonlinear Systems. IEEE/CAA J. Automatica Sinica. 9(11), 2020–2022 (2022)

    Article  MATH  Google Scholar 

  41. Zhang, Y., Niu, B., Zhao, X., Duan, P., Wang, H., Gao, B.: Global predefined-time adaptive neural network control for disturbed pure-feedback nonlinear systems with zero tracking error. IEEE Trans. Neural Netw. Learn. Syst. 34(9), 6328–6338 (2023)

    Article  MathSciNet  MATH  Google Scholar 

  42. Wang, Q., Cao, J., Liu, H.: Adaptive fuzzy control of nonlinear systems with predefined time and accuracy. IEEE Trans. Fuzzy Syst. 30(12), 5152–5165 (2022)

    Article  MATH  Google Scholar 

  43. Ni, J., Shi, P.: Global predefined time and accuracy adaptive neural network control for uncertain strict-feedback systems with output constraint and dead zone. IEEE Trans. Syst. Man Cybernet. 51(12), 7903–7918 (2020)

    Article  MATH  Google Scholar 

  44. Sun, K., Li, Y., Tong, S.: Fuzzy adaptive output feedback optimal control design for strict-feedback nonlinear systems. IEEE Trans. Syst. Man Cybernet. 47(1), 33–44 (2016)

    Article  MATH  Google Scholar 

  45. Chang, Y., Wang, Y., Alsaadi, F.E., Zong, G.: Adaptive fuzzy output-feedback tracking control for switched stochastic pure-feedback nonlinear systems. Int. J. Adaptive Control Signal Process. 33(10), 1567–1582 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  46. Zerari, N., Chemachema, M.: Event-triggered adaptive output-feedback neural-networks control for saturated strict-feedback nonlinear systems in the presence of external disturbance. Nonlinear Dyn. 104(2), 1343–1362 (2021)

    Article  MATH  Google Scholar 

  47. Wang, L., Wang, H., Liu, P.X.: Fuzzy adaptive finite-time output feedback control of stochastic nonlinear systems. ISA Trans. 125, 110–118 (2022)

    Article  MATH  Google Scholar 

  48. Kang, S., Liu, P.X., Wang, H.: Finite-time command filter-based adaptive fuzzy output-feedback control for MIMO nonlinear systems with multiple actuator constraints. Int. J. Syst. Sci. 54(9), 1926–1944 (2023)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Engineering, Shenyang Agricultural University, Shenyang, 110866, China

    Libin Wang, Junlong Niu & Wei Wang

Authors
  1. Libin Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Junlong Niu
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Wei Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Wei Wang.

Ethics declarations

Conflict of Interest

The authors declare that they have no conflict of interest.

Competing interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, L., Niu, J. & Wang, W. Predefined-time Fuzzy Output Feedback Control for Nonlinear Systems with Multiple Actuator Constraints. Int. J. Fuzzy Syst. 27, 410–420 (2025). https://doi.org/10.1007/s40815-024-01786-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-024-01786-0

Keywords