Skip to main content

Advertisement

Springer Nature Link
Log in

Study on Non-iterative Algorithms for Center-of-Sets Type-Reduction of Interval Type-2 Takagi–Sugeno–Kang Fuzzy Logic Systems

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

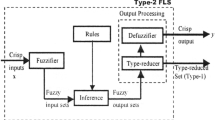
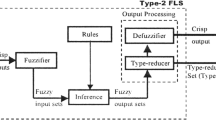
In the application of interval type-2 (IT2) Takagi–Sugeno–Kang (TSK) fuzzy logic systems (FLSs), the center-of-sets (COS) type-reduction (TR) is more advantageous than the centroid TR. This paper proposes three types of discrete non-iterative algorithms to solve the problem of COS TR in IT2 TSK FLSs. Multiple simulation experiments are carried out for the IT2 TSK FLSs with different fuzzy rule numbers. Experimental results show that the computational efficiencies of the three discrete non-iterative algorithms are better than that of Karnik–Mendel (KM) algorithms, which provides latent value for the application of type-2 FLSs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

The authors do not have permission to share data.

References

  1. Niewiadomski, A.: A type-2 fuzzy approach to linguistic summarization of data. IEEE Trans. Fuzzy Syst. 16(1), 198–212 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  2. Wu, D.R., Mendel, J.M.: Linguistic summarization using IF-THEN rules and interval type-2 fuzzy sets. IEEE Trans. Fuzzy Syst. 19(1), 136–151 (2011)

    Article  MATH  Google Scholar 

  3. Castillo, O., Melin, P., Alanis, A., Montiel, O., Sepulveda, R.: Optimization of interval type-2 fuzzy logic controllers using evolutionary algorithms. Soft. Comput. 15(6), 1145–1160 (2011)

    Article  MATH  Google Scholar 

  4. Dereli, T., Baykasoglu, A., Altun, K., Durmusoglu, A., Turksen, I.B.: Industrial applications of type-2 fuzzy sets and systems: a concise review. Comput. Ind. 62(2), 125–137 (2011)

    Article  MATH  Google Scholar 

  5. Hagras, H.: Type-2 FLCs: a new generation of fuzzy controllers. IEEE Comput. Intell. Mag. 2(1), 30–43 (2007)

    Article  MATH  Google Scholar 

  6. Hsiao, M., Li, T.H.S., Lee, J.Z., Chao, C.H., Tsai, S.H.: Design of interval type-2 fuzzy sliding-mode controller. Inf. Sci. 178(6), 1686–1716 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  7. Hidalgo, D., Castillo, O., Melin, P.: Type-1 and type-2 fuzzy inference systems as integration methods in modular neural networks for multimodal biometry and its optimization with genetic algorithms. Inf. Sci. 179(13), 2123–2145 (2009)

    Article  MATH  Google Scholar 

  8. Melin, P., Mendoza, O., Castillo, O.: Face recognition with an improved interval type-2 fuzzy logic Sugeno integral and modular neural networks. IEEE Trans. Syst. Man Cybern. 41(5), 1001–1012 (2011)

    Article  MATH  Google Scholar 

  9. Mitchell, H.B.: Pattern recognition using type-II fuzzy sets. Inf. Sci. 170(2–4), 409–418 (2005)

    Article  MATH  Google Scholar 

  10. Rhee, F.C.-H.: Uncertainty fuzzy clustering: insights and recommendations. IEEE Comput. Intell. Mag. 2(1), 44–56 (2007)

    Article  MATH  Google Scholar 

  11. Liu, X.L., Wan, S.P.: Combinatorial iterative algorithms for computing the centroid of an interval type-2 fuzzy set. IEEE Trans. Fuzzy Syst. 28(4), 607–617 (2020)

    Article  MATH  Google Scholar 

  12. Liu, X.L., Lin, Y.C.: New efficient algorithms for the centroid of an interval type-2 fuzzy set. Inf. Sci. 570(1), 1–19 (2021)

    MathSciNet  MATH  Google Scholar 

  13. Chen, C., John, R., Twycross, J., Garibaldi, J.M.: A direct approach for determining the switch points in the Karnik-Mendel algorithm. IEEE Trans. Fuzzy Syst. 26(2), 1079–1085 (2018)

    Article  Google Scholar 

  14. Mendel, J.M.: On KM algorithms for solving type-2 fuzzy set problems. IEEE Trans. Fuzzy Syst. 21(3), 426–446 (2013)

    Article  MATH  Google Scholar 

  15. Chen, Y., Yang, Y.J.: Study on center-of-sets type-reduction of interval type-2 fuzzy logic systems with noniterative algorithms. J. Intell Fuzzy Syst. 40(6), 11099–11106 (2021)

    Article  MATH  Google Scholar 

  16. Chen, Y.: Study on sampling based discrete Nie-Tan algorithms for computing the centroids of general type-2 fuzzy sets. IEEE Access 7(1), 156984–156992 (2019)

    Article  MATH  Google Scholar 

  17. Wu, D.R.: Approaches for reducing the computational cost of interval type-2 fuzzy logic systems: overview and comparisons. IEEE Trans. Fuzzy Syst. 21(1), 80–99 (2013)

    Article  MATH  Google Scholar 

  18. Li, J., John, R., Coupland, S., Kendall, G.: On Nie-Tan operator and type-reduction of interval type-2 fuzzy sets. IEEE Trans. Fuzzy Syst. 26(2), 1036–1039 (2018)

    Article  MATH  Google Scholar 

  19. Chen, Y., Wang, D.Z.: Study on centroid type-reduction of general type-2 fuzzy logic systems with weighted Nie-Tan algorithms. Soft. Comput. 22(22), 7659–7678 (2018)

    Article  MATH  Google Scholar 

  20. Mendel, J.M., Liu, X.W.: Simplified interval type-2 fuzzy logic systems. IEEE Trans. Fuzzy Syst. 21(6), 1056–1069 (2013)

    Article  MATH  Google Scholar 

  21. Chen, Y., Li, C.X., Yang, J.X.: Design of discrete noniterative algorithms for center-of-sets type reduction of general type-2 fuzzy logic systems. J. Intell. Fuzzy Syst. 24(4), 2024–2035 (2022)

    Article  MATH  Google Scholar 

  22. EI-Nagar, A.M., EI-Bardini, M.: Simplified interval type-2 fuzzy logic system based on new type-reduction. J. Intell. Fuzzy Syst. 27(4), 1999–2010 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  23. Chen, Y.: Study on weighted Nagar-Bardini algorithms for centroid type-reduction of general type-2 fuzzy logic systems. J. Intell. Fuzzy Syst. 37(5), 6527–6544 (2019)

    Article  MATH  Google Scholar 

  24. Chen, Y.: Study on weighted Nagar-Bardini algorithms for centroid type-reduction of interval type-2 fuzzy logic systems. J. Intell. Fuzzy Syst. 34(4), 2417–2428 (2018)

    Article  MATH  Google Scholar 

  25. Biglarbegian, M., Melek, W., Mendel, J.M.: On the robustness of type-1 and interval type-2 fuzzy logic systems in modeling. Inf. Sci. 181(7), 1325–1347 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  26. Biglarbegian, M., Melek, W., Mendel, J.M.: On the stability of interval type-2 TSK fuzzy logic control systems. IEEE Trans. Syst. Man Cybern. 40(3), 798–818 (2010)

    Article  MATH  Google Scholar 

  27. Khanesar, M.A., Jalalian, A., Kaynak, O., Gao, H.J.: Improving the speed of center of set type-reduction in interval type-2 fuzzy systems by eliminating the need for sorting. IEEE Trans. Fuzzy Syst. 25(5), 1193–1206 (2017)

    Article  MATH  Google Scholar 

  28. Chen, Y.: Study on sampling-based discrete noniterative algorithms for centroid type-reduction of interval type-2 fuzzy logic systems. Soft. Comput. 24(15), 11819–11828 (2020)

    Article  MATH  Google Scholar 

  29. Chen, Y., Yang, J.X., Li, C.X.: Design of Takagi Sugeno Kang type interval type-2 fuzzy logic systems optimized with hybrid algorithms. Int. J. Fuzzy Syst. 25(2), 868–879 (2023)

    Article  MATH  Google Scholar 

  30. Ontiveros, E., Melin, P., Castillo, O.: Comparative study of interval type-2 and general type-2 fuzzy systems in medical diagnosis. Inf. Sci. 525, 37–53 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  31. Cuevas, F., Castillo, O., Cortes, P.: Optimal setting of membership functions for interval type-2 fuzzy tracking controllers using a shark smell metaheuristic algorithm. Int. J. Fuzzy Syst. 24(2), 799–822 (2022)

    Article  MATH  Google Scholar 

  32. Castillo, O., Melin, P., Ontiveros, E., et al.: A high-speed interval type 2 fuzzy system approach for dynamic parameter adaptation in metaheuristics. Eng. Appl. Artif. Intell. 85, 666–680 (2019)

    Article  MATH  Google Scholar 

  33. Liu, J., Zhao, T., Cao, J., et al.: Interval type-2 fuzzy neural networks with asymmetric MFs based on the twice optimization algorithm for nonlinear system identification. Inf. Sci. 629, 123–143 (2023)

    Article  MATH  Google Scholar 

  34. Ontiveros, E., Melin, P., Castillo, O.: Higher order \(\alpha \)-planes integration: a new approach to computational cost reduction of general type-2 fuzzy systems. Eng. Appl. Artif. Intell. 74, 186–197 (2018)

    Article  MATH  Google Scholar 

  35. Wu, D.R., Mendel, J.M.: Recommendations on designing practical interval type-2 fuzzy logic systems. Eng. Appl. Artif. Intell. 85, 182–193 (2019)

    Article  MATH  Google Scholar 

  36. Chen, Y., Wang, D.Z.: Forecasting by designing Mamdani general type-2 fuzzy logic systems optimized with quantum particle swarm optimization algorithms. Trans. Inst. Meas. Control. 41(10), 2886–2896 (2019)

    Article  MATH  Google Scholar 

  37. Chen, Y., Wang, D.Z., Ning, W.: Forecasting by TSK general type-2 fuzzy logic systems optimized with genetic algorithms. Optim. Control Appl. Methods 39(1), 393–409 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  38. Chen, Y., Li, C.X., Yang, J.X.: Design and application of Nagar-Bardini structure-based interval type-2 fuzzy logic systems optimized with the combination of backpropagation algorithms and recursive least square algorithms. Expert Syst. Appl. 211, 118596 (2023)

    Article  MATH  Google Scholar 

  39. Ontiveros-Robles, E., Melin, P., Castillo, O.: An efficient high-order \(\alpha \)-plane aggregation in general type-2 fuzzy systems using newton-cotes rules. Int. J. Fuzzy Syst. 23, 1102–1121 (2021)

    Article  MATH  Google Scholar 

  40. Ontiveros-Robles, E., Melin, P., Castillo, O.: New methodology to approximate type-reduction based on a continuous root-finding Karnik Mendel algorithm. Algorithms 10(3), 77 (2017)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This paper is sponsored by the National Natural Science Foundation of China (61973146, 61773188), and 2024 Fundamental Research Project (No. LJ212410154062) of the Educational Department of Liaoning Province.

Author information

Authors and Affiliations

  1. College of Science, Liaoning University of Technology, Jinzhou, 121001, China

    Junge Zhou & Yang Chen

Authors
  1. Junge Zhou
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yang Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yang Chen.

Ethics declarations

Conflict of interest

The authors declare that there exist no conflict of interest.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, J., Chen, Y. Study on Non-iterative Algorithms for Center-of-Sets Type-Reduction of Interval Type-2 Takagi–Sugeno–Kang Fuzzy Logic Systems. Int. J. Fuzzy Syst. 26, 2675–2687 (2024). https://doi.org/10.1007/s40815-024-01873-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-024-01873-2

Keywords