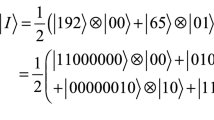Abstract
In the era of big data, image security and real-time processing become more and more important and increasingly difficult to satisfy. To improve the security and processing efficiency of image encryption algorithm, an enhanced quantum scheme is proposed for generalized novel enhanced quantum image representation. The proposed quantum encryption scheme mainly consists of two-stage operation in order, i.e., twice scrambling based generalized Arnold transform and pixel encryption based on the quantum key image (which are generated and prepared based on Logistic map). In the first stage, generalized Arnold transform are employed to simultaneously disturb the coordinate information and pixel gray value of quantum plain image. Following that, the scrambled image is further encrypted into a quantum cipher image based on quantum key image, which is divided into three sub-processes in detail, i.e., CNOT operations, bit-plane scrambling and controlled perfect shuffle permutations are executed orderly. The quantum image decryption process can be easily implemented in a reverse way. The complete quantum circuit implementation for above two stages operation is constructed and analyzed in terms of quantum cost and time complexity. Compared to classical image processing algorithm, the investigated quantum encryption algorithm demonstrates an exponential speedup with computational cost of \({\rm O}\left( n \right)\) for a \(2^{n} \times 2^{n}\) quantum grayscale or color images. The proposed scheme is simulated and verified on a classical computer with MATLAB environments, i.e., not in a real quantum version that not considers the effects of quantum noise. Experimental results and numerical analysis indicate that the presented quantum algorithm has good visual effects and high security.

































Similar content being viewed by others
References
Arnold, V.I., Avez, A.: Ergodic Problems of Classical Mechanics. Benjamin, New York (1968)
Barenco, A., Bennett, C.H., Cleve, R., et al.: Elementary gates for quantum computation. Phys. Rev. A. 52, 3457–3488 (1995)
Dyson, F.J., Falk, H.: Period of a discrete cat mapping. Am. Math. Mon. 99, 603–614 (1992)
Feynman, R.P.: Simulating physics with quantum computers. Int. J. Theor. Phys. 21, 467–488 (1982)
Iliyasu, A.M.: Towards realising secure and efficient image and video processing applications on quantum computers. Entropy. 15, 2874–2974 (2013)
Jiang, N., Dong, X., Hu, H., et al.: Quantum image encryption based on henon mapping. Int. J. Theor. Phys. 58, 979–991 (2019)
Jiang, N., Wang, L., Wu, W.Y.: Quantum hilbert image scrambling. Int. J. Theor. Phys. 53, 2463–2484 (2014a)
Jiang, N., Wang, L.: Analysis and improvement of the quantum Arnold image scrambling. Quantum Inf. Process. 13, 1545–1551 (2014)
Jiang, N., Wu, W.Y., Wang, L.: The quantum realization of Arnold and Fibonacci image scrambling. Quantum Inf. Process. 13, 1223–1236 (2014b)
Le, P.Q., Dong, F., Hirota, K.: A flexible representation of quantum images for polynomial preparation, image compression, and processing operations. Quantum Inf. Process. 10, 63–84 (2011)
Li, H.S., Li, C.Y., Chen, X., Xia, H.Y.: Quantum image encryption algorithm based on NASS. Int. J. Theor. Phys. 57, 3745–3760 (2018a)
Li, H.S., Chen, X., Song, S.X., et al.: A block-based quantum image scrambling for GNEQR. IEEE Access. 7, 138233–138243 (2019a)
Li, H.S., Chen, X., Xia, H.Y., et al.: A Quantum Image Representation Based on Bitplanes. IEEE Access 6, 62396–62404 (2018)
Li, H.S., Fan, P., Xia, H.Y., et al.: Quantum Implementation Circuits of Quantum Signal Representation and Type Conversion. IEEE Trans Circuits Syst. I Regul. Pap. 66, 341–354 (2019b)
Li, H.S., Fan, P., Xia, H.Y., et al.: The multi-level and multi-dimensional quantum wavelet packet transforms. Sci. Rep. 8, 1–23 (2018c)
Li, H.S., Fan, P., Xia, H.Y., Song, S.: Quantum multi-level wavelet transforms. Inf. Sci. 504, 113–135 (2019c)
Li, H.S., Zhu, Q.X., Zhou, R.G., et al.: Multidimensional color image storage, retrieval, and compression based on quantum amplitudes and phases. Inf. Sci. 273, 212–232 (2014)
Li L., Bassem Abd-El-Atty A.A.A.E., Ahmed G.: Quantum color image encryption based on multiple discrete chaotic systems. In: 2017 Federated Conference on Computer Science and Information Systems (2017)
Li, P., Zhao, Y.: A simple encryption algorithm for quantum color image. Int. J. Theor. Phys. 56(6), 1961–1982 (2017)
Liang, H.R., Tao, X.Y., Zhou, N.R.: Quantum image encryption based on generalized affine transform and logistic map. Quantum Inf. Process. 15, 2701–2724 (2016)
Jafarizadeh, M.A., Behnia, S.: Hierarchy of chaotic maps with an invariant measure and their coupling. Phys. D Nonlinear Phenom. 159, 1–21 (2001)
Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)
Ran, Q.W., Wang, L., Ma, J., et al.: A quantum color image encryption scheme based on coupled hyper-chaotic Lorenz system with three impulse injections. Quantum Inf. Process. 17, 188 (2018)
Sang, J.Z., Wang, S., Li, Q.: A novel quantum representation of color digital images. Quantum Inf. Process. 16, 42 (2017)
Stajic, J.: The future of quantum information processing. Science 339, 1163 (2013)
Tan, R.C., Lei, T., Zhao, Q.M., et al.: Quantum color image encryption algorithm based on a hyper-chaotic system and quantum Fourier transform. Int. J. Theor. Phys. 55, 5368–5384 (2016)
Vedral, V., Barenco, A., Ekert, A.: Quantum networks for elementary arithmetic operations. Phys. Rev. A 54, 147 (1996)
Venegas-Andraca S. B.S.: Storing, processing, and retrieving an image using quantum mechanics, In: Proceedings of SPIE Conference of Quantum Information and Computation. pp. 134–147 (2003)
Wang, L., Ran, Q., Ma, J., et al.: QRCI: a new quantum representation model of color digital images. Opt. Commun. 438, 147–158 (2019)
Yan, F., Iliyasu, A.M., Le, P.Q.: Quantum image processing: a review of advances in its security technologies. Int. J. Quantum Inf. 15, 1730001 (2017)
Yan, F., Iliyasu, A.M., Venegas-Andraca, S.E.: A survey of quantum image representations. Quantum Inf. Process. 15, 1–35 (2016)
Yang, Y.G., Jia, X., Sun, S.J., Pan, Q.X.: Quantum cryptographic algorithm for color images using quantum Fourier transform and double random-phase encoding. Inf. Sci. 277, 445–457 (2014)
Yang, Y.G., Xia, J., Jia, X., Zhang, H.: Novel image encryption/decryption based on quantum Fourier transform and double phase encoding. Quantum Inf. Process. 12, 3477–3493 (2013)
Zhang, Y., Lu, K., Gao, Y., Wang, M.: NEQR: a novel enhanced quantum representation of digital images. Quantum Inf. Process. 12(8), 2833–2860 (2013a)
Zhang, Y., Lu, K., Gao, Y., Xu, K.: A novel quantum representation for log-polar images. Quantum Inf. Process. 12, 3103–3126 (2013b)
Zhou, N.R., Chen, W.W., Yan, X.Y., Wang, Y.Q.: Bit-level quantum color image encryption scheme with quantum cross-exchange operation and hyper-chaotic system. Quantum Inf. Process. 17, 137 (2018)
Zhou, N.R., Hua, T.X., Gong, L.H., et al.: Quantum image encryption based on generalized Arnold transform and double random-phase encoding. Quantum Inf. Process. 14, 1193–1213 (2015)
Zhou, R.G., Hu, W.W., Fan, P.: Quantum watermarking scheme through Arnold scrambling and LSB steganography. Quantum Inf. Process. 16, 212 (2017)
Author information
Authors and Affiliations
Contributions
All authors contributed to the study conception and design. Quantum image encryption and decryption algorithms as well as corresponding quantum implementation circuits were proposed by W-WH and R-GZ. The experimental results and numerical analysis are performed by SJ, XL and JL. The first draft of the manuscript was written by W-WH and all authors commented on previous versions of the manuscript. All authors read and approved the final manuscript.
Corresponding author
Ethics declarations
Conflict of interest
On behalf of all authors, the corresponding author states that there is no conflict of interest.
Additional information
This work is supported by the National Key R&D Plan under Grant No. 2018YFC1200200 and 2018YFC1200205.
Appendix 1
Appendix 1
For two n-qubit numbers A and B, \(\left( {A - B} \right)\;\bmod 2^{n}\) can be expressed as:
Proof
1.1 It is obvious that \(\left( {A - B} \right)\;\bmod 2^{n} = A - B\) when \(A \ge B\).
1.2 When \(A < B\), it can be verified as follows:
Assume that \(\overline{B} = \overline{b}_{n - 1} \overline{b}_{n - 2} \cdot \cdot \cdot \overline{b}_{1} \overline{b}_{0}\) denotes the inverse code of B, then we can obtain that:
Thus, \(- B = (\overline{B} + 1) - 2^{n} ,\;\overline{B} + 1 = 2^{n} - B\), and \((A - B)\bmod 2^{n}\) can be deduced as follows:
Since \(A < B \Rightarrow \left[ {2^{n} - \left( {B - A} \right)} \right] < 2^{n}\), we can obtain that:
Rights and permissions
About this article
Cite this article
Hu, WW., Zhou, RG., Jiang, S. et al. Quantum image encryption algorithm based on generalized Arnold transform and Logistic map. CCF Trans. HPC 2, 228–253 (2020). https://doi.org/10.1007/s42514-020-00043-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s42514-020-00043-8