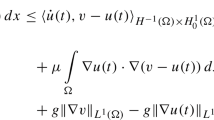Skip to main content
SpringerLink
Log in

Error estimates for the numerical approximation of time-dependent flow of Bingham fluid in cylindrical pipes by the regularization method

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Summary

The flow of a Bingham fluid in a cylindrical pipe can give rise to free boundary problems. The fluid behaves like a viscous fluid if the shear stress, expressed as a linear function of the shear rate, exceeds a yield value, and like a rigid body otherwise. The surfaces dividing fluid and rigid zones are the free boundaries. Therefore the solution for such highly nonlinear problems can in general only be obtained by numerical methods. Considerable progress has been made in the development of numerical algorithms for Bingham fluids [2,20,27,29,30,32]. However, very little research can be found in the literature regarding the rate of convergence of the numerical solution to the true continuous solution, that is the error estimate of these numerical methods. Error estimates are a critically important issue because they tells us how to control the error by appropriately choosing the grid sizes and other related parameters. This paper concerns the error estimates of a unsteady Bingham fluid modeled as a variational inequality due to Duvaut-Lions [16] and Glowinski [30]. The difficulty both in the analytical and numerical treatment of the mathematical model is due to the fact that it contains a nondifferentiable term. A common technique, called the regularization method, is to replace the non-differentiable term by a perturbed differentiable term which depends on a small regularization parameter ε. The regularization method effectively reduces the variational inequality to an equation (a regularized problem) which is much easier to cope with. This paper has achieved the following. (1) Error estimates are derived for a continuous time Galerkin method in suitable norms. (2) We give an estimate of the difference between the true solution and the regularized solution in terms of ε. (3) Some regularity properties for both regularized solution and the true solution are proved. (4) The error estimates for full discretization of the regularized problem using piecewise linear finite elements in space, and backward differencing in time are established for the first time by coupling the regularization parameter ε and the discretization parameters h and Δt. (5) We are able to improve our estimates in the one-dimensional case or under stronger regularity assumptions on the true solution. The estimates for the one-dimensional case are optimal and confirmed by numerical experiment. The estimates from (4) and (5) provide very important information on the measure of the error and give us a powerful mechanism to properly choose the parameters h, Δt and ε in order to control the error (see Corollary 4.4). The above estimates extend the error bounds derived in Glowinski, Lions and Trémolières [32] (chapter 5, pp. 348–404) for the stationary Bingham fluid to the time-dependent one, which is the main contribution of this paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Baiocchi, C.: Discretization of evolution inequalities. In: Partial Differential Equations and the Calculus of Variations, F. Colombini-A. Marino-L. Modica-S. Spagnolo editors, Birkäuser, Boston, 1989, pp. 59–92

  2. Bégis, D.: Analyse numérique de l’écoulement d’un fluide de Bingham, Thèse de 3ème cycle, Université Pierre et Marie Curie, Paris, 1972

  3. Berger, A.E., Falk, R.S.: An error estimate for the truncation method for the solution of parabolic obstacle variational inequalities. Math. Comput. 31, 619–628 (1977)

    MATH  Google Scholar 

  4. Brézis, H.: Monotonicity methods in Hilbert spaces and some applications to nonlinear partial differential equations. In: Contributions to Nonlinear Functional Analysis. E. Zarantonello, Ed., Acad. Press, 1971, pp. 101–156

  5. Brézis, H.: Problèmes Unilatéraux. J. Math. pures et appl. 51, 1–168 (1972)

    Google Scholar 

  6. Brézis, H., Stampacchia, G.: Sur la regularite de la solution d’inequations elliptiques. Bull. Soc. Math. France 96, 254–265 (1968)

    Google Scholar 

  7. Brezzi, F., Hager, W.W., Raviart, P.A.: Error estimates for the finite element solution of variational inequalities. Numer. Math. 28, 431–443 (1977)

    MATH  Google Scholar 

  8. Brezzi, F.: On the existence, uniqueness, and approximation of saddle-point problems arising from Lagrange multiplier. RAIRO Anal. Numér. 8(R-2), 129–151 (1975)

    Google Scholar 

  9. Comparini, E.: Regularization procedures of singular free boundary problems in rotational Bingham flows. Z. Angew. Math. Mech. 77, 543–554 (1997)

    MathSciNet  MATH  Google Scholar 

  10. Comparini, E., Mannucci, P.: Fluid of a Bingham fluid in contact with Newtonian fluid. J. Math. Anal. Appl. 227, 359–381 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  11. Chaplain, V., Mills, P., Guiffant, G., Cerasi, P.: Model for the flow of a yield fluid through a porous-medium. Journal de Physique 2 2, 2145–2158 (1992)

    Article  Google Scholar 

  12. Donati, F.: A penalty method approach to strong solutions of some nonlinear parabolic unilateral problems. Numerical Analysis, Theory, Methods & Applications 6(6), 585–597 (1982)

    Google Scholar 

  13. Dupont, T.: Some L 2 error estimates for parabolic Galerkin methods. In: The Mathematical Foundation of the Finite Element Method with Applications to Partial Differential Equations. A. K. Aziz, ed., Academic Press, New York, 1972

  14. Douglas, J. Jr., Dupont, T.: Galerkin methods for parabolic equations. SIAM J. Numer. Anal. 7(4), (1970)

  15. Dolejs, V., Dolecek, P., Siska, B.: Drag and fall velocity of a spherical particle in generalized Newtonian and viscoplastic fluids. Chem. Eng. Process 37, 189–195 (1998)

    Article  Google Scholar 

  16. Duvaut, G., Lions, J.L.: Inequalities in Mechanics and Physics. Springer-Verlag, 1976

  17. Dorier, C., Tichy, J.: Behavior of A Bingham-like viscous-fluid in lubrication flows. J. Non-Newtonian Fluid Mech. 45, 291–310 (1992)

    Article  MATH  Google Scholar 

  18. Falk, R.S.: Error estimates for the approximation of a class of variational inequalities. Math. Comp. 28, 963–971 (1974)

    MATH  Google Scholar 

  19. Fetter, A.: L ∞-Error estimate for an approximation of a parabolic variational inequality. Numer. Math. 50, 557–565 (1987)

    MathSciNet  MATH  Google Scholar 

  20. Fortin, M.: Calcul numérique des écoulements des fluids de Bingham et des fluides visqueux incompressibles par des méthodes d’éléments finis, Thèse d’Etat Université Pierre et Marie Curie, Paris, 1972

  21. Fortin, M., Glowinski, R.: Augmented Lagrangian Methods. North-Holland, 1983

  22. Fortin, A., Cote, D., Tanguy, P.A.: On the imposition of friction boundary-conditions for the numerical-simulation of Bingham fluid-flows. Comput. Method Appl. Mech. Eng. 88, 97–109 (1991)

    Article  MATH  Google Scholar 

  23. Friedman, A., Kinderlehrer, D.: A one phase Stefan problem. Indiana Univ. Math. J. 24, 1005–1035 (1975)

    MATH  Google Scholar 

  24. Frigaard, I.A.: Stratified exchange flows of two Bingham fluids in an inclined slot. J. Non-Newtonian Fluid Mech. 78, 61–87 (1998)

    Article  MATH  Google Scholar 

  25. Farina, A., Fasano, A.: Flow characteristics of waxy crude oils in laboratory experimental loops. Math. Comput. Modeling 25, 75–86 (1997)

    Article  MATH  Google Scholar 

  26. Frigaard, I.A., Scherzer, O.: Uniaxial exchange flows of two Bingham fluids in a cylindrical duct. IMA J. Appl. Math. 61, 237–266 (1998)

    MathSciNet  MATH  Google Scholar 

  27. Gabay, D.: Application of the method of multipliers to variational inequalities, In: Augmented Lagrangian Methods. M. Fortin and R. Glowinski. eds., North-Holland, 1983

  28. Gastaldi, L., Gilardi, G.: An error estimate for an approximation of a parabolic variational inequality. Bollettino U. M. I. (6) 1-b 1982, pp. 501–521

  29. Glowinski, R.: Lectures on Numerical methods for non-linear variational problems. Tata Institute, Bombay and Springer-Verlag Berlin, 1980

  30. Glowinski, R.: Sur l’écoulement d’un fluide de Bingham dans une conduite cylindrique. Journal de Mécanique 13, 601–621 (1974)

    MATH  Google Scholar 

  31. Glowinski, R.: Splitting methods for the numerical solution of the incompressible Navier-Stokes equations. In: Vistas in applied Mathematics, Optimization Software, New York 1986, pp. 57–95

  32. Glowinski, R., Lions, J.L., Trémolières, R.: Numerical analysis of variational inequalities. Amsterdam, New York-Oxford: North Holland, 1981

  33. Glowinski, R., Le Tallec, P.: Augmented Lagrangian and Operator-Splitting Methods in Nonlinear Mechanics. SIAM, Philadelphia, 1989

  34. Glowinski, R., Marrocco, A.: Sur l’approximation par éléments finis d’ordre un et la résolution par pénalisation-dualité d’une classe de problèmes de Dirichlet non linéaires. C.R. Acad. Sci. Paris 278A, 1649–1652 (1974)

    MATH  Google Scholar 

  35. Glowinski, R., Marrocco, A.: On the solution of a class of nonlinear Dirichlet problems by a penalty-duality method and finite elements of order one. In: Optimization Techniques: IFIP Technical Conference, NOVOSSIBIRSK, U.S.S.R., June 1974, ed. by G.I. Marchuk, Lecture Notes in Computer Sciences, Vol. 27 Springer, Berlin, Heidelberg, New York 1975, pp. 327–333

  36. Glowinski, R., Marrocco, A.: Numerical solution of two-dimensional magneto-static problems by augmented Lagrangian methods. Comput. Mech. Appl. Mech. Eng. 12, 33–46 (1977)

    Article  MATH  Google Scholar 

  37. Guennouni, T., Le Tallec, P.: Calcul à la rupture: Régularisation de Norton-Hoff et Lagrangien augmenté. J. Mécanique 2, 75–99 (1982)

    MATH  Google Scholar 

  38. Hafner, K.: Error Estimates for the finite Element Solution of Quasilinear Obstacle Problems. Numer. Funct. Anal. and Optimiz. 9, 415–433 (1987)

    MathSciNet  MATH  Google Scholar 

  39. Han, W.M.: Quantitative error estimate in modeling the laminar stationary flow of a Bingham fluid. Appl. Math. Comput. 47, 15–24 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  40. He, J.W., Glowinski, R.: Steady Bingham fluid flow in cylindrical pipes: a time dependent approach to the iterative solution. Num. Linear Algebra with Appl. 7, 381–482 (2000)

    Article  MathSciNet  Google Scholar 

  41. Huilgol, R.R., Panizza, M.P.: On the determination of plug-flow region in Bingham fluids through the application of variational inequalities. J. Non-Newtonian Fluid Mech. 58, 207–217 (1995)

    Article  Google Scholar 

  42. Huang, X., Garcia, M.H.: Modeling of non-hydroplaning mudflows on continental slopes. Marine Geology 154, 131–142 (1999)

    Article  Google Scholar 

  43. Huang, X., Garcia, M.H.: A Herschel-Bulkley model for mud flow down a slope. J. Fluid Mech. 374, 305–333 (1998)

    Article  MATH  Google Scholar 

  44. Jerome, J.: Convergent approximations in parabolic variational inequalities II: Hamilton-Jacobi inequalities. Appl. Math. Optim. 8, 265–274 (1982)

    MathSciNet  MATH  Google Scholar 

  45. Johnson, C.: A convergence estimate for an approximation of a parabolic variational inequality. SIAM J. Numer. Anal. 13, 599–606 (1976)

    MATH  Google Scholar 

  46. Kim, J.U.: Semidiscretization method for 3-dimensional motion of a Bingham fluid. SIAM J. Math. Anal. 21, 53–75 (1990)

    MathSciNet  MATH  Google Scholar 

  47. Kuang, P.Q., Kozicki, W.: 2-Phase flow of Bingham fluids thorough porous-media. Mech. Res. Commun. 16, 333–338 (1989)

    Article  MATH  Google Scholar 

  48. Kobayashi, K., Okamura, K., Sakai, T., Sato, M.: Evaluation of the flow rate of an electro-rheological fluid (corn starch kerosene) flowing through a narrow channel formed by a pair of electrodes. Canadian J. Chem. Eng. 74, 394–398 (1996)

    Google Scholar 

  49. Liu, K.F., Mei, C.C.: Long waves in shallow-Water over a layer of Bingham-plastic fluid-mud. 1. Physical aspects. Int. J. Eng. Sci. 31, 125–144 (1993)

    Google Scholar 

  50. Mosco, U., Strang, G.: One-sided approximation and variational inequalities. Bull. Am. Math. Soc. 80, 308–312 (1974)

    MATH  Google Scholar 

  51. Makihara, T., Tanahashi, T.: Theoretical study on equation of motion and yield stress of electrorheological fluid. JSME Int. J. Ser. B 40, 521–528 (1997)

    Google Scholar 

  52. Mariotti, C., Heinrich, P.: Modeling of submarine landslides of rock and soil. Int. J. Numer. Anal. Mech. Geomech. 23, 335–354 (1999)

    Article  MATH  Google Scholar 

  53. Nochetto, R.H.: Sharp L ∞-Error Estimates for Semilinear Elliptic Problems with Free Boundaries. Numer. Math. 54, 243–255 (1988)

    MathSciNet  MATH  Google Scholar 

  54. Pham, T.V., Mitsoulis, E.: Viscoplastic flows in ducts. Canandian J. Chem. Eng. 76, 120–125 (1998)

    Google Scholar 

  55. Rodrigues, J.: Obstacle Problems in Mathematical Physics. Elsevier Science Publishers B.V., 1987

  56. Rulla, J.: Error analysis for implicit approximations to solutions to Cauchy problems. SIAM J. Numer. Anal. 33, 68–87 (1996)

    MathSciNet  MATH  Google Scholar 

  57. Sanchez, F.J.: Application of a first-oder operator splitting method to Bingham fluid flow simulation. Comput. Math. Appl. 36, 71–86 (1998)

    MathSciNet  MATH  Google Scholar 

  58. Savaré, G.: Weak solutions and maximal regularity for abstract evolution inequalities. Advances Math. Sc. Appl. 6, 377–418 (1996)

    MathSciNet  Google Scholar 

  59. Scholz, R.: Numerical solution of the obstacle problem by the penalty method (Time-dependent problems). Numer. Math 49, 255–268 (1986)

    MathSciNet  MATH  Google Scholar 

  60. Scholz, R.: Numerical solution of the obstacle problem by the penalty method. Computing 32, 297–306 (1984)

    MathSciNet  MATH  Google Scholar 

  61. Spann, W.: Error estimates for the approximation of semicoercive variational inequalities. Numer. Math. 69, 103–116 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  62. Tsamopoulos, J.A., Chen, M.F., Borkar, A.V.: On the spin coating of viscoplastic fluids. Rheologica Acta 35, 597–615 (1996)

    Google Scholar 

  63. Vuik, C.: An L 2-Error estimate for an approximation of the solution of a parabolic variational inequality. Numer. Math. 57, 453–471 (1990)

    MathSciNet  MATH  Google Scholar 

  64. Van Kessel, T., Blom, C.: Rheology of cohesive sediments: comparison between a natural and an artificial mud. J. Hydraulic Research 36, 591–612 (1998)

    Google Scholar 

  65. Vradis, G.C., Hammand, K.J.: Strongly coupled block-implicit solution technique for non-Newtonian convective heat transfer problems. Numer. Heat Transfer Pt. B-fund. 33, 79–97 (1998)

    Google Scholar 

  66. Vradis, G.C., Hammand, K.J.: Heat-transfer in flows of non-Newtonian Bingham fluids through axisymmetrical sudden expansions and contractions. Numer. Heat Transfer Pt. A-appl. 28, 339–353 (1995)

    Google Scholar 

  67. Vradis, G.C., Protopapas, A.L.: Macroscopic conductivities for flow of Bingham plastics in porous-media. J. Hydraul. Eng.-Asce 119, 95–108 (1993)

    Google Scholar 

  68. Wu, Y.S., Pruess, K.: A numerical method for simulating non-Newtonian fluid flow and displacement in porous media. Adv. Water Resour. 21, 351–362 (1998)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics and Statistics, SUNY at Stony Brook, Stony Brook, NY, 11794-3600

    Yongmin Zhang

Authors
  1. Yongmin Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yongmin Zhang.

Additional information

Mathematics Subject Classification (2000): 35k85, 65M15, 65M60, 76A05, 76M10

I wish to thank my thesis advisor, Professor Todd Dupont, for his motivation and help on the writing of this paper during my Ph.D study at the University of Chicago. I also want to thank my postdoctoral advisor, Professor James Glimm, for his assistance with improvements to this paper.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhang, Y. Error estimates for the numerical approximation of time-dependent flow of Bingham fluid in cylindrical pipes by the regularization method. Numer. Math. 96, 153–184 (2003). https://doi.org/10.1007/s00211-003-0469-6

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-003-0469-6

Keywords

Search

Navigation