Skip to main content
SpringerLink
Log in

Structural shape optimization using Bézier triangles and a CAD-compatible boundary representation

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

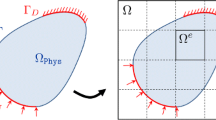
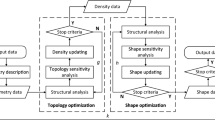
A method for shape optimization using Bézier triangles is introduced. The proposed procedure takes as input a CAD-compatible boundary representation of the domain and outputs an optimal design while maintaining an exact geometry representation at each iteration. The use of a triangular discretization allows the modeling of complex geometric domains, including voids, using a single patch. Some topology changes, such as those resulting from merging boundaries, can also be easily considered. An automatic mesh generator based on a quadtree construction is used to create the mesh. A gradient-based optimization algorithm (the method of moving asymptotes) is employed together with a sensitivity propagation procedure. We apply the method to some standard benchmark problems commonly considered in the literature and show that the proposed method converges to an optimal shape in only a few iterations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Azegami H, Fukumoto S, Aoyama T (2013) Shape optimization of continua using NURBS as basis functions. Struct Multidiscip Optim 47(2):617–622

    MathSciNet  MATH  Google Scholar 

  2. Bennett JA, Botkin ME (1985) Structural shape optimization with geometric description and adaptive mesh refinement. AIAA J 23(3):458–464

    Google Scholar 

  3. Bletzinger KU (1993) Extended method of moving asymptotes based on second-order information. Struct Optim 5(3):175–183

    Google Scholar 

  4. Buhl TC, Pedersen BW, Sigmund O (2000) Stiffness design of geometrically nonlinear structures using topology optimization. Struct Multidiscip Optim 19(2):93–104

    Google Scholar 

  5. Buhl T, Jensen FV, Pellegrino S (2004) Shape optimization of cover plates for retractable roof structures. Comput Struct 82(15–16):1227–1236

    Google Scholar 

  6. Cai S, Zhang W, Zhu J, Gao T (2014) Stress constrained shape and topology optimization with fixed mesh: a B-spline finite cell method combined with level set function. Comput Method Appl Mech Eng 278:361–387

    MathSciNet  MATH  Google Scholar 

  7. Cho S, Ha SH (2008) Isogeometric shape design optimization: exact geometry and enhanced sensitivity. Struct Multidiscip Optim 38(1):53

    MathSciNet  MATH  Google Scholar 

  8. De Leon DM, Alexandersen J, Fonseca JSO, Sigmund O (2015) Stress-constrained topology optimization for compliant mechanism design. Struct Multidiscip Optim 52(5):929–943

    MathSciNet  Google Scholar 

  9. Ding Y (1986) Shape optimization of structures: a literature survey. Comput Struct 24(6):985–1004

    MathSciNet  MATH  Google Scholar 

  10. Engvall L, Evans JA (2016) Isogeometric triangular Bernstein–Bézier discretizations: automatic mesh generation and geometrically exact finite element analysis. Comput Method Appl Mech Eng 304:378–407

    MATH  Google Scholar 

  11. Engvall L, Evans JA (2017) Isogeometric unstructured tetrahedral and mixed-element Bernstein–Bézier discretizations. Comput Method Appl Mech Eng 319:83–123

    MATH  Google Scholar 

  12. Engwirda D (2009) Mesh2d-automatic mesh generation. MatlabCentral: http://www.mathworks.com/matlabcentral/fileexchange/25555-mesh2d-automatic-mesh-generation

  13. Farin G (2001) Curves and surfaces for CAGD: a practical guide, 5th edn. Morgan Kaufmann Publishers Inc., San Francisco

    Google Scholar 

  14. Fußeder D, Simeon B, Vuong A-V (2015) Fundamental aspects of shape optimization in the context of isogeometric analysis. Comput Method Appl Mech Eng 286:313–331

    MathSciNet  MATH  Google Scholar 

  15. George PL, Borouchaki H (2012) Construction of tetrahedral meshes of degree two. Int J Numer Methods Eng 90(9):1156–1182

    MathSciNet  MATH  Google Scholar 

  16. Ghasemi H, Brighenti R, Zhuang X, Muthu J, Rabczuk T (2015) Optimal fiber content and distribution in fiber-reinforced solids using a reliability and NURBS based sequential optimization approach. Struct Multidiscip Optim 51(1):99–112

    MathSciNet  Google Scholar 

  17. Ghasemi H, Park HS, Rabczuk T (2017) A level-set based IGA formulation for topology optimization of flexoelectric materials. Comput Method Appl Mech Engrg 313:239–258

    MathSciNet  MATH  Google Scholar 

  18. Goldman RN, Filip D (1987) Conversion from Bézier rectangles to Bézier triangles. Comput Aided Design 19(1):25–27

    MATH  Google Scholar 

  19. Guo X, Zhang W, Zhong W (2014) Doing topology optimization explicitly and geometrically—a new moving morphable components based framework. J Appl Mech 81(8):081009

    Google Scholar 

  20. Ha SH, Choi KK, Cho S (2010) Numerical method for shape optimization using T-spline based isogeometric method. Struct Multidiscip Optim 42(3):417–428

    MATH  Google Scholar 

  21. Haftka RT, Gürdal Z (2012) Elements of structural optimization, vol 11. Springer, Berlin

    MATH  Google Scholar 

  22. Hassani B, Khanzadi M, Tavakkoli SM (2012) An isogeometrical approach to structural topology optimization by optimality criteria. Struct Multidiscip Optim 45(2):223–233

    MathSciNet  MATH  Google Scholar 

  23. Hojjat M, Stavropoulou E, Bletzinger KU (2014) The vertex morphing method for node-based shape optimization. Comput Method Appl Mech Eng 268:494–513

    MathSciNet  MATH  Google Scholar 

  24. Hou W, Gai Y, Zhu X, Wang X, Zhao C, Xu L, Jiang K, Hu P (2017) Explicit isogeometric topology optimization using moving morphable components. Comput Method Appl Mech Eng 326:694–712

    MathSciNet  MATH  Google Scholar 

  25. Hughes TJR, Cottrell JA, Bazilevs Y (2005) Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput Method Appl Mech Eng 194(39–41):4135–4195

    MathSciNet  MATH  Google Scholar 

  26. Jaxon N, Qian X (2014) Isogeometric analysis on triangulations. Comput Aided Design 46:45–57

    MathSciNet  Google Scholar 

  27. Jia Y, Zhang Y, Xu G, Zhuang X, Rabczuk T (2013) Reproducing kernel triangular B-spline-based FEM for solving PDEs. Comput Method Appl Mech Eng 267:342–358

    MathSciNet  MATH  Google Scholar 

  28. Kiendl J, Schmidt R, Wüchner R, Bletzinger K-U (2014) Isogeometric shape optimization of shells using semi-analytical sensitivity analysis and sensitivity weighting. Comput Method Appl Mech Eng 274:148–167

    MathSciNet  MATH  Google Scholar 

  29. Le C, Norato J, Bruns T, Ha C, Tortorelli D (2010) Stress-based topology optimization for continua. Struct Multidiscip Optim 41(4):605–620

    Google Scholar 

  30. Lian H, Kerfriden P, Bordas S (2016) Implementation of regularized isogeometric boundary element methods for gradient-based shape optimization in two-dimensional linear elasticity. Int J Numer Methods Eng 106(12):972–1017

    MathSciNet  MATH  Google Scholar 

  31. Lian H, Christiansen A, Tortorelli D, Sigmund O, Aage N (2017) Combined shape and topology optimization for minimization of maximal von Mises stress. Struct Multidiscip Optim 55(5):1541–1557

    MathSciNet  Google Scholar 

  32. Lieu QX, Lee J (2019) An isogeometric multimesh design approach for size and shape optimization of multidirectional functionally graded plates. Comput Method Appl Mech Eng 343:407–437

    MathSciNet  MATH  Google Scholar 

  33. Makky SM, Ghalib MA (1979) Design for minimum deflection. Eng Optimiz 4(1):9–13

    Google Scholar 

  34. Manh ND, Evgrafov A, Gersborg AR, Gravesen J (2011) Isogeometric shape optimization of vibrating membranes. Comput Method Appl Mech Eng 200(13–16):1343–1353

    MathSciNet  MATH  Google Scholar 

  35. Meske R, Lauber B, Schnack E (2011) A new optimality criteria method for shape optimization of natural frequency problems. Struct Multidiscip Optim 31(4):295–310

    MathSciNet  MATH  Google Scholar 

  36. Nagy AP, Abdalla MM, Gürdal Z (2010) Isogeometric sizing and shape optimisation of beam structures. Comput Method Appl Mech Eng 199(17–20):1216–1230

    MathSciNet  MATH  Google Scholar 

  37. Nagy AP, Abdalla MM, Gürdal Z (2010) On the variational formulation of stress constraints in isogeometric design. Comput Method Appl Mech Eng 199(41–44):2687–2696

    MathSciNet  MATH  Google Scholar 

  38. Nagy AP, Abdalla MM, Gürdal Z (2011) Isogeometric design of elastic arches for maximum fundamental frequency. Struct Multidiscip Optim 43(1):135–149

    MathSciNet  MATH  Google Scholar 

  39. Nagy AP, IJsselmuiden ST, Abdalla MM (2013) Isogeometric design of anisotropic shells: optimal form and material distribution. Comput Method Appl Mech Eng 264(1):145–162

    MathSciNet  MATH  Google Scholar 

  40. Nanthakumar SS, Valizadeh N, Park HS, Rabczuk T (2015) Surface effects on shape and topology optimization of nanostructures. Comput Mech 56(1):97–112

    MathSciNet  MATH  Google Scholar 

  41. Nanthakumar SS, Lahmer T, Zhuang X, Park HS, Rabczuk T (2016) Topology optimization of piezoelectric nanostructures. J Mech Phys Solids 94:316–335

    MathSciNet  Google Scholar 

  42. Nørtoft P, Gravesen J (2013) Isogeometric shape optimization in fluid mechanics. Struct Multidiscip Optim 48(5):909–925

    MathSciNet  Google Scholar 

  43. Paris J, Navarrina F, Colominas I, Casteleiro M (2009) Topology optimization of continuum structures with local and global stress constraints mechanism design. Struct Multidiscip Optim 39(4):419–437

    MathSciNet  MATH  Google Scholar 

  44. Picelli R, Townsend S, Brampton C, Norato J, Kim HA (2018) Stress-based shape and topology optimization with the level set method. Comput Method Appl Mech Eng 329:1–23

    MathSciNet  MATH  Google Scholar 

  45. Piegl L, Tiller W (2012) The NURBS book, 2nd edn. Springer, Berlin

    MATH  Google Scholar 

  46. Qian X (2010) Full analytical sensitivities in NURBS based isogeometric shape optimization. Comput Method Appl Mech Eng 199(29–32):2059–2071

    MathSciNet  MATH  Google Scholar 

  47. Qian X, Sigmund O (2011) Isogeometric shape optimization of photonic crystals via Coons patches. Comput Method Appl Mech Eng 200(25–28):2237–2255

    MathSciNet  MATH  Google Scholar 

  48. Riehl S, Friederich J, Scherer M, Meske R, Steinmann P (2014) On the discrete variant of the traction method in parameter-free shape optimization. Comput Method Appl Mech Eng 278:119–144

    MathSciNet  MATH  Google Scholar 

  49. Sigmund O (2001) A 99 line topology optimization code written in matlab. Struct Multidiscip Optim 21(2):120–127

    Google Scholar 

  50. Speleers H, Dierckx P, Vandewalle S (2006) Numerical solution of partial differential equations with Powell–Sabin splines. J Comput Appl Math 189(1–2):643–659

    MathSciNet  MATH  Google Scholar 

  51. Speleers, Manni C (2015) Optimizing domain parameterization in isogeometric analysis based on Powell–Sabin splines. J Comput Appl Math 289:68–86

    MathSciNet  MATH  Google Scholar 

  52. Speleers, Manni C, Pelosi F, Sampoli ML (2012) Isogeometric analysis with Powell–Sabin splines for advection–diffusion–reaction problems. Comput Method Appl Mech Eng 221–222:132–148

    MathSciNet  MATH  Google Scholar 

  53. Svanberg K (1987) The method of moving asymptotes—a new method for structural optimization. Int J Numer Methods Eng 24(2):359–373

    MathSciNet  MATH  Google Scholar 

  54. Svanberg K (2004) Some modelling aspects for the Matlab implementation of MMA. KTH Royal Institute of Technology, Stockholm

    Google Scholar 

  55. Valizadeh N, Rabczuk T (2019) Isogeometric analysis for phase-field models of geometric PDEs and high-order PDEs on stationary and evolving surfaces. Comput Method Appl Mech Eng 351:599–642

    MathSciNet  MATH  Google Scholar 

  56. Wall WA, Frenzel MA, Cyron C (2008) Isogeometric structural shape optimization. Comput Method Appl Mech Eng 197(33–40):2976–2988

    MathSciNet  MATH  Google Scholar 

  57. Wang C, Xia S, Wang X, Qian X (2018) Isogeometric shape optimization on triangulations. Comput Method Appl Mech Eng 331:585–622

    MathSciNet  MATH  Google Scholar 

  58. Wang Y, Wang Z, Ia Z, Poh LH (2018) Structural design optimization using isogeometric analysis: a comprehensive review. Comput Model Eng Sci. https://doi.org/10.1002/nme.5593

    Article  Google Scholar 

  59. Xia S, Qian X (2016) Isogeometric analysis with Bézier tetrahedra. Comput Method Appl Mech Eng 316:782–816

    MATH  Google Scholar 

  60. Xia S, Qian X (2018) Generating high-quality high-order parameterization for isogeometric analysis on triangulations. Comput Method Appl Mech Eng 338:1–26

    MathSciNet  MATH  Google Scholar 

  61. Xia S, Wang X, Qian X (2015) Continuity and convergence in rational triangular Bézier spline based isogeometric analysis. Comput Method Appl Mech Eng 297:292–324

    MATH  Google Scholar 

  62. Xie X, Wang S, Xu M, Wang Y (2018) A new isogeometric topology optimization using moving morphable components based on R-functions and collocation schemes. Comput Method Appl Mech Eng 339:61–90

    MathSciNet  MATH  Google Scholar 

  63. Yang RJ, Chen CJ (1996) Stress-based topology optimization. Struct Multidiscip Optim 12(2–3):98–105

    Google Scholar 

  64. Zeng S, Cohen E (2015) Hybrid volume completion with higher-order Bézier elements. Comput Aided Geom D 35–36:180–191

    MATH  Google Scholar 

  65. Zhang W, Yuan J, Zhang J, Guo X (2016) A new topology optimization approach based on Moving Morphable Components (MMC) and the ersatz material model. Struct Multidiscip Optim 53(6):1243–1260

    MathSciNet  Google Scholar 

  66. Zhang W, Chen J, Zhu X, Zhou J, Xue D, Lei X, Guo X (2015) Explicit three dimensional topology optimization via Moving Morphable Void (MMV) approach. Comput Method Appl Mech Eng 322:590–614

    MathSciNet  MATH  Google Scholar 

  67. Zhang W, Huang Q (2017) Unification of parametric and implicit methods for shape sensitivity analysis and optimization with fixed mesh. Int J Numer Methods Eng 109(3):326–344

    MathSciNet  Google Scholar 

  68. Zhang W, Li D, Zhou J, Du Z, Li B, Guo X (2018) A Moving Morphable Void (MMV)-based explicit approach for topology optimization considering stress constraints. Comput Method Appl Mech Eng 334:381–413

    MathSciNet  MATH  Google Scholar 

  69. Zhang W, Yang W, Zhou J, Li D, Guo X (2017) Structural topology optimization through explicit boundary evolution. J Appl Mech 84(1):011011

    Google Scholar 

  70. Zhang W, Zhao L, Cai S (2015) Shape optimization of Dirichlet boundaries based on weighted B-spline finite cell method and level-set function. Comput Method Appl Mech Eng 294:359–383

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors extend their appreciation to the Distinguished Scientist Fellowship Program (DSFP) at King Saud University for funding this work.

Author information

Authors and Affiliations

  1. Department of Computer Engineering, College of Computer and Information Sciences, King Saud University, Riyadh, Saudi Arabia

    Timon Rabczuk & Naif Alajlan

  2. Institute of Structural Mechanics, Bauhaus-Universität Weimar, Marienstr. 15, Weimar, Thüringen, 99423, Germany

    Jorge López, Cosmin Anitescu & Navid Valizadeh

Authors
  1. Jorge López
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Cosmin Anitescu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Navid Valizadeh
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Timon Rabczuk
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Naif Alajlan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Timon Rabczuk.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

López, J., Anitescu, C., Valizadeh, N. et al. Structural shape optimization using Bézier triangles and a CAD-compatible boundary representation. Engineering with Computers 36, 1657–1672 (2020). https://doi.org/10.1007/s00366-019-00788-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-019-00788-z

Keywords

Search

Navigation