Legendre multiwavelet functions for numerical solution of multi-term time-space convection–diffusion equations of fractional order

Abstract

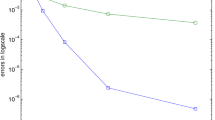
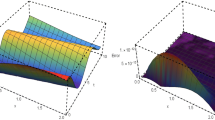
In this study, the Ritz–Galerkin method based on Legendre multiwavelet functions is introduced to solve multi-term time-space convection–diffusion equations of fractional order with variable coefficients and initial-boundary conditions. This method reduces the problem to a set of algebraic equations. The coefficients of approximate solutions are obtained from the coefficients of this system. A convergence analysis for function approximations is also presented together with an upper bound for the error of estimates. Numerical examples are included to demonstrate the validity and applicability of the technique.

Appendix

The ${\hat{m}}^2\times 1$ matrix ${\mathbf {Q}}$ with unknown coefficients $c_{nl}^{ij}$ is as follows:

$$\begin{aligned} {\mathbf {Q}}=\left[ \begin{array}{ccccc} \mathbf {C_1}^T\\ \mathbf {C_2}^T\\ \vdots \\ {\mathbf{C}_{{\hat{\mathbf{m}}}}}^T \\ \end{array} \right] _{{{\hat{m}}}^2 \times 1}, \end{aligned}$$

where

$$\begin{aligned}&\left[ \begin{array}{ccccc} {\mathbf {C}}_1\\ \mathbf {C_2}\\ \vdots \\ {\mathbf {C_{M}}} \\ {\mathbf {C_{M+1}}} \\ \vdots \\ {\mathbf {C_{2M}}} \\ \vdots \\ {\mathbf {C_{(2^p-1)M}}} \\ \vdots \\ {\mathbf {C_{2^pM}}} \\ \end{array} \right] _{{{\hat{m}}} \times {\hat{m}}}=\\&{\left[ \begin{array}{cccccccccccc} c_{00}^{00}&{}c_{00}^{01}&{}\cdots &{} c_{00}^{0(M-1)}&{} c_{00}^{10}&{}\cdots &{} c_{00}^{1(M-1)}&{}\cdots &{} c_{00}^{(2^p-1)0}&{}\cdots &{} c_{00}^{(2^p-1)(M-1)}\\ c_{01}^{00}&{}c_{01}^{01}&{}\cdots &{} c_{01}^{0(M-1)}&{} c_{01}^{10}&{}\cdots &{} c_{01}^{1(M-1)}&{}\cdots &{} c_{01}^{(2^p-1)0}&{}\cdots &{} c_{01}^{(2^p-1)(M-1)}\\ \vdots &{}\vdots &{}\cdots &{}\vdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots \\ c_{0(M-1)}^{00}&{}c_{0(M-1)}^{01}&{}\cdots &{} c_{0(M-1)}^{0(M-1)}&{} c_{0(M-1)}^{10}&{}\cdots &{} c_{0(M-1)}^{1(M-1)}&{}\cdots &{} c_{0(M-1)}^{(2^p-1)0}&{}\cdots &{} c_{0(M-1)}^{(2^p-1)(M-1)}\\ c_{10}^{00}&{}c_{10}^{01}&{}\cdots &{} c_{10}^{0(M-1)}&{} c_{10}^{10}&{}\cdots &{} c_{10}^{1(M-1)}&{}\cdots &{} c_{10}^{(2^p-1)0}&{}\cdots &{} c_{10}^{(2^p-1)(M-1)}\\ \vdots &{}\vdots &{}\cdots &{}\vdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots \\ c_{1(M-1)}^{00}&{}c_{1(M-1)}^{01}&{}\cdots &{} c_{1(M-1)}^{0(M-1)}&{} c_{1(M-1)}^{10}&{}\cdots &{} c_{1(M-1)}^{1(M-1)}&{}\cdots &{} c_{1(M-1)}^{(2^p-1)0}&{}\cdots &{} c_{1(M-1)}^{(2^p-1)(M-1)}\\ \vdots &{}\vdots &{}\cdots &{}\vdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots \\ c_{(2^p-1)0}^{00}&{}c_{(2^p-1)0}^{01}&{}\cdots &{} c_{(2^p-1)0}^{0(M-1)}&{} c_{(2^p-1)0}^{10}&{}\cdots &{} c_{(2^p-1)0}^{1(M-1)}&{}\cdots &{} c_{(2^p-1)0}^{(2^p-1)0}&{}\cdots &{} c_{(2^p-1)0}^{(2^p-1)(M-1)}\\ \vdots &{}\vdots &{}\cdots &{}\vdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots &{}\cdots &{}\vdots \\ c_{(2^p-1)(M-1)}^{00}&{}c_{(2^p-1)(M-1)}^{01}&{}\cdots &{} c_{(2^p-1)(M-1)}^{0(M-1)}&{} c_{(2^p-1)(M-1)}^{10}&{}\cdots &{} c_{(2^p-1)(M-1)}^{1(M-1)}&{}\cdots &{} c_{(2^p-1)(M-1)}^{(2^p-1)0}&{}\cdots &{} c_{(2^p-1)(M-1)}^{(2^p-1)(M-1)}\\ \end{array} \right] .} \end{aligned}$$