Skip to main content
SpringerLink
Log in

A Magnetic Procedure for the Stability Number

  • Original Paper
  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

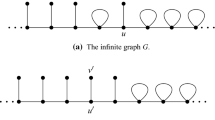
A magnet is a pair u, v of adjacent vertices such that the proper neighbours of u are completely linked to the proper neighbours of v. It has been shown that one can reduce the graph by removing the two vertices u, v of a magnet and introducing a new vertex linked to all common neighbours of u and v without changing the stability number. We prove that all graphs containing no chordless cycle C k (k ≥ 5) and none of eleven forbidden subgraphs can be reduced to a stable set by repeated use of magnets. For such graphs a polynomial algorithm is given to determine the stability number.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Berge C.: Graphes. Gauthier-Villars, Paris (1983)

    MATH  Google Scholar 

  2. Butz, L., Hammer, P.L., Haussmann, D.: Reduction methods for the vertex packing problem. Proceedings of the 7th Conference on Probability Theory, Brasov, Romania 1982, VNU Science Press, Ultrecht, pp. 73–79 (1985)

  3. Chudnovsky M., Robertson N., Seymour P., Thomas R.: The strong perfect graph theorem. Ann. Math. 164, 51–229 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  4. Chvátal, V., Rusu, I.: A note on graphs with no long holes. Proceedings of the DIMACS Workshop on Perfect Graphs at Princeton, NJ (1993)

  5. Fonlupt J., Uhry J.P.: Transformations which preserve perfectness and h-perfectness of graphs. Ann. Discret. Math. 16, 83–85 (1982)

    MATH  MathSciNet  Google Scholar 

  6. Garey, M.R., Johnson, D.S.: Computers and Intractability : A Guide to the Theory of NP-Completeness. W.H. Freeman and Company, New York (1979)

  7. Grötschel M., Lovász L., Schrijver A.: The ellipsoid method and its consequences in combinatorial optimization. Combinatorica 1, 169–197 (1981)

    Article  MATH  MathSciNet  Google Scholar 

  8. Hammer, P.L., Hertz, A.: On a transformation which preserves the stability number. RUTCOR research report 69-91. Rutgers University (1991)

  9. Hayward R.: Weakly triangulated graphs. J. Comb. Theory B 39, 200–209 (1985)

    Article  MATH  MathSciNet  Google Scholar 

  10. Hayward R.: Generating weakly triangulated graphs. J. Graph Theory 21, 67–69 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  11. Hayward, R., Hoang, C.T., Maffray, F.: Optimizing weakly triangulated graphs. Graphs Comb. 5, 339–349 (1989) erratum 6:33–35 (1990)

    Google Scholar 

  12. Hertz A.: On the use of Boolean methods for the computation of the stability number. Discret Appl. Math. 76, 183–203 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  13. Hertz A.: On a transformation which preserves the stability number. Yugosl. J. Oper. Res. 10(1), 1–12 (2000)

    MATH  MathSciNet  Google Scholar 

  14. Lévêque, B.: Coloring graphs: structures and algorithms. Ph.D thesis, Grenoble University. http://tel.archives-ouvertes.fr/tel-00187797 (2007)

  15. Lévêque, B., de Werra, D.: Graph transformations preserving the stability number. Cahier Leibniz 168, INPG Grenoble (2008)

  16. Lovász L.: Normal hypergraphs and the perfect graph conjecture. Discret. Math. 2, 253–267 (1972)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. École Polytechnique de Montréal and GERAD, Montreal, Canada

    Alain Hertz

  2. École Polytechnique Fédérale de Lausanne, Lausanne, Switzerland

    Dominique de Werra

Authors
  1. Alain Hertz
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dominique de Werra
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alain Hertz.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Hertz, A., de Werra, D. A Magnetic Procedure for the Stability Number. Graphs and Combinatorics 25, 707–716 (2009). https://doi.org/10.1007/s00373-010-0886-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00373-010-0886-0

Keywords

Search

Navigation