The combinatorial encoding of disjoint convex sets in the plane

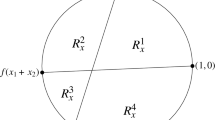
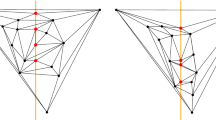
Abstract

We introduce a new combinatorial object, the double-permutation sequence, and use it to encode a family of mutually disjoint compact convex sets in the plane in a way that captures many of its combinatorial properties. We use this encoding to give a new proof of the Edelsbrunner-Sharir theorem that a collection of n compact convex sets in the plane cannot be met by straight lines in more than 2n-2 combinatorially distinct ways. The encoding generalizes the authors’ encoding of point configurations by “allowable sequences” of permutations. Since it applies as well to a collection of compact connected sets with a specified pseudoline arrangement $\mathcal{A}$ of separators and double tangents, the result extends the Edelsbrunner-Sharir theorem to the case of geometric permutations induced by pseudoline transversals compatible with $\mathcal{A}$.