Green investment in a supply chain based on price and quality competition

Abstract

Along with the significant improvement of environmental consciousness, consumers not only consider the price and quality level of products, but also pay more attention to their green level. In order to strengthen the competitive advantage, the manufacturers should consider the green level of products in addition to their price and the quality level. In this paper, we investigate the green investment of two competing manufacturers in a supply chain based on price and quality competition and analyze the effect of green investment on the quality level of the product. The research shows that the manufacturer is willing to make a green investment with a relatively low value of green sensitivity regardless of whether the manufacturer’s rival makes a green investment. Further, we find that the profit of the manufacturer who makes a green investment is greater than the profit of the manufacturer who does not invest regardless of the market size. When both competing manufacturers make green investments, the profit of the manufacturer who is in a large potential market is higher than that of the manufacturer who is in a small potential market. While in a same potential market, the profits of the two competing manufacturers are the same. Finally, we conclude that the green investment counterintuitively will not always improve the quality level of the products.

Appendix: Green investment in a price and quality-based supply chain

Proof of Proposition 1

For given $w_i$ and $q_i$, $i=1,2,$ we conclude that $\pi ^\mathrm{NN}_{r}$ is jointly concave in $p_1$ and $p_2$ because the Hessian matrix of $\pi ^\mathrm{NN}_{r}$, which are $\frac{\partial ^2 \pi ^{\mathrm{N N}}_{r}}{\partial p_{1}^2}=-\,2<0$, $\frac{\partial ^2 \pi ^{\mathrm{N N}}_{r}}{\partial p_{1}^2} \frac{\partial ^2 \pi ^{\mathrm{N N}}_{r}}{\partial p_{2}^2} -\frac{\partial ^2 \pi ^{\mathrm{N N}}_{r}}{\partial p_{1}\partial p_2} \frac{\partial ^2 \pi ^{\mathrm{N N}}_{r}}{\partial p_{2}\partial p_1}=4(1-\alpha ^2)>0$, is negative definite. By solving the following first-order condition, $\frac{\partial \pi ^\mathrm{NN}_{r}}{\partial p_1}=0$ and $\frac{\partial \pi ^\mathrm{NN}_{r}}{\partial p_2}=0$, we obtain the unique optimal retail price (4).

Thus, substituting Eq. (4) into the manufacturers’ profit functions we obtain $\pi ^\mathrm{NN}_{m_{i}}$. To ensure that $\pi ^\mathrm{NN}_{m_{i}}$ is jointly concave in $q_i$ and $w_i$, we require that $\frac{\partial ^2\pi ^{\mathrm{NN}}_{m_{i}}}{\partial q_{i}^2}=-\,\tau <0$, $\frac{\partial ^2\pi ^{\mathrm{NN}}_{m_{i}}}{\partial q_{i}^2} \frac{\partial ^2\pi ^{\mathrm{NN}}_{m_{i}}}{\partial w_{i}^2} -\frac{\partial ^2\pi ^{\mathrm{NN}}_{m_{i}}}{\partial q_{i}\partial w_{i}} \frac{\partial ^2\pi ^{\mathrm{NN}}_{m_{i}}}{\partial w_{i}\partial q_{i}}=\tau -\frac{1}{4}>0$, which implies the Hessian matrix of $\pi ^\mathrm{NN}_{m_{i}}$ is negative definite. By solving the first-order condition, that is $\frac{\pi ^\mathrm{NN}_{m_{i}}}{\partial q_{i}}=0$ and $\frac{\pi ^\mathrm{NN}_{m_{i}}}{\partial w_i}=0$, we have the unique optimal quality levels $q_{i}^{\mathrm{NN}}$ and wholesale price $w_{i}^{\mathrm{NN}}$ (6).

Thus substituting Eq. (6) into Eq. (4) and demands function (2), the optimal retail price $p_{i}^{\mathrm{NN}}$ and the demand $D_{i}^{\mathrm{NN}}$ (7) are obtained.

Substituting Eqs. (6) and (7) into $\pi ^\mathrm{NN}_{r}$ and $\pi ^\mathrm{NN}_{m_{i}}$, we obtain the retailer’s and the manufacturers’ optimal profits $\pi ^\mathrm{NN}_{m_{i}}$ and $\pi ^\mathrm{NN}_{r}$ (8).

The proof is completed. $\square$

Proof of Corollaries 1

From the concavity of the retailer’s profit and the manufacturers’ profit, we derive $4\tau -1>0$. In order to make decision variables to be positive, we require

$$\begin{aligned} \max \left\{ -\,(4\tau -1),-\frac{(4\tau -1)a_{i}}{a_{j}}\right\}<2\alpha \tau -\beta <4\tau -1, \end{aligned}$$

(24)

or

$$\begin{aligned} 2\alpha \tau -\beta <\min \left\{ -\,(4\tau -1),\, -\,\frac{(4\tau -1)a_{i}}{a_{j}}\right\} . \end{aligned}$$

(25)

For $f(2\alpha \tau -\beta )=a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j}\left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right)$, we conclude that $f(2\alpha \tau -\beta )>0$ when $a_i<a_j$, and $f(2\alpha \tau -\beta )$ has two different roots $\frac{-\,a_i\pm \sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1)$ when $a_i>a_j$. Therefore, we have $f(2\alpha \tau -\beta )<0$ when

$$\begin{aligned}&\frac{-\,a_i-\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1)\nonumber \\&\quad<2\alpha \tau -\beta <\frac{-\,a_i+\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1), \end{aligned}$$

(26)

and $f(2\alpha \tau -\beta )>0$ when

$$\begin{aligned} 2\alpha \tau -\beta> & {} \frac{-\,a_i+\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1) ~~or ~~ 2\alpha \tau -\beta \nonumber \\< & {} -\,\frac{-\,a_i-\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1). \end{aligned}$$

(27)

From (24) (25) and (26), we can derive $f(2\alpha \tau -\beta )<0$ when

$$\begin{aligned} -\,(4\tau -1)<2\alpha \tau -\beta <\frac{-\,a_i+\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1), \end{aligned}$$

(28)

or

$$\begin{aligned} \frac{-\,a_i-\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1)<2\alpha \tau -\beta <\frac{-\,a_i}{a_{j}}(4\tau -1). \end{aligned}$$

(29)

From (24) (25) and (27), we can derive $f(2\alpha \tau -\beta )>0$ when

$$\begin{aligned} \frac{-\,a_i+\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1)<2\alpha \tau -\beta <4\tau -1, \end{aligned}$$

(30)

or

$$\begin{aligned} 2\alpha \tau -\beta <\frac{-\,a_i-\sqrt{a_{i}^{2}-a_{j}^{2}}}{a_{j}}(4\tau -1). \end{aligned}$$

(31)

• (i) For any given $\alpha$, we have

  $$\begin{aligned} \frac{\partial q_i^{\mathrm{NN}}}{\partial \alpha }= & {} {\frac{2\,\tau \, \left( a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j} \left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right) \right) }{ \left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}- 16\,{\tau }^{2}+8\,\tau -1 \right) ^{2}}},\\ \frac{\partial w_i^{\mathrm{NN}}}{\partial \alpha }= & {} {\frac{4\,\tau ^2\, \left( a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j} \left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right) \right) }{ \left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}- 16\,{\tau }^{2}+8\,\tau -1 \right) ^{2}}},\\ \frac{\partial D_i^{\mathrm{NN}}}{\partial \alpha }= & {} {\frac{2\,\tau ^2\, \left( a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j}\left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right) \right) }{ \left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}- 16\,{\tau }^{2}+8\,\tau -1 \right) ^{2}}},\\ \frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \alpha }= & {} {\frac{-2\,{\tau }^{2} \left( 4\,\tau -1 \right) \left( a_{j}\, \left( 2\,\tau \,\alpha -\beta \right) +a_{i}\, \left( 4\,\tau -1 \right) \right) \left( a_{j}\, \left( 2\,\tau \,\alpha -\beta \right) ^{2}+a_{j}\, \left( 4\,\tau -1 \right) ^{2}+2\,a_{i}\, \left( 4\,\tau -1 \right) \left( 2\,\tau \,\alpha -\beta \right) \right) }{ \left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}- 16\,{\tau }^{2}+8\,\tau -1 \right) ^{3}}}. \end{aligned}$$

  When $a_i<a_j$, we have $f(2\alpha \tau -\beta )>0$. Therefore, we conclude $\frac{\partial q_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \alpha }>0$. When $a_i>a_j$, we have $f(2\alpha \tau -\beta )<0$ from (28) and (29). Therefore, we obtain $\frac{\partial q_i^{\mathrm{NN}}}{\partial \alpha }<0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \alpha }<0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \alpha }<0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \alpha }<0$. Similarly, we obtain $f(2\alpha \tau -\beta )>0$ from (30) and (31), which make $\frac{\partial q_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \alpha }>0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \alpha }>0$ to be hold.

• (ii) For any given $\beta$, we have

  $$\begin{aligned} \frac{\partial q_i^{\mathrm{NN}}}{\partial \beta }= & {} -\,{\frac{ a_{j} \left( 2\,\tau \,\alpha -\beta \right) ^{2}+ a_{j} \left( 4\,\tau -1 \right) ^{2}+2\,a_{i}\left( 4\,\tau -1 \right) \left( 2\,\tau \,\alpha -\beta \right) }{\left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}- 16\,{\tau }^{2}+8\,\tau -1 \right) ^{2}}},\\ \frac{\partial w_i^{\mathrm{NN}}}{\partial \beta }= & {} -\,{\frac{2\,\tau \, \left( a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j} \left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right) \right) }{\left( 4\,{\alpha }^{2}{\tau }^{2} -4\,\alpha \,\beta \,\tau +{\beta }^{2}-16\,{\tau }^{2}+8\,\tau -1\right) ^{2}}},\\ \frac{\partial D_i^{\mathrm{NN}}}{\partial \beta }= & {} -\,{\frac{\tau \, \left( a_{j} \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ a_{j}\left( 4\,\tau -1 \right) ^{2} +2\,a_{i} \left( 4\,\tau -1 \right) \left( 2\,\alpha \,\tau -\beta \right) \right) }{\left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2}-16\,{\tau }^{2}+8\,\tau -1 \right) ^{2}}},\\ \frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \beta }= & {} {\frac{\tau \left( 4\,\tau -1 \right) \left( a_{j}\, \left( 2\,\tau \,\alpha -\beta \right) +a_{i}\, \left( 4\,\tau -1 \right) \right) \left( a_{j}\, \left( 2\,\tau \,\alpha -\beta \right) ^{2}+a_{j}\, \left( 4\,\tau -1 \right) ^{2}+2\,a_{i}\, \left( 4\,\tau -1 \right) \left( 2\,\tau \,\alpha -\beta \right) \right) }{\left( 4\,{\alpha }^{2}{\tau }^{2}-4\,\alpha \,\beta \,\tau +{\beta }^{2} -16\,{\tau }^{2}+8\,\tau -1 \right) ^{3}}}. \end{aligned}$$

  When $a_i<a_j$, we have $f(2\alpha \tau -\beta )>0$. Therefore, we conclude $\frac{\partial q_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \beta }<0$. When $a_i>a_j$, we have $f(2\alpha \tau -\beta )<0$ from (28) and (29). Therefore, we obtain $\frac{\partial q_i^{\mathrm{NN}}}{\partial \beta }>0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \beta }>0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \beta }>0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \beta }>0$. Similarly, we obtain $f(2\alpha \tau -\beta )>0$ from (30) and (31), which make $\frac{\partial q_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial w_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial D_i^{\mathrm{NN}}}{\partial \beta }<0$, $\frac{\partial \pi _{m_{i}}^{\mathrm{NN}}}{\partial \beta }<0$ to be hold. $\square$

Proof of Proposition 2

By the same reason in the proof of Proposition 1, we conclude that $\pi ^\mathrm{GN}_{r}$ is jointly concave in $p_1$ and $p_2$. By solving $\frac{\partial \pi ^\mathrm{GN}_{r}}{\partial p_1}=0$ and $\frac{\partial \pi ^\mathrm{GN}_{r}}{\partial p_2}=0$, we obtain the unique optimal retail price (13).

Thus substituting Eq. (13) into the manufacturer $M_1$’s profit functions $\pi ^\mathrm{GN}_{m_{1}}$, we obtain that the Hessian matrix of $\pi ^\mathrm{GN}_{m_{1}}$ is as follows:

$$\begin{aligned} H(\pi _{m_{1}}^{GN})=\begin{pmatrix} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial q_{1}^2} &{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial q_{1}\partial w_1} &{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial q_{1}\partial \theta _1}\\ \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial w_{1}\partial q_1} &{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial w_{1}^2} &{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial w_{1}\partial \theta _1}\\ \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial \theta _1\partial q_{1}}&{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial \theta _1\partial w_{1}} &{} \frac{\partial ^2 \pi _{m_{1}}^{GN}}{\partial \theta _{1}^2}\\ \end{pmatrix} =\begin{pmatrix} -\,\tau &{} \frac{1}{2} &{}0 \\ \frac{1}{2}&{} -\,1 &{}\frac{1}{2}\gamma \\ 0&{} \frac{1}{2}\gamma &{}-\,\mu \end{pmatrix}. \end{aligned}$$

To ensure $\pi ^\mathrm{GN}_{m_{1}}$ is jointly concave in $q_1$, $w_1$ and $\theta _1$, we need that the leading principal minors $S_1=-\,\tau <0$, $S_2=\tau -\frac{1}{4}>0$, $S_3=-\,\tau \mu +\frac{1}{4}\mu +\frac{1}{4}\gamma ^2\tau <0$, which implies the Hessian matrix is negative definite.

Similarly, substituting Eq. (13) into the manufacturer $M_2$’s profit functions $\pi ^\mathrm{GN}_{m_{2}}$, we obtain that the Hessian matrix of $\pi ^\mathrm{GN}_{m_{2}}$ is as follows:

$$\begin{aligned} H(\pi _{m_{2}}^{GN})=\begin{pmatrix} \frac{\partial ^2 \pi _{m_{2}}^{GN}}{\partial q_{2}^2} &{} \frac{\partial ^2 \pi _{m_{2}}^{GN}}{\partial q_{2}\partial w_2} \\ \frac{\partial ^2 \pi _{m_{2}}^{GN}}{\partial w_{2}\partial q_2} &{} \frac{\partial ^2 \pi _{m_{2}}^{GN}}{\partial w_{2}^2} \end{pmatrix} =\begin{pmatrix} -\,\tau &{}\frac{1}{2}\\ \frac{1}{2} &{} -\,1 \end{pmatrix}. \end{aligned}$$

To ensure $\pi ^\mathrm{GN}_{m_{2}}$ is jointly concave in $q_2$ and $w_2$, we need that the leading principal minor $S_1=-\,\tau <0$, $S_2=\tau -\frac{1}{4}>0$. In order to maintain the concavity of the profit function $\pi ^\mathrm{GN}_{m_{1}}$ and $\pi ^\mathrm{GN}_{m_{2}}$ , the condition $\tau >\frac{1}{4}$ and $\mu (4\tau -1)>\gamma ^2 \tau$ must be hold simultaneously.

Solving the first-order condition, that is $\frac{\pi ^\mathrm{GN}_{m_{i}}}{\partial q_{i}}=0$, $\frac{\pi ^\mathrm{GN}_{m_{i}}}{\partial w_i}=0$, and $\frac{\pi ^\mathrm{GN}_{m_{1}}}{\partial \theta _{1}}=0$, the unique optimal quality levels $q_{i}^\mathrm{GN}$, optimal wholesale price $w_{i}^\mathrm{GN}$ and optimal the green level $\theta _1$ (15) are derived.

Thus substituting Eq. (15) into Eq. (13) and demands function (11), we get the optimal retail price $p_{i}^\mathrm{GN}$ and the demand $D_{i}^\mathrm{GN}$ (16).

Substituting Eqs. (15) and (16) into $\pi ^\mathrm{GN}_{m_{i}}$ and $\pi ^\mathrm{GN}_{r}$, we conclude the retailer’s optimal profit $\pi ^\mathrm{GN}_{r}$ and the manufacturers’ optimal profits $\pi ^\mathrm{GN}_{m_{i}}$ (17).

The proof is completed. $\square$

Proof of Corollaries 2

From the concavity of the retailer’s profit and the manufacturers’ profit, we derive $4\tau -1>0$ and $\gamma ^2 \tau -\mu (4\tau -1)<0$. In order to make decision variables to be positive, we require $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$.

We assume

$$\begin{aligned} L_{1}= & {} \left( 4\,\tau -1 \right) \left( -1/2\,{\gamma }^{2} \tau +\mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) , \\ L_{2}= & {} \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1\right) -{\gamma }^{2}\tau \right) \right) ,\\ L_{3}= & {} \left( \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}\right. \\&\left. +\,\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) ,\\ L_{4}= & {} \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) . \end{aligned}$$

• (i) For any given $\alpha$, we have

  $$\begin{aligned}&\frac{\partial q_1^\mathrm{GN}}{\partial \alpha }= {\frac{\tau \,\mu \, \left( 4\,a_{1}\, \left( 4\,\tau -1 \right) \left( -\,\frac{1}{2}\,{\gamma }^{2}\tau +\mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) +2\,a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau - \beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\, \tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2 \,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \, \tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial q_2^\mathrm{GN}}{\partial \alpha }= -\,{\frac{2\,\tau \, \left( a_{1}\, \left( \left( \mu \, \left( 2\, \alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{ \gamma }^{2}\tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\, \alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial w_1^\mathrm{GN}}{\partial \alpha }= {\frac{4\,{\tau }^{2}\mu \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2} \tau \right) +a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial w_2^\mathrm{GN}}{\partial \alpha }= -\,{\frac{4\,{\tau }^{2} \left( a_{1}\, \left( \left( \mu \, \left( 2\, \alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{ \gamma }^{2}\tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\, \alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial \theta _1^\mathrm{GN}}{\partial \alpha }= {\frac{2\,\gamma \,{\tau }^{2} \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2} \tau \right) +a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial D_1^\mathrm{GN}}{\partial \alpha }= {\frac{2\,{\tau }^{2}\mu \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2} \tau \right) +a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial D_2^\mathrm{GN}}{\partial \alpha }= -{\frac{ \left( a_{1}\, \left( \left( \mu \, \left( 2\,\alpha \,\tau - \beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\,\alpha \,\tau - \beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) {\tau }^{2}}{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}}, \nonumber \\&\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \alpha } = -{\frac{2\,{\tau }^{2}\left( 2\,a_{1}\,L_{1}+a_{2}\, L_{2}\right) \left( \mu \,\left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \left( a_{1}\,\left( 4\,\tau -1 \right) +a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2} \tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}}},\nonumber \\&\frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \alpha }= {\frac{8\,{\tau }^{2} \left( \tau -1/4 \right) \left( a_{1}\, L_{3} +a_{2}\, L_{4} \right) \left( a_{1}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) +a_{2}\, \left( \mu \, \left( 4\, \tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \,\tau -{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}}}, \end{aligned}$$

  (32)

  When $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$, from (32), we can conclude that $\frac{\partial q_1^\mathrm{GN}}{\partial \alpha }>0$, $\frac{\partial q_2^\mathrm{GN}}{\partial \alpha }<0$, $\frac{\partial w_1^\mathrm{GN}}{\partial \alpha }>0$, $\frac{\partial w_2^\mathrm{GN}}{\partial \alpha }<0$, $\frac{\partial \theta _1^\mathrm{GN}}{\partial \alpha }>0$, $\frac{\partial D_1^\mathrm{GN}}{\partial \alpha }>0$, $\frac{\partial D_2^\mathrm{GN}}{\partial \alpha }<0$, $\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \alpha }>0$, $\frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \alpha }<0$.

• (ii) For any given $\beta$, we have

  $$\begin{aligned}&\frac{\partial q_1^\mathrm{GN}}{\partial \beta }= -\,{\frac{\mu \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) +a_{ 2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4 \,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2} \tau \right) \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \, \mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial q_2^\mathrm{GN}}{\partial \beta }= {\frac{a_{1}\, \left( \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\,\alpha \,\tau - \beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) }{ \left( 2\,\alpha \,\tau -\beta -4\, \tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial w_1^\mathrm{GN}}{\partial \beta }= -\,{\frac{2\,\tau \,\mu \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2} \tau \right) +a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial w_2^\mathrm{GN}}{\partial \beta }= {\frac{2\,\tau \, \left( a_{1}\, \left( \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\, \tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2} \tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\,\alpha \,\tau - \beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau - \beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2} \tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial \theta _1^\mathrm{GN}}{\partial \beta }= -\,{\frac{\gamma \,\tau \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\,\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2} \tau \right) +a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \right) }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau - {\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial D_1^\mathrm{GN}}{\partial \beta }= -\,{\frac{\tau \,\mu \, \left( a_{1}\, \left( 4\,\tau -1 \right) \left( 2\mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) + a_{2}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{ \gamma }^{2}\tau \right) \right) \right) }{ \left( 2\,\alpha \,\tau - \beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2} \tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial D_2^\mathrm{GN}}{\partial \beta }= {\frac{\tau \, \left( a_{1}\, \left( \left( \mu \, \left( 2\,\alpha \, \tau -\beta \right) -{\gamma }^{2}\tau \right) ^{2}+\mu \, \left( 4\,\tau -1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) +a_{2}\, \left( 2\,\mu \, \left( 2\,\alpha \,\tau - \beta \right) -{\gamma }^{2}\tau \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau - \beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2} \tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \beta }= {\frac{\tau \, \left( 2\,a_{1}\,L_{1}+a_{2}\, L_{2}\right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \left( a_{1}\, \left( 4\,\tau -1 \right) +a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}}},\nonumber \\&\frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \beta }= -{\frac{8\,\tau \, \left( \tau -1/4 \right) \left( a_{1}\, L_{3}+a_{2}\,L_{4}\right) \left( a_{1}\, \left( \mu \, \left( 62\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) +a_{2}\, \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \,\tau -{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}}}, \end{aligned}$$

  (33)

  When $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$, from (33), we can derive that $\frac{\partial q_1^\mathrm{GN}}{\partial \beta }<0$, $\frac{\partial q_2^\mathrm{GN}}{\partial \beta }>0$, $\frac{\partial w_1^\mathrm{GN}}{\partial \beta }<0$, $\frac{\partial w_2^\mathrm{GN}}{\partial \beta }>0$, $\frac{\partial \theta _1^\mathrm{GN}}{\partial \beta }<0$, $\frac{\partial D_1^\mathrm{GN}}{\partial \beta }<0$, $\frac{\partial D_2^\mathrm{GN}}{\partial \beta }>0$, $\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \beta }<0$, $\frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \beta }>0$.

• (iii) For any given $\gamma$, we have

  $$\begin{aligned} \frac{\partial q_1^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{2\,\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma \,\tau }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \, \tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial q_2^\mathrm{GN}}{\partial \gamma }= & {} {\frac{2\,\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) + a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma \,\tau }{ \left( 2\, \alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \, \tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial w_1^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{4\,{\tau }^{2}\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau - \beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2 \,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma } ^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial w_2^\mathrm{GN}}{\partial \gamma }= & {} {\frac{4\,{\tau }^{2}\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma }{ \left( 2 \,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial \theta _1^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{\tau \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) +a _{1}\, \left( 4\,\tau -1 \right) \right) \left( \mu \, \left( 2\, \alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) +{\gamma }^{ 2}\tau \right) }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\, \tau -1 \right) -{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial D_1^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{2\,{\tau }^{2}\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau - \beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2 \,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma } ^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial D_2^\mathrm{GN}}{\partial \gamma }= & {} {\frac{2\,{\tau }^{2}\mu \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) \gamma }{ \left( 2 \,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{\mu \,{\tau }^{2}\gamma \, \left( a_{2}\, \left( 2\,\alpha \,\tau -\beta \right) +a_{1}\, \left( 4\,\tau -1 \right) \right) ^{2} \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) +{\gamma }^{2}\tau \right) }{ \left( \mu \, \left( 2\,\alpha \, \tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) ^{3} \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2}}},\nonumber \\ \frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \gamma }= & {} -{\frac{8\,\gamma \,\mu \,{\tau }^{2} \left( a_{1} \left( \mu \left( 2\, \alpha \tau -\beta \right) -{\gamma }^{2}\tau \right) +a_{2} \left( \mu \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \left( \tau -1/4 \right) \left( a_{1} \left( 4\,\tau -1 \right) +a_{2} \left( 2\,\alpha \tau -\beta \right) \right) }{ \left( \mu \, \left( 2\, \alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{ 2}\tau \right) ^{3} \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2}}}.\nonumber \\ \end{aligned}$$

  (34)

  When $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$, from (33), we can derive that $\frac{\partial q_1^\mathrm{GN}}{\partial \gamma }>0$, $\frac{\partial q_2^\mathrm{GN}}{\partial \gamma }<0$, $\frac{\partial w_1^\mathrm{GN}}{\partial \gamma }>0$, $\frac{\partial w_2^\mathrm{GN}}{\partial \gamma }<0$, $\frac{\partial \theta _1^\mathrm{GN}}{\partial \gamma }>0$, $\frac{\partial D_1^\mathrm{GN}}{\partial \gamma }>0$, $\frac{\partial D_2^\mathrm{GN}}{\partial \gamma }<0$, $\frac{\partial \pi _{m_{2}}^\mathrm{GN}}{\partial \gamma }<0$.

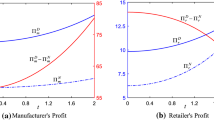
For the profit of manufacturer $\mathrm{M_1}$ with a green investment, we have $\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \gamma }>0$ if $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta < \frac{\mu (4\tau -1)-\gamma ^{2}\tau }{\mu }$, we have $\frac{\partial \pi _{m_{1}}^\mathrm{GN}}{\partial \gamma }<0$ if $\max \left\{ \frac{\gamma ^{2}\tau }{\mu },\frac{\mu (4\tau -1)-\gamma ^{2}\tau }{\mu } \right\}< 2\alpha \tau -\beta <4\tau -1$. $\square$

Proof of Proposition 3

By the same reason in the proof of Proposition 1, we conclude that $\pi ^\mathrm{GG}_{r}$ is jointly concave in $p_1$ and $p_2$. By solving $\frac{\partial \pi ^\mathrm{GG}_{r}}{\partial p_1}=0$ and $\frac{\partial \pi ^\mathrm{GG}_{r}}{\partial p_2}=0$, we obtain the unique optimal retail price (19).

Thus substituting Eq. (19) into the manufacturers’ profit functions $\pi ^\mathrm{GG}_{m_{i}}$, we obtain that the Hessian matrix of $\pi ^\mathrm{GG}_{m_{i}}$ is as follows:

$$\begin{aligned} H(\pi _{m_{i}}^{GN})=\begin{pmatrix} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial q_{1}^2} &{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial q_{1}\partial w_1} &{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial q_{1}\partial \theta _1}\\ \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial w_{1}\partial q_1} &{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial w_{1}^2} &{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial w_{1}\partial \theta _1}\\ \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial \theta _1\partial q_{1}}&{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial \theta _1\partial w_{1}} &{} \frac{\partial ^2 \pi _{m_{i}}^{GN}}{\partial \theta _{1}^2}\\ \end{pmatrix} =\begin{pmatrix} -\,\tau &{} \frac{1}{2} &{}0 \\ \frac{1}{2}&{} -\,1 &{}\frac{1}{2}\gamma \\ 0&{} \frac{1}{2}\gamma &{}-\,\mu \end{pmatrix}. \end{aligned}$$

To ensure $\pi ^\mathrm{GG}_{m_{i}}$ is jointly concave in $q_i$, $w_i$ and $\theta _i$, we need that the leading principal minors $S_1=-\,\tau <0$, $S_2=\tau -\frac{1}{4}>0$, $S_3=-\,\tau \mu +\frac{1}{4}\mu +\frac{1}{4}\gamma ^2\tau <0$, which implies the Hessian matrix is negative definite.

By solving the first-order condition, that is $\frac{\pi ^\mathrm{GG}_{m_{i}}}{\partial q_{i}}=0$, $\frac{\pi ^\mathrm{GG}_{m_{i}}}{\partial w_i}=0$, and $\frac{\pi ^\mathrm{GG}_{m_{i}}}{\partial \theta _{i}}=0$, the unique optimal quality levels $q_{i}^\mathrm{GG}$, optimal wholesale price $w_{i}^\mathrm{GG}$ and optimal the green level $\theta _{i}^\mathrm{GG}$ (21) are derived.

By substituting Eq. (21) into Eq. (19) and demands function (1), the optimal retail price $p_{i}^\mathrm{GG}$ and the demand $D_{i}^\mathrm{GG}$ (22) are obtained.

Substituting Eqs. (21) and (22) into $\pi ^\mathrm{GG}_{m_{i}}$ and $\pi ^\mathrm{GG}_{r}$, we get the retailer’s optimal profit $\pi ^\mathrm{GG}_{r}$ and the manufacturers’ optimal profits $\pi ^\mathrm{GG}_{m_{i}}$ (23).

The proof is completed. $\square$

Proof of Corollaries 3

From the concavity of the retailer’s profit and the manufacturers’ profit, we have $4\tau -1>0$ and $\gamma ^2 \tau -\mu (4\tau -1)<0$. In order to make decision variables to be positive, we need $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$. We assume

$$\begin{aligned} T_1= & {} a_{i}\, \left( 2\,{\gamma }^{2}\tau -2\,\mu \, \left( 4\,\tau -1 \right) \right) \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^ {2}\tau \right) \\&+a_{j}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta -4\, \tau +1 \right) \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2} \tau \right) \right. \\&+ \left( {\gamma }^{2}\tau -\mu \, \left( 2\,\alpha \,\tau - \beta \right) \right) \\&\left. \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -2\,{\gamma }^{2}\tau \right) \right) . \end{aligned}$$

We can conclude $T_1<0$.

• (i) For any given $\alpha$, we have

  $$\begin{aligned}&\frac{\partial q_i^\mathrm{GG}}{\partial \alpha }= -{\frac{2\,\tau \, T_1 }{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial w_i^\mathrm{GG}}{\partial \alpha }= -{\frac{4\,{\tau }^{2} T_1 }{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial \theta _i^\mathrm{GG}}{\partial \alpha }= -{\frac{2\,{\tau }^{2}\gamma \, T_1 }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{ 2}\mu }},\nonumber \\&\frac{\partial D_i^\mathrm{GG}}{\partial \alpha }= -{\frac{2\,{\tau }^{2} T_1 }{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\&\frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \alpha }= {\frac{2\,{\tau }^{2} \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^ {2}\tau \right) \left( a_{i}\, \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) +a_{j}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \right) { T_1}}{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \, \tau -2\,{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}\mu }}. \end{aligned}$$

  (35)

  When $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$, from (35), we can conclude that $\frac{\partial q_i^\mathrm{GG}}{\partial \alpha }>0$, $\frac{\partial w_i^\mathrm{GG}}{\partial \alpha }>0$, $\frac{\partial \theta _i^\mathrm{GG}}{\partial \alpha }>0$, $\frac{\partial D_i^\mathrm{GG}}{\partial \alpha }>0$, $\frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \alpha }>0$.

• (ii) For any given $\beta$, we have

  $$\begin{aligned} \frac{\partial q_i^\mathrm{GG}}{\partial \beta }= & {} {\frac{T_1 }{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{\gamma }^{2}\tau -\beta \, \mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\ \frac{\partial w_i^\mathrm{GG}}{\partial \beta }= & {} {\frac{2\,\tau \, T_1 }{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\ \frac{\partial \theta _i^\mathrm{GG}}{\partial \beta }= & {} {\frac{\tau \,\gamma \, T_1 }{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}\mu }},\nonumber \\ \frac{\partial D_i^\mathrm{GG}}{\partial \beta }= & {} -{\frac{\tau \, T_1}{ \left( 2\,\alpha \, \tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,\alpha \,\mu \,\tau -2\,{ \gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{2}}},\nonumber \\ \frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \beta }= & {} -{\frac{{\tau } \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^ {2}\tau \right) \left( a_{i}\, \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) +a_{j}\, \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \right) { T_1}}{ \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{3} \left( 2\,\alpha \,\mu \, \tau -2\,{\gamma }^{2}\tau -\beta \,\mu +4\,\mu \,\tau -\mu \right) ^{3}\mu }}. \end{aligned}$$

  (36)

  When $\frac{\gamma ^{2}\tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$, we can conclude from (36) that $\frac{\partial q_i^\mathrm{GG}}{\partial \beta }<0$, $\frac{\partial w_i^\mathrm{GG}}{\partial \beta }<0$, $\frac{\partial \theta _i^\mathrm{GG}}{\partial \beta }<0$, $\frac{\partial D_i^\mathrm{GG}}{\partial \beta }>0$, $\frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \beta }<0$.

• (iii) For any given $\gamma$, we have

  $$\begin{aligned} \frac{\partial q_i^\mathrm{GG}}{\partial \gamma }= & {} {\frac{ 2\,\left( a_{i}-a_{j} \right) \mu \,\tau \,\gamma }{ \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -2\,{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial w_i^\mathrm{GG}}{\partial \gamma }= & {} {\frac{4\, \left( a_{i}-a_{j} \right) \mu \,{\tau }^{2}\gamma }{ \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -2\,{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial D_i^\mathrm{GG}}{\partial \gamma }= & {} {\frac{2\, \left( a_{i}-a_{j} \right) \mu \,{\tau }^{2}\gamma }{ \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -2\,{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial \theta _i^\mathrm{GG}}{\partial \gamma }= & {} {\frac{2a_{i}\, L_{5}+2a_{j}\, L_{6}}{\mu \, \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\, \tau -1 \right) -2\,{\gamma }^{2}\tau \right) ^{2}}},\nonumber \\ \frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \gamma }= & {} {\frac{{\tau }^{2}\gamma \, L_{7}L_{8}}{\mu \, \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^{2} \left( 2\,{\gamma }^{2}\tau -\mu \, \left( 2\,\alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) \right) ^{3}}},\nonumber \\ \end{aligned}$$

  (37)

  where

  $$\begin{aligned} L_{5}= & {} \left( \mu \,\tau \, \left( 2\,\alpha \,\tau -\beta +4\, \tau -1 \right) \left( 3\,{\gamma }^{2}\tau -\mu \, \left( 4\,\tau -1 \right) \right) \right. \\&\left. -2\,{\gamma }^{2}{\tau }^{2} \left( {\gamma }^{2}\tau + \mu \, \left( 4\,\tau -1 \right) \right) \right) ,\\ L_{6}= & {} \mu \,\tau \, \left( 2\,\alpha \left( 3\,{\gamma }^{2}\tau -\mu \, \left( 2\, \alpha \,\tau -\beta \right) \right) \right. \\&\left. \tau \left( 3\,{\gamma }^{2}\tau - \mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) \right. \\&\left. -\beta \left( 3\,{\gamma }^{2} \tau -\mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) -1 \right) \\&+4\,\mu \,\tau ^{2} \left( 3\,{\gamma }^{2}\tau -\mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) \\&-2\,{\gamma }^{2}{\tau }^{2} \left( {\gamma }^{2} \tau +\mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) ,\\ L_{7}= & {} \left( a_{i}\, \left( {\gamma }^{2}\tau -\mu \, \left( 4\,\tau -1 \right) \right) \right. \\&\left. +a_{j}\, \left( {\gamma }^{2}\tau - \mu \, \left( 2\,\alpha \,\tau -\beta \right) \right) \right) ,\\ L_{8}= & {} 2\, \left( a_{i}-a_{j} \right) \left( {\gamma }^{2}\tau -\mu \, \left( 4\,\tau -1 \right) \right) \\&\times \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) \right) \\&-\, \left( a_{i}\,\left( {\gamma }^{2}\tau -\mu \, \left( 4\,\tau -1 \right) \right) \right. \\&\left. +a_{j}\, \left( {\gamma }^{2}\tau -\mu \, \left( 2\,\alpha \, \tau -\beta \right) \right) \right) \left( 2\,{\gamma }^{2}\tau -\mu \, \right. \\&\times \left. \left( 2\,\alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) \right) . \end{aligned}$$

From (37), when $a_i<a_j$, we can conclude that $\frac{\partial q_i^\mathrm{GG}}{\partial \gamma }<0$, $\frac{\partial w_i^\mathrm{GG}}{\partial \gamma }<0$, $\frac{\partial D_i^\mathrm{GG}}{\partial \gamma }<0$, $\frac{\partial \pi _{m_{i}}^\mathrm{GG}}{\partial \gamma }<0$ and $\frac{\partial \theta _i^\mathrm{GG}}{\partial \gamma }>0$, if $\frac{3\gamma ^2 \tau }{\mu }< 2\alpha \tau -\beta <4\tau -1$.

When $a_i>a_j$, we can conclude that $\frac{\partial q_i^\mathrm{GG}}{\partial \gamma }>0$, $\frac{\partial w_i^\mathrm{GG}}{\partial \gamma }>0$, $\frac{\partial D_i^\mathrm{GG}}{\partial \gamma }>0$. $\square$

Proof of Proposition 4

When manufacturer $M_2$ does not make a green investment, from

$$\begin{aligned}&\pi ^{\mathrm{GN}}_{m_{1}}-\pi ^{\mathrm{NN}}_{m_{1}} =-\,{\frac{ \left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) ^{2}+ {\gamma }^{2}\tau \, \left( 4\,\tau -1 \right) -\mu \, \left( 4\,\tau -1 \right) ^{2} \right) \left( a_{1}\, \left( 4\,\tau -1 \right) +a_{2} \, \left( 2\,\alpha \,\tau -\beta \right) \right) ^{2}{\gamma }^{2}{\tau }^{2}}{ 2\left( \left( 4\,\tau -1 \right) ^{2}- \left( 2\,\alpha \,\tau - \beta \right) ^{2} \right) ^{2} \left( {\gamma }^{2}\tau -\mu \, \left( 2 \,\alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) \right) ^{2}}}>0, \end{aligned}$$

we obtain that the manufacturer $M_1$ will invest if $\gamma \leqslant \sqrt{{\frac{\mu \, \left( \left( 4\,\tau -1 \right) ^{2}-\left( 2\,\alpha \,\tau -\beta \right) ^{2} \right) }{\tau \, \left( 4\,\tau -1 \right) }}}$. Otherwise, manufacturer $M_1$ will not invest. By the same reason, when manufacturer $M_1$ does not make a green investment, manufacturer $M_2$ will invest if $\gamma \leqslant \sqrt{{\frac{\mu \, \left( \left( 4\,\tau -1 \right) ^{2}-\left( 2\,\alpha \,\tau -\beta \right) ^{2} \right) }{\tau \, \left( 4\,\tau -1 \right) }}}$. Otherwise, manufacturer $M_2$ will not invest.

When manufacturer $M_2$ makes a green investment, from

$$\begin{aligned}&\pi ^{\mathrm{GG}}_{m_{1}}-\pi ^{\mathrm{NG}}_{m_{1}}\\&\quad ={\frac{{\tau }^{2}{\gamma }^{2} \left( {\mu }^{2} \left( \left( 4\,\tau -1 \right) ^{2}- \left( 2\,\alpha \,\tau -\beta \right) ^{2} \right) +\tau \,{\gamma }^{2} \left( 2\,\mu \, \left( 2\,\alpha \,\tau - \beta \right) -\mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) \right) \left( a_{1}\, \left( \mu \, \left( 4\,\tau -1 \right) -{\gamma }^{2}\tau \right) +a_{2}\, \left( \mu \, \left( 2\, \alpha \,\tau -\beta \right) -{\gamma }^{2}\tau \right) \right) ^{2}}{ 2\left( \mu \, \left( 2\,\alpha \,\tau -\beta \right) +\mu \, \left( 4\, \tau -1 \right) -2\,{\gamma }^{2}\tau \right) ^{2} \left( \mu \, \left( 2 \,\alpha \,\tau -\beta \right) +\mu \, \left( 4\,\tau -1 \right) -{\gamma } ^{2}\tau \right) ^{2} \left( 2\,\alpha \,\tau -\beta -4\,\tau +1 \right) ^ {2}\mu }}\\&\quad >0, \end{aligned}$$

we conclude that the manufacturer $M_1$ will invest if

$$\begin{aligned} \gamma \leqslant \sqrt{{\frac{2\,\mu \, \left( 2\, \alpha \,\tau -\beta \right) -\mu \, \left( 4\,\tau -1 \right) +\mu \, \sqrt{ \left( 4\,\tau -1 \right) \left( -\,8\,\alpha \,\tau +4\,\beta +20 \,\tau -5 \right) }}{2\tau }}}. \end{aligned}$$

(38)

Otherwise, the manufacturer $M_1$ will not invest.

Since manufacturers $M_1$ and $M_2$ are symmetrical, manufacturer $M_2$ has the same green investment strategy as manufacturer $M_1$. $\square$