Skip to main content
SpringerLink
Log in

The construction of operational matrix of fractional integration for solving fractional differential and integro-differential equations

  • Original Article
  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

Abstract

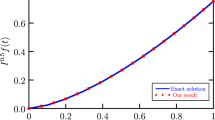
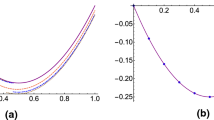
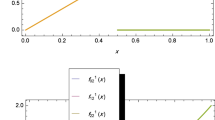
This study intends to present a general formulation for the hybrid Jacobi and block pulse operational matrix of fractional integral operator in order to solve fractional differential and integro-differential equations. First, we define hybrid Jacobi polynomials and block pulse functions as an orthogonal basis for function approximation. Then, we construct the operational matrix of fractional integration for these hybrid functions. With the combined features of these hybrid functions and their operational matrix of fractional integration, the governing equations that take the form of fractional differential and integro-differential equations are reduced to a system of algebraic equations. Illustrative examples are given to demonstrate the validity and reliability of the present technique.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Bagley RL, Torvik PJ (1986) On the fractional calculus model of viscoelastic behavior. J Rheol 30(1):133–155. doi:10.1122/1.549887

    Article  MATH  Google Scholar 

  2. Calderon AJ, Vinagre BM, Feliu V (2006) Fractional order control strategies for power electronic buck converters. Signal Process 86(10):2803–2819. doi:10.1016/j.sigpro.2006.02.022

    Article  MATH  Google Scholar 

  3. Grigorenko I, Grigorenko E (2003) Chaotic dynamics of the fractional Lorenz system. Phys Rev Lett. doi:10.1103/PhysRevLett.91.034101

    Google Scholar 

  4. Kumar S, Kumar D, Singh J (2016) Fractional modelling arising in unidirectional propagation of long waves in dispersive media. Adv Nonlinear Anal 5(4):383–394. doi:10.1515/anona-2013-0033

    MathSciNet  MATH  Google Scholar 

  5. Al Jarbouh A (2012) Rheological behaviour modelling of viscoelastic materials by using fractional model. Energy Procedia 19:143–157. doi:10.1016/j.egypro.2012.05.194

    Article  Google Scholar 

  6. Mainardi F (2012) Fractional calculus: some basic problems in continuum and statistical mechanics. arXiv preprint arXiv:1201.0863. In: Carpinteri A, Mainardi F (eds) (1997) Fractals and fractional calculus in continuum mechanics. Springer Verlag, Wien and New York, pp 291–348

  7. Tavazoei MS, Haeri M (2008) Chaos control via a simple fractional-order controller. Phys Lett A 372(6):798–807. doi:10.1016/j.physleta.2007.08.040

    Article  MATH  Google Scholar 

  8. Aghajani A, Jalilian Y, Trujillo J (2012) On the existence of solutions of fractional integro-differential equations. Fract Calc Appl Anal 15(1):44–69. doi:10.2478/s13540-012-0005-4

    Article  MathSciNet  MATH  Google Scholar 

  9. Deng J, Ma L (2010) Existence and uniqueness of solutions of initial value problems for nonlinear fractional differential equations. Appl Math Lett 23(6):676–680. doi:10.1016/j.aml.2010.02.007

    Article  MathSciNet  MATH  Google Scholar 

  10. Kumar S, Kumar A, Baleanu D (2016) Two analytical methods for time-fractional nonlinear coupled Boussinesq–Burgers equations arise in propagation of shallow water waves. Nonlinear Dyn 85(2):699–715. doi:10.1007/s11071-016-2716-2

    Article  MathSciNet  MATH  Google Scholar 

  11. Inc M (2008) The approximate and exact solutions of the space-and time-fractional Burgers equations with initial conditions by variational iteration method. J Math Anal Appl 345(1):476–484. doi:10.1016/j.jmaa.2008.04.007

    Article  MathSciNet  MATH  Google Scholar 

  12. Ray SS (2009) Analytical solution for the space fractional diffusion equation by two-step Adomian decomposition method. Commun Nonlinear Sci Numer Simul 14(4):1295–1306. doi:10.1016/j.cnsns.2008.01.010

    Article  MathSciNet  MATH  Google Scholar 

  13. Heydari MH, Hooshmandasl MR, Mohammadi F, Cattani C (2014) Wavelets method for solving systems of nonlinear singular fractional Volterra integro-differential equations. Commun Nonlinear Sci Numer Simul 19(1):37–48. doi:10.1016/j.cnsns.2013.04.026

    Article  MathSciNet  MATH  Google Scholar 

  14. Hosseini VR, Shivanian E, Chen W (2016) Local radial point interpolation (MLRPI) method for solving time fractional diffusion-wave equation with damping. J Comput Phys 312:307–332. doi:10.1016/j.jcp.2016.02.030

    Article  MathSciNet  MATH  Google Scholar 

  15. Hosseini VR, Shivanian E, Chen W (2015) Local integration of 2-D fractional telegraph equation via local radial point interpolant approximation. EPJ Plus 130(2):1–21. doi:10.1140/epjp/i2015-15033-5

    Google Scholar 

  16. Hosseini VR, Chen W, Avazzadeh Z (2014) Numerical solution of fractional telegraph equation by using radial basis functions. Eng Anal Bound Elem 38:31–39. doi:10.1016/j.enganabound.2013.10.009

    Article  MathSciNet  MATH  Google Scholar 

  17. Kazem S (2013) An integral operational matrix based on Jacobi polynomials for solving fractional-order differential equations. Appl Math Model 37(3):1126–1136. doi:10.1016/j.apm.2012.03.033

    Article  MathSciNet  MATH  Google Scholar 

  18. Lia C, Kumarb A, Kumarb S, Yangc XJ (2016) On the approximate solution of nonlinear time-fractional KdV equation via modified homotopy analysis Laplace transform method. J Nonlinear Sci Appl 9:5463–5470

    Article  MathSciNet  Google Scholar 

  19. Yin XB, Kumar S, Kumar D (2015) A modified homotopy analysis method for solution of fractional wave equations. Adv Mech Eng 7(12):1–8. doi:10.1177/1687814015620330

    Article  Google Scholar 

  20. Atabakzadeh MH, Akrami MH, Erjaee GH (2013) Chebyshev operational matrix method for solving multi-order fractional ordinary differential equations. Appl Math Model 37(20):8903–8911. doi:10.1016/j.apm.2013.04.019

    Article  MathSciNet  MATH  Google Scholar 

  21. Kilicman A, Al Zhour ZAA (2007) Kronecker operational matrices for fractional calculus and some applications. Appl Math Comput 187(1):250–265. doi:10.1016/j.amc.2006.08.122

    MathSciNet  MATH  Google Scholar 

  22. Keshavarz E, Ordokhani Y, Razzaghi M (2014) Bernoulli wavelet operational matrix of fractional order integration and its applications in solving the fractional order differential equations. Appl Math Model 38(24):6038–6051. doi:10.1016/j.apm.2014.04.064

    Article  MathSciNet  MATH  Google Scholar 

  23. Zhu L, Fan Q (2013) Numerical solution of nonlinear fractional-order Volterra integro-differential equations by SCW. Commun Nonlinear Sci Numer Simul 18(5):1203–1213. doi:10.1016/j.cnsns.2012.09.024

    Article  MathSciNet  MATH  Google Scholar 

  24. Saeedi H, Moghadam MM, Mollahasani N, Chuev GN (2011) A CAS wavelet method for solving nonlinear Fredholm integro-differential equations of fractional order. Commun Nonlinear Sci Numer Simul 16(3):1154–1163. doi:10.1016/j.cnsns.2010.05.036

    Article  MathSciNet  MATH  Google Scholar 

  25. Saadatmandi A, Dehghan M (2010) A new operational matrix for solving fractional-order differential equations. Comput Math Appl 59(3):1326–1336. doi:10.1016/j.camwa.2009.07.006

    Article  MathSciNet  MATH  Google Scholar 

  26. Yi M, Huang J (2014) Wavelet operational matrix method for solving fractional differential equations with variable coefficients. Appl Math Comput 230:383–394. doi:10.1016/j.amc.2013.06.102

    MathSciNet  MATH  Google Scholar 

  27. Podlubny I (1999) Fractional differential equations. Academic Press, San Diego

    MATH  Google Scholar 

  28. Canuto C, Hussaini MY, Quarteroni AM, Thomas JrA (2012) Spectral methods in fluid dynamics. Springer, Berlin

    MATH  Google Scholar 

  29. Jiang Z, Schoufelberger W, Thoma M, Wyner A (1992) Block pulse functions and their applications in control systems. Springer, New York

    Book  Google Scholar 

  30. Ervin VJ, Roop JP (2006) Variational formulation for the stationary fractional advection dispersion equation. Numer Methods Partial Differ Equ 22(3):558–576. doi:10.1002/num.20112

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Soft Matter Mechanics, Department of Engineering Mechanics, Hohai University, Nanjing, 210098, China

    Farzane Yousefi & Wen Chen

  2. Department of Applied Mathematics, Faculty of Mathematics and Computer, Shahid Bahonar University of Kerman, Kerman, Iran

    Farzane Yousefi & Azim Rivaz

  3. Young Researchers Society, Shahid Bahonar University of Kerman, Kerman, Iran

    Farzane Yousefi

Authors
  1. Farzane Yousefi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Azim Rivaz
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wen Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Azim Rivaz.

Ethics declarations

Conflict of interest

The authors declare that they have no conflicts of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yousefi, F., Rivaz, A. & Chen, W. The construction of operational matrix of fractional integration for solving fractional differential and integro-differential equations. Neural Comput & Applic 31, 1867–1878 (2019). https://doi.org/10.1007/s00521-017-3163-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-017-3163-9

Keywords

Search

Navigation