Error Analysis of the Strang Time-Splitting Laguerre–Hermite/Hermite Collocation Methods for the Gross–Pitaevskii Equation

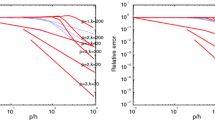
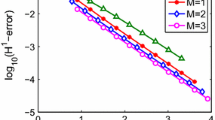
Abstract

The aim of this paper is to carry out a rigorous error analysis for the Strang splitting Laguerre–Hermite/Hermite collocation methods for the time-dependent Gross–Pitaevskii equation (GPE). We derive error estimates for full discretizations of the three-dimensional GPE with cylindrical symmetry by the Strang splitting Laguerre–Hermite collocation method, and for the d-dimensional GPE by the Strang splitting Hermite collocation method.

Appendix: The Proof of Lemma 4.4

Proof

The proof of Lemma 4.4 is analogous to the corresponding results for the Schrödinger–Poisson equation in [18]. For simplicity, we only verify (4.17). To this end, let $\widehat{H}=\widehat{T}+\widehat{V}$, and denote by D _H , D _T , and D _V the corresponding Lie derivatives (cf. [18]) of $\widehat{H}$, $\widehat{T}$, and $\widehat{V}$, respectively. According to Sect. 4.4 of [18],

$$ \psi^1-\psi(\tau)=\tau f\biggl(\frac{1}{2} \tau\biggr)- \int^\tau_0f(s)\, \mathrm{d}s+r_2-r_1, $$

(A.1)

where f(s)=exp((τ−s)D _T )D _V exp(sD _T )Id(ψ ₀), Id is the identity operator, and

Next we write the principal error term in the second-order Peano form:

$$\tau f\biggl(\frac{1}{2} \tau\biggr)-\int^\tau_0f(s) \,\mathrm{d}s=\tau^3\int^1_0 \nu (\theta)f^{\prime\prime}(\theta\tau)\,\mathrm{d}\theta $$

with the Peano kernel ν of the midpoint rule. We have (cf. Sect. 5.2 of [18])

where [D _T ,D _V ]=D _T D _V −D _V D _T . Hence by (4.14), the quadrature error is bounded in $H^{k}_{A}(\varOmega)$ by $c\tau^{3}\|\psi_{0}\|^{3}_{H^{k+4}_{A}(\varOmega)}$. Let us denote

$$g(s,\sigma)=\exp\bigl((\tau-s-\sigma)D_T\bigr)D_V \exp(\sigma D_T)D_V\exp(s D_T)\mathrm{Id}( \psi_0). $$

Then the remainder term can be expressed as

$$r_2-r_1=\frac{1}{2} \tau^2g\biggl(\frac{1}{2} \tau,0 \biggr)-\int^\tau_0\int ^{\tau -s}_0g(s,\sigma)\,\mathrm{d}\sigma \, \mathrm{d}s+\widetilde{r}_2-\widetilde{r}_1, $$

where

$$ \widetilde{r}_1=r_1-\int^\tau_0\int^{\tau -s}_0g(s,\sigma)\,\mathrm{d}\sigma \,\mathrm{d}s,\qquad\widetilde{r}_2=r_2-\frac{1}{2} \tau^2g\biggl(\frac{1}{2} \tau,0\biggr). $$

It is clear that (cf. [18])

By using a similar argument as in Sect. 5.2 of [18], we can obtain

$$\biggl\|\frac{1}{2} \tau^2g\biggl(\frac{1}{2} \tau,0\biggr)-\int^\tau_0\int^{\tau -s}_0g(s,\sigma)\,\mathrm{d}\sigma \,\mathrm{d}s \biggr\|_{H^{k}_A(\varOmega)}\leq c_1\tau^3, $$

where c ₁ depends only on $\|\psi_{0}\|_{H^{k+2}_{A}(\varOmega)}$.

Next, we estimate the term $\|\widetilde{r}_{2}\|_{H^{k}_{A}(\varOmega)}$. By the Taylor expansion,

$$\exp(\theta\tau D_V)=I+\int^{\theta\tau}_0 \exp(\xi D_V)D_V \,\mathrm{d}\xi=I+\theta\tau \int^1_0\exp(\theta\tau\varsigma D_V)D_V\,\mathrm{d}\varsigma, $$

whence

$$\widetilde{r}_2=\tau^3\int ^1_0\int^1_0 \theta(1-\theta)\exp \biggl(\frac{1}{2} \tau D_T\biggr)\exp(\theta\tau \varsigma D_V)D_V^3\exp\biggl(\frac{1}{2} \tau D_T\biggr)\mathrm{Id}(\psi_0)\,\mathrm{d}\varsigma \, \mathrm{d}\theta. $$

Setting $\phi=\mathrm{e}^{-\mathrm{i}\frac{\tau}{2}(\mathcal{A}_{r}+\mathcal{B}_{z})}\psi_{0}$ and $\eta=\mathrm{e}^{-\mathrm{i}\theta\tau\varsigma\beta|\phi|^{2}}\phi$, a direct calculation shows that

$$\exp\biggl(\frac{1}{2} \tau D_T\biggr)\exp(\theta\tau\varsigma D_V)D_V^3\exp\biggl(\frac{1}{2} \tau D_T\biggr)\mathrm{Id}(\psi_0)=\mathrm{i} \beta^3\mathrm{e}^{-\mathrm{i}\frac{\tau}{2}(\mathcal{A}_r+\mathcal {B}_z)}\bigl(|\eta|^6\eta \bigr). $$

Hence, by (4.2)–(4.4) and (4.10), we find that for j=max(k,2),

where c ₂ depends only on $\|\psi_{0}\|_{H^{j}_{A}(\varOmega)}$. Therefore, $\|\widetilde{r}_{2}\|_{H^{k}_{A}(\varOmega)}\leq c_{2}\tau^{3}$.

It remains to estimate the term $\|\widetilde{r}_{1}\|_{H^{k}_{A}(\varOmega)}$. By using the nonlinear variation-of-constants formula (cf. [18]), we obtain that

By (4.2)–(4.4), a direct calculation gives

Therefore, $\|\widetilde{r}_{1}\|_{H^{k}_{A}(\varOmega)}\leq c_{3}\tau^{3}$, where c ₃ depends only on $\max_{0\leq t\leq \tau}\|\psi\|_{H^{j}_{A}(\varOmega)}$. A combination of the previous statements leads to (4.17). □