Skip to main content
SpringerLink
Log in

The practical Gauss type rules for Hadamard finite-part integrals using Puiseux expansions

  • Published:
Advances in Computational Mathematics Aims and scope Submit manuscript

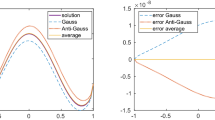
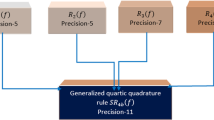
Abstract

A general framework is constructed for efficiently and stably evaluating the Hadamard finite-part integrals by composite quadrature rules. Firstly, the integrands are assumed to have the Puiseux expansions at the endpoints with arbitrary algebraic and logarithmic singularities. Secondly, the Euler-Maclaurin expansion of a general composite quadrature rule is obtained directly by using the asymptotic expansions of the partial sums of the Hurwitz zeta function and the generalized Stieltjes constant, which shows that the standard numerical integration formula is not convergent for computing the Hadamard finite-part integrals. Thirdly, the standard quadrature formula is recast in two steps. In step one, the singular part of the integrand is integrated analytically and in step two, the regular integral of the remaining part is evaluated using the standard composite quadrature rule. In this stage, a threshold is introduced such that the function evaluations in the vicinity of the singularity are intentionally excluded, where the threshold is determined by analyzing the roundoff errors caused by the singular nature of the integrand. Fourthly, two practical algorithms are designed for evaluating the Hadamard finite-part integrals by applying the Gauss-Legendre and Gauss-Kronrod rules to the proposed framework. Practical error indicator and implementation involved in the Gauss-Legendre rule are addressed. Finally, some typical examples are provided to show that the algorithms can be used to effectively evaluate the Hadamard finite-part integrals over finite or infinite intervals.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Aroca, F., Ilardi, G., Lopez de Medrano, L.: Puiseux power series solutions for systems of equations. Int. J. Math. 21, 1439–1459 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  2. Boykov, I.V.: Numerical methods of computation of singular and hypersingular integrals. Int. J. Math. Math. Sci. 28, 127–179 (2001)

    Article  MathSciNet  Google Scholar 

  3. Boykov, I.V., Ventsel, E.S., Boykova, A.I.: Accuracy optimal methods for evaluating hypersingular integrals. Appl. Numer. Math. 59, 1366–1385 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  4. Broughan, K.A.: Vanishing of the integral of the Hurwitz zeta function. Bull. Austral. Math. Soc. 65, 121–127 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  5. Calvetti, D., Golub, G.H., Gragg, W.B., Reichel, L.: Computation of Gauss-Kronrod quadrature rules. Math. Comp. 69, 1035–1052 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  6. Carley, M.: Numerical quadratures for singular and hypersingular integrals in boundary element methods. SIAM J. Sci. Comput. 29, 1207–1216 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  7. Choudhury, B.K.: The Riemann zeta-function and its derivatives. Proc. R. Soc. Lond. A Math. Phys. 450, 477–499 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  8. Coffey, M.W.: Series representations for the Stieltjes constants. Rocky Mt. J. Math. 44, 443–477 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  9. Conceicão, A.C., Kravchenko, V.G., Pereira, J.C.: Computing some classes of Cauchy type singular integrals with Mathematica software. Adv. Comput. Math. 39, 273–288 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  10. Criscuolo, G.: A new algorithm for Cauchy principal value and Hadamard finite-part integrals. J. Comput. Appl. Math. 78, 255–275 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  11. Criscuolo, G.: Numerical evaluation of certain strongly singular integrals. IMA J. Numer. Anal. 34, 651–674 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  12. Davis, P.J., Rabinowitz, P.: Methods of numerical integration, 2nd edn. Academic Press, San Dieggo (1984)

    MATH  Google Scholar 

  13. Diethelm, K.: Modified compound quadrature rules for strongly singular integrals. Computing 52, 337–354 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  14. Ehrich, S.: High order error constants of Gauss-Kronrod quadrature formulas. Analysis 16, 335–345 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  15. Ehrich, S.: Stieltjes polynomials and the error of Gauss-Kronrod quadrature formulas. In: Gautschi, W., Golub, G.H., Opfer, G (eds.) Applications and Computation of Orthogonal Polynomials, p 131. Proceedings Conference Oberwolfach, International Series Numerical Mathematics, Birkhäuser, Basel (1999)

  16. Elliott, D.: Three algorithms for Hadamard finite-part integrals and fractional derivatives. J. Comput. Appl. Math. 62, 267–283 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  17. Greynat, D., Sesma, J., Vulvert, G.: Derivatives of the Pochhammer and reciprocal Pochhammer symbols and their use in epsilon-expansions of Appell and Kampé de Fériet functions. J. Math. Phys. 55(043501), 1–16 (2014)

    MATH  Google Scholar 

  18. Hadamard, J.: Lectures on Cauchy’s Problem in Linear Partial Differential Equations. Yale University Press, New Haven (1923)

  19. Handelsman, R.A., Olmstead, W.E.: Asymptotic solution to a class of nonlinear Volterra integral equations. SIAM J. Appl. Math. 22, 373–384 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  20. Hasegawa, T., Sugiura, H.: Algorithms for approximating finite Hilbert transform with end-point singularities and its derivatives. J. Comput. Appl. Math. 236, 243–252 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  21. Huang, J., Wang, Z., Zhu, R.: Asymptotic error expansions for hypersingular integrals. Adv. Comput. Math. 38, 257–279 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  22. Ioakimidis, N.I.: On the uniform convergence of Gaussian quadrature rules for Cauchy principal value integrals and their derivatives. Math. Comp. 44, 191–198 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  23. Johansson, F.: Rigorous high-precision computation of the Hurwitz zeta function and its derivatives. Numer. Algorithm. 69, 253–270 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  24. Kanemitsu, S., Kumagai, S., Srivastava, H.M., Yoshimoto, M.: Some integral and asymptotic formulas associated with the Hurwitz zeta function. Appl. Math. Comput. 154, 641–664 (2004)

    MathSciNet  MATH  Google Scholar 

  25. Kolwankar, K.M., Gangal, A.D.: Fractional differentiability of nowhere differentiable functions and dimensions. Chaos 6, 505–513 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  26. Kolwankar, K.M.: Recursive local fractional derivative. arXiv (2013). 1312.7675v1

  27. Laurie, D.P.: Calculation of Gauss-Kronrod quadrature rules. Math. Comp. 66, 1133–1145 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  28. Linz, P.: On the approximate computation of certain strongly singular integrals. Computing 35, 345–353 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  29. Lubinsky, D.S., Rabinowitz, P.: Rates of convergence of Gaussian quadrature for singular integrands. Math. Comp. 43, 219–242 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  30. Lyness, J.N., Ninham, B.W.: Numerical quadrature and asymptotic expansions. Math. Comp. 21, 162–178 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  31. Lyness, J.N.: Finite-part integrals and the Euler-Maclaurin expansion. In: Zahar, R.V.M. (ed.) Approximation and Computation, pp 397–407, Birkhäuser Verlag (1994)

  32. Monegato, G.: Numerical evaluation of hypersingular integrals. J. Comput. Appl. Math. 50, 9–31 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  33. Monegato, G., Lyness, J.N.: The Euler-Maclaurin expansion and finite-part integrals. Numer. Math. 81, 273–291 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  34. Monegato, G.: An overview of the computational aspects of Kronrod quadrature rules. Numer. Algorithm. 26, 173–196 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  35. Monegato, G.: Definitions, properties and applications of finite-part integrals. J. Comput. Appl. Math. 229, 425–439 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  36. Navot, I.: An extension of the Euler-Maclaurin summation formula to functions with a branch singularity. J. Math. Phys. 40, 271–276 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  37. Navot, I.: A further extension of the Euler-Maclaurin summation formula. J. Math. Phys. 41, 155–163 (1962)

    Article  MATH  Google Scholar 

  38. Ninham, B.W.: Generalised functions and divergent integrals. Numer. Math. 8, 444–457 (1966)

    Article  MathSciNet  MATH  Google Scholar 

  39. Olver, F.W.J., Lozier, D.W., Boisvert, R.F., Clark, C.W.: NIST handbook of mathematical functions. Cambridge University Press, Cambridge (2010). http://dlmf.nist.gov

    MATH  Google Scholar 

  40. Paget, D.F.: The numerical evaluation of Hadamard finite-part integrals. Numer. Math. 36, 447–453 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  41. Petras, K.: On the computation of the Gauss-Legendre quadrature formula with a given precision. J. Comput. Appl. Math. 112, 253–267 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  42. Poteaux, A., Rybowicz, M.: Good reduction of Puiseux series and applications. J. Symb. Comput. 47, 32–63 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  43. Sellier, A.: Asymptotic expansions of a class of integrals. Proc. R. Soc. Lond. A Math. Phys. 445, 693–710 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  44. Sidi, A.: Practical Extrapolation Methods–Theory and Applications. Cambridge University Press, Cambridge (2003)

  45. Sidi, A.: Euler-Maclaurin expansions for integrals with endpoint singularities: a new perspective. Numer. Math. 98, 371–387 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  46. Sidi, A.: Asymptotic expansions of Gauss-Legendre quadrature rules for integrals with endpoint singularities. Math. Comp. 78, 241–253 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  47. Sidi, A.: Euler-Maclaurin expansions for integrals with arbitrary algebraic endpoint singularities. Math. Comp. 81, 2159–2173 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  48. Sidi, A.: Euler-Maclaurin expansions for integrals with arbitrary algebraic-logarithmic endpoint singularities. Constr. Approx. 36, 331–352 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  49. Sidi, A.: Compact numerical quadrature formulas for hypersingular integrals and integral equations. J. Sci. Comput. 54, 145–176 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  50. Sidi, A.: Richardson extrapolation on some recent numerical quadrature formulas for singular and hypersingular integrals and its study of stability. J. Sci. Comput. 60, 141–159 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  51. Steffensen, J.F.: Interpolation, 2nd edn. Dover, New York (2006)

    MATH  Google Scholar 

  52. Sun, W.W., Wu, J.M.: Interpolatory quadrature rules for Hadamard finite-part integrals and their superconvergence. IMA J. Numer. Anal. 28, 580–597 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  53. Swarztrauber, P.N.: On computing the points and weights for Gauss-Legendre quadrature. SIAM J. Sci. Comput. 24, 945–954 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  54. Verlinden, P.: Acceleration of Gauss-Legendre quadrature for an integrand with an endpoint singularity. J. Comput. Appl. Math. 77, 277–287 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  55. Wang, H.Y., Zhang, L., Huybrechs, D.: Asymptotic expansions and fast computation of oscillatory Hilbert transforms. Numer. Math. 123, 709–743 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  56. Wang, J.Z., Li, J., Zhou, Y.T.: The trapezoidal rule for computing supersingular integral on interval. Appl. Math. Comput. 219, 1616–1624 (2012)

    MathSciNet  MATH  Google Scholar 

  57. Wang, T.K., Li, N., Gao, G.H.: The asymptotic expansion and extrapolation of trapezoidal rule for integrals with fractional order singularities. Int. J. Comput. Math. 92, 579–590 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  58. Wang, T.K., Liu, Z.F., Zhang, Z.Y.: The modified composite Gauss type rules for singular integrals using Puiseux expansions. Math. Comp. (2016) http://dx.doi.org/10.1090/mcom/3105

  59. Wu, J.M., Sun, W.W.: The superconvergence of the composite trapezoidal rule for Hadamard finite part integrals. Numer. Math. 102, 343–363 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  60. Wu, J.M., Sun, W.W.: The superconvergence of Newton-Cotes rules for the Hadamard finite-part integral on an interval. Numer. Math. 109, 143–165 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  61. Xiang, S.H., Bornemann, F.: On the convergence rates of Gauss and Clenshaw-Curtis quadrature for functions of limited regularity. SIAM J. Numer. Anal 50, 2581–2587 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  62. Zhang, N.Y., Williams, K.S.: Some results on the generalized Stieltjes constants. Analysis 14, 147–162 (1994)

    MathSciNet  MATH  Google Scholar 

  63. Zhang, X.P., Wu, J.M., Yu, D.H.: Superconvergence of the composite Simpson’s rule for a certain finite-part integral and its applications. J. Comput. Appl. Math. 223, 598–613 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  64. Zozulya, V.V.: Regularization of divergent integrals: A comparison of the classical and generalized-functions approaches. Adv. Comput. Math. 41, 727–780 (2015)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Tianjin Normal University, Tianjin, 300387, China

    Tongke Wang

  2. Jiangsu Provincial Key Laboratory for NSLSCS, School of Mathematical Sciences, Nanjing Normal University, Nanjing, 210023, China

    Zhiyue Zhang

  3. School of Mathematical Sciences, Tianjin Normal University, Tianjin, 300387, China

    Zhifang Liu

Authors
  1. Tongke Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhiyue Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Zhifang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tongke Wang.

Additional information

Communicated by: Martin Stynes

This project is partially supported by the National Natural Science Foundation of China (grant No.11471166), Natural Science Foundation of Jiangsu Province (grant No.BK20141443) and the Priority Academic Program Development of Jiangsu Higher Education Institutions (PAPD).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, T., Zhang, Z. & Liu, Z. The practical Gauss type rules for Hadamard finite-part integrals using Puiseux expansions. Adv Comput Math 43, 319–350 (2017). https://doi.org/10.1007/s10444-016-9487-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10444-016-9487-7

Keywords

Mathematics Subject Classification (2010)

Search

Navigation