Abstract
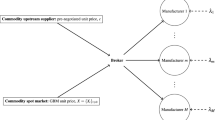
We consider a firm buying a commodity from a spot market as raw material and selling a final product by submitting bids. Bidding opportunities (i.e., demand arrivals) are random, and the likelihood of winning bids (i.e., selling the product) depends on the bid price. The price of the commodity raw material is also stochastic. The objective of the firm is to jointly decide on the procurement and bidding strategies to maximize its expected total discounted profit in the face of this demand and supply randomness. We model the commodity prices in the spot market as a Markov chain and the bidding opportunities as a Poisson process. Subsequently, we formulate the decision-making problem of the firm as an infinite-horizon stochastic dynamic program and analytically characterize its structural properties. We prove that the optimal procurement strategy follows a price-dependent base-stock policy and the optimal bidding price is decreasing with respect to the inventory level. We also formulate and analyze three intuitively appealing heuristic strategies, which either do not allow for carrying inventory or adopt simpler bidding policies (e.g., a constant bid price or myopically set bid prices). Using historical daily prices of several commodities, we then calibrate our models and conduct an extensive numerical study to compare the performances of the different strategies. Our study reveals the importance of adopting the optimal integrative procurement and bidding strategy, which is particularly rewarding when the raw material prices are more volatile and/or when there is significant competition on the demand side (the probability of winning is much smaller when submitting the same bid price). We establish that the relative performances of the three heuristic strategies depend critically on the holding cost of raw material inventory and the competitive environment, and identify conditions under which the shortfalls in profits from adopting such strategies are relatively less significant.


Similar content being viewed by others
Notes
Data about oil and copper prices are from http://www.datastream.com.
Since the majority of the projects are rather similar in nature, indeed the company can buy for future projects.
When the firm does not hold any inventory, the planning periods are decoupled, and hence a myopic bidding strategy is indeed optimal.
The optimal policy for ZI has already been discussed in the previous section.
The values of these 10 price points and the corresponding \(\gamma _{ij}\) are the same as those used in Sect. 5.
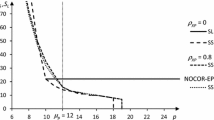
We terminate the algorithm once the average reward reaches four-digit accuracy.
ZI, by definition, holds no inventory.
Note that the different strategies are derived from the estimated parameters for the price processes.
References
Akella, R., Araman, V. F., & Kleinknecht, J. (2002). B2B markets: Procurement and supplier risk management in e-business. In J. Geunes, P. M. Pardalos, & H. E. Romeijn (Eds.), Supply chain management: Models, applications, and research directions (pp. 33–66). Dordrecht: Kluwer Academic Publishers.
Berling, P., & Martínez de Albéniz, V. (2011). Optimal inventory policies when purchase price and demand are stochastic. Operations Research, 59(1), 109–124.
Billingsley, P. (1968). Statistical inference for Markov processes. Chicago: University of Chicago Press.
Bladt, M., & Sørensen, M. (2009). Efficient estimation of transition rates between credit ratings from observations at discrete time points. Quantitative Finance, 9(2), 147–160.
Cohen, M. A., & Agrawal, N. (1999). An analytical comparison of long and short term contracts. IIE Transactions, 31(8), 783–796.
Economist Intelligence Unit. (2009). Managing supply-chain risk for reward. Technical report, Economist Intelligence Unit.
Fabian, T., Fisher, J. L., Sasieni, M. W., & Yardeni, A. (1959). Purchasing raw material on a fluctuating market. Operations Research, 7(1), 107–122.
Federgruen, A., & Heching, A. (1999). Combined pricing and inventory control under uncertainty. Operations Research, 47(3), 454–475.
Fu, Q., Lee, C. Y., & Teo, C. P. (2010). Procurement risk management using options: Random spot price and the portfolio effect. IIE Transactions, 42(11), 793–811.
Gavirneni, S. (2004). Periodic review inventory control with fluctuating purchasing costs. Operations Research Letters, 32(4), 374–379.
Gayon, J. P., Benjaafar, S., & de Vericourt, F. (2009a). Using imperfect advance demand information in production–inventory systems with multiple customer classes. Manufacturing and Service Operations Management, 11(1), 128–143.
Gayon, J. P., Talay-Değirmenci, I., Karaesmen, F., & Örmeci, E. (2009b). Optimal pricing and production policies of a make-to-stock system with fluctuating demand. Probability in the Engineering and Informational Sciences, 23(2), 205–230.
Goel, A., & Gutierrez, G. (2007). Integrating commodity markets in the optimal procurement policies of a stochastic inventory system. Technical report, University of Texas-Austin.
Golabi, K. (1985). Optimal inventory policies when ordering prices are random. Operations Research, 33(3), 575–588.
Gümüş, M., Ray, S., & Gurnani, H. (2012). Supply-side story: Risks, guarantees, competition, and information asymmetry. Management Science, 58(9), 1694–1714.
Guo, X., Kaminsky, P., Tomecek, P., & Yuen, M. (2011). Optimal spot market inventory strategies in the presence of cost and price risk. Mathematical Methods of Operations Research, 73(1), 109–137.
Guttorp, P. (1995). Stochastic modeling of scientific data. London: Chapman & Hall.
Ha, A. Y. (1997). Inventory rationing in a make-to-stock production system with several demand classes and lost sales. Management Science, 43(8), 1093–1103.
Haksöz, Ç., & Seshadri, S. (2007). Supply chain operations in the presence of a spot market: A review with discussion. Journal of the Operational Research Society, 58(11), 1412–1429.
Kalymon, B. A. (1971). Stochastic prices in a single-item inventory purchasing model. Operations Research, 19(6), 1434–1458.
Kingsman, B. G. (1986). Purchasing raw materials with uncertain fluctuating prices. European Journal of Operational Research, 25(3), 358–372.
Lippman, S. A. (1975). Applying a new device in the optimization of exponential queuing systems. Operations Research, 23(4), 687–710.
Martínez de Albéniz, V., & Simchi-Levi, D. (2005). A portfolio approach to procurement contracts. Production and Operations Management, 14(1), 90–114.
O’Marah, K. (2009). Supply chain risk: Kanban won’t cut it. Technical report, AMR Research.
Özekici, S., & Parlar, M. (1999). Inventory models with unreliable suppliers in a random environment. Annals of Operations Research, 91(1), 123–136.
Porteus, E. (1982). Conditions for characterizing the structure of optimal strategies in infinite-horizon dynamic programs. Journal of Optimization Theory and Applications, 36(3), 419–432.
Porteus, E. L. (2002). Foundations of stochastic inventory theory. Stanford: Stanford University Press.
Puterman, M. L. (1994). Markov decision processes: Discrete stochastic dynamic programming. New York: Wiley.
Ross, S. M. (1999). An introduction to mathematical finance: Options and other topics. New York: Cambridge University Press.
Schwartz, E. S. (1997). The stochastic behavior of commodity prices: Implications for valuation and hedging. Journal of Finance, 52(3), 923–973.
Seifert, R. W., Thonemann, U. W., & Hausman, W. H. (2004). Optimal procurement strategies for online spot markets. European Journal of Operational Research, 152(3), 781–799.
Tang, C. S. (2006). Perspectives in supply chain risk management. International Journal of Production Economics, 103(2), 451–488.
Vakharia, A. J., & Yenipazarlı, A. (2009). Managing supply chain disruptions. Foundations and Trends in Technology, Information and Operations Management, 2(4), 243–325.
Wang, Y. (2001). The optimality of myopic stocking policies for systems with decreasing purchasing prices. European Journal of Operational Research, 133(1), 153–159.
Yang, J., & Xia, Y. (2009). Acquisition management under fluctuating raw material prices. Production and Operations Management, 18(2), 212–225.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: Proofs of Proposition 1 and Theorems 1 and 2
Proof of Proposition 1
In this proposition, we verify that the functional operators associated with MB, SB, and DB preserve Conditions 1 and 2 stated in Definition 1. Since the verification steps for MB and SB are very similar to those for DB, here, we only provide the proof for DB.
To simplify the proof, we rewrite \(T_{\text{ DB }}\) as a linear combination of functional operators \(G\) and \(H\) as follows:
where
and
Now, we show that the functional operator \(T_{\text{ DB }}\) preserves Conditions 1 and 2. We divide the proof into two steps, one for each condition. In each step, we consider \(G\) and \(H\) separately and show that Conditions 1 and 2 are preserved by each one of them.
Step 1. Verification of Condition 1.
Step 1.1. Preservation of Condition 1 by \({\mathbf {G}}\). We first focus on the concavity of \(Gv(x,i)\) with respect to \(x\).
If \(x=0,\) we want to prove \(Gv(2,i) - Gv(1,i) \le Gv(1,i) - Gv(0,i),\) that is, \(\max _{b \ge 0} \bigl \{ (1-b) (\max \{v(2,i)-p_i,v(1,i) \} +b)+ bv(2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(1,i)-p_i,v(0,i) \} +b)+ bv(1,i) \bigl \} \,\,\le \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(1,i)-p_i,v(0,i) \} +b)+ bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(0,i)-p_i +b )+ bv(0,i) \bigl \}\). We consider the following four cases:
-
Case A1.
\(v(2,i)-p_i \ge v(1,i)\) and \(v(1,i)-p_i \ge v(0,i)\);
-
Case A2.
\(v(2,i)-p_i \ge v(1,i)\) and \(v(1,i)-p_i < v(0,i)\);
-
Case A3.
\(v(2,i)-p_i < v(1,i)\) and \(v(1,i)-p_i \ge v(0,i)\);
-
Case A4.
\(v(2,i)-p_i < v(1,i)\) and \(v(1,i)-p_i < v(0,i)\).
Case A1. \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b) (v(2,i) +b -p_i )+ bv(2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)( v(1,i) + b - p_i)+ bv(1,i) \bigl \}\,\le \max _{b \ge 0} \bigl \{ (1-b)( v(1,i) + b -p_i)+ bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(v(0,i) + b -p_i)+ bv(0,i) \bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)(b-p_i)\bigl \} + v(2,i) -\max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(1,i) \le \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} + v(1,i) -\max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(0,i).\) \(\Leftrightarrow v(2,i) - v(1,i) \le v(1,i) - v(0,i).\)
Case A2. This case is impossible since it contradicts with \(v(2,i)-v(1,i) \le v(1,i)-v(0,i)\).
Case A3. \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)(v(1,i) +b)+ bv(2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(1,i) + b - p_i)+ bv(1,i) \bigl \} \,\le \max _{b \ge 0} \bigl \{ (1-b) ( v(1,i) + b -p_i)+ bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(0,i) + b - p_i) + b v(0,i)\bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)b -b(v(1,i) - v(2,i)) \bigl \} \,\le \max _{b \ge 0} \bigl \{ (1-b)(b -p_i) \bigl \} + v(1,i) - v(0,i).\) As for \(\max _{b \ge 0} \bigl \{ (1-b)b -b(v(1,i) - v(2,i)) \bigl \}\), \(b^{*}= \frac{1}{2}(1-(v(1,i)-v(2,i)))\) and \(\max _{b \ge 0} \bigl \{ (1-b)b -b(v(1,i) - v(2,i)) \bigl \}= \frac{1}{4}(1-(v(1,i)-v(2,i)))^2\). As for \(\max _{b \ge 0} \bigl \{ (1-b)(b -p_i) \bigl \}\), \(b^{*}= \frac{1}{2}(1+p_i)\) and \(\max _{b \ge 0} \bigl \{ (1-b)(b -p_i) \bigl \} = \frac{1}{4}(1-p_i)^2\). Hence, \(\Leftrightarrow \frac{1}{4}(1-(v(1,i)-v(2,i)))^2 \le \frac{1}{4}(1-p_i)^2 + v(1,i) - v(0,i)\). Since \(v(2,i) - v(1,i) < p_i\) and \(1 + v(2,i) - v(1,i) \ge 1 - 1 = 0\), \(\frac{1}{4}(1-(v(1,i)-v(2,i)))^2 = \frac{1}{4}(1+v(2,i)-v(1,i))^2 \le \frac{1}{4}(1+p_i)^2\). Moreover, \(\frac{1}{4}(1-p_i)^2 + v(1,i) - v(0,i) \ge \frac{1}{4}(1-p_i)^2 + p_i = \frac{1}{4}(1 + p_i)^2.\)
Case A4. \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b) (v(1,i) +b)+ bv(2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(0,i) + b ) + bv(1,i) \bigl \}\, \le \max _{b \ge 0} \bigl \{ (1-b) ( v(0,i) + b)+ bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(0,i) + b - p_i) + b v(0,i)\bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)b - b (v(1,i) - v(2,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)b - b( v(0,i) - v(1,i)) \bigl \} - (v(0,i)-v(1,i)) \,\le \max _{b \ge 0} \bigl \{ (1-b)b - b( v(0,i) - v(1,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b -p_i) \bigl \}.\) \(\Leftrightarrow \frac{1}{4}(1-(v(1,i)-v(2,i)))^2 - \frac{1}{4}(1-(v(0,i)-v(1,i)))^2 - (v(0,i)-v(1,i)) \le \frac{1}{4}(1-(v(0,i)-v(1,i)))^2 - \frac{1}{4}(1 - p_i)^2.\) \(\Leftrightarrow (1-(v(1,i)-v(2,i)))^2 - (1 + v(0,i)-v(1,i))^2 \le (1+v(1,i)-v(0,i))^2 - (1 - p_i)^2.\) Since \(v(2,i)-v(1,i) \le v(1,i)-v(0,i)\), \(1+v(2,i)-v(1,i) \ge 1-1=0,\) and \(1+v(1,i)-v(0,i) \ge 1- 1 = 0,\) we have \((1-(v(1,i)-v(2,i)))^2 \le (1+v(1,i)-v(0,i))^2.\) Moreover, since \(v(0,i)-v(1,i) > -p_i,\) we have \(1+v(0,i)-v(1,i)>1 - p_i \ge 0.\) Then, we have \((1 + v(0,i)-v(1,i))^2 > (1 - p_i)^2.\)
If \(x \ge 1,\) we want to prove \(Gv(x+2,i) - Gv(x+1,i) \le Gv(x+1,i) - Gv(x,i),\) that is, \(\max _{b \ge 0} \bigl \{ (1-b) (\max \{v(x+2,i)-p_i,v(x+1,i) \} +b )+ bv(x+2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(x+1,i)-p_i,v(x,i) \} +b)+ bv(x+1,i) \bigl \} \,\le \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(x+1,i)-p_i,v(x,i) \} +b)+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(x,i)-p_i,v(x-1,i) \} +b) + bv(x,i) \bigl \}.\) Since \(v(x,i)\) is concave with respect to \(x\), there exists an integer \(x^*\) such that if \(x \le x^*\), \(v(x,i)-p_i \ge v(x-1,i)\) and if \( x > x^*,\) \(v(x,i) - p_i < v(x-1,i)\). We consider the following four cases:
-
Case B1.
\(x \le x^* -2\);
-
Case B2.
\(x = x^* -1\);
-
Case B3.
\(x = x^*\);
-
Case B4.
\(x \ge x^* + 1\).
Case B1. \(\max _{b \ge 0} \bigl \{ (1-b) (v(x+2,i) +b - p_i)+ bv(x+2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(x+1,i) + b - p_i )+ bv(x+1,i) \bigl \} \,\le \max _{b \ge 0} \bigl \{ (1-b)( v(x+1,i) + b -p_i )+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(x,i) + b - p_i ) + b v(x,i) \bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} + v(x+2,i) - \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(x+1,i) \le \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} + v(x+1,i) - \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(x,i).\) \(\Leftrightarrow v(x+2,i) - v(x+1,i) \le v(x+1,i) - v(x,i).\)
Case B2. \(\max _{b \ge 0} \bigl \{ (1-b)(v(x+1,i) +b)+ bv(x+2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)( v(x+1,i) + b - p_i)+ bv(x+1,i) \bigl \} \,\,\le \max _{b \ge 0} \bigl \{ (1-b) ( v(x+1,i) + b -p_i )+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(v(x,i) + b - p_i ) + b v(x,i)\bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)b -b(v(x+1,i) - v(x+2,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b - p_i) \bigl \}\,\, \le v(x+1,i) - v(x,i).\) \(\Leftrightarrow \frac{1}{4}(1-(v(x+1,i)-v(x+2,i)))^2 - \frac{1}{4}(1-p_i)^2 \le v(x+1,i) - v(x,i).\) Since \(x+2 = x^*-1+2=x^*+1 > x^*,\) we have \(v(x+2,i)-v(x+1,i) <p_i.\) So, \(1-(v(x+1,i)-v(x+2,i)) = 1 + v(x+2,i)-v(x+1,i) < 1+p_i.\) Combining with the fact that \(1-(v(x+1,i)-v(x+2,i)) = 1 + v(x+2,i)-v(x+1,i) \ge 1 - 1 = 0,\) we have \(\frac{1}{4}(1-(v(x+1,i)-v(x+2,i)))^2 - \frac{1}{4}(1-p_i)^2 \le \frac{1}{4}(1+p_i)^2 - \frac{1}{4}(1-p_i)^2 = p_i.\) Moreover, since \(x=x^*-1,\) that is, \(x+1=x^*,\) we have \(v(x+1,i)-p_i \ge v(x,i).\) Hence, \(v(x+1,i)-v(x,i) \ge p_i.\)
Case B3. \(\max _{b \ge 0} \bigl \{ (1-b) (v(x+1,i) +b)+ bv(x+2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b )+ bv(x+1,i) \bigl \} \,\le \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b )+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(x,i) + b - p_i ) + b v(x,i)\bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x+1,i) - v(x+2,i) ) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x,i) - v(x+1,i)) \bigl \} - (v(x,i) - v(x+1,i)) \le \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x,i) - v(x+1,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b - p_i) \bigl \}.\) \(\Leftrightarrow \frac{1}{4}(1-(v(x+1,i)-v(x+2,i)))^2 - \frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - (v(x,i) - v(x+1,i)) \le \frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1-p_i)^2.\) \(\Leftrightarrow (1+v(x+2,i)-v(x+1,i))^2 - (1+v(x,i)-v(x+1,i))^2 \le (1+v(x+1,i)-v(x,i))^2 - (1-p_i)^2.\) Since \(v(x+2,i)-v(x+1,i) \le v(x+1,i) - v(x,i)\), \(1+v(x+2,i)-v(x+1,i) \ge 1 - 1 = 0,\) and \(1+v(x+1,i)-v(x,i) \ge 1 - 1 = 0,\) we have \((1+v(x+2,i)-v(x+1,i))^2 \le (1+v(x+1,i)-v(x,i))^2.\) Moreover, since \(v(x+1,i) - v(x,i) < p_i,\) we have \(v(x,i) - v(x+1,i) > -p_i.\) Combining with the fact that \(1 + v(x,i) - v(x+1,i) \ge 1 - p_i \ge 0\), we have \((1+v(x,i)-v(x+1,i))^2 \ge (1-p_i)^2.\)
Case B4. \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b) (v(x+1,i) +b)+ bv(x+2,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)( v(x,i) + b)+ bv(x+1,i) \bigl \} \, \le \max _{b \ge 0} \bigl \{ (1-b)( v(x,i) + b)+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) (v(x-1,i) + b) + b v(x,i)\bigl \}.\) \(\Leftrightarrow \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x+1,i) - v(x+2,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x,i) - v(x+1,i)) \bigl \} -(v(x,i) - v(x+1,i)) \le \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x,i) - v(x+1,i)) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)b - b(v(x-1,i) - v(x,i)) \bigl \}-(v(x-1,i) - v(x,i)).\) \(\Leftrightarrow \frac{1}{4}(1-(v(x+1,i)-v(x+2,i)))^2 - \frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - (v(x,i) - v(x+1,i)) \le \frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1-(v(x-1,i)-v(x,i)))^2 - (v(x-1,i) - v(x,i)).\) \(\Leftrightarrow (1+v(x+2,i)-v(x+1,i))^2 - (1+v(x,i)-v(x+1,i))^2 \le (1+v(x+1,i)-v(x,i))^2 - (1+v(x-1,i)-v(x,i))^2.\) Since \(v(x+2,i) -v(x+1,i) \le v(x+1,i) - v(x,i),\) \(1+v(x+2,i)-v(x+1,i) \ge 1-1 = 0,\) and \(1+v(x+1,i)-v(x,i) \ge 1-1= 0,\) we have \((1+v(x+2,i)-v(x+1,i))^2 \le (1+v(x+1,i)-v(x,i))^2.\) Moreover, since \(v(x+1,i)-v(x,i) \le v(x,i)-v(x-1,i),\) we have \(v(x,i)-v(x+1,i) \ge v(x-1,i)-v(x,i).\) Combining with the facts that \(1+v(x,i)-v(x+1,i) \ge 0\) (since \(v(x+1,i)-p_i < v(x,i),\) we have \(v(x,i)-v(x+1,i) > -p_i.\) So, \(1+v(x,i)-v(x+1,i) > 1- p_i \ge 0\)) and \(1+v(x-1,i)-v(x,i) \ge 0\) (since \(v(x,i)-p_i < v(x-1,i),\) we have \(v(x-1,i)-v(x,i) > -p_i.\) So, \(1+v(x-1,i)-v(x,i) > 1- p_i \ge 0\)), we have \((1+v(x,i)-v(x+1,i))^2 \ge (1+v(x-1,i)-v(x,i))^2.\)
Step 1.2. Preservation of Condition 1 by \({\mathbf {H}}\). Now, we focus on the concavity of \(Hv(x,j)\) with respect to \(x\). Define
So, \(Hv(x,j) = \tilde{H}v(x,j) + p_j x.\) Since \(p_j x\) is linear in \(x\), we focus on the concavity of \(\tilde{H}v(x,j)\) with respect to \(x\), that is, we want to prove \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) \le \tilde{H}v(x+1,j)-\tilde{H}v(x,j).\) Let \(y^*\) be an integer such that \(y^* = \arg \max _{y=0,1,\ldots } \bigl \{ v(y,j) -p_j y \bigl \}.\) Then, from the concavity of \(v(x,j)\) with respect to \(x\), we have
We consider the following three cases:
-
Case C1.
\(x \le y^* -2\);
-
Case C2.
\(x = y^* -1\);
-
Case C3.
\(x \ge y^*\).
Case C1. \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) = v(y^*,j)-p_j y^* - (v(y^*,j)-p_j y^*) = 0 = \tilde{H}v(x+1,j)-\tilde{H}v(x,j).\)
Case C2. \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) = v(y^*+1,j)-p_j (y^*+1) - (v(y^*,j)-p_j y^*) \le 0.\) The last inequality follows because of the optimality of \(y^*.\) Moreover, \(\tilde{H}v(x+1,j)-\tilde{H}v(x,j) = v(y^*,j)-p_j y^* - (v(y^*,j)-p_j y^*) = 0.\) Hence, \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) \le \tilde{H}v(x+1,j)-\tilde{H}v(x,j).\)
Case C3. \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) = v(x+2,j)-p_j (x+2) - (v(x+1,j)-p_j(x+1)) = v(x+2,j) - v(x+1,j) - p_j.\) \(\tilde{H}v(x+1,j)-\tilde{H}v(x,j) = v(x+1,j)-p_j (x+1) - (v(x,j)-p_jx) = v(x+1,j) - v(x,j) - p_j.\) Since \( v(x+2,j) - v(x+1,j) \le v(x+1,j) - v(x,j),\) we have \(\tilde{H}v(x+2,j)-\tilde{H}v(x+1,j) \le \tilde{H}v(x+1,j)-\tilde{H}v(x,j).\)
Step 1.3. Preservation of Condition 1 by \({\mathbf {T}}_{\text{ DB }}\). Summarizing the above results and observing that \(-h(x)\) is concave, we conclude that \(T_{\text{ DB }}\) preserves Condition 1, since it is the linear combination of terms that satisfy Condition 1.
Step 2. Verification of Condition 2.
Step 2.1. Preservation of Condition 2 by \({\mathbf {G}}\). First, we focus on \(Gv(x,i).\) We want to prove \(Gv(x+1,i)-Gv(x,i) \ge -1\) for \(x \in N\) and \(i \in M\).
If \(x=0\), \(Gv(1,i)-Gv(0,i) = \max _{b \ge 0} \bigl \{ (1-b) (\max \{v(1,i)-p_i,v(0,i) \} +b)+ bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(0,i).\) We consider the following two cases:
-
Case D1.
\( v(1,i) - p_i \ge v(0,i)\);
-
Case D2.
\( v(1,i) - p_i < v(0,i)\).
Case D1. \(Gv(1,i)-Gv(0,i) = \max _{b \ge 0} \bigl \{ (1-b) (v(1,i) + b - p_i) + bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(0,i) = v(1,i) - v(0,i) \ge -1.\)
Case D2. \(Gv(1,i)-Gv(0,i) = \max _{b \ge 0} \bigl \{ (1-b) ( v(0,i) + b ) + bv(1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b)(b-p_i) \bigl \} - v(0,i) = \frac{1}{4}(1-(v(0,i)-v(1,i)))^2 - \frac{1}{4}(1-p_i)^2.\) Since \(v(1,i) - v(0,i) \ge -1,\) we have \(1-(v(0,i) - v(1,i)) = 1 +v(1,i) - v(0,i) \ge 1-1 = 0.\) Hence, \(\frac{1}{4}(1-(v(0,i)-v(1,i)))^2 - \frac{1}{4}(1-p_i)^2 \ge 0 - \frac{1}{4}(1-p_i)^2 = - \frac{1}{4}(1-p_i)^2 \ge -1.\)
If \(x \ge 1,\) we consider the following three cases:
-
Case E1.
\(x \le x^* -1\);
-
Case E2.
\(x = x^*\);
-
Case E3.
\(x \ge x^* + 1\).
Case E1. \(Gv(x+1,i)-Gv(x,i) = \max _{b \ge 0} \bigl \{ (1-b) ( v(x+1,i) + b -p_i)+ bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b -p_i) + bv(x,i) \bigl \} = v(x+1,i) - v(x,i) \ge - 1.\)
Case E2. \(Gv(x+1,i)-Gv(x,i) = \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b) + bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b -p_i) + bv(x,i) \bigl \} = \frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1-p_i)^2.\) Since \(v(x+1,i) - v(x,i) \ge -1,\) we have \(1-(v(x,i) - v(x+1,i)) = 1 +v(x+1,i) - v(x,i) \ge 1-1=0.\) Hence, \(\frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1-p_i)^2 \ge 0 - \frac{1}{4}(1-p_i)^2 = - \frac{1}{4}(1-p_i)^2 \ge -1.\)
Case E3. \(Gv(x+1,i)-Gv(x,i) = \max _{b \ge 0} \bigl \{ (1-b) ( v(x,i) + b) + bv(x+1,i) \bigl \} - \max _{b \ge 0} \bigl \{ (1-b) ( v(x-1,i) + b ) + bv(x,i) \bigl \}\, =\frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1-(v(x-1,i)-v(x,i)))^2 - (v(x-1,i) - v(x,i))=\frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 - \frac{1}{4}(1+v(x-1,i)-v(x,i))^2.\) Since \(v(x+1,i)-v(x,i) \ge -1,\) we have \(1+v(x+1,i)-v(x,i) \ge 1- 1 = 0.\) Hence, \(\frac{1}{4}(1-(v(x,i)-v(x+1,i)))^2 = \frac{1}{4}(1+v(x+1,i)-v(x,i))^2 \ge 0.\) Moreover, since \(v(x+1,i)-v(x,i) \ge -1\) and \(v(x+1,i)-v(x,i) \le v(x,i) - v(x-1,i),\) we have \(v(x,i)-v(x-1,i) \ge -1,\) that is, \(v(x-1,i)-v(x,i) \le 1.\) Since \(0 \le 1-p_i \le 1 + v(x-1,i)-v(x,i) \le 1+1 =2,\) we have \(\frac{1}{4}(1+v(x-1,i)-v(x,i))^2 \le \frac{1}{4} \times 2^2 = 1.\) Hence, \(Gv(x+1,i)-Gv(x,i) \ge 0 - 1 = -1.\)
Step 2.2. Preservation of Condition 2 by \({\mathbf {H}}\). Here, we focus on \(Hv(x,j).\) We want to prove \(Hv(x+1,j)-Hv(x,j) \ge -1\) for \(x \in N\) and \(i \in M\). We consider the following two cases:
-
Case F1.
\(x \le y^* -1\);
-
Case F2.
\(x \ge y^*\).
Case F1. \(Hv(x+1,j)-Hv(x,j) = v(y^*,j) - p_jy^* + p_j(x+1)-(v(y^*,j) - p_jy^* + p_jx) = p_j \ge -1.\)
Case F2. \(Hv(x+1,j)-Hv(x,j) = v(x+1,j) - p_j(x+1) + p_j(x+1)-(v(x,j) - p_jx + p_jx) = v(x+1,j) -v(x,j) \ge -1.\)
Step 2.3. Preservation of Condition 2 by \({\mathbf {T}}_{\text{ DB }}\). In conclusion,
Proof of Theorem 1
Since the results follow from the concavity of \(V_{\text{ I }}\), we only need to prove \(V_{\text{ I }} \in {\mathcal {V}}.\) This can be easily shown by using the facts that (i) \( V_{\text{ I }} = \lim _{n \rightarrow \infty } T^n_{\text{ I }} v\) for any \(v\) in \({\mathcal {V}}\), where \(T^n_{\text{ I }}\) is the \(n\)-fold composition of \(T_{\text{ I }}\) for \(\text{ I } \in \{\text{ MB, } \text{ SB, } \text{ DB }\}\) and (ii) \(V_{\text{ I }}\) is the unique solution of \(v = T_{\text{ I }}v\) (see Theorem 5.1 of Porteus 1982 and Theorem 6.10.4 of Puterman 1994).
Proof of Theorem 2
Recall that
where
and
Note that the optimal bid prices are determined by the maximization of \((1-b)(FV_{\text{ DB }}(x,i) +b)+ bV_{\text{ DB }}(x,i)\) with respect to \(b\). Let \(Z(b,x) = (1-b)(FV_{\text{ DB }}(x,i) +b)+ bV_{\text{ DB }}(x,i)\). To prove the monotonicity of the optimal bid prices with respect to the inventory level, it suffices to show \(Z(b^{'},x+1)-Z(b^{'},x) \le Z(b,x+1) - Z(b,x)\) for all \(x \ge 0\) and \(1 \ge b^{'} \ge b \ge 0.\)
If \(x=0,\) we want to prove \(Z(b^{'},1)-Z(b^{'},0) \le Z(b,1) - Z(b,0)\), that is, \((1-b^{'})(FV_{\text{ DB }}(1,i)+b^{'}) + b^{'}V_{\text{ DB }}(1,i) - (1-b^{'})(FV_{\text{ DB }}(0,i)+b^{'}) - b^{'}V_{\text{ DB }}(0,i) \le (1-b)(FV_{\text{ DB }}(1,i)+b) + bV_{\text{ DB }}(1,i) - (1-b)(FV_{\text{ DB }}(0,i)+b) - bV_{\text{ DB }}(0,i).\) We consider the following two cases:
-
Case G1.
\(V_{\text{ DB }}(1,i)-p_i \ge V_{\text{ DB }}(0,i)\);
-
Case G2.
\(V_{\text{ DB }}(1,i)-p_i < V_{\text{ DB }}(0,i)\).
Case G1. \(\Leftrightarrow (1-b^{'})(V_{\text{ DB }}(1,i) - p_i + b^{'}) + b^{'}V_{\text{ DB }}(1,i) - (1-b^{'})(V_{\text{ DB }}(0,i)-p_i+b^{'}) - b^{'}V_{\text{ DB }}(0,i) \le (1-b)(V_{\text{ DB }}(1,i) - p_i + b) + bV_{\text{ DB }}(1,i) - (1-b)(V_{\text{ DB }}(0,i)-p_i+b) - bV_{\text{ DB }}(0,i).\) \(\Leftrightarrow V_{\text{ DB }}(1,i) - V_{\text{ DB }}(0,i) \le V_{\text{ DB }}(1,i) - V_{\text{ DB }}(0,i).\)
Case G2. \(\Leftrightarrow (1-b^{'})(V_{\text{ DB }}(0,i) + b^{'}) + b^{'}V_{\text{ DB }}(1,i) - (1-b^{'})(V_{\text{ DB }}(0,i)-p_i+b^{'}) - b^{'}V_{\text{ DB }}(0,i) \le (1-b)(V_{\text{ DB }}(0,i) + b) + bV_{\text{ DB }}(1,i) - (1-b)(V_{\text{ DB }}(0,i)-p_i+b) - bV_{\text{ DB }}(0,i).\) \(\Leftrightarrow b^{'}(V_{\text{ DB }}(1,i)-V_{\text{ DB }}(0,i)-p_i) + p_i \le b(V_{\text{ DB }}(1,i)-V_{\text{ DB }}(0,i)-p_i) + p_i.\) \(\Leftrightarrow b^{'} \ge b.\)
If \(x \ge 1,\) we want to prove \(Z(b^{'},x+1)-Z(b^{'},x) \le Z(b,x+1) - Z(b,x)\), that is, \((1-b^{'})(FV_{\text{ DB }}(x+1,i)+b^{'}) + b^{'}V_{\text{ DB }}(x+1,i) - (1-b^{'})(FV_{\text{ DB }}(x,i)+b^{'}) - b^{'}V_{\text{ DB }}(x,i) \le (1-b)(FV_{\text{ DB }}(x+1,i)+b) + bV_{\text{ DB }}(x+1,i) - (1-b)(FV_{\text{ DB }}(x,i)+b) - bV_{\text{ DB }}(x,i).\) From the proof of Theorem 1, \(V_{\text{ DB }}(x,i)\) is concave with respect to \(x\). Hence, there exists an integer \(x^{*}\) such that for \(x \ge 1,\)
We consider the following three cases:
-
Case H1.
\(x \le x^{*}-1\);
-
Case H2.
\(x = x^{*}\);
-
Case H3.
\(x \ge x^{*}+1.\)
Case H1. \(\Leftrightarrow (1-b^{'})(V_{\text{ DB }}(x+1,i) - p_i +b^{'}) + b^{'}V_{\text{ DB }}(x+1,i) - (1-b^{'})(V_{\text{ DB }}(x,i) -p_i + b^{'}) - b^{'}V_{\text{ DB }}(x,i) \le (1-b)(V_{\text{ DB }}(x+1,i)- p_i +b) + bV_{\text{ DB }}(x+1,i) - (1-b)(V_{\text{ DB }}(x,i) - p_i +b) - bV_{\text{ DB }}(x,i).\) \(\Leftrightarrow V_{\text{ DB }}(x+1,i) - V_{\text{ DB }}(x,i) \le V_{\text{ DB }}(x+1,i) - V_{\text{ DB }}(x,i).\)
Case H2. \(\Leftrightarrow (1-b^{'})(V_{\text{ DB }}(x,i)+b^{'}) + b^{'}V_{\text{ DB }}(x+1,i) - (1-b^{'})(V_{\text{ DB }}(x,i) -p_i + b^{'}) - b^{'}V_{\text{ DB }}(x,i) \le (1-b)(V_{\text{ DB }}(x,i)+b) + bV_{\text{ DB }}(x+1,i) - (1-b)(V_{\text{ DB }}(x,i) - p_i +b) - bV_{\text{ DB }}(x,i).\) \(\Leftrightarrow b^{'}(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i)) + (1-b^{'})p_i \le b(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i)) + (1-b)p_i.\) \(\Leftrightarrow b^{'}(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i)-p_i) \le b(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i) - p_i).\) Since \(x+1=x^{*}+1 > x^{*},\) we have \(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i)-p_i < 0.\) Hence, \(\Leftrightarrow b^{'} \ge b.\)
Case H3. \(\Leftrightarrow (1-b^{'})(V_{\text{ DB }}(x,i)+b^{'}) + b^{'}V_{\text{ DB }}(x+1,i) - (1-b^{'})(V_{\text{ DB }}(x-1,i) + b^{'}) - b^{'}V_{\text{ DB }}(x,i) \le (1-b)(V_{\text{ DB }}(x,i)+b) + bV_{\text{ DB }}(x+1,i) - (1-b)(V_{\text{ DB }}(x-1,i)+b) - bV_{\text{ DB }}(x,i).\) \(\Leftrightarrow V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x-1,i) + b^{'}(V_{\text{ DB }}(x-1,i) - V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x,i) + V_{\text{ DB }}(x+1,i)) \le V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x-1,i) + b(V_{\text{ DB }}(x-1,i) - V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x,i) + V_{\text{ DB }}(x+1,i)).\) \(\Leftrightarrow b^{'}(V_{\text{ DB }}(x-1,i) - V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x,i) + V_{\text{ DB }}(x+1,i)) \le b(V_{\text{ DB }}(x-1,i) - V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x,i) + V_{\text{ DB }}(x+1,i)).\) From the proof of Theorem 1, \(V_{\text{ DB }}(x,i)\) is concave with respect to \(x\), that is, \(V_{\text{ DB }}(x+1,i)-V_{\text{ DB }}(x,i) \le V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x-1,i).\) So, \(V_{\text{ DB }}(x-1,i) - V_{\text{ DB }}(x,i) - V_{\text{ DB }}(x,i) + V_{\text{ DB }}(x+1,i) \le 0.\) Hence, \(\Leftrightarrow b^{'} \ge b.\)
Appendix 2. Tables for the other commodities: food index, oil, and platinum
See Tables 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, and 18
Rights and permissions
About this article
Cite this article
Nie, X., Boyacı, T., Gümüş, M. et al. Joint procurement and demand-side bidding strategies under price volatility. Ann Oper Res 257, 121–165 (2017). https://doi.org/10.1007/s10479-015-1838-0
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-015-1838-0