Adaptation and shunting inhibition leads to pyramidal/interneuron gamma with sparse firing of pyramidal cells

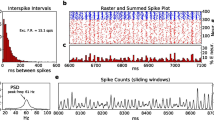
Abstract

Gamma oscillations are a prominent phenomenon related to a number of brain functions. Data show that individual pyramidal neurons can fire at rate below gamma with the population showing clear gamma oscillations and synchrony. In one kind of idealized model of such weak gamma, pyramidal neurons fire in clusters. Here we provide a theory for clustered gamma PING rhythms with strong inhibition and weaker excitation. Our simulations of biophysical models show that the adaptation of pyramidal neurons coupled with their low firing rate leads to cluster formation. A partially analytic study of a canonical model shows that the phase response curves with a near zero flat region, caused by the presence of the slow adaptive current, are the key to the formation of clusters. Furthermore we examine shunting inhibition and show that clusters become robust and generic

Appendix: Review of the result of Krupa and Szmolyan (2001) and the estimate of T e s c

Consider

$$\begin{array}{@{}rcl@{}} \frac{d x}{dt}&=&f(x,y)=-y +x^{2}+O(xy,x^{2})\\ \frac{d y}{dt} &=&-\epsilon(1+O(x,y)). \end{array} $$

(30)

Note that system (30) has the same form as system (2.5) in Krupa and Szmolyan (2001). The following estimate is a consequence of Theorem 2.1 and Remark 2.11 in Krupa and Szmolyan (2001). Let Ω₀ be the smallest positive zero of the function

$$J_{-1/3}\left (\frac{2z^{2/3}}{3}\right )+J_{1/3}\left (\frac{2z^{2/3}}{3}\right ), $$

where J _−1/3 (resp. J _1/3) are Bessel functions of the first kind.

Proposition 3

Let y ₀ ≥0 and x ₀ <0 satisfy f(x ₀ ,y ₀ )=0. Also fix δ>0. Consider a family of solutions of Eq. ( 30 ) with initial conditions x(0)=x ₀ +O(ε) and y(0)=y ₀ . Let (δ,h(ε)) be the intersection point of this trajectory with the line x=δ. Then, for sufficiently small δ,

$$ h(\epsilon)={\Omega}_{0}\epsilon^{2/3}+O(\epsilon\ln\epsilon). $$

(31)

Now let T be the time needed for a trajectory with initial condition x ₀<0, y ₀=0. It follows from Eq. (31) and the form of Eq. (30) that ε T≈−Ω₀ ε ^2/3. It follows that

$$ T\approx-{\Omega}_{0}\epsilon^{-1/3}. $$

(32)

By scaling the variables and time (30) can be brought to the form Eq. (15) and estimate Eq. (16) can be obtained.