Skip to main content

Advertisement

SpringerLink
Log in

Variational inequality formulation for the games with random payoffs

  • Published:
Journal of Global Optimization Aims and scope Submit manuscript

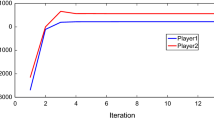
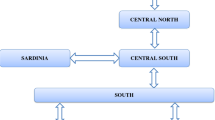
Abstract

We consider an n-player non-cooperative game with random payoffs and continuous strategy set for each player. The random payoffs of each player are defined using a finite dimensional random vector. We formulate this problem as a chance-constrained game by defining the payoff function of each player using a chance constraint. We first consider the case where the continuous strategy set of each player does not depend on the strategies of other players. If a random vector defining the payoffs of each player follows a multivariate elliptically symmetric distribution, we show that there exists a Nash equilibrium. We characterize the set of Nash equilibria using the solution set of a variational inequality (VI) problem. Next, we consider the case where the continuous strategy set of each player is defined by a shared constraint set. In this case, we show that there exists a generalized Nash equilibrium for elliptically symmetric distributed payoffs. Under certain conditions, we characterize the set of a generalized Nash equilibria using the solution set of a VI problem. As an application, the random payoff games arising from electricity market are studied under chance-constrained game framework.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adler, I.: The equivalence of linear programs and zero-sum games. Int. J. Game Theory 42(1), 165–177 (2013)

    Article  MathSciNet  Google Scholar 

  2. Basar, T., Olsder, G.J.: Dynamic Noncooperative Game Theory, 2nd edn. SIAM, Philadelphia, PA (1999)

    MATH  Google Scholar 

  3. Blau, R.A.: Random-payoff two person zero-sum games. Oper. Res. 22(6), 1243–1251 (1974)

    Article  MathSciNet  Google Scholar 

  4. Cassidy, R.G., Field, C.A., Kirby, M.J.L.: Solution of a satisficing model for random payoff games. Manag. Sci. 19(3), 266–271 (1972)

    Article  MathSciNet  Google Scholar 

  5. Charnes, A., Cooper, W.W.: Deterministic equivalents for optimizing and satisficing under chance constraints. Oper. Res. 11(1), 18–39 (1963)

    Article  MathSciNet  Google Scholar 

  6. Charnes, A., Kirby, M.J.L., Raike, W.M.: Zero–zero chance-constrained games. Theory Probab. Appl. 13(4), 628–646 (1968)

    Article  MathSciNet  Google Scholar 

  7. Cheng, J., Leung, J., Lisser, A.: Random-payoff two-person zero-sum game with joint chance constraints. Eur. J. Oper. Res. 251(1), 213–219 (2016)

    Article  MathSciNet  Google Scholar 

  8. Conejo, A.J., Nogales, F.J., Arroyo, J.M., García-Bertrand, R.: Risk-constrained self-scheduling of a thermal power producer. IEEE Trans. Power Syst. 19(3), 1569–1574 (2004)

    Article  Google Scholar 

  9. Couchman, P., Kouvaritakis, B., Cannon, M., Prashad, F.: Gaming strategy for electric power with random demand. IEEE Trans. Power Syst. 20(3), 1283–1292 (2005)

    Article  Google Scholar 

  10. Dantzig, G.B.: A proof of the equivalence of the programming problem and the game problem. In: Koopmans, T. (ed.) Activity Analysis of Production and Allocation, pp. 330–335. Wiley, New York (1951)

    Google Scholar 

  11. Facchinei, F., Fischer, A., Piccialli, V.: On generalized Nash games and variational inequalities. Oper. Res. Lett. 35, 159–164 (2007)

    Article  MathSciNet  Google Scholar 

  12. Facchinei, F., Pang, J.S.: Finite-Dimensional Variational Inequalities and Complementarity Problems. Springer, New York (2003)

    MATH  Google Scholar 

  13. Fang, K.T., Kotz, S., Ng, K.W.: Symmetric Multivariate and Related Distributions. Chapman and Hall, London (1990)

    Book  Google Scholar 

  14. Faraci, F., Raciti, F.: On generalized Nash equilibrium in infinite dimension: the Lagrange multipliers approach. Optimization 64(2), 321–338 (2015)

    Article  MathSciNet  Google Scholar 

  15. Henrion, R.: Structural properties of linear probabilistic constraints. Optimization 56(4), 425–440 (2007)

    Article  MathSciNet  Google Scholar 

  16. Jadamba, B., Raciti, F.: Variational inequality approach to stochastic Nash equilibrium problems with an application to Cournot oligopoly. J. Optim. Theory Appl. 165(3), 1050–1070 (2015)

    Article  MathSciNet  Google Scholar 

  17. Jiang, H., Shanbhag, U.V., Meyn, S.P.: Distributed computation of equilibria in misspecified convex stochastic Nash games. IEEE Trans. Autom. Control 63(2), 360–371 (2018)

    Article  MathSciNet  Google Scholar 

  18. Kakutani, S.: A generalization of Brouwer’s fixed point theorem. Duke Math. J. 8(3), 457–459 (1941)

    Article  MathSciNet  Google Scholar 

  19. Kannan, A., Shanbhag, U.V., Kim, H.M.: Addressing supply-side risk in uncertain power markets: stochastic Nash models, scalable algorithms and error analysis. Optim. Methods Softw. 28(5), 1095–1138 (2013)

    Article  MathSciNet  Google Scholar 

  20. Koshal, J., Nedić, A., Shanbhag, U.V.: Regularized iterative stochastic approximation methods for stochastic variational inequality problems. IEEE Trans. Autom. Control 58(3), 594–609 (2013)

    Article  MathSciNet  Google Scholar 

  21. Kulkarni, A.A.: Generalized Nash games with shared constraints: existence, efficiency, refinement and equilibrium constraints. Ph.D. thesis, University of Illinois at Urbana-Champaign. https://www.ideals.illinois.edu/handle/2142/18469 (2011)

  22. Kulkarni, A.A., Shanbhag, U.V.: On the variational equilibrium as a refinement of the generalized Nash equilibrium. Automatica 48(1), 45–55 (2012)

    Article  MathSciNet  Google Scholar 

  23. Lemke, C., Howson, J.: Equilibrium points of bimatrix games. SIAM J. 12, 413–423 (1964)

    MathSciNet  MATH  Google Scholar 

  24. Mazadi, M., Rosehart, W.D., Zareipour, H., Malik, O.P., Oloomi, M.: Impact of wind integration on electricity markets: a chance-constrained Nash Cournot model. Int. Trans. Electr. Energy Syst. 23(1), 83–96 (2013)

    Article  Google Scholar 

  25. Nash, J.F.: Equilibrium points in n-person games. Proc. Natl. Acad. Sci. 36(1), 48–49 (1950)

    Article  MathSciNet  Google Scholar 

  26. von Neumann, J.: On the theory of games. Math. Annalen 100, 295–320 (1928)

    Article  MathSciNet  Google Scholar 

  27. Prékopa, A.: Stochastic Programming. Springer, Dordrecht (1995)

    Book  Google Scholar 

  28. Ravat, U., Shanbhag, U.V.: On the characterization of solution sets of smooth and nonsmooth convex stochastic Nash games. SIAM J. Optim. 21(3), 1168–1199 (2011)

    Article  MathSciNet  Google Scholar 

  29. Rosen, J.B.: Existence and uniqueness of equilibrium points for concave N-person games. Econometrica 33(3), 520–534 (1965)

    Article  MathSciNet  Google Scholar 

  30. Singh, V.V., Jouini, O., Lisser, A.: Equivalent nonlinear complementarity problem for chance-constrained games. Electron. Notes Discrete Math. 55, 151–154 (2016)

    Article  Google Scholar 

  31. Singh, V.V., Jouini, O., Lisser, A.: Existence of Nash equilibrium for chance-constrained games. Oper. Res. Lett. 44(5), 640–644 (2016)

    Article  MathSciNet  Google Scholar 

  32. Singh, V.V., Jouini, O., Lisser, A.: Distributionally robust chance-constrained games: existence and characterization of Nash equilibrium. Optim. Lett. 11(7), 1385–1405 (2017)

    Article  MathSciNet  Google Scholar 

  33. Xu, H., Zhang, D.: Stochastic Nash equilibrium problems: sample average approximation and applications. Comput. Optim. Appl. 55(3), 597–645 (2013)

    Article  MathSciNet  Google Scholar 

  34. Yousefian, F., Nedić, A., Shanbhag, U.V.: Self-tuned stochastic approximation schemes for non-Lipschitzian stochastic multi-user optimization and Nash games. IEEE Trans. Autom. Control 61(7), 1753–1766 (2016)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Indian Institute of Technology Delhi, Hauz Khas, New Delhi, 110016, India

    Vikas Vikram Singh

  2. Laboratoire de Recherche en Informatique, Université Paris Sud, 91405, Orsay, France

    Abdel Lisser

Authors
  1. Vikas Vikram Singh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abdel Lisser
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vikas Vikram Singh.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Singh, V.V., Lisser, A. Variational inequality formulation for the games with random payoffs. J Glob Optim 72, 743–760 (2018). https://doi.org/10.1007/s10898-018-0664-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10898-018-0664-8

Keywords

Mathematics Subject Classification

Search

Navigation