Skip to main content
SpringerLink
Log in

A New Error Analysis of Crank–Nicolson Galerkin FEMs for a Generalized Nonlinear Schrödinger Equation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

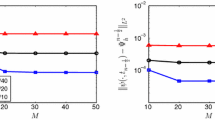
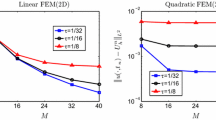
In this paper, we study linearized Crank–Nicolson Galerkin FEMs for a generalized nonlinear Schrödinger equation. We present the optimal \(L^2\) error estimate without any time-step restrictions, while previous works always require certain conditions on time stepsize. A key to our analysis is an error splitting, in terms of the corresponding time-discrete system, with which the error is split into two parts, the temporal error and the spatial error. Since the spatial error is \(\tau \)-independent, the numerical solution can be bounded in \(L^{\infty }\)-norm by an inverse inequality unconditionally. Then, the optimal \(L^2\) error estimate can be obtained by a routine method. To confirm our theoretical analysis, numerical results in both two and three dimensional spaces are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Akrivis, G.D., Dougalis, V.A., Karakashian, O.A.: On fully discrete Galerkin methods of second-order temporal accuracy for the nonlinear Schrödinger equation. Numer. Math. 59, 31–53 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  2. Antoine, X., Besse, C., Klein, P.: Absorbing boundary conditions for general nonlinear Schrödinger equations. SIAM J. Sci. Comput. 33, 1008–1033 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  3. Bao, W., Cai, Y.: Uniform error estimates of finite difference methods for the nonlinear Schrödinger equation with wave operator. SIAM J. Numer. Anal. 50, 492–521 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  4. Borzi, A., Decker, E.: Analysis of a leap-frog pseudospectral scheme for the Schrödinger equation. J. Comput. Appl. Math. 193, 65–88 (2006)

    Google Scholar 

  5. Bratsos, A.G.: A modified numerical scheme for the cubic Schrödinger equation. Numer. Methods Partial Differ. Equ. 27, 608–620 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  6. Cannon, J.R., Lin, Y.: Nonclassical \(H^1\) projection and Galerkin methods for nonlinear parabolic integro-differential equations. Calcolo 25, 187–201 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  7. Chang, Q., Jia, E., Sun, W.: Difference schemes for solving the generalized nonlinear Schrödinger equation. J. Comput. Phys. 148, 397–415 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  8. Dehghan, M., Taleei, A.: Numerical solution of nonlinear Schrödinger equation by using time-space pseudo-spectral method. Numer. Methods Partial Differ. Equ. 26, 979–990 (2010)

    MATH  MathSciNet  Google Scholar 

  9. Douglas Jr, J., Ewing, R.E., Wheeler, M.F.: A time-discretization procedure for a mixed finite element approximation of miscible displacement in porous media. RAIRO Anal. Numer. 17, 249–265 (1983)

    MATH  MathSciNet  Google Scholar 

  10. Dupont, T., Fairweather, G., Johnson, J.P.: Three-level Galerkin methods for parabolic equations. SIAM J. Numer. Anal. 11, 392–410 (1974)

    Article  MATH  MathSciNet  Google Scholar 

  11. Ewing, R.E., Wheeler, M.F.: Galerkin methods for miscible displacement problems in porous media. SIAM J. Numer. Anal. 17, 351–365 (1980)

    Article  MATH  MathSciNet  Google Scholar 

  12. He, Y.: The Euler implicit/explicit scheme for the 2D time-dependent Navier-Stokes equations with smooth or non-smooth initial data. Math. Comput. 77, 2097–2124 (2008)

    Article  MATH  Google Scholar 

  13. Hou, Y., Li, B., Sun, W.: Error analysis of splitting Galerkin methods for heat and sweat transport in textile materials. SIAM J. Numer. Anal. 51, 88–111 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  14. Jin, J., Wu, X.: Analysis of finite element method for one-dimensional time-dependent Schrödinger equation on unbounded domain. J. Comput. Appl. Math. 220, 240–256 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  15. Li, B.: Mathematical modeling, analysis and computation for some complex and nonlinear flow problems. PhD Thesis, City University of Hong Kong, Hong Kong, July (2012)

  16. Li, B., Sun, W.: Error analysis of linearized semi-implicit Galerkin finite element methods for nonlinear parabolic equations. Int. J. Numer. Anal. Model. 10, 622–633 (2013)

    MATH  MathSciNet  Google Scholar 

  17. Li, B., Sun, W.: Unconditional convergence and optimal error estimates of a Galerkin-mixed FEM for incompressible miscible flow in porous media. SIAM J. Numer. Anal. 51, 1959–1977 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  18. Li, B.K., Fairweather, G., Bialecki, B.: Discrete-time orthogonal spline collocation methods for Schrödinger equations in two space variables. SIAM J. Numer. Anal. 35, 453–477 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  19. Liao, H., Sun, Z., Shi, H.: Error estimate of fourth-order compact scheme for linear Schrödinger equations. SIAM J. Numer. Anal. 47, 4381–4401 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  20. López-Marcos, J.C., Sanz-Serna, J.M.: A definition of stability for nonlinear problems. In: Numerical Treatment of Differential Equations, Teubner-Texte zur Mathematik, Band 104, Leipzig, pp. 216–226 (1988)

  21. Pathria, D.: Exact solutions for a generalized nonlinear Schrödinger equation. Physica Scripta 39, 673–679 (1989)

    Article  Google Scholar 

  22. Pelinovsky, D.E., Afanasjev, V.V., Kivshar, Y.S.: Nonlinear theory of oscillating, decaying, and collapsing solitons in the generalized nonlinear Schrödinger equation. Phys. Rev. E 53, 1940–1953 (1996)

    Article  Google Scholar 

  23. Reichel, B., Leble, S.: On convergence and stability of a numerical scheme of coupled nonlinear Schrödinger equations. Comput. Math. Appl. 55, 745–759 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  24. Sanz-Serna, J.M.: Methods for the numerical solution of nonlinear Schrödinger equation. Math. Comput. 43, 21–27 (1984)

    Article  MATH  MathSciNet  Google Scholar 

  25. Schürmann, H.W.: Traveling-wave solutions of the cubic-quintic nonlinear Schrödinger equation. Phys. Rev. E 54, 4312–4320 (1996)

    Article  Google Scholar 

  26. Shen, J.: On an unconditionally stable scheme for the unsteady Navier-Stokes equations. J. Comput. Math. 8, 276–288 (1990)

    MATH  MathSciNet  Google Scholar 

  27. Sun, Z., Zhao, D.: On the \(L_{\infty }\) convergence of a difference scheme for coupled nonlinear Schrödinger equations. Comput. Math. Appl. 59, 3286–3300 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  28. Thomée, V.: Galerkin Finite Element Methods for Parabolic Problems. Springer, Berlin (1997)

    Book  MATH  Google Scholar 

  29. Tourigny, Y.: Optimal \(H^1\) estimates for two time-discrete Galerkin approximations of a nonlinear Schrödinger equation. IMA J. Numer. Anal. 11, 509–523 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  30. Wang, T., Guo, B., Zhang, L.: New conservative difference schemes for a coupled nonlinear Schrödinger system. Appl. Math. Comput. 217, 1604–1619 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  31. Wu, H., Ma, H., Li, H.: Optimal error estimates of the Chebyshev-Legendre spectral method for solving the generalized Burgers equation. SIAM J. Numer. Anal. 41, 659–672 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  32. Zlámal, M.: Curved elements in the finite element method. I*. SIAM J. Numer. Anal. 10, 229–240 (1973)

    Article  MATH  MathSciNet  Google Scholar 

  33. Zouraris, G.E.: On the convergence of a linear two-step finite element method for the nonlinear Schrödinger equation. M2AN Math. Model. Numer. Anal. 35, 389–405 (2001)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, City University of Hong Kong, Kowloon, Hong Kong

    Jilu Wang

Authors
  1. Jilu Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jilu Wang.

Additional information

The work was supported in part by a grant from the Research Grants Council of the Hong Kong Special Administrative Region, China (Project No. CityU 102613).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wang, J. A New Error Analysis of Crank–Nicolson Galerkin FEMs for a Generalized Nonlinear Schrödinger Equation. J Sci Comput 60, 390–407 (2014). https://doi.org/10.1007/s10915-013-9799-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-013-9799-4

Keywords

Search

Navigation