Skip to main content
SpringerLink
Log in

The Lower/Upper Bound Property of the Crouzeix–Raviart Element Eigenvalues on Adaptive Meshes

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

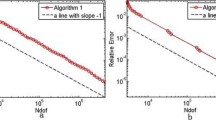
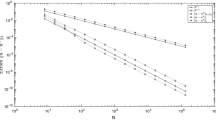
In this paper we first discover and prove that on adaptive meshes the eigenvalues by the Crouzeix–Raviart element approximate the exact ones from below when the corresponding eigenfunctions are singular. In addition, we use conforming finite elements to do the interpolation postprocessing to get the upper bound of the eigenvalues. Using the upper and lower bounds of eigenvalues we design the control condition of adaptive algorithm, and some numerical experiments are carried out under the package of Chen to validate our theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Agouzal, A.: A posteriori error estimator for finite element discretizations of Quasi–Newtonian flows. Int. J. Numer. Anal. Model. 2(2), 221–239 (2005)

    Google Scholar 

  2. Ainsworth, M., Oden, J.T.: A posteriori error estimators for the Stokes and Oseen equations. SIAM J. Numer. Anal. 34, 228–245 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  3. Ainsworth, M., Oden, J.D.: A Posteriori Error Estimates for the Finite Element Analysis. Wiley, New York (2000)

    Book  Google Scholar 

  4. Armentano, M.G., Duran, R.G.: Asymptotic lower bounds for eigenvalues by nonconforming finit element methods. Electron. Trans. Numer. Anal. 17, 92–101 (2004)

    MathSciNet  Google Scholar 

  5. Armentano, M.G., Padra, C.: A posteriori error estimates for the Steklov eigenvalue problem. Appl. Numer. Math. 58, 593–601 (2008)

    Google Scholar 

  6. Babuska, I., Rheinboldt, W.C.: A posteriori error estimates for the finite element method. Int J. Numer. Methods Eng. 12, 1597–1615 (1978)

    Article  MATH  Google Scholar 

  7. Babuska, I., Kellog, R.B., Pitkaranta, J.: Direct and inverse error estimates for finite elements with mesh refinement. Numer. Math. 33, 447–471 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  8. Babuska, I., Osborn, J.E.: Eigenvalue problems. In: Ciarlet, P.G., Lions, J.L. (eds.) Finite Element Methods (Part 1), Handbook of Numerical Analysis, vol. 2, pp. 640–787. Elsevier Science Publishers, North-Holand (1991)

    Google Scholar 

  9. Becker, R., Mao, S., Shi, Z.: A Convergent nonconforming adaptive finite element method with quasi-optimal complexity. SIAM J. Numer. Anal. 47(6), 4639–4659 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  10. Brenner, S.C., Sung, L.Y.: Linear finite element methods for planar linear elasticity. Math. Comp. 59, 321–338 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  11. Brenner, S.C., Scott, L.R.: The Mathematical Theory of Finite Element Methods, 2nd edn. Springer, New york (2002)

    Book  MATH  Google Scholar 

  12. Carstensen, C., Hu, J., Orlando, A.: Framework for the a posteriori error analysis of nonconforming finite elements. SIAM J. Numer. Anal. 45(1), 68–82 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  13. Carstensen, C., Gallistl, D.: Guaranteed lower eigenvalue bounds for the biharmonic equation. Numer. Math. 10.1007/s00211-013-0559-z

  14. Chen, L.: iFEM: an innovative finite element methods package in MATLAB. www.math.uci.edu/~chenlong/Papers/iFEMpaper.pdf (2008)

  15. Chen, Z., Nochetto, R.H.: Residual type a posteriori error estimates for elliptic obstacle problems. Numer. Math. 84, 527–548 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  16. Crouzeix, M., Raviart, P.A.: Conforming and nonconforming finite element methods for solving the stationary stokes equations. RAIRO Anal. Numer. 3, 33–75 (1973)

    MathSciNet  Google Scholar 

  17. Dai, X., Xu, J., Zhou, A.: Convergence and optimal complexity of adaptive finite element eigenvalue computations. Numer. Math. 110, 313–355 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  18. Dari, E., Durán, R., Padra, C., Vampa, V.: A posteriori error estimators for noconforming finite element methods, r.a.i.r.o., Model Math. Anal. Numer. 30, 385–400 (1996)

    MATH  Google Scholar 

  19. Durán, R.G., Gastaldi, L., Padra, C.: A posteriori error estimators for mixed approximations of eigenvalue problems. Math. Models Methods Appl. Sci. 9, 1165–1178 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  20. Durán, R.G., Padra, C., Rodriguez, R.: A posteriori error estimators for the finite element approximations of eigenvalue problems. Math. Models Methods Appl. Sci. 13, 1219–1229 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  21. Falk, R.S.: Nonconforming finite element methods for the equations of linear elasticity. Math. Comput. 57, 529–550 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  22. Grisvard, P.: Elliptic Problems in Nonsmooth Domains. Pitman, London (1985)

    MATH  Google Scholar 

  23. Hu, J., Huang, Y., Lin, Q.: The lower bounds for eigenvalues of elliptic operators-by Nonconforming finite element methods. arXiv:1112.1145v1 [math.NA] (2011)

  24. Hu, J., Huang, Y., Shen, Q.: The lower/upper bounds property of approximate eigenvalues by nonconforming finite element methods for elliptic operators. J. Sci. Comput. 58(3), 574–591 (2013)

    Google Scholar 

  25. Li, Q., Lin, Q., Xie, H.: Nonconforming finite element approximations of the steklov eigenvalue problems and its lower bound approximations. Appl. Math. 58, 129–151 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  26. Li, Y.: A posteriori error analysis of nonconforming methods for eigenvalue problem. J. Syst. Sci. Complex 22, 495–502 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  27. Lin, Q., Xie, H., Luo, F., Li, Y., Yang, Y.: Stokes eigenvalue approximations from below with nonconforming mixed finite element methods (in chinese). Math. Pract. Theory 40, 157–168 (2010)

    MathSciNet  Google Scholar 

  28. Lin, Q., Xie, H.: Recent results on lower bounds of eigenvalue problems by nonconforming finite element methods. Inverse Probl. Imaging 7(3), 795–811 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  29. Luo, S., Lin, Q., Xie, H.: Computing the lower and upper bounds of Laplace eigenvalue problem: by combining conforming and nonconforming finite element methods. Sci. China Math. 55, 1069–1082 (2012)

    Google Scholar 

  30. Mekchay, K., Nochetto, R.H.: Convergence of adaptive finite element methods for general second order linear elliptic pdes. SIAM J. Numer. Anal. 43, 1803–1827 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  31. Morin, P., Nochetto, R.H., Siebert, K.: Convergence of adaptive finite element methods. SIAM Rev. 44, 631–658 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  32. Russo, A.D., Alonso, A.E.: A posteriori error estimates for nonconforming approximations of Steklov eigenvalue problems. Comput. Math. Appl. 62(11), 4100–4117 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  33. Verfürth, R.: A Review of A Posteriori Error Estimates and Adaptive Mesh-Refinement Techniques. Wiley, New York (1996)

  34. Wang, L., Xu, X.: Foundation of Mathematics in Finite Element Methods (in Chinese). Science Press, Beijing (2004)

  35. Widlund, O.: On best error bounds for approximation by piecewise polynomial functions. Numer. Math. 27, 327–338 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  36. Wu, H., Zhang, Z.: Can we have superconvergent gradient recovery under adaptive meshes? SIAM J. Numer. Anal. 45, 1701–1722 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  37. Yang, Y., Li, Q., Li, S.: Nonconforming finite element approximations of the Steklov eigenvalue problem. Appl. Numer. Math. 59, 2388–2401 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  38. Yang, Y., Lin, F., Zhang, Z.: N-simplex Crouzeix–Raviart element for the second-order elliptic/eigenvalue problems. Int J. Numer. Anal. Model. 6(4), 615–626 (2009)

    MathSciNet  Google Scholar 

  39. Yang, Y., Zhang, Z., Lin, F.: Eigenvalue approximation from below using non-conforming finite elements. Sci. China. Math. 53, 137–150 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  40. Yang, Y., Lin, Q., Bi, H., Li, Q.: Lower eigenvalues approximation by Morley elements. Adv. Comput. Math. 36(3), 443–450 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  41. Yang, Y.: Finite Element Methods for Eigenvalue Problems (in Chinese). Science Press, Beijing (2012)

    Google Scholar 

  42. Zhang, Z., Yang, Y., Chen, Z.: Eigenvalue approximation from below by Wilsons elements. Chin. J. Numer. Math. Appl. 29, 81–84 (2007)

    Google Scholar 

Download references

Acknowledgments

The authors cordially thank the referees and the editor for their valuable comments and suggestions that led to the great improvement of this paper.

Author information

Authors and Affiliations

  1. School of Mathematics and Computer Science, Guizhou Normal University, Guiyang, 550001, China

    Yidu Yang, Jiayu Han, Hai Bi & Yuanyuan Yu

Authors
  1. Yidu Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiayu Han
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Hai Bi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Yuanyuan Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yidu Yang.

Additional information

Project supported by the National Natural Science Foundation of China (Grant Nos. 11161012 , 11201093).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, Y., Han, J., Bi, H. et al. The Lower/Upper Bound Property of the Crouzeix–Raviart Element Eigenvalues on Adaptive Meshes. J Sci Comput 62, 284–299 (2015). https://doi.org/10.1007/s10915-014-9855-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-014-9855-8

Keywords

Search

Navigation