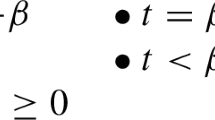Abstract
This paper is predicated on the idea that some modal operators are better understood as quantificational expressions over worlds that determine not only first-order facts but modal facts also. In what follows, we will present a framework in which these two types of facts are brought closer together. Structural features will be located in (or determined by) the worlds themselves. This result will be achieved by decomposing worlds into parts, where some of these parts will have “modal import” in the sense that they will determine structure on other worldly parts. The main upshot of this will be a clearer grasp of the interaction between modal notions.




Similar content being viewed by others
Notes
A non-determinable property in this system will be treated like a determinable with only two determinates, the having of the property and the not having.
This notion of extension is perhaps specific to epistemic states.
In symbols: Kϕ → KKϕ.
An alternative formulation is that K(Kp → q), where ‘p’ and ‘q’ have very similar truth conditions.
See Williamson [17] for details.
We switched from possible worlds talk to possibility space talk here.
The notion of possibilities being eliminated or left open by an epistemic state is more or less analogous to Lewis’s [11] notion of possibilities being eliminated or left open by the total evidence of an agent.
u̅ = (u̅ 1, u̅ 2,…u̅ 6,) and v̅ = (v̅ 1, v̅ 2,…v̅ 6) are points in the total possibility space.
In order to prove most of the technical results, we must also require μ(m) ∩ m = ∅ and that composite state types be finite subsets of N.
It is important to notice that, according to this general definition, there can be non-atomic modal states. We must then modify our definition of Ω to take this into account. If m ∈ M is a composite modal state, then Ω(w̅ m ) is defined as the natural extension of Ω(w̅ m ) to W. If M ⊂ M is a set of modal state types, then Ω(w̅ M ) is defined as the intersection of all the Ω(w̅ m ), for m ∈ M.
Note that the determinant state type is a composite state type, not a basic one.
One can think of the determinant, base and support as arities of a certain kind.
We will serve ourselves to a slight generalization of this semantics for modalities. Instead of having only one modality determined by the modal state type m, and defined in terms of Φ(w̅ m ), for w̅ m ∈ w̅ m , we will allow a pair of modalities to be determined by m, one defined in terms of Φ(w̅ m ) and the other in terms of the converse of Φ(w̅ m ). This is a straightforward adaptation of bidirectional frames to possibility spaces (cf. Blackburn et al. [2: 21–22]).
𝒯 is a tree iff < is an anti-reflexive and transitive relation < on T such that, for all t ∈ T, its restriction to {s ∈ T : s ≤ t} is linear. In a tree, there is only one path from right to left but possibly many from left to right.
Zanardo [18] also develops an non moment-history version of Ockhamist semantics.
It should be clear that ≈ t is a subset of the relation ≡ t , where ≡ t is total binary relation on W t . The Priorean semantics are the special case where ≈ t = ≡ t .
R is trichotomous iff, for all x and y, xRy, x = y or yRx.
References
Aumann, R.J. (1976). Agreeing to disagree. Annals of Statistics, 4, 1236–1239.
Blackburn, P., de Rijke, M., Venema, Y. (2001). Modal logic, Cambridge tracts in theoretical computer science (Vol. 53). Cambridge: Cambridge University Press.
Burgess, J. (1979). Logic and time. Journal of Symbolic Logic, 44, 556–582.
Davies, M., & Humberstone, L. (1980). Two notions of necessity. Philosophical Studies, 38, 1–30.
Fine, K. (2011). An abstract characterization of the deteminate/determinable relation. Philosophical Perspectives, 25(1), 161–187.
Gabbay, D., & Shehtman, V.B. (1998). Products of modal logics: part 1. Logic Journal of IGPL, 6(1).
Gaifman, H. (1988). A theory of higher order probabilities. In B. Skyrms & W. Harper (Eds.), Causation, chance, and credence. Dordrecht: Kluwer Academic Publishers.
García-Carpintero, M., & Maciá, J. (Eds.) (2006). Two-dimensional semantics. Oxford: Oxford University Press.
Kamp, H. (1979). The logic of historical necessity: part I. Unpublished typescript.
Lewis, D.K. (1973). Counterfactuals. Malden: Blackwell.
Lewis, D.K. (1986). On the plurality of worlds. Malden: Blackwell.
Lewis, D.K. (1996). Elusive knowledge. Australian Journal of Philosophy, 74, 549–567.
Prior, A. (1967). Past, present and future. Oxford: Clarendon Press.
Segerberg, K. (1973). Two-dimensional modal logic. Journal of Philosophical Logic, 2(1), 77–96.
Stalnaker, R. (1968). A theory of conditionals. In Studies in logical theory (pp. 47–65). American Philosophical Quarterly. Blackwell.
Thomason, R. (1984). Combinations of tense and modality. In D. Gabbay, & F. Guenthner (Eds.), The handbook of philosophical logic (Vol. 3, pp. 135–165). Kluwer Academic Publishers.
Thomason, R., & Gupta, A. (1980). A theory of conditionals in the context of branching time. Philosophical Review, 89(1), 65–90.
Williamson, T. (2000). Knowledge and its limits. Oxford: Oxford University Press.
Zanardo, A. (1996). Branching-time logic with quantification over branches: the point of view of modal logic. Journal of Symbolic Logic, 6(1), 1–39.
Author information
Authors and Affiliations
Corresponding author
Additional information
This paper was made possible by Grant #149410 of the Fonds de recherche du Québec - Société et culture.
Rights and permissions
About this article
Cite this article
Kennedy, N. On Possible Worlds with Modal Parts. J Philos Logic 43, 1129–1152 (2014). https://doi.org/10.1007/s10992-013-9311-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10992-013-9311-5