Abstract
Sven-Ove Hansson and Erik Olsson studied in Hansson and Olsson (Notre Dame Journal of Formal Logic, 36(1), 103–119 1995) the logical properties of an operation of contraction first proposed by Isaac Levi in Levi (1991). They provided a completeness result for the simplest version of contraction that they call Levi-contraction but left open the problem of characterizing axiomatically the more complex operation of value-based contraction or saturatable contraction. In this paper we propose an axiomatization for this operation and prove a completeness result for it. We argue that the resulting operation is better behaved than various rival operations of contraction defined in recent years.
Similar content being viewed by others
Notes
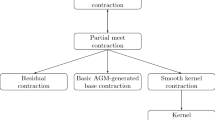
Maxichoice contraction plays also an important role when contracting unclosed set of sentences, usually called belief bases in the literature. See the work done in this area by Sven Ove Hansson in [5]. In this paper we are dealing only with contraction of theories.
In spite of the fact that candidate counterexamples to recovery abound some of them remain controversial. For example, David Makinson has argued against the validity of some of these examples in [13]. This paper examines the principal alleged counterexamples to the recovery postulate for operations of contraction on closed theories, and shows that the theories considered are implicitly ‘clothed’ with additional justificational structure. Recovery remains appropriate for ‘naked’ closed theories. A rebuttal is presented by Isaac Levi in [9]. We do not want to elucidate this issue here. We will assume that the role of recovery is at least controversial and we will explore alternative options from a logical point of view.
As we make explicit in various places below we will adopt definitions of saturatable sets commonly used in the logical literature (especially in [6]). It is unclear whether these definitions capture all the relevant aspects of contraction suggested by Levi in various writings. We think that these definitions are nevertheless interesting and deserve to be studied logically. It is important to emphasize that in this article we do not attempt to provide a complete formalization of the many ideas on contraction expressed by Levi in a multiplicity of papers and books during the last 15 years or so. We have the more modest goal of solving a logical problem left open in [6].
Levi suggested in a personal communication that perhaps there is such gap but the detailed consideration of this issue is left for future work.
We thank Arthur Paul Pedersen for pointingout this important qualification. Note that for anyα ∈ Cn(∅)it holds that S(K,α) = ∅.And it follows from the definition of selection function thatγ(S(K,α)) = K.If the condition on γ were required to hold for α ∈ Cn(∅),it would imply that K ∈ S(K,α),which contradicts the condition S(K,α) = ∅.
In the presence of all the six basic postulates including recovery, conjunctive inclusion and partial antitony do follow from conjunctive factoring. Without recovery, however, we need both conjunctive inclusion and partial antitony, in addition to conjunctive factoring, in order to establish the completeness result in the next section.
The axiom system presented in [2] substitutes α ∈ Cn(LK) for ⊩ α, where LK is a basic theory included in K.
Levi has defended antitony by appealing to the use of partitions in the presentation of contraction. Much of the counterexamples to antitony appeal to cases where the sentences α and β used in the postulate are mutually irrelevant. The use of partitions permits to filter irrelevant cases, in the sense that the two sentences in question are potential answers to the same issue. One can certainly use a semantics where partitions of this sort are deployed. In [2] such a semantics is used. But in [2] a complete axiomatization is presented from which the axioms discussed here are derivable. In particular the axiom we are discussing here is derivable for any sentence α, β, without any further syntactic restrictions. We are considering here the adequacy of axioms independently of the semantics utilized to validate them (the possible world semantics of Rott and Pagnucco, Levi’s partitional semantics, etc).
But even if one only considers instances of this axiom where the two sentences are potential answers to the same issue, the requirement that any two representable arbitrary contractions obey this tidy entailment pattern seems too orderly to be true.
References
Alchourrón, C.E., Gärdenfors, P., Makinson, D. (1985). On the logic of theory change: partial meet contraction and revision functions. Journal of Symbolic Logic, 50(2), 510–530.
Arló-Costa, H., & Levi, I. (2006). Contraction: on the decision-theoretical origins of minimal change and entrenchment. Synthese, 152(1), 129–154.
Arló-Costa, H., & Pedersen, A.P. (2010). Social norms, rational choice and belief change. In Olsson, E., & Enqvist, S. (Eds.) Belief revision meets philosophy of science, vol. 21 of logic, epistemology, and the unity of science (pp. 163–212). Dordrecht: Springer.
Gärdenfors, P. (1988). Knowledge in flux: modeling the dynamics of epistemic states, bradford book. Cambridge: MIT Press.
Hansson, S.O. (1999). A textbook of belief dynamics: theory change and database updating. Norwell: Kluwer Academic Publishers.
Hansson, S.O., & Olsson, E. (1995). Levi contractions and AGM contractions: a comparison. Notre Dame Journal of Formal Logic, 36(1), 103–119.
Kreps, D.M. (1988). Notes on the theory of choice. Boulder: Westview Press.
Levi, I. (1991). The fixation of belief and its undoing: changing beliefs through enquiry. Cambridge: Cambridge University Press.
Levi, I. (2003). Counterexamples to recovery and the filtering condition. Studia Logica, 73(2), 209–218.
Levi, I. (2004). Mild contraction: evaluation loss of information due to loss of belief. Oxford: Oxford University Press.
Lindstrom, S., & Rabinowicz, W. (1991). Epistemic entrenchment with incomparabilities and relational belief revision. In Fuhrmann, A., & Morreau, M. (Eds.) The Logic of Theory Change: workshop, Konstanz, FRG, October 1989, Proceedings, vol. 465 of Lecture Notes in Artificial Intelligence (pp. 93–126). Berlin: Springer.
Makinson, D. (1987). On the status of the postulate of recovery in the logic of theory change. Journal of Philosophical Logic, 16(4), 383–394.
Makinson, D. (1997). On the force of some apparent counterexamples to recovery. In Garzón Valdés, E., & et al. (Eds.) Normative Systems in Legal and Moral Theory: Festschrift for Carlos Alchourrón and Eugenio Bulygin. Berlin: Duncker & Humblot.
Meyer, T. (1999). Semantic belief change, PhD Thesis, Department of Computer Science and Information Systems, University of South Africa, Pretoria, South Africa.
Meyer, T., Heidema, J., Labuschagne, W., Leenen, L. (2002). Systematic withdrawal. Journal of Philosophical Logic, 31(5), 415–443.
Quine, W.V.O. (1951). Two dogmas of empiricism. The Philosophical Review, 60(1), 20–43. Reprinted in Quine, W. V. O.: From a Logical Point of View, Harvard University Press, 1953.
Rott, H. (1992). Modellings of belief change: prioritization and entrenchment. Theoria, 58(1), 21–57.
Rott, H., & Pagnucco, M. (1999). Severe withdrawal (and Recovery). Journal of Philosophical Logic, 28(5), 501–547.
Rott, H. (2004). A counterexample to six fundamental principles of belief formation. Synthese, 139(2), 225–240.
Suzumura, K. (1983). Rational choice: collective decisions and social welfare. Cambridge: Cambridge University Press.
Acknowledgements
David Makinson read an early version and we did benefit greately from his detailed comments. The authors are also grateful to Isaac Levi for comments about some philosophical issues related to this model and other useful comments. The authors are particularly grateful to the insightful and extremely useful comments made by Paul Pedersen. He read various versions of the completeness proof and pointed out certain problems, which lead to improvements and a more compact formulation of the main argument. The second author wants to acknowledge the financial support received from the Youth Program of National Social Science Foundation of China (No. 17CZX073).
Author information
Authors and Affiliations
Corresponding author
Additional information
This is a revised and extended version of a paper originally appeared in Proceedings of the 13th Conference on Theoretical Aspects of Rationality and Knowledge (TARK XIII), Groningen, pp. 55-64, July 2011. At the beginning of July 2011, the authors already planned to submit this manuscript to the present journal after incorporating possible comments from the conference participants. However, Horacio Arló Costa passed away suddenly on July 14, 2011. The second author was deeply shocked and saddened by the sudden death of Horacio Arló Costa, and it took a long time before he felt ready to carry out the task of finalizing the manuscript for submission. The second author thus would like to dedicate this paper to the memory of his revered mentor Horacio Arló Costa. His premature demise was undoubtedly a great loss not only to his family but also to the whole intellectual community.
Appendix
Appendix
Lemma 17
Let K be a theory and let÷be a contraction operator for K that satisfies success, failure, and conjunctive inclusion.Then it satisfies conjunctive covering.
Proof
For any propositions α and β,consider the following cases. First, if ⊩ α ∧ β,then it follows that K ÷ (α ∧ β) = K by vacuity. Moreover, we have that ⊩ α which also implies that K ÷ α = K.Hence the claim holds in this case. Next, suppose that\(\nvdash \alpha \wedge \beta \).According to success, we have that α ∧ β∉K ÷ (α ∧ β).Thus either α∉K ÷ (α ∧ β)or β∉K ÷ (α ∧ β).This implies that either K ÷ (α ∧ β) ⊆ K ÷ α or K ÷ (α ∧ β) ⊆ K ÷ β by conjunctive inclusion. □
Lemma 18
Let K be a theory and let÷be a relation-based saturatable contraction for K. Then it satisfies the postulate ofconjunctive reduction.
Proof
Assume that α ∈ K ÷ (α ∧ β). Andsuppose that x ∈ K ÷ β. Weneed to show that x ∈ K ÷ (α ∧ β).Consider the following cases.
- Case 1::
-
If α∉K or β∉K,then it follows that α ∧ β∉K.Then by vacuity, we have that K ÷ (α ∧ β) = K.According to inclusion, we have that x ∈ K ÷ α ⊆ K = K ÷ (α ∧ β)as required.
- Case 2::
-
If α ∈ K,β ∈ K,and ⊩ α ∧ β,then again by vacuity, we have that K ÷ (α ∧ β) = K.Similarly, it follows that x ∈ K ÷ α ⊆ K ÷ (α ∧ β)by inclusion.
- Case 3::
-
Now consider the case that α,β ∈ K ∖ Cn(∅).Assume that x∉K ÷ (α ∧ β).It follows that there is some Z such thatx∉Z ∈ γ(S(K,α ∧ β)).Since \(K\div (\alpha \wedge \beta )=\bigcap \gamma (S(K,\alpha \wedge \beta ))\),we have that K ÷ (α ∧ β) ⊆ Z.Thus it follows from α ∈ K ÷ (α ∧ β)that α ∈ Z.Furthermore, according to the definition of saturatable set, we have thatCn(Z ∪{¬(α ∧ β)})is maximally consistent. Hence, either β or ¬β belongs to Cn(Z ∪{¬(α ∧ β)}).
If β ∈ Cn(Z ∪{¬(α ∧ β)}), thenZ ⊩¬(α ∧ β) → β. From this itfollows that Z ⊩ β, since(¬(α ∧ β) → β) ≡ β. However, sinceα ∈ Z, it follows thatα ∧ β ∈ Z, which is contrary tothe assumption that Z ∈ S(K,α ∧ β).
If ¬β ∈ Cn(Z ∪{¬(α ∧ β)}), thenZ ⊩¬(α ∧ β) →¬β. It followsthat Z ⊩ β → α from thefact (¬(α ∧ β) →¬β) ≡ β → α. Sinceβ → α ∈ Z, we thus havethat Cn(Z ∪{¬(α ∧ β)}) = Cn(Z ∪{¬β}). Thismeans that Z ∈ S(K,β)according to the definition of saturatable set. Now let X be any element ofS(K,β). According to the upper bound property, there is some X′such that X ⊆ X′∈ K⊥β. SinceK⊥β ⊆ K⊥(α ∧ β), thus we havethat X′∈ K⊥(α ∧ β). Further, weknow that for any Y ∈ K⊥α,Y ∈ S(K,α). Thus weknow that X′∈ S(K,α ∧ β).According to the property of weak monotonicity, it follows fromX ⊆ X′thatX ≤ X′. Thenfrom X′∈ S(K,α ∧ β)andZ ∈ γ(S(K,α ∧ β))we have thatX′≤ Z. By transitivity, itimplies that X ≤ Z. Since X isan arbitrary element of S(K,β)and Z ∈ S(K,β), this impliesthat Z ∈ γ(S(K,α)). Andthus K ÷ α ⊆ Z. Sincex ∈ K ÷ α, it implies thatx ∈ Z, which contradictsthe fact that x∉Z(which was derived from the main assumption, namely thatx∉K ÷ (α ∧ β)).
Therefore, we can conclude x ∈ Z,which establishes that K ÷ β ⊆ K ÷ (α ∧ β).□
Lemma 19
Let÷be a relation-based saturatable contraction over K. Then it satisfies partial antitony.
Proof
Suppose that x ∈ K ÷ α and x ∈ Cn(α). We needto show that x ∈ K ÷ (α ∧ β).Consider the following cases.
- Case 1::
-
If α∉K or β∉K,then it follows that α ∧ β∉K.Then by vacuity, we have that K ÷ (α ∧ β) = K.According to inclusion, we have that x ∈ K ÷ α ⊆ K = K ÷ (α ∧ β)as required.
- Case 2::
-
If α ∈ K,β ∈ K,and ⊩ α ∧ β,then again by vacuity, we have that K ÷ (α ∧ β) = K.Similarly, it follows that x ∈ K ÷ α ⊆ K ÷ (α ∧ β)by inclusion.
- Case 3::
-
Now consider the case that α,β ∈ K ∖ Cn(∅).Assume that x∉K ÷ (α ∧ β).It follows that there is some Z such thatx∉Z ∈ γ(S(K,α ∧ β)).According to the definition of saturatable set, we have thatCn(Z ∪{¬(α ∧ β)})is maximally consistent in L. Hence, either α or ¬α belongs to Cn(Z ∪{¬(α ∧ β)}).
If α ∈ Cn(Z ∪{¬(α ∧ β)}), thenZ ⊩¬(α ∧ β) → α. This followsthat Z ⊩ α, since(¬(α ∧ β) → α) ≡ α. However,since x ∈ Cn{α}, itfollows that x ∈ Z,which contradicts the assumption.
If ¬α ∈ Cn(Z ∪{¬(α ∧ β)}), thenZ ⊩¬(α ∧ β) →¬α. It followsthat Z ⊩ α → β from thefact (¬(α ∧ β) →¬α) ≡ α → β. Sinceα → β ∈ Z, we thus havethat Cn(Z ∪{¬(α ∧ β)}) = Cn(Z ∪{¬α}). Thismeans that Z ∈ S(K,α)according to the definition of saturatable set. Now let X be any element ofS(K,α). According to the upperbound property, there is some X′such that X ⊆ X′∈ K⊥α. SinceK⊥α ⊆ K⊥(α ∧ β), thus we have thatX′∈ K⊥(α ∧ β). Further, we know thatfor any Y ∈ K⊥α it follows thatY ∈ S(K,α), which implies thatX′∈ S(K,α ∧ β). According to the propertyof weak monotonicity, from X ⊆ X′we have that X ≤ X′.It follows from X′∈ S(K,α ∧ β)and Z ∈ γ(S(K,α ∧ β))thatX′≤ Z. By transitivity, wehave that X ≤ Z. Since X isan arbitrary element of S(K,α)and Z ∈ S(K,α), itimplies that Z ∈ γ(S(K,α)),and thus K ÷ α ⊆ Z.Since x ∈ K ÷ α, itimplies that x ∈ Z,which again contradicts the assumption.
Therefore, we can concludex ∈ Z, which establishes that(K ÷ α) ∩ Cn(α) ⊆ K ÷ (α ∧ β).□
Lemma 20
Let K be a theory and let÷be a contraction operator for K that satisfies closure, inclusion, vacuity, extensionality, andconjunctive inclusion. Then it satisfies conjunctive overlap and conjunctive reduction if andonly if it satisfies conjunctive factoring.
Proof
(⇐) Conjunctive overlap follows directly from conjunctive factoring. Itremains to be shown that conjunctive reduction holds. Assume thatα ∈ K ÷ (α ∧ β).In the limiting case when ⊩ α, conjunctive reduction trivially holds. Now consider the case when\(\nvdash \alpha \).Then vacuity yields α∉K ÷ α.Since α ∈ K ÷ (α ∧ β),it is impossible that K ÷ (α ∧ β) = K ÷ α and K ÷ (α ∧ β) = (K ÷ α) ∩ (K ÷ β).By ruling out these two possibilities, we can conclude from conjunctive factoring thatK ÷ (α ∧ β) = K ÷ β.Therefore, conjunctive reduction holds as well.
(⇒)Consider K ÷ (α ∧ β).There are three cases that are mutually exclusive and exhaustive: eitherα ∈ K ÷ (α ∧ β)and β∉K ÷ (α ∧ β),or α∉K ÷ (α ∧ β)and β ∈ K ÷ (α ∧ β),or α∉K ÷ (α ∧ β)and β∉K ÷ (α ∧ β).We will show that in the first case K ÷ β = K ÷ (α ∧ β),in the second case K ÷ α = K ÷ (α ∧ β)and in the third case K ÷ α ∩ K ÷ β = K ÷ (α ∧ β).
Consider the first case: α ∈ K ÷ (α ∧ β)and β∉K ÷ (α ∧ β).Then by conjunctive inclusion we have that K ÷ (α ∧ β) ⊆ K ÷ β.On the other hand since α ∈ K ÷ (α ∧ β),we have by conjunctive reduction that K ÷ β ⊆ K ÷ (α ∧ β).
The case α∉K ÷ (α ∧ β)and β ∈ K ÷ (α ∧ β)is analogous, yielding the desired K ÷ α = K ÷ (α ∧ β).
Finally in the third case by two applications of conjunctive inclusion we have thatK ÷ (α ∧ β) ⊆ (K ÷ α) ∩ (K ÷ β). Conjunctive overlap provides the converse inclusion, proving the proposition.□
Proposition 23
Let K be a theory and letγbe a selection function for K. Then:
-
(i)
The completion functionγ∗is a selection function for K;
-
(ii)
γ(S(K,α)) ⊆ γ∗(S(K,α))for allα;
-
(iii)
\(\bigcap \gamma (S(K,\alpha ))=\bigcap \gamma ^{*}(S(K,\alpha ))\) for all α .
Proof
-
(i)
It is easy to verified that γ∗satisfies the two conditions of the definition of selection function.
-
(ii)
If S(K,α)is empty, then we have that γ(S(K,α)) = γ∗(S(K,α)) = {K},which follows that γ(S(K,α)) ⊆ γ∗(S(K,α))as desired. If S(K,α)is nonempty, then it holds for every X ∈ S(K,α) that \(\bigcap \gamma (S(K,\alpha ))\subseteq X\).According to the definition of γ∗we have that X ∈ γ∗(S(K,α)).Therefore, we can conclude that γ(S(K,α)) ⊆ γ∗(S(K,α))for all α.
-
(iii)
First, if S(K,α)is empty, then similarly we have that γ(S(K,α)) = γ∗(S(K,α)) = {K},which implies that \(\bigcap \gamma (S(K,\alpha ))=\bigcap \gamma ^{*}(S(K,\alpha ))\).Second, if S(K,α)is nonempty. From the definition of γ∗we have that \(\bigcap \gamma (S(K,\alpha ))\subseteq X\)for every X ∈ γ∗(S(K,α)).It shows that \(\bigcap \gamma (S(K,\alpha ))\subseteq \bigcap \gamma ^{*}(S(K,\alpha ))\).Moreover, by (ii) of the present proof, we know thatγ(S(K,α)) ⊆ γ∗(S(K,α)).This implies that \(\bigcap \gamma ^{*}(S(K,\alpha ))\subseteq \bigcap \gamma (S(K,\alpha ))\).We can therefore conclude that \(\bigcap \gamma (S(K,\alpha ))=\bigcap \gamma ^{*}(S(K,\alpha ))\).
□
Theorem 24
Let K be a theory and let÷be an operator for K that satisfies closure, inclusion, vacuity, success, extensionality,conjunctive inclusion, partial antitony and conjunctive factoring. Then÷is a relation-based saturatable contraction determined by a selection functionγwhichsatisfies the following conditions:
-
(i)
γsatisfies the marking-off identity, i.e.,γ(S(K,α)) = {X ∈ S(K,α)∣Y ≤ X, for all Y ∈ S(K,α)},for allα ∈ K ∖ Cn(∅);
-
(ii)
The relation≤is transitive;
-
(iii)
The relation≤satisfies the weak monotonicity, i.e., ifY ⊂ X,thenY ≤ X.
Proof
Let K be any theory, and ÷a Levi-contraction function over K, determined by a selection functionγ. We have toshow that if ÷satisfies in addition conjunctive inclusion, partial antitony, and conjunctive factoring, then there is a transitive and weakly monotonic relation≤suchthat γ satisfies the marking-off identity with respect to this relation. It is easy to verify that the selectionfunction γ i question is complete. So let us define explicitly the relation≤over thedomain KΔL = {X∣X ∈ S(K,α) for some α ∈ K ∖ Cn(∅)}suchthat for all X,Y ∈ KΔL,Y ≤ X if andonly if one of the following conditions holds
-
(1)
Y ⊆ X;
-
(2)
There is α ∈ K ∖ Cn(∅)such that Y ∈ S(K,α)AND There is some α ∈ K ∖ Cn(∅)[(X ∈ S(K,α),and K ÷ α ⊆ X),AND for all β ∈ K ∖ Cn(∅)(if X ⊆ X′∈ K⊥(β ∧ α)and Y ⊆ Y′∈ K⊥(β ∧ α),and K ÷ β ⊆ Y′,then (if K ÷ (α ∧ β)≠K ÷ α,then K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β)and K ÷ β ⊆ X′)].
We need to show that the relation defined in this way satisfies the three conditions given in theproposition.
-
(i)
We first show that γ satisfies the marking-off identity. Let α ∈ K ∖ Cn(∅).Suppose that X ∈ γ(S(K,α)) ⊆ S(K,α).We need to show that X ∈{X ∈ S(K,α)∣Y ≤ X, for all Y ∈ S(K,α)}.It suffices to show that Y ≤ X for any Y ∈ S(K,α).
Then it is clear that the first part of condition (2) is already satisfied. Letβ ∈ K ∖ Cn(∅).And suppose that K ÷ β ⊆ Y′We need to show that K ÷ β ⊆ X′and, if K ÷ α ∧ β≠K ÷ α,then K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β.
Let us focus first on K ÷ (α ∧ β).We know by conjunctive covering that eitherK ÷ (α ∧ β) ⊆ K ÷ β or K ÷ (α ∧ β) ⊆ K ÷ α.Assume that K ÷ (α ∧ β) ⊆ K ÷ β.Since it is assumed K ÷ β ⊆ Y′,we have K ÷ (α ∧ β) ⊆ K ÷ β ⊆ Y′∈ K⊥α,which implies that α∉K ÷ (α ∧ β).Thus by conjunctive inclusion we have thatK ÷ (α ∧ β) ⊆ K ÷ α.Therefore in both cases we have that K ÷ (α ∧ β)⊆ K ÷ α.
By conjunctive factoring K ÷ (α ∧ β)is either equal to K ÷ α,to K ÷ β or to the intersection of these two sets. Suppose thatK ÷ (α ∧ β)≠K ÷ α.If K ÷ (α ∧ β) = K ÷ β,then it implies that K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β,for we already have that K ÷ (α ∧ β) ⊆ K ÷ α.Hence it holds that K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β.This proves that if K ÷ (α ∧ β)≠K ÷ α,then K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β.
We need to prove now that K ÷ β ⊆ X′.Assume that δ ∈ K ÷ β.Consider the following instance of partial antitony:
$$(K\div\beta)\cap Cn(\beta)\subseteq K\div(\alpha\wedge\beta). $$Since we assumed that δ ∈ K ÷ β we have as well that δ ∨ β ∨ α ∈ K ÷ β,and since δ ∨ β ∨ α ∈ Cn(β)we havethat δ ∨ β ∨ α ∈ K ÷ (α ∧ β). Moreoversince we have that K ÷ (α ∧ β) ⊆ K ÷ α ⊆ X ⊆ X′,we also have that δ ∨ β ∨ α ∈ X′.
Note that X′∈ K⊥(β ∧ α)and δ ∈ K. It thusimplies that X′∪{α,β}⊩ δ. Sowe have that ¬β → (¬α → δ) ∈ X′and β → (α → δ) ∈ X′, whichentail that δ ∈ X′. Itfollows that K ÷ β ⊆ X′.This completes the proof by establishing the desired result thatY ≤ X.
For the converse, suppose that X∉γ(S(K,α))for some α ∈ K ∖ Cn(∅). We needto show that X∉{X ∈ S(K,α)∣Y ≤ X, for all X ∈ S(K,α)}. Notethat if X∉S(K,α), then ittrivially follows that X∉{X ∈ S(K,α)∣Y ≤ X, for all X ∈ S(K,α)}.Thus, suppose that X ∈ S(K,α).To show that X∉{X ∈ S(K,α)∣Y ≤ X, for all X ∈ S(K,α)}, itsuffices to find some Y ∈ S(K,α) with \(Y\nleq X\). SinceX ∈ S(K,α), it means thatS(K,α)is nonempty, whichfollows that γ(S(K,α))is nonempty.Thus let Y ∈ γ(S(K,α)). Then we havethat \(K\div \alpha =\bigcap \gamma (S(K,\alpha ))\subseteq Y\). On the other hand,since X∉γ(S(K,α)), we can concludefrom the property of γ that \(\bigcap \gamma (S(K,\alpha ))\nsubseteq X\). Thus we have\(K\div \alpha \nsubseteq X\). From these, wecan conclude that \(Y\nsubseteq X\),which establishes that condition (1) does not hold. And note thatX,Y ∈ S(K,α),K ÷ α ⊆ Y, but\(K\div \alpha \nsubseteq X\).
We have to check that condition (2) is not satisfied either. Notice that the negation ofcondition (2) is given as follows:
¬Thereis α ∈ K ∖ Cn(∅)suchthat Y ∈ S(K,α)ORFor all α ∈ K ∖ Cn(∅)[if (X,Y ∈ S(K,α), andK ÷ α ⊆ X), THENthere is β ∈ K ∖ Cn(∅)(X ⊆ X′∈ K⊥(β ∧ α)andY ⊆ Y′∈ K⊥(β ∧ α), andK ÷ β ⊆ Y′, andeither ¬(if K ÷ (α ∧ β)≠K ÷ α,then K ÷ (α ∧ β) = K ÷ α ∩ K ÷ β)or K ÷ β⫅̸X′)].
We need to find an adequate β in K in order to prove the truth of the second disjunct. Can we argue that such aβ exists in K? The answer is yes if the belief sets used in the theory are finitely axiomatizable in the sense that a formulaψ represents a beliefset K if and only if K = {ϕ : ψ ⊩ ϕ}.If we assume that all belief sets are representable by a formulain the language we can argue as follows to identify the neededβ in K:
Let w be the maximal and consistent belief set such thatCn(Y ∪{¬α}) = w. Let¬ψ represent w.Then clearly ψ ∈ K. Setβ = ψ. Clearly also in thiscase K ÷ β ⊆ Y′. Assume now bycontradiction that K ÷ β ⊆ X′.Let [K ÷ β]the set of worlds that correspond to the theoryK ÷ β. It is clear thatw is the only ¬α world in [K ÷ β]. Nownotice that since X′≠Y′we have that [Y′]= {w}∪ [K]and[Y′]={w∗}∪ [K]where w andw ∗should be distinct¬α worlds. But from ourassumption we have that [X′] ⊆ [K ÷ β],which entails that w ∗is in [K ÷ β].But this is impossible because we already saw that[K ÷ β]should containa unique ¬α world, namely w.
In addition we also have that X′,Y′∈ K⊥(β ∧ α).Notice that X,Y ∈ S(K,α).Therefore X′,Y′∈ K⊥α,and since K⊥α ⊆ K⊥(β ∧ α)we are done.
-
(ii)
Next we are going to show that ≤is transitive. Let X,Y,Z ∈ KΔL.Suppose that Z ≤ Y and Y ≤ X. We needto show that Z ≤ X.Let us consider the following cases.
- Case 1::
-
Both Z ≤ Y and Y ≤ X hold due to condition (1). In this case, we have thatZ ⊆ Y and Y ⊆ X.Thus according to the definition, we have thatZ ≤ X as required.
- Case 2::
-
Z ≤ Y holds because of condition (1), and Y ≤ X holds due to condition (2). So, we have that:
- (2’):
-
There is α ∈ K ∖ Cn(∅)such that Y ∈ S(K,α)AND There is some α′∈ K ∖ Cn(∅)[(X ∈ S(K,α′),and K ÷ α′⊆ X),AND for all β ∈ K ∖ Cn(∅)(if X ⊆ X′∈ K⊥(β ∧ α′)and Y ⊆ Y′∈ K⊥(β ∧ α′),and K ÷ β ⊆ Y′,then (if K ÷ (α′∧ β)≠K ÷ α′,then K ÷ (α′∧ β) = K ÷ α′∩ K ÷ β)and K ÷ β ⊆ X′)].
And we need to prove that:
- (2”):
-
There is α ∈ K ∖ Cn(∅)such that Z ∈ S(K,α)AND There is some α′∈ K ∖ Cn(∅)[(X ∈ S(K,α′),and K ÷ α′⊆ X),AND for all β ∈ K ∖ Cn(∅)(if X ⊆ X′∈ K⊥(β ∧ α′)and Z ⊆ Z′∈ K⊥(β ∧ α′),and K ÷ β ⊆ Z′,then (if K ÷ (α′∧ β)≠K ÷ α′,then K ÷ (α′∧ β) = K ÷ α′∩ K ÷ β)and K ÷ β ⊆ X′)].
First since Z ≤ Y holds becauseof condition (1), we have that Z ⊆ Y.And note that Z,Y ∈ KΔL. It thusmust be the case that Z′ = Y′and Z′,Y′∈ K⊥α for someα ∈ K ∖ Cn(∅). Consider thisparticular α. It thusfollows from Z′,Y′∈ K⊥α that Z,Y ∈ S(K,α)for thisparticular α.So the first existential clause is satisfied. The second existential clause is also satisfied given thatY ≤ X holds due to condition (2). And the universal clause can be easily established forZ′ = Y′and thefact that Y ≤ X holds due to condition (2).
We are first going to show that K ÷ β ⊆ X′.Let δ ∈ K ÷ β. First itfollows from X′∈ K⊥(β ∧ α′),Z′∈ K⊥(β ∧ α′), andY′∈ K⊥α thatX′,Y′,Z′∈ K⊥(α ∧ β ∧ α′). SinceY ≤ X holds because of condition(2), we can infer that K ÷ (α ∧ β) ⊆ X′by taking (α ∧ β)as theinstance of β in (2’).It follows from δ ∈ K ÷ β that δ ∨ β ∨ α′∈ K ÷ β. Andclearly δ ∨ β ∨ α′∈ Cn(β).Now consider the following instance of partial antitony:
Then it implies that δ ∨ β ∨ α′∈ K ÷ (α ∧ β) ⊆ X′.Moreover, since we assumed that X′∈ K⊥(β ∧ α′)and δ ∈ K ÷ β ⊆ K, we have aswell that X′∪{α′,β}⊩ δ. So theseimply that δ ∈ X′. Thiscompletes the proof of K ÷ β ⊆ X′.
- Case 3::
-
Z ≤ Y holds because of condition (2), and Y ≤ X holds due to condition (1). This case is similar to the previous case.
- Case 4::
-
Both Z ≤ Y and Y ≤ X hold because condition (2). Unpacking definitions we have:
- (2-Z-Y):
-
There is α ∈ K ∖ Cn(∅)such that Z ∈ S(K,α)AND There is some α′∈ K ∖ Cn(∅)[(Y ∈ S(K,α′),and K ÷ α′⊆ Y ),AND for all β ∈ K ∖ Cn(∅)(if Z ⊆ Z′∈ K⊥(β ∧ α′)and Y ⊆ Y′∈ K⊥(β ∧ α′),and K ÷ β ⊆ Z′,then (if K ÷ (α′∧ β)≠K ÷ α′,then K ÷ (α′∧ β) = K ÷ α′∩ K ÷ β)and K ÷ β ⊆ Y′)].
On the other hand we also have:
- (2-Y-X):
-
There is α′∈ K ∖ Cn(∅)such that Y ∈ S(K,α′)AND There is some α″∈ K ∖ Cn(∅)[(X ∈ S(K,α″),and K ÷ α″⊆ X),AND for all β ∈ K ∖ Cn(∅)(if X ⊆ X′∈ K⊥(β ∧ α″)and Y ⊆ Y′∈ K⊥(β ∧ α″),and K ÷ β ⊆ Y′,then (if K ÷ α″∧ β≠K ÷ α″,then K ÷ (α″∧ β) = K ÷ α″∩ K ÷ β)and K ÷ β ⊆ X′)].
And we need to prove:
- (2-Z-X):
-
There is α ∈ K ∖ Cn(∅)such that Z ∈ S(K,α)AND There is some α″∈ K ∖ Cn(∅)[(X ∈ S(K,α″),and K ÷ α″⊆ X),AND for all β ∈ K ∖ Cn(∅)(if X ⊆ X′∈ K⊥(β ∧ α″)and Z ⊆ Z′∈ K⊥(β ∧ α″),and K ÷ β ⊆ Z′,then (if K ÷ (α″∧ β)≠K ÷ α″,then K ÷ (α″∧ β) = K ÷ α″∩ K ÷ β)and K ÷ β ⊆ X′)].
It is clear that the first existential clause of (2-Z-X) is given by (2-Z-Y) while the second isgiven by (2-Y-X). We need to prove the universally quantified clause. Assume thatX ⊆ X′∈ K⊥β ∧ α″andZ ⊆ Z′∈ K⊥β ∧ α″, andK ÷ β ⊆ Z′. We need toprove that K ÷ β ⊆ X′and (if K ÷ α″∧ β≠K ÷ α″, thenK ÷ (α″∧ β) = K ÷ α″∩ K ÷ β). We will prove fist thatK ÷ β ⊆ X′, and then we will use thisresult to prove that (if K ÷ α″∧ β≠K ÷ α″,then K ÷ (α″∧ β) = K ÷ α″∩ K ÷ β).
By the property of reminder sets establishing thatK⊥(β ∧ α′∧ α″) = K⊥(β ∧ α″) ∪ K⊥α′we havethat Z′,X′,Y′∈ K⊥(β ∧ α′∧ α″). Infact, Y ∈ S(K,α′), andtherefore Y′∈ K⊥α′and Z′,X′are in K⊥(β ∧ α″).
By covering, either K ÷ (β ∧ α′∧ α″) ⊆ K ÷ β orK ÷ (β ∧ α′∧ α″) ⊆ K ÷ (α′∧ α″). In the first case we have thatK ÷ (β ∧ α′∧ α″) ⊆ Z′. So, by taking appropriateinstances of β in (2-Z-Y)and (2-Y-X) we have that K ÷ (β ∧ α′∧ α″) ⊆ X′.
On the other hand if we consider the caseK ÷ (β ∧ α′∧ α″) ⊆ K ÷ (α′∧ α″), we proceed by cases. Byconjunctive covering either K ÷ (α′∧ α″) = K ÷ α′orK ÷ (α′∧ α″) ⊆ K ÷ α″. In the first case we have thatK ÷ (β ∧ α′∧ α″) ⊆ K ÷ α′⊆ Y′. Then we can fire (2-Y-X)and we get K ÷ (β ∧ α′∧ α″) ⊆ X′. In the secondcase we have that K ÷ (β ∧ α′∧ α″) ⊆ K ÷ α″⊆ X′. So, in allpossible cases we have that K ÷ (β ∧ α′∧ α″) ⊆ X′.
We need to prove that K ÷ β ⊆ X′.Assume that δ ∈ K ÷ β.Consider the following instance of partial antitony:
Since we assumed that δ ∈ K ÷ β we have as well that (β ∨ α′∨ α″∨ δ) ∈ K ÷ β,and since (β ∨ α′∨ α″∨ δ) ∈ Cn(β)wehave that (β ∨ α′∨ α″∨ δ) ∈ K ÷ (β ∧ α′∧ α″) ⊆ X′.
On the other hand since X ∈ K⊥(α″∧ β)and δ ∈ K wehave that X′∪{α″,β}⊩ δ from which (by monotonicity of the notion of consequence) we have:X′∪{α″,α′,β}⊩ δ. So, clearlyδ ∈ X′. This completesthe proof of K ÷ β ⊆ X′.
Now we need to prove that (if K ÷ α″∧ β≠K ÷ α″,then K ÷ (α″∧ β) = K ÷ α″∩ K ÷ β).Assume that antecedent. By conjunctive covering eitherK ÷ (α″∧ β) ⊆ K ÷ β orK ÷ (α″∧ β) ⊆ K ÷ α″. Let’s start with the firstdisjunct. Since we have that K ÷ (α″∧ β) ⊆ K ÷ β and we proved that K ÷ β ⊆ X′⊆ K⊥α″, we knowthat α″∉K ÷ (α″∧ β). Therefore by conjunctive inclusionK ÷ (α″∧ β) ⊆ K ÷ α″. In addition byconjunctive overlap we have that K ÷ α″∩ K ÷ β ⊆ K ÷ (α″∧ β).Therefore we have that K ÷ α″∩ K ÷ β = K ÷ (α″∧ β) as desired.
Let’s consider now the second disjunct K ÷ (α″∧ β) ⊆ K ÷ α″.Since we assumed that K ÷ (α″∧ β)≠K ÷ α″,we know that K ÷ α″⫅̸K ÷ (α″∧ β).Therefore by conjunctive reduction we have thatβ∉K ÷ (α″∧ β), and this by conjunctive inclusion yields that K ÷ (α″∧ β) ⊆ K ÷ β.Now by invoking conjunctive overlap again, we have thatK ÷ α″∩ K ÷ β = K ÷ (α″∧ β)asdesired. This completes the general proof.
-
(iii)
We need to show that ≤satisfies the weak monotonicity. Let X,Y ∈ S(K,α)for some α ∈ K ∖ Cn(∅).Assume that Y ⊂ X.According to condition (1), it directly follows fromY ⊂ X that Y ≤ X as desired.
□
Rights and permissions
About this article
Cite this article
Costa, H.A., Liu, H. A Representation Result for Value-based Contraction. J Philos Logic 47, 965–989 (2018). https://doi.org/10.1007/s10992-018-9457-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10992-018-9457-2