Skip to main content
SpringerLink
Log in

Virtual matrix pencil method for 2-D DOA estimation with a two-nested-shape-array

  • Published:
Multidimensional Systems and Signal Processing Aims and scope Submit manuscript

Abstract

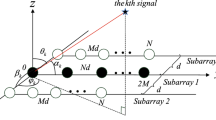
A new two-nested-shape array geometry with three different inter-sensor spacings is designed to achieve enhanced degrees of freedom and doubled aperture length. The virtual matrix pencil method (VMPM) is proposed for this new array to estimate the two-dimensional direction-of-arrival (2-D DOA) of the signal sources, where the three different inter-sensor spacings are used to construct a virtual sparse two-parallel-shape array with much larger number sensors and achieve more accurate DOA estimations. Compared with the improved propagator method, the VMPM has better angle estimation accuracy, and is capable of resolving \(\hbox {O}(P^{2}/32)\) sources with \(P\) sensors. The statistical analysis of these two methods are studied, and the asymptotic variance expressions of their estimation errors are derived. Moreover, these asymptotic variance expressions are simplified for the case of one signal, and the quantitative comparisons are performed to demonstrate the performance advantage of the VMPM. The simulation results verify the validity of the VMPM.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Notes

  1. For the case when the number of sensors in each ULA is even, we can assume there is a virtual element 0 at the center of the ULA.

References

  • Al-Jazzar, S. O., McLernon, D. C., & Smadi, M. A. (2010). SVD-based joint azimuth/elevation estimation with automatic pairing. Signal Processing, 90(5), 1669–1675.

    Article  MATH  Google Scholar 

  • Chambers, C., Tozer, T. C., Sharman, K. C., & Durrani, T. S. (1996). Temporal and spatial sampling influence on the estimates of superimposed narrowband signals: When less can mean more. IEEE Transactions on Signal Processing, 44(12), 3085–3098.

    Article  Google Scholar 

  • Changuel, H., Harabi, F., & Gharsallah, A. (2006). 2-L-shape two-dimensional arrival angle estimation with a classical subspace algorithm. In IEEE international symposium on industrial electronics, Montreal, Quebec, Canada (Vol. 1, pp. 603–607).

  • Chevalier, P., Albera, L., Ferreol, A., & Comon, P. (2005). On the virtual array concept for higher order array processing. IEEE Transactions on Signal Processing, 53(4), 1254–1271.

    Article  MathSciNet  Google Scholar 

  • Chevalier, P., Ferreol, A., & Albera, L. (2006). High-resolution direction finding from higher order statistics: The 2q-MUSIC algorithm. IEEE Transactions on Signal Processing, 54(8), 2986–2997.

    Article  Google Scholar 

  • Dogan, M. C., & Mendel, J. M. (1995). Applications of cumulants to array processing. I: Aperture extension and array calibration. IEEE Transactions on Signal Processing, 43(5), 1200–1216.

    Article  Google Scholar 

  • Fernandez, J. E., Rio, D., & Catedra-Perez, M. F. (1997). The matrix pencil method for two-dimensional direction of arrival estimation employing an L-shaped array. IEEE Transactions on Antennas and Propagation, 45(11), 1693–1694.

    Article  Google Scholar 

  • Gu, J. F., & Wei, P. (2007). Joint SVD of two cross-correlation matrices to achieve automatic pairing in 2-D angle estimation problems. IEEE Antennas and Wireless Propagation Letters, 6, 553–556.

    Article  Google Scholar 

  • He, J., & Liu, Z. (2009). Extended aperture 2-D direction finding with a two-parallel-shape-array using propagator method. IEEE Antennas and Wireless Propagation Letters, 8, 323–327.

    Article  Google Scholar 

  • He, Z., Zhao, Z., Nie, Z., Ma, P., & Liu, Q. H. (2012). Resolving manifold ambiguities for sparse array using planar substrates. IEEE Transactions on Antennas and Propagation, 60(5), 2558–2562.

    Article  MathSciNet  Google Scholar 

  • Hua, Y., Sarkar, T. K., & Weiner, D. D. (1991). An L-shaped array for estimating 2-D directions of wave arrival. IEEE Transactions on Antennas and Propagation, 39(2), 143–146.

    Article  Google Scholar 

  • Janssen, P. H. M., & Stoica, P. (1988). On the expectation of the product of four matrix-valued Gaussian random variables. IEEE Transactions on Automatic Control, 33(9), 867–870.

    Google Scholar 

  • Kikuchi, S., Tsuji, H., & Sano, A. (2006). Pair-matching method for estimating 2-D angle of arrival with a cross-correlation matrix. IEEE Antennas and Wireless Propagation Letters, 5(1), 35–40.

    Article  Google Scholar 

  • Li, J., Zhang, X., & Chen, H. (2012). Improved two-dimensional DOA estimation algorithm for two-parallel uniform linear arrays using propagator method. Signal Processing, 92(12), 3032–3038.

    Article  Google Scholar 

  • Liang, J., & Liu, D. (2010). Joint elevation and azimuth direction finding using L-shaped array. IEEE Transactions on Antennas and Propagation, 58(6), 2136–2141.

    Article  MathSciNet  Google Scholar 

  • Liu, T. H., & Mendel, J. M. (1998). Azimuth and elevation direction finding using arbitrary array geometries. IEEE Transactions on Signal Processing, 46(7), 2061–2065.

    Article  Google Scholar 

  • Liu, Z., Ruan, X., & He, J. (2013). Efficient 2-D DOA estimation for coherent sources with a sparse acoustic vector-sensor array. Multidimensional Systems and Signal Processing, 24(1), 105–120.

    Article  MATH  MathSciNet  Google Scholar 

  • Pal, P., & Vaidyanathan, P. P. (2010). Nested arrays: A novel approach to array processing with enhanced degrees of freedom. IEEE Transactions on Signal Processing, 58(8), 4167–4181.

    Article  MathSciNet  Google Scholar 

  • Rao, B. D., & Hari, K. V. S. (1989). Performance analysis of ESPRIT and TAM in determining the direction of arrival of plane waves in noise. IEEE Transactions on Acoustics, Speech, and Signal Processing, 37(12), 1990–1995.

    Article  Google Scholar 

  • Tayem, N., & Kwon, H. M. (2005). L-shape 2-dimensional arrival angle estimation with propagator method. IEEE Transactions on Antennas and Propagation, 53(5), 1622–1630.

    Article  Google Scholar 

  • Tayem, N., & Kwon, H. M. (2006). Azimuth and elevation angle estimation with no failure and no eigen-decomposition. Signal processing, 86(1), 8–16.

    Article  MATH  Google Scholar 

  • Wang, G., Xin, J., Zheng, N., & Sano, A. (2011). Computationally efficient subspace-based method for two-dimensional direction estimation with L-shaped array. IEEE Transactions on Signal Processing, 59(7), 3197–3212.

    Article  MathSciNet  Google Scholar 

  • Wu, Y., Liao, G., & So, H. C. (2003). A fast algorithm for 2-D direction-of-arrival estimation. Signal Processing, 83(8), 1827–1831.

    Article  MATH  Google Scholar 

  • Xia, T. Q., Yi, Z., Qun, W., & Wang, X. G. (2007). Decoupled estimation of 2-D angles of arrival using two parallel uniform linear arrays. IEEE Transactions on Antennas and Propagation, 55(9), 2627–2632.

    Article  Google Scholar 

  • Yuen, N., & Friedlander, B. (1996). Asymptotic performance analysis of ESPRIT, higher order ESPRIT, and virtual ESPRIT algorithms. IEEE Transactions on Signal Processing, 44(10), 2537–2550.

    Article  Google Scholar 

  • Zheng, Z., & Li, G. J. (2013). Fast DOA estimation of incoherently distributed sources by novel propagator. Multidimensional Systems and Signal Processing, 24(3), 573–581.

    Article  MATH  Google Scholar 

Download references

Acknowledgments

This work was supported by Natural Science Foundation of Jiangsu province (BK20131005), national natural science foundation of China (61302188), ministry funding (CASC04-02), (9140A07010713BQ02025) and (20113219110018).

Author information

Authors and Affiliations

  1. Department of Electronic Engineering, Nanjing University of Science and Technology, Nanjing , 210094, China

    Hua Shao, Weimin Su, Hong Gu & Jinyu Fan

  2. College of Electronic and Information Engineering, Nanjing University of Information Science and Technology, Nanjing , 210044, China

    Jinli Chen

Authors
  1. Hua Shao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Weimin Su
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Hong Gu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jinyu Fan
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Jinli Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Weimin Su.

Appendices

Appendix A

Substituting the first term in (41) into \(\hbox {E}\{|\bar{{\gamma }}_{w,k} |^{2}\}\) and with some simplifications, we have

$$\begin{aligned} \hbox {E}\left\{ {|\bar{{\gamma }}_{w,k}|^{2}} \right\}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \hbox {E}\{\Delta {\bar{\mathbf{c}}}_{z,m} \Delta {\bar{\mathbf{c}}}_{z,n}^\mathrm{H} \}{\bar{\mathbf{t}}}_{w,k}^*}} \nonumber \\&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \hbox {E}\{({\hat{{\bar{\mathbf{c}}}}}_{z,m} -{\bar{\mathbf{c}}}_{z,m})({\hat{{\bar{\mathbf{c}}}}}_{z,n} -{\bar{\mathbf{c}}}_{z,n})^{\mathrm{H}}\}{\bar{\mathbf{t}}}_{w,k}^*}}\nonumber \\&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \left( {\hbox {E}\{{\hat{{\bar{\mathbf{c}}}}}_{z,m} {\hat{{\bar{\mathbf{c}}}}}_{z,n}^\mathrm{H} \}-{\bar{\mathbf{c}}}_{z,m} {\bar{\mathbf{c}}}_{z,n}^\mathrm{H}}\right) {\bar{\mathbf{t}}}_{w,k}^*}} \nonumber \\&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \left( {\mathbf{E}_m \hbox {E}\{{\hat{{\bar{\mathbf{r}}}}}_z {\hat{{\bar{\mathbf{r}}}}}_z^\mathrm{H} \}\mathbf{E}_n^\mathrm{H} -{\bar{\mathbf{c}}}_{z,m} {\bar{\mathbf{c}}}_{z,n}^\mathrm{H} } \right) {\bar{\mathbf{t}}}_{w,k}^*}} \end{aligned}$$
(82)

By using the fact that \(\mathbf{z}_2^*(t)\otimes \mathbf{z}_1 (t)=(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1})\mathbf{z}_1 (t)=(\mathbf{I}_{N_2} \otimes \mathbf{z}_1 (t))\mathbf{z}_2^*(t), {\hat{{\bar{\mathbf{r}}}}}_z \) in (21) can be equivalently expressed as \({\hat{{\bar{\mathbf{r}}}}}_z =T^{-1}\sum \nolimits _{t=1}^T {(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1 } )\mathbf{z}_1 (t)} =T^{-1}\sum \nolimits _{t=1}^T (\mathbf{I}_{N_2 } \otimes \mathbf{z}_1 (t))\) \(\mathbf{z}_2^*(t)\). Thus, \(\hbox {E}\{{\hat{{\bar{\mathbf{r}}}}}_z {\hat{{\bar{\mathbf{r}}}}}_z^\mathrm{H}\}\) in (82) is given by

$$\begin{aligned} \hbox {E}\{{\hat{{\bar{\mathbf{r}}}}}_z {\hat{{\bar{\mathbf{r}}}}}_z^\mathrm{H}\}&= T^{-2}\sum \limits _{t=1}^T {\sum \limits _{l=1}^T {\hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1})\mathbf{z}_1 (t)\mathbf{z}_2^\mathrm{T} (l)(\mathbf{I}_{N_2 } \otimes \mathbf{z}_1 (l))^{\mathrm{H}}\}} } \nonumber \\&= T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1 } )\mathbf{z}_1 (t)\}\hbox {E}\{\mathbf{z}_2^\mathrm{T} (l)(\mathbf{I}_{N_2 } \otimes \mathbf{z}_1 (l))^{\mathrm{H}}\}\nonumber \\&+\,\,\hbox {E}\{\mathbf{z}_2^\mathrm{T} (l)\otimes (\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1} )\}\cdot \hbox {E}\{(\mathbf{I}_{N_2} \otimes \mathbf{z}_1 (l))^{\mathrm{H}}\otimes \mathbf{z}_1 (t)\}\nonumber \\&+\,\,\hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1 } )\hbox {E}\{\mathbf{z}_1 (t)\mathbf{z}_2^\mathrm{T} (l)\}(\mathbf{I}_{N_2} \otimes \mathbf{z}_1 (l))^{\mathrm{H}}\}\nonumber \\&= T^{-2}\sum \limits _{t=1}^T {\sum \limits _{l=1}^T {{\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{H} +(\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{I}_{N_1 })(\mathbf{I}_{N_2 } \otimes \mathbf{R}_{z,1} )\delta _{t,l} +0} }\nonumber \\&= {\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{H} +T^{-1}(\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{R}_{z,1}) \end{aligned}$$
(83)

where the formula for the expectation of the product of four complex Gaussian random matrices and vectors with zero-mean and compatible dimensions \(\hbox {E}\{\mathbf{Abc}^{\mathrm{T}}\mathbf{D}\}=\hbox {E}\{\mathbf{Ab}\}\hbox {E}\{\mathbf{c}^{\mathrm{T}}\mathbf{D}\}+\hbox {E}\{\mathbf{c}^{\mathrm{T}}\otimes \mathbf{A}\}\hbox {E}\{\mathbf{D}\otimes \mathbf{b}\}+ \hbox {E}\{\mathbf{A}\hbox {E}\{\mathbf{bc}^{\mathrm{T}}\}\mathbf{D}\}\) is used (Janssen and Stoica 1988). Substituting (83) into (82) and using the fact that \(\mathbf{E}_m \hbox {E}\{{\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{H} \}\mathbf{E}_n^\mathrm{H} ={\bar{\mathbf{c}}}_{z,m} {\bar{\mathbf{c}}}_{z,n}^\mathrm{H} \), we have

$$\begin{aligned} \hbox {E}\{|\bar{{\gamma }}_{w,k} |^{2}\}=T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \mathbf{E}_m (\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{R}_{z,1} )\mathbf{E}_n^\mathrm{H} {\bar{\mathbf{t}}}_{w,k}^*}} \end{aligned}$$
(84)

Similar to \(\hbox {E}\{|\bar{{\gamma }}_{w,k} |^{2}\}\) in (84), we can get

$$\begin{aligned} \hbox {E}\{\bar{{\gamma }}_{w,k} \tilde{\gamma }_{w,k}^*\}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T}}}\nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1})\mathbf{z}_1 (t)\mathbf{z}_1^\mathrm{T} (l)(\mathbf{z}_2^*(l)\otimes \mathbf{I}_{N_1})^{\mathrm{T}}\}\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -\,{\bar{\mathbf{c}}}_{z,m} \tilde{\mathbf{c}}_{z,n}^\mathrm{H}\right] \tilde{\mathbf{t}}_{w,k}^*\nonumber \\&= T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*{\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \mathbf{E}_m {\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{T}\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H} \tilde{\mathbf{t}}_{w,k}^*}}\end{aligned}$$
(85)
$$\begin{aligned} \hbox {E}\{\tilde{\gamma }_{w,k} \bar{{\gamma }}_{w,k}^*\}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*\tilde{\mathbf{t}}_{w,k}^\mathrm{T}}} \nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \mathbf{J}_{2N} \hbox {E}\{(\mathbf{z}_2 (t)\otimes \mathbf{I}_{N_1 } )\mathbf{z}_1^*(t)\mathbf{z}_1^\mathrm{H} (l)(\mathbf{z}_2 (l)\otimes \mathbf{I}_{N_1})^{\mathrm{T}}\}\mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -\, \tilde{\mathbf{c}}_{z,m} {\bar{\mathbf{c}}}_{z,n}^\mathrm{H}\right] {\bar{\mathbf{t}}}_{w,k}^*\nonumber \\&= T^{-1}\sum \limits _{m=1}^K \sum \limits _{n=1}^K v_{w,k,m} v_{w,k,n}^*\tilde{\mathbf{t}}_{w,k}^\mathrm{T} \mathbf{E}_m \mathbf{J}_{2N} \bar{\mathbf{r}}_z^*\bar{\mathbf{r}}_z^\mathrm{H} \mathbf{E}_n^\mathrm{H} \bar{\mathbf{t}}_{w,k}^*\end{aligned}$$
(86)
$$\begin{aligned} \hbox {E}\left\{ {|\tilde{\gamma }_{w,k}|^{2}}\right\}&= \sum \limits _{m=1}^K \sum \limits _{n=1}^K v_{w,k,m} v_{w,k,n}^*\tilde{\mathbf{t}}_{w,k}^\mathrm{T}\nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \mathbf{J}_{2N} \hbox {E}\{(\mathbf{z}_2 (t)\otimes \mathbf{I}_{N_1}) \mathbf{z}_1^*(t)\mathbf{z}_2^\mathrm{H} (l)(\mathbf{I}_{N_2} \otimes \mathbf{z}_1 (l))^{\mathrm{T}}\}\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -\,\tilde{\mathbf{c}}_{z,m} \tilde{\mathbf{c}}_{z,n}^\mathrm{H}\right] \tilde{\mathbf{t}}_{w,k}^*\nonumber \\&= T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n}^*\tilde{\mathbf{t}}_{w,k}^\mathrm{T} \mathbf{E}_m \mathbf{J}_{2N} (\mathbf{R}_{z,2} \otimes \mathbf{R}_{z,1}^\mathrm{T})\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H} \tilde{\mathbf{t}}_{w,k}^*}}\end{aligned}$$
(87)
$$\begin{aligned} \hbox {E}\{\bar{{\gamma }}_{w,k}^2 \}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} {\bar{\mathbf{t}}}_{w,k}^\mathrm{T}}} \nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1 } )\mathbf{z}_1 (t)\mathbf{z}_1^\mathrm{T} (l)(\mathbf{z}_2^*(l)\otimes \mathbf{I}_{N_1})^{\mathrm{T}}\}\mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -\,{\bar{\mathbf{c}}}_{z,m} {\bar{\mathbf{c}}}_{z,n}^\mathrm{T}\right] {\bar{\mathbf{t}}}_{w,k} \nonumber \\&= T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} { \bar{\mathbf{t}}}_{w,k}^\mathrm{T} \mathbf{E}_m {\bar{\mathbf{r}}}_z { \bar{\mathbf{r}}}_z^\mathrm{T} \mathbf{E}_n^\mathrm{T} {\bar{\mathbf{t}}}_{w,k} } }\end{aligned}$$
(88)
$$\begin{aligned} \hbox {E}\{\bar{{\gamma }}_{w,k} \tilde{\gamma }_{w,k}\}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} {\bar{\mathbf{t}}}_{w,k}^\mathrm{T}}} \nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \hbox {E}\{(\mathbf{z}_2^*(t)\otimes \mathbf{I}_{N_1})\mathbf{z}_1 (t)\mathbf{z}_2^\mathrm{T} (l)(\mathbf{I}_{N_2 } \otimes \mathbf{z}_1^*(l))^{\mathrm{T}}\}\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -{\bar{\mathbf{c}}}_{z,m} \tilde{\mathbf{c}}_{z,n}^\mathrm{T}\right] \tilde{\mathbf{t}}_{w,k} \nonumber \\&= T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} {\bar{\mathbf{t}}}_{w,k}^\mathrm{T} \mathbf{E}_m (\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{R}_{z,1})\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{T} \tilde{\mathbf{t}}_{w,k}}} \end{aligned}$$
(89)
$$\begin{aligned} \hbox {E}\{\tilde{\gamma }_{w,k}^2\}&= \sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} \tilde{\mathbf{t}}_{w,k}^\mathrm{T}}} \nonumber \\&\left[ T^{-2}\sum \limits _{t=1}^T \sum \limits _{l=1}^T \mathbf{E}_m \mathbf{J}_{2N} \hbox {E}\{(\mathbf{z}_2 (t)\otimes \mathbf{I}_{N_1 } )\mathbf{z}_1^*(t)\mathbf{z}_1^\mathrm{H} (l)(\mathbf{z}_2 (l)\otimes \mathbf{I}_{N_1})^{\mathrm{T}}\}\mathbf{J}_{2N} \mathbf{E}_n^\mathrm{H}\right. \nonumber \\&\quad \left. -\,\tilde{\mathbf{c}}_{z,m} \tilde{\mathbf{c}}_{z,n}^\mathrm{T}\right] \tilde{\mathbf{t}}_{w,k} \nonumber \\&= T^{-1}\sum \limits _{m=1}^K {\sum \limits _{n=1}^K {v_{w,k,m} v_{w,k,n} \tilde{\mathbf{t}}_{w,k}^\mathrm{T} \mathbf{E}_m \mathbf{J}_{2N} {\bar{\mathbf{r}}}_z^*{\bar{\mathbf{r}}}_z^\mathrm{H} \mathbf{J}_{2N} \mathbf{E}_n^\mathrm{T} \tilde{\mathbf{t}}_{w,k}}} \end{aligned}$$
(90)

Appendix B

Substituting (63) and (66) into the first term in (61), we can get

$$\begin{aligned}&{\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 (\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{R}_{z,1} )\mathbf{E}_1^\mathrm{H} {\bar{\mathbf{t}}}_w^*\nonumber \\&={\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 [(\mathbf{a}_2^*r_s \mathbf{a}_2^\mathrm{T})\otimes (\mathbf{a}_1 r_s \mathbf{a}_1^\mathrm{H} )+\sigma _n^2 \mathbf{I}_{N_2}\otimes (\mathbf{a}_1 r_s \mathbf{a}_1^\mathrm{H} )+\sigma _n^2 (\mathbf{a}_2^*r_s \mathbf{a}_2^\mathrm{T} )\otimes \mathbf{I}_{N_1} +\sigma _n^4 \mathbf{I}_{2N} ]\mathbf{E}_1^\mathrm{H} {\bar{\mathbf{t}}}_w^*\nonumber \\&={\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 [\sigma _n^2 r_s (\mathbf{I}_{N_2} \otimes (\mathbf{a}_1 \mathbf{a}_1^\mathrm{H})+(\mathbf{a}_2^*\mathbf{a}_2^\mathrm{T})\otimes \mathbf{I}_{N_1 })+\sigma _n^4 \mathbf{I}_{2N}]\mathbf{E}_1^\mathrm{H} {\bar{\mathbf{t}}}_w^*\nonumber \\&=(2\sigma _n^2 r_s +\sigma _n^4 ){\bar{\mathbf{t}}}_w^\mathrm{T} \left[ {\begin{array}{lll} 1&{} \ldots &{} 0 \\ \vdots &{} \ddots &{} \vdots \\ 0&{} \ldots &{} 1 \\ \end{array}}\right] {\bar{\mathbf{t}}}_w^*\nonumber \\&={(2\sigma _n^2 r_s +\sigma _n^4 )}/{(2N^{2})} \end{aligned}$$
(91)

where \({\bar{\mathbf{t}}}_u^\mathrm{T} \mathbf{E}_1 (\mathbf{a}_2^*\otimes \mathbf{a}_1 )={\bar{\mathbf{t}}}_u^\mathrm{T} {\bar{\mathbf{a}}}_1 =0\) is used, and ‘...’ denotes the parts we do not concern. Similar to (91) and using (63), (64) and (66), other terms in (61) can be directly calculated as

$$\begin{aligned} {\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 {\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{T} \mathbf{J}_{2N} \mathbf{E}_1^\mathrm{H} \tilde{\mathbf{t}}_w^*&= \tilde{\mathbf{t}}_w^\mathrm{T} \mathbf{E}_1 \mathbf{J}_{2N} {\bar{\mathbf{r}}}_z^*{\bar{\mathbf{r}}}_z^\mathrm{H} \mathbf{E}_1^\mathrm{H} {\bar{\mathbf{t}}}_w^*=0\end{aligned}$$
(92)
$$\begin{aligned} \tilde{\mathbf{t}}_w^\mathrm{T} \mathbf{E}_1 \mathbf{J}_{2N} (\mathbf{R}_{z,2} \otimes \mathbf{R}_{z,1}^\mathrm{T} )\mathbf{J}_{2N} \mathbf{E}_1^\mathrm{H} \tilde{\mathbf{t}}_w^*&= \tilde{\mathbf{t}}_w^\mathrm{T} \mathbf{E}_1 \mathbf{J}_{2N} [\sigma _n^2 r_s (\mathbf{I}_{N_2 } \otimes (\mathbf{a}_1^*\mathbf{a}_1^\mathrm{T} )+(\mathbf{a}_2 \mathbf{a}_2^\mathrm{H} )\otimes \mathbf{I}_{N_1})\nonumber \\&\quad +\,\sigma _n^4 \mathbf{I}_{2N} ]\mathbf{J}_{2N} \mathbf{E}_1^\mathrm{H} \tilde{\mathbf{t}}_w^*\nonumber \\&= {(2\sigma _n^2 r_s +\sigma _n^4 )}/{(2N^{2})}\end{aligned}$$
(93)
$$\begin{aligned} {\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 {\bar{\mathbf{r}}}_z {\bar{\mathbf{r}}}_z^\mathrm{T} \mathbf{E}_1^\mathrm{T} {\bar{\mathbf{t}}}_w&= \tilde{\mathbf{t}}_w^\mathrm{T} \mathbf{E}_1 \mathbf{J}_{2N} {\bar{\mathbf{r}}}_z^*{\bar{\mathbf{r}}}_z^\mathrm{H} \mathbf{J}_{2N} \mathbf{E}_1^\mathrm{T} \tilde{\mathbf{t}}_w =0\end{aligned}$$
(94)
$$\begin{aligned} 2{\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 (\mathbf{R}_{z,2}^\mathrm{T} \otimes \mathbf{R}_{z,1} )\mathbf{J}_{2N} \mathbf{E}_1^\mathrm{T} \tilde{\mathbf{t}}_w&= 2{\bar{\mathbf{t}}}_w^\mathrm{T} \mathbf{E}_1 [\sigma _n^2 r_s (\mathbf{I}_{N_2 } \otimes (\mathbf{a}_1 \mathbf{a}_1^\mathrm{H} )+(\mathbf{a}_2^*\mathbf{a}_2^\mathrm{T} )\otimes \mathbf{I}_{N_1})\nonumber \\&\quad +\,\sigma _n^4 \mathbf{I}_{2N} ]\mathbf{J}_{2N} \mathbf{E}_1^\mathrm{T} \tilde{\mathbf{t}}_w \nonumber \\&= {(2\sigma _n^2 r_s +\sigma _n^4 )}/{(2N^{2})} \end{aligned}$$
(95)

Substituting \(v_w\) of (65) and (91)–(95) into (61), we have

$$\begin{aligned} \hbox {var}_{\mathrm{VMPM}} ({\hat{\theta }})=\frac{\lambda ^{2}}{8T(\pi \Delta _1 \sin \theta )^{2}}\frac{2\sigma _n^2 r_s +\sigma _n^4 }{2N^{2}r_s^2}=\frac{\lambda ^{2}(2\hbox {SNR}+1)}{16T(\pi N\Delta _1 \sin \theta \hbox {SNR})^{2}} \end{aligned}$$
(96)

If \(P=2N_1 +2N_2 -1\) and \(N_1 =N_2 +1\), we have \(N={(P+3)(P-1)}/{32}\). Substituting \(N\) in (96) and after some simplifications, we can get the result of (67).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Shao, H., Su, W., Gu, H. et al. Virtual matrix pencil method for 2-D DOA estimation with a two-nested-shape-array. Multidim Syst Sign Process 26, 619–644 (2015). https://doi.org/10.1007/s11045-013-0274-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11045-013-0274-z

Keywords

Search

Navigation