Construction of a quantum Carnot heat engine cycle

Abstract

The microscopic state description of an irreversible quantum Carnot cycle for a general quantum working medium is investigated. An efficiency lag term, which quantifies the deviation of the irreversible cycle efficiency from the classical Carnot efficiency, is given in terms of the total entropy increase in the universe. The efficiency lag and the total entropy increase in the universe are directly connected to the quantum relative entropy between the density matrices obtained at the end of the quantum adiabatic and the relaxation steps of the cycle. The total entropy increase and the efficiency lag are found to be always nonnegative quantities. Our results give a direct proof that the irreversible cycle efficiency is always smaller than the classical Carnot efficiency. Two interacting spins under an external magnetic field are proposed as the working medium of the irreversible quantum Carnot cycle. The external magnetic field is considered to be quasistatically changed during the steps of the cycle. The coupling between the spins is found to break down the scale invariance and make the quantum Carnot cycle irreversible. It is shown that while the quantum coupling can lower the cycle efficiency monotonically to zero, it can make the irreversible cycle to produce more work than the one obtained from the uncoupled spins. The conditions in which one can always construct a reversible Carnot cycle for the coupled spin working medium are also given.

Appendix

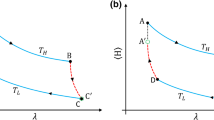
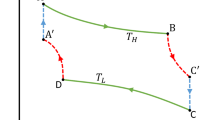
Here, we explicitly derive the equivalence in Eq. (6). Using Boltzmann–Gibbs distribution $P_n(i)=1/{Z(i)}\exp \{-\beta _i E_n(i)\}$, the thermodynamic entropy can be written into a useful form as

$$\begin{aligned} S(i)= & {} -k_B \sum _n P_n(i) \ln {P_n(i)} \nonumber \\= & {} k_B \beta _i \sum _n P_n(i) E_n(i) + k_B \sum _n P_n(i) \ln {Z(i)}\nonumber \\= & {} k_B \beta _i \left\langle H(i)\right\rangle + k_B \ln {Z(i)}, \end{aligned}$$

(10)

where $\beta _{A}=\beta _{B}=(k_B T_H)^{-1}\equiv \beta _{H}$ and $\beta _{C}=\beta _{D}=(k_B T_L)^{-1}\equiv \beta _{L}$. Using Eq. (10), the total entropy increase in the universe in Eq. (3) can be rewritten as

$$\begin{aligned} \varDelta S_{C^{\prime } \rightarrow C}^{\mathrm{total}}= & {} k_B \left[ \ln {Z(C)} - \ln {Z(B)} + \beta _L \left\langle H(C^{\prime })\right\rangle - \beta _H \left\langle H(B)\right\rangle \right] , \nonumber \\ \varDelta S_{A^{\prime } \rightarrow A}^{\mathrm{total}}= & {} k_B \left[ \ln {Z(A)} - \ln {Z(D)} + \beta _H \left\langle H(A^{\prime })\right\rangle - \beta _L \left\langle H(D)\right\rangle \right] . \end{aligned}$$

(11)

We are now in a position to show that Eq. (11) can also be given by the quantum relative entropy between the density matrices obtained at the end of the adiabatic and the relaxation processes of the cycle. First, consider the quantum relative entropy for the cycle process $B \rightarrow C^{\prime } \rightarrow C$,

$$\begin{aligned} S(\rho _{C^{\prime }} || \rho _C)= & {} tr(\rho _{C^{\prime }} \ln {\rho _{C^{\prime }}}) - tr(\rho _{C^{\prime }} \ln {\rho _C)}. \end{aligned}$$

(12)

The first term on the right-hand side is simply the von Neumann entropy at instant $C^{\prime }$ which is invariant under a unitary transformation. Therefore, using Eq. (10) it can be written as

$$\begin{aligned} tr(\rho _{C^{\prime }} \ln {\rho _{C^{\prime }}})= & {} -S(B)/k_B \nonumber \\= & {} -\beta _H \left\langle H(B)\right\rangle - \ln {Z(B)}. \end{aligned}$$

(13)

Using the spectral resolution of the Hamiltonian $H(i)=\sum _n E_n(i) \left| n(i)\right\rangle \left\langle n(i)\right|$ and the density matrix $\rho _i=\sum _n P_n(i) \left| n(i)\right\rangle \left\langle n(i)\right|$, the second term on the right-hand side of Eq. (12) can be expanded as,

$$\begin{aligned} tr(\rho _{C^{\prime }} \ln {\rho _C})= & {} tr\left( \rho _{C^{\prime }} \sum _n \ln {P_n(C)} \left| n(C)\right\rangle \left\langle n(C)\right| \right) \nonumber \\= & {} \sum _n \ln {P_n(C)} \left\langle n(C)\right| \rho _{C^{\prime }} \left| n(C)\right\rangle \nonumber \\= & {} \sum _n \left[ -\beta _L E_n(C) - \ln {Z(C)}\right] \left\langle n(C)\right| \rho _{C^{\prime }} \left| n(C)\right\rangle \nonumber \\= & {} -\beta _L \left\langle H(C^{\prime })\right\rangle -\ln {Z(C)}. \end{aligned}$$

(14)

Therefore, we get the following result

$$\begin{aligned} S(\rho _{C^{\prime }} || \rho _C) =\ln {Z(C)} - \ln {Z(B)} + \beta _L \left\langle H(C^{\prime })\right\rangle - \beta _H \left\langle H(B)\right\rangle . \end{aligned}$$

(15)

A similar calculation procedure can be applied for the relative entropy $S(\rho _{A^{\prime }} || \rho _A)$. The result is

$$\begin{aligned} S(\rho _{A^{\prime }} || \rho _A) = \ln {Z(A)} - \ln {Z(D)} + \beta _H \left\langle H(A^{\prime })\right\rangle - \beta _L \left\langle H(D)\right\rangle . \end{aligned}$$

(16)

From the comparison of Eq. (11) with Eqs. (15) and (16), we immediately conclude the following results

$$\begin{aligned} \varDelta S_{C^{\prime } \rightarrow C}^{\mathrm{total}}= & {} k_B S(\rho _{C^{\prime }} || \rho _C), \nonumber \\ \varDelta S_{A^{\prime } \rightarrow A}^{\mathrm{total}}= & {} k_B S(\rho _{A^{\prime }} || \rho _A). \end{aligned}$$

(17)

With respect to the Klein’s inequality [68], $\varDelta S_{C^{\prime } \rightarrow C}^{\mathrm{total}}$ and $\varDelta S_{A^{\prime } \rightarrow A}^{\mathrm{total}}$ are always nonnegative quantities.