Time-varying tandem queues with blocking: modeling, analysis, and operational insights via fluid models with reflection

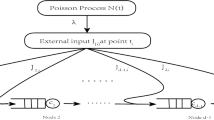
Abstract

In this paper, we develop time-varying fluid models for tandem networks with blocking. Beyond having their own intrinsic value, these mathematical models are also limits of corresponding many-server stochastic systems. We begin by analyzing a two-station tandem network with a general time-varying arrival rate, a finite waiting room before the first station, and no waiting room between the stations. In this model, customers that are referred from the first station to the second when the latter is saturated (blocked) are forced to wait in the first station while occupying a server there. The finite waiting room before the first station causes customer loss and, therefore, requires reflection analysis. We then specialize our model to a single station (many-server fluid limit of the $G_t/M/N/(N +H)$ queue), generalize it to k stations in tandem, and allow finite internal waiting rooms. Our models yield operational insights into network performance, specifically on the effects of line length, bottleneck location, waiting room size, and the interaction among these effects.

Appendices

Appendix A: Proof of Theorem 1

Let T be an arbitrary positive constant. Using the Lipschitz property (Appendix C) and subtracting the equation for r in (10) from the equation for $r^{\eta }$ in (9) yields that

$$\begin{aligned}&\left||r_1^{\eta } - r_1\right||_T \vee \left||r_2^{\eta } - r_2\right||_T \le G\Bigg [ \left| r_1^{\eta }(0) - r_1(0)\right| + \Bigg \vert \Bigg \vert \int _0^{\cdot }{\lambda (u)\,\mathrm {d}u} - \eta ^{-1}A^{\eta }(\cdot )\Bigg \vert \Bigg \vert _T\nonumber \\&+ \Bigg \vert \Bigg \vert \eta ^{-1}D_1\left( \eta p\mu _1\int _0^{\cdot }{\left[ \Big (N_1 + H - r_1^{\eta }(u)\Big )\wedge \Big (N_1-b^{\eta }(u)\Big )\right] }\,\mathrm {d}u\right) \nonumber \\&- p\mu _1\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right] \,\mathrm {d}u}\Bigg \vert \Bigg \vert _T \nonumber \\&+ \Bigg \vert \Bigg \vert \eta ^{-1}D_3\left( \eta (1-p)\mu _1\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right] }\,\mathrm {d}u\right) \nonumber \\&- (1-p)\mu _1\int _0^{\cdot }{\left[ \Big (N_1 + H - r_1^{\eta }(u)\Big )\wedge \left( N_1-b^{\eta }(u)\right) \right] \,\mathrm {d}u}\Bigg \vert \Bigg \vert _T\nonumber \\&+ \Bigg \vert \Bigg \vert \mu _1 \int _0^{\cdot }\left[ \Big (N_1 + H - r_1^{\eta }(u)\Big )\wedge \left( N_1-b^{\eta }(u)\right) \right. \nonumber \\&\left. - \left( N_1 + H - r_1(u)\right) \wedge \Big (N_1-b(u)\Big )\right] \,\mathrm {d}u\Bigg \vert \Bigg \vert _T\Bigg ] \,\vee \nonumber \\&G \Bigg [\left| r_2^{\eta }(0) - r_2(0)\right| + \left|| \int _0^{\cdot }{\lambda (u)\,\mathrm {d}u} - \eta ^{-1}A^{\eta }(\cdot )\right||_T \nonumber \\&+ \Bigg \vert \Bigg \vert \eta ^{-1}D_3\Bigg (\eta (1-p)\mu _1\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right] }\,\mathrm {d}u\Bigg ) \nonumber \\&- (1-p)\mu _1\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right] \,\mathrm {d}u}\Bigg \vert \Bigg \vert _T \nonumber \\&+ \Bigg \vert \Bigg \vert \eta ^{-1}D_2\left( \eta \mu _2\int _0^{\cdot }{\left[ N_2 \wedge (r_1^{\eta }(u) - r_2^{\eta }(u) + N_2)\right] }\,\mathrm {d}u\right) \nonumber \\&- \mu _2\int _0^{\cdot }{\left[ N_2 \wedge (r_1^{\eta }(u) - r_2^{\eta }(u) + N_2)\right] \, \mathrm {d}u}\Bigg \vert \Bigg \vert _T\nonumber \\&+ \Bigg \vert \Bigg \vert (1-p)\mu _1 \int _0^{\cdot }\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right. \nonumber \\&\left. -\left( N_1 + H - r_1(u)\right) \wedge \left( N_1-b(u)\right) \right] \,\mathrm {d}u\Bigg \vert \Bigg \vert _T \nonumber \\&+ \Bigg \vert \Bigg \vert \mu _2\int _0^{\cdot }\left[ \left( N_2 \wedge \big (r_1^{\eta }(u) - r_2^{\eta }(u) + N_2\big )\right) \right. \nonumber \\&\left. - \left( N_2 \wedge (r_1(u) - r_2(u)+N_2)\right) \right] \,\mathrm {d}u \Bigg \vert \Bigg \vert _T\Bigg ], \end{aligned}$$

(20)

where G is the Lipschitz constant.

The first, second, sixth, and seventh terms on the right-hand side converge to zero by the conditions of the theorem. For proving convergence to zero of the third, fourth, eighth, and ninth terms, we use Lemma 1 in Appendix D. By the FSLLN for Poisson processes,

$$\begin{aligned} \sup _{0 \le u \le t}\left| \eta ^{-1}D(\eta u)- u\right| \rightarrow 0, \quad \forall t \ge 0 \quad a.s. \end{aligned}$$

Note that the functions $p\mu _1\int _0^{t}{\left[ \left( N_1+H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) \right] }\,\mathrm {d}u$ and $\mu _2\int _0^{t}{\left[ N_2 \wedge \Big (r_1^{\eta }(u) - r_2^{\eta }(u) + N_2\Big )\right] \,\mathrm {d}u}$ are bounded by $p\mu _1 \cdot (N_1 + H) \cdot T$ and $\mu _2 \cdot N_2 \cdot T$, respectively, for $0 \le p \le 1$ and $t \in [0, T]$. This, together with Lemma 1, implies that the third, fourth, eighth, and ninth terms in (20) converge to 0.

We get that

$$\begin{aligned}&\left||r_1^{\eta } - r_1\right||_T \vee \left||r_2^{\eta } - r_2\right||_T \nonumber \\&\le \left[ \epsilon _1^{\eta }(T) + G\mu _1 \left||\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) - \left( N_1 + H - r_1(u)\right) \wedge \left( N_1-b(u)\right) \right] }\,\mathrm {d}u\right||_T \right] \, \vee \nonumber \\&\Bigg [\epsilon _2^{\eta }(T) + G (1-p)\mu _1 \left||\int _0^{\cdot }{\left[ \left( N_1 + H - r_1^{\eta }(u)\right) \wedge \left( N_1-b^{\eta }(u)\right) - \left( N_1 + H - r_1(u)\right) \wedge \left( N_1-b(u)\right) \right] }\,\mathrm {d}u\right||_T \nonumber \\&\ \ \ +G\mu _2\left||\int _0^{\cdot }{{\left[ N_2 \wedge (r_1^{\eta }(u) - r_2^{\eta }(u) + N_2)\right] - \left[ N_2 \wedge (r_1(u) - r_2(u) + N_2)\right] \,\mathrm {d}u}}\right||_T \Bigg ] \nonumber \\ \le&\left[ \epsilon _1^{\eta }(T) +G\mu _1 \left||\int _0^{\cdot }{\left[ r_1^{\eta }(u) - r_1(u) \right] }\,\mathrm {d}u\right||_T +G\mu _1 \left||\int _0^{\cdot }{\left[ b^{\eta }(u) - b(u)\right] }\,\mathrm {d}u\right||_T\right] \, \vee \nonumber \\&\quad \Bigg [\epsilon _2^{\eta }(T)+ G(1-p)\mu _1 \left||\int _0^{\cdot }{\left[ r_1^{\eta }(u) - r_1(u) \right] }\,\mathrm {d}u\right||_T \nonumber \\&\quad +G(1-p)\mu _1 \left||\int _0^{\cdot }{\left[ b^{\eta }(u) - b(u)\right] }\,\mathrm {d}u\right||_T \nonumber \\&\ \ \ + G\mu _2\left||\int _0^{\cdot }{{\left[ r_1^{\eta }(u) - r_1(u) \right] \,\mathrm {d}u}}\right||_T + G\mu _2 \left||\int _0^{\cdot }{{\left[ r_2^{\eta }(u) - r_2(u)\right] \,\mathrm {d}u}}\right||_T\Bigg ] \nonumber \\ \le&\left[ \epsilon _1^{\eta }(T) + G\mu _1 \int _0^{T}{\left||r_1^{\eta } - r_1\right|| _u}\,\mathrm {d}u +G\mu _1 \int _0^{T}{\left||b^{\eta } - b\right||_u\,\mathrm {d}u}\right] \, \vee \nonumber \\&\Bigg [\epsilon _2^{\eta }(T)+G\mu _1 \int _0^{T}{\left||r_1^{\eta } - r_1 \right||_u\,\mathrm {d}u} +G\mu _1 \int _0^{T}{\left||b^{\eta } - b\right||_u\,\mathrm {d}u} \nonumber \\&\ \ \ + G \mu _2\int _0^{T}{{\left||r_1^{\eta } - r_1\right||_u\,\mathrm {d}u}} + G\mu _2 \int _0^{T}{{\left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}}\Bigg ], \end{aligned}$$

(21)

where $\epsilon _1^{\eta }(T)$ bounds the sum of the first four terms on the right-hand side of (20), and $\epsilon _2^{\eta }(T)$ bounds the sum of the sixth to ninth terms; these two quantities $\epsilon _1^{\eta }(T)$ and $\epsilon _2^{\eta }(T)$ converge to zero, as $\eta \rightarrow \infty$. The second inequality in (21) is obtained by using the inequalities $| a \wedge b - a \wedge c | \le | b-c |$ and $| a \wedge b - c \wedge d | \le | a-c | + |b-d|$ for any a, b, c, and d. The third equality in (21) is because $0\le p \le 1$.

We now use

$$\begin{aligned} \int _0^{T}{\left||b^{\eta } - b\right||_u\,\mathrm {d}u}&= \int _0^{T}{\left||\left( r_1^{\eta } - r_2^{\eta }\right) ^+ - \left( r_1 - r_2\right) ^+\right||_u\,\mathrm {d}u} \nonumber \\&= \int _0^{T}{\left||r_1^{\eta } - r_1^{\eta } \wedge r_2^{\eta } - r_1 + r_1 \wedge r_2\right||_u\,\mathrm {d}u} \nonumber \\&\le \int _0^{T}{\Big [\left||r_1^{\eta } - r_1\right||_u +\left||r_1^{\eta } \wedge r_2^{\eta } - r_1 \wedge r_2\right||_u\Big ]\,\mathrm {d}u} \nonumber \\&\le \int _0^{T}{\Big [2\left||r_1^{\eta } - r_1\right||_u +\left||r_2^{\eta } - r_2\right||_u\Big ]\,\mathrm {d}u}\nonumber \\&= 2\int _0^{T}{\left||r_1^{\eta } - r_1\right||_u\,\mathrm {d}u} + \int _0^{T}{\left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}. \end{aligned}$$

(22)

From (21) and (22), we get that

$$\begin{aligned}&\left||r_1^{\eta } - r_1\right||_T \vee \left||r_2^{\eta } - r_2\right||_T\nonumber \\&\le \left[ \epsilon _1^{\eta }(T) \vee \epsilon _2^{\eta }(T)\right] + G\left( 3 \mu _1 + \mu _2\right) \int _0^{T}{\left||r_1^{\eta } - r_1\right||_u\,\mathrm {d}u} + G\left( \mu _1 \vee \mu _2\right) \int _0^{T}{\left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}\nonumber \\&\le \left[ \epsilon _1^{\eta }(T) \vee \epsilon _2^{\eta }(T)\right] + 2G\left( 3\mu _1 \vee \mu _2\right) \left[ \int _0^{T}{\left||r_1^{\eta } - r_1\right||_u\,\mathrm {d}u} + \int _0^{T}{\left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}\right] \nonumber \\&\le \left[ \epsilon _1^{\eta }(T) \vee \epsilon _2^{\eta }(T)\right] + 4G\left( 3\mu _1 \vee \mu _2\right) \left[ \int _0^{T}{\left||r_1^{\eta } - r_1\right||_u\,\mathrm {d}u} \vee \int _0^{T}{\left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}\right] \nonumber \\&\le \left[ \epsilon _1^{\eta }(T) \vee \epsilon _2^{\eta }(T)\right] + 4G\left( 3\mu _1 \vee \mu _2\right) \left[ \int _0^{T}{\left||r_1^{\eta } - r_1\right||_u \vee \left||r_2^{\eta } - r_2\right||_u\,\mathrm {d}u}\right] . \end{aligned}$$

(23)

The first equality in (23) is obtained by using the inequality $(a+b) \vee (c+d) \le a \vee c + b \vee d$, for any a, b, c, and d. Applying Gronwall’s inequality [22] to (23) completes the proof for both the existence and uniqueness of r.

Appendix B: Proof of Proposition 1

We begin by proving that the solution for (11) satisfies, for $t \ge 0$,

$$\begin{aligned} l(t)&= \int _0^t{1_{\left\{ x_1(u) \ge \, N_1+H\right\} }\cdot 1_{\left\{ x_1(u)+ x_2(u)< N_1 + N_2+H\right\} }\left[ \lambda (u) - l_1(u)\right] ^{+} \,\mathrm {d}u} \, \nonumber \\&\quad + \int _0^t{1_{\left\{ x_1(u) < \, N_1+H\right\} }\cdot 1_{\left\{ x_1(u)+ x_2(u) \ge N_1 + N_2+H\right\} }\left[ \lambda (u) - l_2(u)\right] ^{+} \,\mathrm {d}u}\nonumber \\&\quad + \int _0^t{1_{\big \{x_1(u) \ge \, N_1+H\big \}}\cdot 1_{\big \{x_1(u)+ x_2(u) \ge N_1 + N_2 + H\big \}}} \Big [\lambda (u) - l_1(u) \wedge l_2(u) \Big ]^{+} \,\mathrm {d}u, \end{aligned}$$

(24)

where

$$\begin{aligned}&l_1(u) = \mu _{1} \left( x_{1}(u) \wedge \left( N_{1} - b(u)\right) \right) ;\\&l_{2}(u) = \mu _2 \left( x_2(u) \wedge N_2\right) + (1-p)\mu _{1} \left( x_{1}(u) \wedge \left( N_{1} - b(u)\right) \right) . \end{aligned}$$

In order to prove this, we substitute (24) in (11) and show that the properties in (11) prevail. We begin by substituting (24) in the first line of (11). Using $(a-b)^+=[a-a\wedge b]$, for any a, b, we obtain

$$\begin{aligned} x_1(t)&= x_1(0) + \int _0^t{\left[ \lambda (u)- \mu _1 \left[ x_1(u) \wedge \left( N_1-b(u)\right) \right] \right] \,\mathrm {d}u}\\&\quad - \int _0^t{1_{\left\{ x_1(u) \ge N_1 + H\right\} }\cdot 1_{\left\{ x_1(u) + x_2(u)< N_1 + N_2 + H\right\} }\left[ \lambda (u) - \lambda (u) \wedge l_1(u)\right] \,\mathrm {d}u} \\&\quad - \int _0^t{1_{\left\{ x_1(u) < \, N_1+ H\right\} }\cdot 1_{\left\{ x_1(u)+ x_2(u) \ge N_1 + N_2 + H\right\} }\left[ \lambda (u) - \lambda (u) \wedge l_2(u)\right] \,\mathrm {d}u}\\&\quad - \int _0^t{1_{\left\{ x_1(u) \ge \, N_1 + H\right\} }\cdot 1_{\left\{ x_1(u)+ x_2(u) \ge N_1 + N_2 + H\right\} }} \left[ \lambda (u) -\lambda (u) \wedge l_1(u) \wedge l_2(u)\right] \,\mathrm {d}u, \end{aligned}$$

and therefore,

$$\begin{aligned} x_1(t)&= x_1(0) + \int _0^t\Big [1_{\left\{ x_1(u)< \, N_1+ H\right\} } \cdot 1_{\left\{ x_1(u) + x_2(u)< \, N_1 + N_2 + H\right\} } \cdot \lambda (u)\nonumber \\&\quad - \mu _1 \left[ x_1(u) \wedge \left( N_1-b(u)\right) \right] \Big ]\,\mathrm {d}u \nonumber \\&\quad + \int _0^t{\left[ 1_{\left\{ x_{1}(u) \ge N_{1} + H\right\} } \cdot 1_{\left\{ x_1(u) + x_{2}(u)< N_1 + N_{2}+ H \right\} } \cdot \left( \lambda (u) \wedge l_1(u) \right) \right] \,\mathrm {d}u}\nonumber \\&\quad + \int _0^t{\left[ 1_{\left\{ x_{1}(u) < N_{1}+H\right\} } \cdot 1_{\left\{ x_1(u) + x_{2}(u) \ge N_1 + N_2 + H \right\} } \cdot \left( \lambda (u) \wedge l_2(u)\right) \right] \,\mathrm {d}u}\nonumber \\&\quad + \int _0^t\left[ 1_{\left\{ x_{1}(u) \ge N_{1}+H\right\} } \cdot 1_{\left\{ x_1(u) + x_{2}(u) \ge N_1 + N_2+H \right\} } \cdot \left( \lambda (u) \wedge l_1(u) \wedge l_2(u)\right) \right] \,\mathrm {d}u;\nonumber \\ x_2(t)&= x_2(0) + \int _0^t{\left[ p \mu _1 \left[ x_1(u) \wedge \left( N_1-b(u)\right) \right] - \mu _2 \left( x_2(u) \wedge N_2 \right) \right] \,\mathrm {d}u}. \end{aligned}$$

(25)

Clearly, the properties in the third and fourth lines in (11) prevail. It is left to verify that the first and second conditions prevail. This is done by the following proposition.

Proposition 2

The functions $x_1(\cdot )$ and $x_1(\cdot ) + x_2(\cdot )$ as in (25) are bounded by $N_1 + H$ and $N_1+N_2 + H$, respectively.

Proof

First, we prove that the function $x_1(\cdot )$, as in (25), is bounded by $N_1+H$. Assume that, for some t, $x_1(t) > N_1+H$. Since $x_1(0) \le N_1+H$ and $x_1$ is continuous (being an integral), there must be a last ${\tilde{t}}$ in [0, t], such that $x_1({\tilde{t}}) = N_1+H$ and $x_1(u) > N_1+H$, for $u \in [{\tilde{t}}, t]$. Without loss of generality, assume that ${\tilde{t}}=0$; thus $x_1(0) = N_1+H$ and $x_1(u) > N_1+H$ for $u \in (0, t]$. From (25), we get that

$$\begin{aligned} x_1(t)&= N_1+H +\int _0^t{\left[ 1_{\left\{ x_{1}(u) + x_2(u) < N_1 + N_2+H\right\} }\cdot \left( \lambda (u) \wedge l_1(u) \right) \right] \,\mathrm {d}u}\, \\&\quad + \int _0^t{\left[ 1_{\left\{ x_{1}(u) + x_{2}(u) \ge N_1 + N_2+H\right\} }\cdot \left( \lambda (u) \wedge l_1(u) \wedge l_2(u)\right) \right] \,\mathrm {d}u}\\&\quad - \mu _1 \int _0^t{\left[ x_1(u) \wedge \left( N_1-b(u)\right) \right] \,\mathrm {d}u}\\&\le N_1 +H+ \int _0^t{\left[ l_1(u) - \mu _1 \left[ x_1(u) \wedge \left( N_1-b(u)\right) \right] \right] \,\mathrm {d}u}=N_1+H, \end{aligned}$$

which contradicts our assumption and proves that $x_1(\cdot )$ cannot exceed $H_1+N_1$.

What is left to prove now is that the function $x_1(\cdot ) + x_2(\cdot )$ is bounded by $N_1+N_2$. Without loss of generality, assume that $x_1(0) + x_2(0) = N_1 + N_2+H$ and $x_1(u) + x_2(u) > N_1 + N_2+H$ for $u \in (0, t]$. This assumption, together with $x_1 \le N_1+H$, yields that $x_2 > N_2$; hence, from (25), we get that

$$\begin{aligned}&x_1(t) +x_2(t)\\&\quad = N_1 + N_2 + H \int _0^t{\left[ 1_{\left\{ x_{1}(u) \ge N_{1}+N_1\right\} } \cdot \left( \lambda (u) \wedge l_1(u) \wedge l_2(u)\right) \right] \,\mathrm {d}u}\\&\qquad + \int _0^t{\left[ 1_{\left\{ x_{1}(u) < N_{1}+H\right\} } \cdot \left( \lambda (u) \wedge l_2(u)\right) \right] \,\mathrm {d}u}\\&\qquad - \int _0^t{\left[ (1-p)\mu _1 \left( x_1(u) \wedge \left( N_1-b(u)\right) \right) + \mu _2 \left( x_2(u) \wedge N_2 \right) \right] \,\mathrm {d}u}\\&\quad \le N_1 + N_2 + H +\int _0^t\left[ l_2(u) - (1-p)\mu _1 \left( x_1(u)\wedge \left( N_1-b(u)\right) \right) \right. \\&\qquad \left. - \,\mu _2 \left( x_2(u) \wedge N_2 \right) \right] \,\mathrm {d}u\\&\quad = N_1+N_2+H, \end{aligned}$$

which contradicts the assumption that $x_1(t) + x_2(t) > N_1 + N_2 + H$ and proves that $x_1(\cdot )+x_2(\cdot )$ is bounded by $N_1+N_2+H$. $\square$

By the solution uniqueness (Proposition 3), we have established that x, the fluid limit for the stochastic queueing family $X^{\eta }$ in (2), is given by (25).

The following two remarks explain why (25) is equivalent to (12):

• 1. After proving that $x_1(\cdot ) \le N_1 + H$ and $x_1(\cdot ) + x_2(\cdot ) \le N_1 + N_2 + H$ in Proposition 2, the indicators in (24) can accommodate only the cases when $x_1(\cdot ) = N_1 + H$ and $x_1(\cdot ) + x_2(\cdot ) = N_1 + N_2+ H$.

• 2. When $x_1(u)=N_1+H$ and $x_1(u)+x_2(u) < N_1+N_2+H$, $x_2(u) < N_2$, and hence $b(u)=0$ and $l_1(u) = l_1^*(u)$. Alternatively, when $x_1(u)< N_1+H$ and $x_1(u)+x_2(u) = N_1+N_2+H$, $x_2(u) > N_2$, and therefore $l_2(u)=l_2^*(u)$.

Appendix C: Uniqueness and Lipschitz property

Let $C \equiv C[0,\infty ]$. We now define mappings $\psi : C^2 \rightarrow C$ and $\phi : C^2 \rightarrow C^2$ for $m \in C^2$ by setting

$$\begin{aligned} \psi (m)(t)&= \sup _{0 \le s \le t} \left( -\Big (m_1(s) \wedge m_2(s)\right) \Big )^+; \\ \phi (m)(t)&= m(t) + \psi (m)(t)\begin{bmatrix} 1\\ 1 \end{bmatrix}, \quad t \ge 0. \end{aligned}$$

Proposition 3

Suppose that $m \in C^2$ and $m(0) \ge 0$. Then, $\psi (m)$ is the unique function l, such that

• 1. l is continuous and non-decreasing with $l(0)=0$,

• 2. $r(t) = m(t) + l(t) \ge 0$ for all $t \ge 0$,

• 3. l increases only when $r_1=0$ or $r_2=0$.

Proof

Let $l^*$ be any other solution. We set $y=r_1^* - r_1 = r_2^* - r_2 = l^* - l$. Using the Riemann–Stieltjes chain rule [31, Ch. 2.2]

$$\begin{aligned} f(y_t)=f(y_0) + \int _0^t f'(y) \,\mathrm {d}y, \end{aligned}$$

for any continuously differentiable $f: R \rightarrow R$. Taking $f(y) = y^2/2$, we get that

$$\begin{aligned} \frac{1}{2}\left( r_i^*(t) - r_i(t)\right) ^2 = \int _0^t (r_i^*-r_i)\,\mathrm {d}l^* + \int _0^t (r_i-r_i^*)\,\mathrm {d}l. \end{aligned}$$

(26)

The function $l^*$ increases when either $r_1^*=0$ or $r_2^*=0$. In addition, $r_1 \ge 0$ and $r_2 \ge 0$. Thus, either $(r_1^*-r_1)\,\mathrm {d}l^* \le 0$ or $(r_2^*-r_2)\,\mathrm {d}l^* \le 0$. Since $r_1^*-r_1 = r_2^*-r_2$, both terms are non-positive. The same principles yield that the second terms in both lines on the right-hand side of (26) are non-positive. Since the left-hand side $\ge 0$, both sides must be zero; thus, $r_1^*=r_1$, $r_2^*=r_2$, and $l^*=l$. $\square$

Proposition 4

The mappings $\psi$ and $\phi$ are Lipschitz continuous on $D_o[0,t]$ under the uniform topology for any fixed t.

Proof

We begin by proving the Lipschitz continuity of $\psi$. For this, we show that for any $T >0$ there exists $C \in R$ such that

$$\begin{aligned} \left||\psi (m) - \psi (m')\right||_T \le C\Big [\left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T\Big ], \end{aligned}$$

for all $m, m' \in D_0^2$.

$$\begin{aligned}&\left||\psi (m) - \psi (m')\right||_T \nonumber \\&\quad = \left||\sup _{0 \le s \le \cdot } \Big (-\big (m_1(s) \wedge m_2(s)\big )\Big )^+ - \sup _{0 \le s \le t} \Big (-\big (m'_1(s) \wedge m'_2(s)\big )\Big )^+\right||_T \nonumber \\&\quad \le \left||\sup _{0 \le s \le \cdot } \left| \big (m_1(s) \wedge m_2(s)\big ) - \big (m'_1(s) \wedge m'_2(s)\big )\right| \right||_T\nonumber \\&\quad = \left|| \big (m_1 \wedge m_2\big ) - \big (m'_1 \wedge m'_2\big )\right||_T \le 2\Big [\left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T\Big ]. \end{aligned}$$

(27)

The last inequality derives from

$$\begin{aligned} m_1(t) \wedge m_2(t) = \big (m_1(t)-m'_1(t) +m'_1(t)\big ) \wedge \big (m_2(t)-m'_2(t) +m'_2(t)\big ); \end{aligned}$$

therefore,

$$\begin{aligned} m_1(t) \wedge m_2(t) \le m'_1(t) \wedge m'_2(t) + \left||m_1-m'_1\right||_T + \left||m_2-m'_2\right||_T, \\ m_1(t) \wedge m_2(t) \ge m'_1(t) \wedge m'_2(t) - \left||m_1-m'_1\right||_T - \left||m_2-m'_2\right||_T, \end{aligned}$$

and

$$\begin{aligned} \left| m_1(t) \wedge m_2(t) - m'_1(t) \wedge m'_2(t)\right| \le \left||m_1-m'_1\right||_T + \left||m_2-m'_2\right||_T, \end{aligned}$$

which yields

$$\begin{aligned} \left||m_1(t) \wedge m_2(t) - m'_1(t) \wedge m'_2(t)\right||_T&\le \left||m_1-m'_1\right||_T + \left||m_2-m'_2\right||_T \\&\le 2 \left( \left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T\right) . \end{aligned}$$

Our next step is proving the Lipschitz continuity of $\phi$. For this, we show that for any $T >0$ there exists $C \in R$ such that

$$\begin{aligned} \left||\phi _1(m) - \phi _1(m')\right||_T \vee \left||\phi _2(m) - \phi _2(m')\right||_T \le C\Big [\left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T\Big ], \end{aligned}$$

for all $m, m' \in D_0^2$.

We begin with the left-hand side:

$$\begin{aligned}&\left||\phi _1(m) - \phi _1(m')\right||_T \vee \left||\phi _2(m) - \phi _2(m')\right||_T \\&= \left||m_1(t) + \psi (m)(t) - m'_1(t) - \psi (m')(t)\right||_T \vee \\&\qquad \left||m_2(t) + \psi (m)(t) - m'_2(t) - \psi (m')(t)\right||_T\\&= \left||m_1(t) - m'_1(t) + \psi (m)(t) - \psi (m')(t)\right||_T \vee \\&\qquad \left||m_2(t) - m'_2(t) + \psi (m)(t) - \psi (m')(t)\right||_T \\&\le \left||m_1(t) - m'_1(t)\right||_T + \left||\psi (m)(t) - \psi (m')(t)\right||_T \vee \\&\qquad \left||m_2(t) - m'_2(t)\right||_T + \left||\psi (m)(t) - \psi (m')(t)\right||_T \\&\le \left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T + \left||\psi (m)(t) - \psi (m')(t)\right||_T \\&\le 3 \left( \left||m_1-m'_1\right||_T \vee \left||m_2-m'_2\right||_T \right) , \end{aligned}$$

where the last inequality is derived from (27). $\square$

Appendix D: Lemma 1

Lemma 1

Let the function $f_{\eta }(\cdot ) \rightarrow 0$, u.o.c. as ${\eta } \rightarrow \infty$. Then, $f_{\eta }(g_{\eta }(\cdot )) \rightarrow 0$, u.o.c. as ${\eta } \rightarrow \infty$, for any $g_{\eta }(\cdot )$ that are locally bounded uniformly in $\eta$.

Proof

Choose $T>0$, and let $C_{T}$ be a constant such that $\left| g_{\eta }(t)\right| \le C_{T}$, for all $t \in [0, T]$. By the assumption on $f_{\eta }(\cdot )$, we have $\Vert f_\eta \Vert _{C_T} \rightarrow 0$ as $\eta \rightarrow \infty$. It follows that $\Vert f_\eta (g_\eta (\cdot ))\Vert _T \rightarrow 0$ as $\eta \rightarrow \infty$, which completes the proof. $\square$