A generic buffer occupancy expression for stop-and-wait hybrid automatic repeat request protocol over unstable channels

Abstract

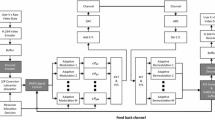
Recently, there has been a rapid progress in the field of wireless networks and mobile communications which makes the constraints on the used links clearly unconcealed. Wireless links are characterized by limited bandwidth and high latencies. Moreover, the bit-error-rate (BER) is very high in such environments for various reasons out of which weather conditions, cross-link interference, and mobility. High BER causes corruption in the data being transmitted over these channels. Therefore, convolutional encoding has been originated to be a professional means of communication over noisy environments. Sequential decoding, a category of convolutional codes, represents an efficient error detection and correction mechanism which attracts the attention for most of current researchers as for having a complexity that is dependent to the channel condition. In this paper, we propose a new queuing study over networking systems that make use of sequential decoders. Hence, the adopted flow and error control refer to stop-and-wait hybrid automatic repeat request. However, our queuing study is a novel extension to our prior work in which the lowest decoding complexity was fixed and did not account for the channel state. In other words, our proposed closed-form expression of the average buffer occupancy is totally generic and parameterized by not only channel condition and packet incoming rate, but also those that are automatically adapted to the channel conditions which include lower and upper bound decoding limits.

Appendices

Appendix 1: Proofs of Lemma 1 and Corollaries 1 and 2

Using (3) in (7), we can write $p_{1,T-1}$ as

$$\begin{aligned} p_{1,T-1} =(1-\lambda )^{T-1}(1-F_{T-1} )p_{1,0} . \end{aligned}$$

(52)

Substituting (52) into (6) (specifically in place of $p_{1,j}$ where $T-1=j)$ and using (1), (6) can be written as

$$\begin{aligned} \lambda p_{0,0} =\sum _{j=M-1}^{T-1} {c_{j+1} (1-\lambda )^{j+1}p_{1,0} } . \end{aligned}$$

(53)

After writing for $p_{1,0}$, (52) can be written as

$$\begin{aligned} p_{1,T-1} =\frac{\lambda (1-\lambda )^{T-1}(1-F_{T-1} )}{\sum _{\mathrm{j}=\hbox {M}-1}^{\hbox {T}-1} {c_{j+1} (1-\lambda )^{j+1}} }p_{0,0.} \end{aligned}$$

(54)

Substituting (52) into (12) and utilizing (3) give

$$\begin{aligned} p_{0,0,1,1} =(1-\lambda )^{T}(1-F_T )p_{1,0} . \end{aligned}$$

(55)

Extracting $p_{1,0}$ from (53) and using it in (55) produce

$$\begin{aligned} p_{0,0,1,1} =\alpha p_{0,0} , \end{aligned}$$

(56)

where,

$$\begin{aligned} \alpha =\frac{\lambda \hbox {(1-}\lambda )^{\hbox {T}}(1-F_T )}{{\sum \nolimits _{\mathrm{j}=M-\mathrm{{1}}}^{\mathrm{T}-1}} {c_{j+1} (1-\lambda \hbox {)}^{j+1}}}. \end{aligned}$$

(57)

Substituting (54) into (17) provides

$$\begin{aligned} p_{0,0,1,2} =(1-\lambda )\alpha \hbox { }p_{0,0} . \end{aligned}$$

(58)

Similarly, (18) can be written as

$$\begin{aligned} p_{1,0,1,2} =\lambda \alpha p_{0,0} . \end{aligned}$$

(59)

Appendix 2: Proofs of Theorem 1, Corollaries (3)–(5), Lemma 2, and Corollary 6

Referring to (22), we find that

$$\begin{aligned} P_0 (z)=p_{1,0} z+\sum _{n=2}^\infty {p_{n,0} z^{n}.} \end{aligned}$$

(60)

Substituting (9) and (10) into (60) and rearranging yield

$$\begin{aligned} P_0 (z)= & {} \sum _{j=M-1}^{T-1} \mu _{j-(M-2)}\nonumber \\&\left[ {\lambda \sum _{n=1}^\infty {p_{n,j} } z^{n}+(1-\lambda )\sum _{n=1}^\infty {p_{n+1,j} } z^{n}} \right] \nonumber \\&+\,\lambda zp_{0,0} +p_{0,0,1,2} z. \end{aligned}$$

(61)

Using $\sum _{n=1}^\infty {p_{n+1,j} z^{n}} =\frac{1}{z}\left( {P_j (z)-p_{1,j} z} \right)$ and accordingly (6) provide

$$\begin{aligned} P_0 (z)= & {} \frac{f(z)}{z}\sum _{j=M-1}^{T-1} {\mu _{j-(M-2)} P_j (z)}\nonumber \\&+\,\lambda (z-1)p_{0,0} +p_{0,0,1,2} z. \end{aligned}$$

(62)

For the derivation of$P_1 (z)$, we can write (22) as

$$\begin{aligned} P_1 (z)=p_{1,1} z+p_{2,1} z^{2}+\sum _{n=3}^\infty {p_{n,1} z^{n}.} \end{aligned}$$

(63)

Substituting (7), (15), and (16) into (63) and rearranging yield

$$\begin{aligned} P_1 (z)=\sum _{n=1}^\infty {(1-\lambda )p_{n,0} z^{n}} +\sum _{n=2}^\infty {\lambda p_{n-1,0} z^{n}+p_{1,0,1,2} z^{2}.}\nonumber \\ \end{aligned}$$

(64)

Using $\sum _{n=2}^\infty {p_{n-1,0} z^{n}} =z\sum _{n=2}^\infty {p_{n-1,0} z^{n-1}} =zP_0 (z)$ gives

$$\begin{aligned} P_1 (z)=f(z)P_0 (z)+p_{1,0,1,2} z^{2}. \end{aligned}$$

(65)

As for deriving$P_2 (z)$, (22) can be written as

$$\begin{aligned} P_2 (z)=p_{1,2} z+\sum _{n=2}^\infty {p_{n,2} z^{n}.} \end{aligned}$$

(66)

Substituting (7) and (11) into (66), rearranging, and simplifying, we acquire

$$\begin{aligned} P_2 (z)=f(z)P_1 (z)+zP_{1,1,2} (z). \end{aligned}$$

(67)

Using (65), we come into the possession of

$$\begin{aligned} P_2 (z)=f(z)^{2}P_0 (z)+f(z)p_{1,0,1,2} z^{2}+zP_{1,1,2} (z). \end{aligned}$$

(68)

To derive the general form (i.e., $P_j (z))$ where $M-1\ge j\ge 3$ at $M >3$, we use

$$\begin{aligned} P_j (z)=p_{1,j} z+\sum _{n=2}^\infty {p_{n,j} z^{n}.} \end{aligned}$$

(69)

Substituting (7) and (8) into (69) and rearranging, we obtain

$$\begin{aligned} P_j (z)=f(z)P_{j-1} (z). \end{aligned}$$

(70)

Using (2), we can write (69) recursively as

$$\begin{aligned} P_j (z)=f(z)^{j-2}P_2 (z). \end{aligned}$$

(71)

Utilizing (68), we attain

$$\begin{aligned} P_j (z)=f(z)^{j-2}\left[ {f(z)^{2}P_0 (z)+f(z)p_{1,0,1,2} z^{2}+zP_{1,1,2} (z)} \right] \nonumber \\ \end{aligned}$$

(72)

About the general form where $j\ge M$, (22) can be written after using (7) and (8) as

$$\begin{aligned} P_j (z)=(1-\mu _{j-(M-1)} )f(z)P_{j-1} (z). \end{aligned}$$

(73)

Using (3), we can write (73) recursively as

$$\begin{aligned} P_j (z)=(1-F_j )f(z)^{j-(M-1)}P_{M-1} (z). \end{aligned}$$

(74)

Using (71), we can find that

$$\begin{aligned} P_{M-1} (z)=f(z)^{M-3}P_2 (z). \end{aligned}$$

(75)

Substituting (68) into (75) and consequently into (74), we get

$$\begin{aligned} P_j (z)= & {} (1-F_j )f(z)^{j-2}\nonumber \\&\left[ {f^{2}(z)P_0 (z)+f(z)p_{1,0,1,2} z^{2}+zP_{1,1,2} (z)} \right] .\nonumber \\ \end{aligned}$$

(76)

As far as $P_{0,1,1} (z)$ is concerned, we use (12) and (13) in (23) as follows

$$\begin{aligned} P_{0,1,1} (z)= & {} \left( {1-\mu _{T-(M-1)} } \right) \nonumber \\&\left[ {(1-\lambda )\sum _{n=1}^\infty {p_{n+1,T-1} z^{n}+\lambda \sum _{n=1}^\infty {p_{n,T-1} z^{n}} } } \right] .\nonumber \\ \end{aligned}$$

(77)

Using $\sum _{n=1}^\infty {p_{n+1,T-1} z^{n}} =\frac{1}{z}\left( {P_{T-1} (z)-p_{1,T-1} z} \right)$ and simplifying yield

$$\begin{aligned} P_{0,1,1} (z)= & {} \left( {1-\mu _{T-(M-1)} } \right) \nonumber \\&\left[ {\frac{f(z)}{z}P_{T-1} (z)-(1-\lambda )p_{1,T-1} } \right] . \end{aligned}$$

(78)

Substituting (76) into (78) (particularly in place of $P_{T-1} (z)$ where $j=T-1)$ gives

$$\begin{aligned} P_{0,1,1} (z)= & {} \left( {1-\mu _{T-(M-1)} } \right) \frac{f(z)}{z}\nonumber \\&\left[ (1-F_{T-1} )f(z)^{T-2}\left( f(z)P_0 (z)\right. \right. \nonumber \\&\quad \left. \left. +p_{1,0,1,2}z^{2}+zP_{0,1,1} (z) \right) \right] \nonumber \\&-\,\left( {1-\mu _{T-(M-1)} } \right) (1-\lambda )p_{1,T-1} . \end{aligned}$$

(79)

After rearrangement and using (3), (54), and (59), we get

$$\begin{aligned} P_{0,1,1} (z)=\frac{\left( {1-F_T } \right) f(z)^{T-1}\left[ {f(z)P_0 (z)+p_{1,0,1,2} z^{2}} \right] -zp_{0,0,1,1} }{z\left[ {1-f(z)^{T-1}(1-F_T )} \right] }.\nonumber \\ \end{aligned}$$

(80)

Concerning the derivation of $P_{1,1,2} (z)$, (24) can be written after considering (19) and (20) as

$$\begin{aligned} P_{1,1,2} (z)=\sum _{n=1}^\infty {(1-\lambda )p_{n,0,1,1} z^{n}} +\sum _{n=2}^\infty {\lambda p_{n-1,0,1,1} z^{n}.}\nonumber \\ \end{aligned}$$

(81)

Using $\sum _{n=2}^\infty {\lambda p_{n-1,0,1,1} z^{n}=zP_{0,1,1} (z)}$, we obtain

$$\begin{aligned} P_{1,1,2} (z)=f(z)P_{0,1,1} (z). \end{aligned}$$

(82)

Referring to (62), it can be rewritten as

$$\begin{aligned} P_0 (z)= & {} \frac{1}{z}\mu _1 f(z)P_{M-1} (z)+\frac{1}{z}\sum _{j=M}^{T-1} {\mu _{j-(M-2)} f(z)P_j (z)}\nonumber \\&+\,\lambda (z-1)p_{0,0} +p_{0,0,1,2} z. \end{aligned}$$

(83)

Simplifying the summation part and using (1) and (76), we achieve

$$\begin{aligned}&\sum _{j=M}^{T-1} {\mu _{j-(M-2)} f(z)P_j (z)} =\left( {C(z)-c_M f(z)^{M}} \right) \nonumber \\&\quad \left[ {P_0 (z)+p_{1,0,1,2} \frac{z^{2}}{f(z)}+\frac{z}{f(z)}P_{0,1,1} (z)} \right] . \end{aligned}$$

(84)

where,

$$\begin{aligned} C(z)=\sum _{j=M-1}^{T-1} {c_{j+1} f(z)^{j+1}.} \end{aligned}$$

(85)

Consequently, (83) can be written as

$$\begin{aligned} P_0 (z)= & {} \frac{1}{z}\mu _1 f(z)P_{M-1} (z)+\frac{1}{z}\left( {C(z)-c_M f(z)^{M}} \right) \nonumber \\&\left[ {P_0 (z)+p_{1,0,1,2} \frac{z^{2}}{f(z)}+\frac{z}{f(z)}P_{0,1,1} (z)} \right] \nonumber \\&+\,\lambda (z-1)p_{0,0} +p_{0,0,1,2} z. \end{aligned}$$

(86)

Substituting (68) into (75) and using it in (86) and considering (82), we come into

$$\begin{aligned}&P_0 (z)\left( {z-\mu _1 f(z)^{M}-\left( {C(z)-c_M f(z)^{M}} \right) } \right) \nonumber \\&\quad =z^{2}\mu _1 f(z)^{M-1}p_{1,0,1,2}\nonumber \\&\qquad +\,\frac{z^{2}}{f(z)}\left( {C(z)-c_M f(z)^{M}} \right) p_{1,0,1,2} \nonumber \\&\qquad +\,\lambda z(z-1)p_{0,0} +p_{0,0,1,2} z^{2}\nonumber \\&\qquad +\,\left( \frac{z}{f(z)}\left( {C(z)-c_M f(z)^{M}} \right) \right. \nonumber \\&\qquad \left. +\,\mu _1zf(z)^{M-1} \right) P_{0,1,1} (z). \end{aligned}$$

(87)

Using (1) and (2), we can show that

$$\begin{aligned} \mu _1 =c_M . \end{aligned}$$

(88)

Presently, substituting (56) and (59) into (80) and accordingly the expression of $P_{0,1,1} (z)$into (87) after utilizing (88) reach

$$\begin{aligned} P_0 (z)=g(z)p_{0,0} , \end{aligned}$$

(89)

where,

$$\begin{aligned} g(z)=\frac{g_1 (z)}{f(z)\left( {z-C(z)} \right) +zf(z)^{T}(F_T -1)}, \end{aligned}$$

(90)

and,

$$\begin{aligned} g_1 (z)= & {} -\left( {\left( {\left( {\alpha (\lambda -1)z+\lambda (1-z)} \right) f(z)+\alpha (1-\lambda z)C(z)} \right. } \right. \nonumber \\&+\,\left( {\alpha (\lambda -1)z+\lambda (1-z)} \right) f(z)^{T}\left. {\left. {(F_T -1)} \right) z} \right) \nonumber \\ \end{aligned}$$

(91)

Substituting (56), (59), and (89) into (80), we obtain

$$\begin{aligned} P_{0,1,1} (z)=h(z)p_{0,0} , \end{aligned}$$

(92)

where,

$$\begin{aligned} h(z)=\frac{f(z)^{T-1}(1-F_T )\left[ {f(z)g(z)+\lambda \alpha z^{2}} \right] -z\alpha }{\left[ {1-f(z)^{T-1}(1-F_T )} \right] z}. \end{aligned}$$

(93)

On the other hand, using (92) in (82) provides

$$\begin{aligned} P_{1,1,2} (z)=f(z)h(z)p_{0,0} . \end{aligned}$$

(94)

Appendix 3: A Proof of Lemma 3

Alluding to (21) and utilizing the fact that $P(1)=1$, we get

$$\begin{aligned}&p_{0,0,1,1} +p_{0,0,1,2} +p_{1,0,1,2} +\sum _{k=0}^{T-1} P_k (1)\nonumber \\&\quad +P_{0,1,1} (1)+P_{1,1,2} (1) +p_{0,0} =1. \end{aligned}$$

(95)

Substituting $z=1$ into (89) induces

$$\begin{aligned} P_0 (1)=g(1)p_{0,0} . \end{aligned}$$

(96)

Having $z=1$ in (90) gives 0/0, then we apply L’Hospital’s rule as

$$\begin{aligned} g(1)=\mathop {\lim }\limits _{z\rightarrow 1} \frac{g_1 (z)}{\left( {z-C(z)} \right) f(z)+(F_T -1)zf(z)^{T}}, \end{aligned}$$

(97)

After reordering and simplifying, we have

$$\begin{aligned} g(1)=\frac{\alpha (\lambda -1)\left( {\lambda (T-1)+\rho } \right) +F_T \left( {\lambda +\alpha \left( {1-T\lambda (\lambda -1)} \right) } \right) }{\lambda (1-T)-\rho +F_T \left( {1+\lambda (T-1)} \right) },\nonumber \\ \end{aligned}$$

(98)

where,

$$\begin{aligned} \rho =\lambda \sum _{j=M-1}^{T-1} {(j+1)c_{j+1} } . \end{aligned}$$

(99)

Moreover, substituting $z=1$ into (65), (68), (72), and (76), and accordingly their expressions into (95) along with (25), (27), (28), and (96), we obtain

$$\begin{aligned} p_{0,0} =\frac{1}{1+2(g(1)+h(1)+\alpha )+\lambda \alpha +(g(1)+\lambda \alpha +h(1))(\omega +M-2)},\nonumber \\ \end{aligned}$$

(100)

where,

$$\begin{aligned} \omega =\sum _{j=M}^{T-1} {(1-F_j )} . \end{aligned}$$

(101)

Appendix 4: A Proof of Preposition 1

The derivative of (30) can be found as follows

$$\begin{aligned} {g}'(z)=\frac{a_1 (z)+a_2 (z)+a_3 (z)+a_4 (z)+a_5 (z)}{\left( {f(z)\left( {z-C(z)} \right) +zf(z)^{T}(F_T -1)} \right) ^{2}}, \end{aligned}$$

(102)

where,

$$\begin{aligned} a_1 (z)= & {} -\left( {\alpha (\lambda -1)-\lambda } \right) z^{2}f(z)^{2T}(F_T -1)^{2}\nonumber \\&-\,\alpha z(\lambda z-1)\left( {z-C(z)} \right) C(z){f}'(z)\nonumber \\&-\,\alpha T(\lambda z-1)(F_T -1){f}'(z)z^{2}f(z)^{T-1}C(z), \nonumber \\ \end{aligned}$$

(103)

$$\begin{aligned} a_2 (z)= & {} (F_T -1)\left( C(z)\left( \alpha \lambda z+(T-1)\left( -\alpha z+\lambda \right. \right. \right. \nonumber \\&+\,\left. \left. \left. (\alpha -1)\lambda z \right) {f}'(z) \right) \alpha z(\lambda z-1){C}'(z) \right) zf(z)^{T}, \nonumber \\\end{aligned}$$

(104)

$$\begin{aligned} a_3 (z)= & {} \alpha \left( C(z)\left( {\lambda z^{2}+C(z)-2\lambda zC(z)} \right) \right. \nonumber \\&+\,\left. z^{2}(\lambda z-1){C}'(z) \right) f(z), \end{aligned}$$

(105)

$$\begin{aligned} a_4 (z)= & {} (F_T -1)\left( {-\left( {-2\alpha z+\lambda +2\lambda (\alpha -1)z} \right) C(z)} \right. \nonumber \\&\left. +z\left( -2\alpha z+2\lambda (\alpha -1)z+\left( -\alpha z+\lambda \right. \right. \right. \nonumber \\&+\,\left. \left. \left. \lambda (\alpha -1)z \right) {C}'(z) \right) \right) f(z)^{T+1}, \end{aligned}$$

(106)

and,

$$\begin{aligned} a_5 (z)= & {} \left( \left( {-2\alpha z+\lambda +2\lambda (\alpha -1)z} \right) C(z)\right. \nonumber \\&+\,z\left( {z\left( {\alpha +\lambda (1-\alpha )} \right) } f(z)^{2} \right. \nonumber \\&\left. \left. +\left( -\lambda +z\left( \alpha +\lambda (1-\alpha ) \right) \right) {C}'(z) \right) \right) . \end{aligned}$$

(107)

Substituting $z=1$ into (102) yields

$$\begin{aligned} {g}'(1)=\frac{a_1 (1)+a_2 (1)+a_3 (1)+a_4 (1)+a_5 (1)}{\left( {f(1)\left( {1-C(1)} \right) +f(1)^{T}(F_T -1)} \right) ^{2}}. \end{aligned}$$

(108)

Taking into consideration that $f(1)=1$ and ${f}'(1)=\lambda$ and using (2) and (85), we get

$$\begin{aligned} C(1)=F_T . \end{aligned}$$

(109)

Taking the first derivation of (85) and then substituting for $z=1$, we obtain

$$\begin{aligned} {C}'(1)=\lambda \sum _{j=M-1}^{T-1} {(j+1)c_{j+1} =\rho .} \end{aligned}$$

(110)

Substituting $z=1$ into (103)–(107) and utilizing (109)–(110), we write

$$\begin{aligned} a_1 (1)= & {} (F_T -1)\left( \alpha (\lambda -1)-\lambda \right. \nonumber \\&\left. +\,F_T \left( {\lambda -\alpha (\lambda -1)\left( {1+\lambda (T-1)} \right) } \right) \right) , \end{aligned}$$

(111)

$$\begin{aligned} a_2 (1)= & {} (F_T -1)\left( \alpha \rho \left( {\lambda -1} \right) \right. \nonumber \\&+\,\left. \alpha \lambda F_T \left( {2+T(\lambda -1)-\lambda } \right) \right) , \end{aligned}$$

(112)

$$\begin{aligned} a_3 (1)= & {} \alpha \left( \rho \left( {\lambda -1} \right) +F_T \left( {\lambda +F_T (1-2\lambda )} \right) \right) , \end{aligned}$$

(113)

$$\begin{aligned} a_4 (1)= & {} (F_T -1)\left( 2\lambda -\alpha (\lambda -1)(2+\rho )\right. \nonumber \\&\left. +\,F_T \left( {2\alpha (\lambda -1)-\lambda } \right) \right) , \end{aligned}$$

(114)

and,

$$\begin{aligned} a_5 (1)=\lambda -\alpha \left( {\lambda -1} \right) (1+\rho )+F_T \left( {2\alpha \left( {\lambda -1} \right) -\lambda } \right) .\nonumber \\ \end{aligned}$$

(115)

Substituting (109), (111)–(115) into (108) yields 0/0. Hence, we apply L’Hospital’s rule to (102) as follows

$$\begin{aligned} {g}'(z)=\frac{{a}'_1 (z)+{a}'_2 (z)+{a}'_3 (z)+{a}'_4 (z)+{a}'_5 (z)}{2\left( {f(z)\left( {z-C(z)} \right) +zf(z)^{T}(F_T -1)} \right) \left( {f(z)\left( {z-C(z)} \right) +zf(z)^{T}(F_T -1)} \right) ^{\prime }}, \end{aligned}$$

(116)

Substituting $z=1$, we get

$$\begin{aligned} {g}'(1)=\frac{{a}'_1 (1)+{a}'_2 (1)+{a}'_3 (1)+{a}'_4 (1)+{a}'_5 (1)}{2\left( {f(1)\left( {1-C(1)} \right) +f(1)^{T}(F_T -1)} \right) \left( {\lambda \left( {1-T} \right) -\rho +F_T \left( {1+(T-1)\lambda } \right) } \right) }, \end{aligned}$$

(117)

Examining the derivative of (103)–(107), applying $z=1$, and utilizing (109)–(110) give

$$\begin{aligned} {a}'_1 (1)= & {} 2\lambda (1+\lambda T)+\alpha (\lambda -1)( {-2+T\lambda (\rho -2)-\lambda \rho } )\nonumber \\&+\,F_T ( {-4\lambda (1+\lambda T)+\alpha ( {-4+T\lambda ^{3}(T-1)} } \nonumber \\&+\,\lambda ( {6+T(\rho -6)-2\rho } )-\lambda ^{2} ( 3-2\rho \nonumber \\&+\,T({-8+T+\rho })))\nonumber \\&+\,F_T ( 2\lambda (1+\lambda T)+\alpha ( 2+\lambda ( -3+2\lambda \nonumber \\&+\,T( {4+\lambda ( {-6+T(1-\lambda )+\lambda })}))))), \end{aligned}$$

(118)

$$\begin{aligned} {a}'_2 (1)= & {} (F_T -1)( {\alpha \rho ( {-2+\lambda ( {5+2T(\lambda -1)-\lambda } )} )} \nonumber \\&+\,\lambda F_T ( \lambda (1-T)+\alpha ( 2-T+\lambda (T-1) )\nonumber \\&( {2+T\lambda } ))+\alpha \rho _1 (\lambda -1) ),\end{aligned}$$

(119)

$$\begin{aligned} {a}'_3 (1)= & {} \alpha ( \rho ( {-2+\lambda (3+\lambda )} )+F_T ( \lambda ( {2+\lambda -4\rho } )\nonumber \\&+\,2\rho )-\lambda F_T ^{2}(1+2\lambda )+\rho _1 (\lambda -1) ), \end{aligned}$$

(120)

$$\begin{aligned} {a}'_4 (1)= & {} (F_T -1)( 2\lambda ( {2+\lambda (1+T)})+F_T ( 2\alpha (\lambda -1)\nonumber \\&( {1+\lambda (1+T)})-\lambda ( {2+\lambda (1+T)})) \nonumber \\&\, {-\,\alpha (\lambda -1)( {4+(1+T)\lambda (2+\rho )+\rho _1 } )} ), \end{aligned}$$

(121)

and,

$$\begin{aligned} {a}'_5 (1)= & {} 2\lambda (1+\lambda )+F_T ( -2\lambda (1+\lambda )+2\alpha (\lambda -1)(1+2\lambda ) )\nonumber \\&-\,\alpha (\lambda -1)( {2+2\lambda (1+\rho )+\rho _1 } ). \end{aligned}$$

(122)

Substituting (118)–(122) and (109) into (117) lead to 0/0 one more time. By applying L’Hospital’s rule, for the second time, we get

$$\begin{aligned} {g}'(z)= & {} \frac{{a}''_1 (z)+{a}''_2 (z)+{a}''_3 (z)+{a}''_4 (z)+{a}''_5 (z)}{2\left( {b_1 ^{2}(z)+\left( {f(z)\left( {z-C(z)} \right) +zf(z)^{T}(F_T -1)} \right) \left( {b_2 (z)-b_3 (z)} \right) } \right) },\nonumber \\ \end{aligned}$$

(123)

where,

$$\begin{aligned} b_1 (z)= & {} f(z)+{f}'(z)( {z-C(z)} )+f(z)^{T-1}(F_T-1)\nonumber \\&({f(z)+Tz{f}'(z)} )-f(z){C}'(z), \end{aligned}$$

(124)

$$\begin{aligned} b_2 (z)= & {} Tf(z)^{T-2}(F_T -1)( (T-1)z{f}'(z)^{2}\nonumber \\&+\,f(z)({2{f}'(z)+z{f}''(z)} ) ), \end{aligned}$$

(125)

and,

$$\begin{aligned} b_3 (z)= & {} 2{f}'(z)\left( {{C}'(z)-1} \right) \nonumber \\&+\,\left( {C(z)-z}\right) {f}''(z)+f(z){C}''(z). \end{aligned}$$

(126)

Substituting $z=1$ into (123) yields

$$\begin{aligned} {g}'(1)=\frac{{a}''_1 (1)+{a}''_2 (1)+{a}''_3 (1)+{a}''_4 (1)+{a}''_5 (1)}{2\left( {b_1 ^{2}(1)+\left( {f(1)\left( {1-C(1)} \right) +f(1)^{T}(F_T -1)} \right) \left( {b_2 (1)-b_3 (1)} \right) } \right) }. \end{aligned}$$

(127)

Keeping into account that ${f}''(1)=0$ and referring to (85), we write

$$\begin{aligned} {C}''(1)=\lambda ^{2}\sum _{j=M-1}^{T-1} {j(j+1)c_{j+1} =\rho _1 } , \end{aligned}$$

(128)

and,

$$\begin{aligned} {C}'''(1)=\lambda ^{3}\sum _{j=M-1}^{T-1} {j(j+1)(j-1)c_{j+1} =\rho _2 } . \end{aligned}$$

(129)

Examining the second derivative of (103)–(107), substituting for $z=1,$ and using (109)–(110) and (128)–(129) provide

$$\begin{aligned} {a}''_1 (1)= & {} -2( {\alpha (\lambda -1)-\lambda } )( {1+T\lambda ( {4+\lambda (2T-1)} )} )\nonumber \\&+\,2\alpha (T-1)\lambda \rho ( {-2+( {3+T(\lambda -1)} )\lambda } )\nonumber \\&+\,2\alpha \lambda \rho ^{2}(\lambda -1)+F_T ^{2}( 2\lambda ( 1+T\lambda ( 4\nonumber \\&+\,\lambda (2T-1) ) )+\alpha ( 2+\lambda (2(\lambda -1)+T(10\nonumber \\&+\,\lambda ( -20+8T+( 10+(T-13)T )\lambda \nonumber \\&-\,(T-2)(T-1)\lambda ^{2} ) ) ) ) )+\alpha \lambda \rho _1 (T-1)(\lambda -1) \nonumber \\&+\,F_T ( -4\lambda +4T\lambda ^{2}( -4+\lambda (1-2T) )\nonumber \\&+\,\alpha ( -4+\lambda ( T^{3}(\lambda -1) \lambda ^{2}+T^{2}\lambda \nonumber \\&(\lambda ( 17-3\lambda -2\rho )+2(\rho -6) ) \nonumber \\&+\,2T( -9+2\rho +\lambda ( 15-4\rho +\lambda ( {\lambda +T-6} )) )\nonumber \\&-\,T\rho _1 (\lambda -1) \nonumber \\&{ { +2( {3(1-\lambda )+\rho (4\lambda -2)+(\lambda -1)\rho _1 } ) )} )} ),\nonumber \\\end{aligned}$$

(130)

$$\begin{aligned} {a}''_2 (1)= & {} (F_T -1)( -2\lambda ^{2}\rho (T-1)+\lambda F_T \nonumber \\&(-2(T-1)\lambda (1+T\lambda )+\alpha \nonumber \\&( {2-T+\lambda (T-1)} )(2+T\lambda ( {4+\lambda (T-1)} ) ) ) \nonumber \\&+\,\alpha ( \rho ( -2+\lambda ( 14-4\lambda \nonumber \\&+\,T( -8+3\lambda ( {5-T+\lambda (T-1)} ) ) ) )+\rho _1 \nonumber \\&{ {( -4+\lambda ( {8+3T(\lambda -1)-\lambda } ) )+\rho _2 (\lambda -1)} )} ),\nonumber \\\end{aligned}$$

(131)

$$\begin{aligned} {a}''_3 (1)= & {} \alpha ( 2\rho (1+\lambda )(4\lambda -1)+\rho ^{2}(2-4\lambda )\nonumber \\&-\,4\lambda ^{2}F_T ^{2}+\rho _1 ( {-4+\lambda ( {5+2\lambda } )} ) \nonumber \\&+\,2F_T ( {\lambda ( {1+\lambda (2-4\rho )-2( {\rho +\rho _1 } )} )+\rho _1 } )\nonumber \\&+\,\rho _1 (\lambda -1)),\end{aligned}$$

(132)

$$\begin{aligned} {a}''_4 (1)= & {} (F_T -1)( (T+1)\lambda F_T ( 2\alpha (\lambda -1)(2+T\lambda )\nonumber \\&-\,\lambda (4+T\lambda ) )+\lambda ( 4+2(1+T)\lambda (4+\lambda )\nonumber \\&-\,2\rho +\rho _1 )-\alpha (\lambda -1)( 4-2(\rho -\rho _1 )\nonumber \\&+\,(1+T)\lambda (T\lambda (2+\rho )+2(4+\rho _1 ) )+\rho _2 )),\nonumber \\ \end{aligned}$$

(133)

and,

$$\begin{aligned} {a}''_5 (1)= & {} 2\lambda F_T ( {-\lambda (4+\lambda )+2\alpha ( {-2+\lambda (1+\lambda )} )} )\nonumber \\&+\,\lambda ({2+2\lambda (4+\lambda )-2\rho +\rho _1 } )\nonumber \\&-\,\alpha (\lambda -1)( 2( 1-\rho +\rho _1\nonumber \\&+\,\lambda ( {4+\lambda (1+\rho )+2\rho _1 } ))+\rho _2 ). \end{aligned}$$

(134)

Substituting $z=1$ into (124)–(126), utilizing (109)–(110) and (128), and accordingly substituting them along with (130)–(134) into (127), we ultimately have (after simplifying)

$$\begin{aligned} {g}'(1)=\frac{\psi _1 +\left( {\psi _2 +\psi _3 } \right) F_T }{2\left( {\lambda (1-T)-\rho +\left( {\lambda (T-1)+1} \right) F_T } \right) ^{2}}, \end{aligned}$$

(135)

where,

$$\begin{aligned} \psi _1= & {} -2( \lambda ( {T-1} )+\rho )( \lambda ( {T-1} )\nonumber \\&\quad (\alpha ( \lambda -1 )-\lambda )-\alpha \rho ( 1+\lambda ( \lambda -3 ))), \end{aligned}$$

(136)

$$\begin{aligned} \psi _2= & {} -(T-1)\lambda ( \lambda (2+( 3T-2 )\lambda )\nonumber \\&+\,\alpha (4+\lambda (4( {\lambda -2})+T(4+( {\lambda -6})\lambda )))) \nonumber \\&-\,2\rho ( \lambda +\lambda ^{2}( {T-1} )+\alpha ( 2+\lambda ( 2( {\lambda -2})\nonumber \\&+\,T(2+\lambda (\lambda -4)))) ), \end{aligned}$$

(137)

and,

$$\begin{aligned} \psi _3= & {} \lambda \rho _1 (1-\alpha (\lambda -2 ))\nonumber \\&+\,F_T (\lambda (2+\lambda ( {T-1})( 2+T\lambda )))\nonumber \\&+\,\alpha F_T ( 2+\lambda ( -2+T( 4+\lambda (-(\lambda -4)( {\lambda -2} )\nonumber \\&+\,T(2+\lambda ( {\lambda -4})))))). \end{aligned}$$

(138)