Cooperative spectrum sensing using amplify-and-forward relaying with partial relay selection in cognitive radio networks

Abstract

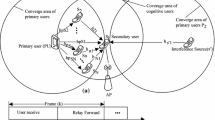
Cooperative spectrum sensing has been shown to be an effective approach to improve the detection performance by exploiting the spatial diversity among multiple cognitive nodes. By using the amplify-and-forward relaying with partial relay selection, this paper proposes a novel cooperative spectrum sensing scheme, which provides higher detection performance and is interesting in distributed cognitive radio networks. In the proposed sensing scheme, the “best” cognitive relay by means of partial relay selection technique amplifies and forwards the signals transmitted from the primary user (PU) to the cognitive user (CU). Then the CU detects PU’s states (i.e., presence or absence) via an energy detector. Moreover, the average missed-detection probability of proposed sensing scheme is studied over Nakagami-m fading channels, where m is a positive integer. In particular, the tight closed-form lower bounds of the average missed-detection probability are presented for the convenience of performance evaluation in practice. Finally, numerical results are provided to validate the derived closed-form lower bounds and the influence of the number of cognitive relays on the detection performance is also discussed.

Appendices

Appendix 1: Proof of Lemma 1

In order to derive the PDF of γ _r  = γ _P,r γ _C,r /(γ _P,r  + γ _C,r  + 1), we first find the PDF of γ _P,r and the CDF of γ _C,r . By recalling the definition of γ _P,r , its PDF over Nakagami-m fading channels can be given by [23, Eq. (2.21)]

$$f_{\gamma_{P,r}}(\gamma)= \frac{1}{\Upgamma(m_1)} {\left(\frac{m_1}{\upsilon}\right)}^{m_1} \gamma^{m_1 -1}e^{-\frac{m_1}{\upsilon}\gamma} U(\gamma)$$

(18)

where $\Upgamma(\cdot)$ is the gamma function [20, Eq. (8.310.1)], and $\Upgamma(m)=(m-1)!$ for ${m\in \mathbb{Z}^+}$. Owing to the “best” cognitive relay $r = \arg {\mathop{\max}\limits_{k\in \{1,2,\dots,K\}}} \{\gamma_{C,k}\}$, the CDF of γ _C,r over independent and identically distributed Nakagami-m fading channels can be calculated as

$$\begin{aligned} F_{\gamma_{C,r}}(\gamma)=& {\left[\int\limits_{0}^{\gamma}\frac{1}{\Upgamma(m_2)}{\left(\frac{m_2}{\omega} \right)}^{m_2} x^{m_2 -1} e^{-\frac{m_2}{\omega}x} dx\right]}^K\\ =& {\left[1-e^{-\frac{m_2}{\omega}\gamma}\sum_{k=0}^{m_2 -1} \frac{1}{k!} {\left(\frac{m_2}{\omega}\gamma\right)}^{k} \right]}^K\\ =& 1+ \sum_{a_0 =1}^{K} \left({\begin{array}{l} K\\ a_0\\ \end{array}}\right)(-1)^{a_0} e^{-\frac{a_0 m_2}{\omega}\gamma} {\left(\sum_{k=0}^{m_2 -1} \frac{1}{k!} {\left(\frac{m_2}{\omega}\gamma\right)}^{k}\right)}^{a_0}. \end{aligned}$$

(19)

When m ₂ > 1 and ${m_2 \in \mathbb{Z}^+}$, by making use of the binomial theorem, we have

$$\begin{aligned} &\left({\sum_{k=0}^{m_2 -1} \frac{1}{k!}\left({\frac{m_2}{\omega} \gamma}\right)^{k}}\right)^{a_0}\\ &\quad=\sum_{a_1 =0}^{a_0} \left({\begin{array}{l} a_0\\ a_1\\ \end{array}}\right) \left({\frac{m_2}{\omega} \gamma}\right)^{a_1} \sum_{a_2 =0}^{a_1} \left({\begin{array}{l} a_1\\ a_2\\ \end{array}}\right) \left({\frac{1}{2} \frac{m_2}{\omega}\gamma} \right)^{a_2} \sum_{a_3 =0}^{a_2} \left({\begin{array}{l} a_2\\ a_3\\ \end{array}}\right) \left({\frac{1}{3} \frac{m_2}{\omega}\gamma}\right)^{a_3}\\ &\quad\quad\times{\cdots}\times \sum\limits_{a_{m_2 -1} =0}^{a_{m_2 -2}} \left({\begin{array}{l} a_{m_2 -2}\\ a_{m_2 -1}\\ \end{array}}\right) \left({\frac{1}{m_2 -1} \frac{m_2}{\omega}\gamma}\right)^{a_{m_2 -1}}\\ &\quad=\sum_{a_1 =0}^{a_0} \sum_{a_2 =0}^{a_1} {\cdots} \sum_{a_{m_2 -1} =0}^{a_{m_2 -2}} \left\{{\prod_{b=0}^{m_2 -2} \left({\begin{array}{l} a_b\\ a_{b+1}\\ \end{array}}\right) \frac{1}{(b+1)^{a_{b+1}}}}\right\} \left({\frac{m_2}{\omega}\gamma}\right)^{\sum_{b=1}^{m_2 -1} a_b}. \end{aligned}$$

(20)

Hence, by substituting (20) into (19), we obtain the CDF of γ _C,r as follows

$$\begin{aligned} F_{\gamma_{C,r}}(\gamma)&=1+\sum_{a_0 =1}^{K} \sum_{a_1 =0}^{a_0} \sum_{a_2 =0}^{a_1} \cdots \sum_{a_{m_2 -1} =0}^{a_{m_2 -2}} \left({\begin{array}{l} K\\ a_0 \\ \end{array}}\right)(-1)^{a_0}\\ &\quad\times \left\{\prod_{b=0}^{m_2 -2} \left({\begin{array}{l} a_b\\ a_{b+1}\\ \end{array}}\right) \frac{1}{(b+1)^{a_{b+1}}}\right\} e^{-\frac{a_0 m_2}{\omega}\gamma} {\left(\frac{m_2}{\omega}\gamma\right)}^{\sum_{b=1}^{m_2 -1} a_b}. \end{aligned}$$

(21)

From (18) and (21), we can derive the CDF of γ _r as

$$\begin{aligned} F_{\gamma_r}(\gamma) &= Pr\left\{\frac{\gamma_{P,r}\gamma_{C,r}}{\gamma_{P,r} +\gamma_{C,r} +1} \leq \gamma\right\}\\ &= \int\limits_{0}^{\gamma} Pr\left\{\gamma_{C,r} \geq \frac{(x+1)\gamma}{x-\gamma}\right\} f_{\gamma_{P,r}}(x) dx + \int\limits_{\gamma}^{\infty} Pr\left\{\gamma_{C,r} \leq \frac{(x+1)\gamma}{x-\gamma}\right\} f_{\gamma_{P,r}}(x) dx\\ &=1+\sum_{a_0 =1}^{K} \sum_{a_1 =0}^{a_0} \sum_{a_2 =0}^{a_1} \cdots \sum_{a_{m_2 -1} =0}^{a_{m_2 -2}} \left({\begin{array}{l} K\\ a_0\\ \end{array}}\right) (-1)^{a_0} \left\{\prod_{b=0}^{m_2 -2} \left({\begin{array}{l} a_b\\ a_{b+1}\\ \end{array}}\right) \frac{1}{(b+1)^{a_{b+1}}}\right\}\\ &\quad\times \underbrace{\int\limits_{\gamma}^{\infty} e^{-\frac{a_0 m_2}{\omega} \frac{(x+1)\gamma}{x-\gamma}} {\left(\frac{m_2}{\omega} \,\frac{(x+1)\gamma}{x-\gamma}\right)}^{\sum_{b=1}^{m_2 -1} a_b} f_{\gamma_{P,r}}(x) dx}_{\varDelta}. \end{aligned}$$

(22)

Let u = x − γ. $\varDelta$ in (22) can be written as

$$\begin{aligned} \varDelta =& \frac{1}{\Upgamma(m_1)} {\left(\frac{m_1}{\upsilon}\right)}^{m_1} {\left(\frac{m_2}{\omega}\right)}^{\sum_{b=1}^{m_2 -1} a_b} \gamma^{\left(\sum_{b=1}^{m_2 -1} a_b\right) +m_1 -1} e^{-\frac{a_0 m_2 \upsilon + m_1 \omega}{\upsilon\omega}\gamma}\\ &\times \int\limits_{0}^{\infty} {\left(1+ \frac{1+\gamma}{u}\right)}^{\sum_{b=1}^{m_2 -1} a_b} {\left(1+ \frac{u}{\gamma}\right)}^{m_1 -1} e^{-\frac{a_0 m_2 (1+\gamma)\gamma}{\omega u} - \frac{m_1}{\upsilon}u} du. \end{aligned}$$

(23)

With the aid of the binomial theorem, (23) can be rewritten as

$$\begin{aligned} \varDelta =& \frac{1}{\Upgamma(m_1)} {\left(\frac{m_1}{\upsilon} \right)}^{m_1} {\left(\frac{m_2}{\omega}\right)}^{\sum_{b=1}^{m_2 -1} a_b} \gamma^{\left(\sum_{b=1}^{m_2 -1} a_b\right) +m_1 -1} e^{-\frac{a_0 m_2 \upsilon + m_1 \omega}{\upsilon\omega} \gamma}\\ &\times \sum\nolimits_{k_1 =0}^{\sum_{b=1}^{m_2 -1} a_b} \sum_{k_2 =0}^{m_1 -1} \left({\begin{array}{l} \sum\nolimits_{b=1}^{m_2 -1} a_b\\ k_1 \end{array}}\right) \left({\begin{array}{l} m_1 -1\\ k_2\end{array}}\right) (1+\gamma)^{k_1} \gamma^{-k_2}\\ &\times \int\limits_{0}^{\infty} u^{k_2 -k_1} e^{-\frac{a_0 m_2 (1+\gamma)\gamma}{\omega u} - \frac{m_1}{\upsilon}u} du. \end{aligned}$$

(24)

Then, making use of [20, Eq. (3.471.9), 24], the integral term in (24) can be written in closed form as follows:

$$\begin{aligned} &\int\limits_{0}^{\infty} u^{k_2 -k_1} e^{-\frac{a_0 m_2 (1+\gamma)\gamma}{\omega u} - \frac{m_1}{\upsilon}u} du\\ &=2{\left({\frac{a_0 m_2 \upsilon (1+\gamma)\gamma}{m_1 \omega}}\right)}^{\frac{k_2 -k_1 +1}{2}} K_{k_2 -k_1 +1} \left({2\sqrt{\frac{a_0 m_1 m_2 (1+\gamma)\gamma}{\upsilon \omega}}}\right) \end{aligned}$$

(25)

where $K_v(\cdot)$ is the vth order modified Bessel function of the second kind.

By substituting (24), (25) into (22), we arrive at the CDF of γ _r , which can be expressed as

$$\begin{aligned} F_{\gamma_r}(\gamma)=\,&1+\sum_{a_0 =1}^{K} \sum_{a_1 =0}^{a_0} \sum_{a_2 =0}^{a_1} \cdots \sum_{a_{m_2 -1} =0}^{a_{m_2 -2}} \left({\begin{array}{l} K\\ a_0 \\ \end{array}}\right)(-1)^{a_0}\\ &\times \left\{\prod_{b=0}^{m_2 -2} \left({\begin{array}{l} a_b\\ a_{b+1}\\ \end{array}}\right) \frac{1}{(b+1)^{a_{b+1}}}\right\} \times \varDelta \end{aligned}$$

(26)

in which $\varDelta$ is given by

$$\begin{aligned} \varDelta =& 2 \frac{1}{(m_1 -1)!} {\left(\frac{m_1}{\upsilon} \right)}^{m_1} {\left(\frac{m_2}{\omega}\right)}^{\sum_{b=1}^{m_2 -1} a_b} \sum_{k_1 =0}^{\sum_{b=1}^{m_2 -1} a_b} \sum_{k_2 =0}^{m_1 -1} \left({\begin{array}{l} \sum\nolimits_{b=1}^{m_2 -1} a_b\\ k_1 \end{array}}\right) \\ &\times \left({\begin{array}{l} m_1 -1\\ k_2 \end{array}}\right) \left({\frac{a_0 m_2 \upsilon}{m_1 \omega}}\right)^{\frac{k_2 -k_1 +1}{2}}\gamma^{\left(\sum_{b=1}^{m_2 -1} a_b\right) +m_1 - \frac{k_1 +k_2+1}{2}} (1+\gamma)^{\frac{k_1 +k_2 +1}{2}}\\ &\times e^{-\frac{a_0 m_2 \upsilon +m_1 \omega}{\upsilon \omega}\gamma} K_{k_2 -k_1 +1} \left(2\sqrt{\frac{a_0 m_1 m_2 (1+\gamma)\gamma}{\upsilon\omega}}\right) \end{aligned}$$

where m ₂ > 1 and ${m_1, m_2 \in \mathbb{Z}^+}$.

Then, by taking the derivative of (26) with respect to γ, the closed-form PDF expression of γ _r over Nakagami-m fading channels is derived as shown in Lemma 1. The proof is completed.

Appendix 2: Proof of Lemma 2

Owing to X = min(γ _P,r , γ _C,r ), the CDF of X is calculated as

$$F_X (x) = 1-\left[1-F_{\gamma_{P,r}}(x)\right] \left[1-F_{\gamma_{C, r}}(x)\right]$$

(27)

where $F_{\gamma_{P,r}}(x) = 1- e^{-\frac{m_1}{\upsilon}x} \sum_{k=0}^{m_1 -1} \frac{1}{k!} {\left(\frac{m_1}{\upsilon} x\right)}^k$, and $F_{\gamma_{C,r}}(x)$ is given in (21). Hence, the PDF of X can be derived by taking the derivative of F _X (x) with respect to x, and the lemma is proved.

Appendix 3: Proof of Theorem 1

By substituting (13) into (12), we have

$$\begin{aligned} \bar{P}_{m,Nak}^{lb} =& 1- \sum\limits_{a_0 = 1}^{K} \sum\limits_{a_1 = 0}^{a_0} \sum\limits_{a_2 = 0}^{a_1} \cdots \sum\limits_{a_{m_2 - 1} = 0}^{a_{m_2 - 2}} \left({\begin{array}{l} K\\ a_0\\ \end{array}}\right)(-1)^{a_0}\\ &\times\left\{{\prod_{b=0}^{m_2 - 2} \left({\begin{array}{l} a_b\\ a_{b+1} \end{array}}\right) \frac{1}{(b+1)^{a_{b+1}}}}\right\} \left\{-\underbrace{\int_{0}^{\infty} Q(\sqrt{2\gamma}, \sqrt{\lambda}) \Uppsi_1 d\gamma}_{\Upphi_1}\right.\\ &\left.+\underbrace{\int_{0}^{\infty} Q(\sqrt{2\gamma}, \sqrt{\lambda}) \Uppsi_2 d\gamma}_{\Upphi_2} -\underbrace{\int_{0}^{\infty} Q(\sqrt{2 \gamma}, \sqrt{\lambda}) \Uppsi_3 d\gamma}_{\Upphi_3}\right\}. \end{aligned}$$

(28)

Let $\sqrt{2\gamma} = t, \Upphi_1$ in (28) can be written as

$$\begin{aligned} \Upphi_1 &= a_0 {\left(\frac{m_2}{\omega}\right)}^{\left(\sum_{b =1}^{m_2 -1} a_b\right) +1} \sum_{k=0}^{m_1 -1} \left\{\frac{1}{k!} {\left(\frac{m_1}{\upsilon}\right)}^k \frac{1}{2^{\left(\sum_{b=1}^{m_2 -1} a_b\right) +k}}\right.\\ &\left.\times \int\limits_{0}^{\infty} t^{2\left[\left(\sum_{b=1}^{m_2 -1} a_b\right) +k +1\right] -1} e^{-\frac{a_0 m_2 \upsilon +m_1 \omega}{\upsilon\omega} \times \frac{t^2}{2}} Q(t,\sqrt{\lambda}) dt\right\}. \end{aligned}$$

(29)

With the help of [22, Eq. (9)], the integral term in (29) can be calculated in closed form as follows:

$$\begin{aligned} &\int\limits_{0}^{\infty} t^{2\left[{\left({\sum_{b=1}^{m_2 -1} a_b}\right) +k +1}\right] -1} e^{-\frac{a_0 m_2 \upsilon +m_1 \omega}{\upsilon\omega} \times \frac{t^2}{2}} Q(t,{\sqrt{\lambda}})dt\\ &=2^{\left({\sum_{b=1}^{m_2 -1} a_b}\right) +k} \left({\left({\left({ \sum\nolimits_{b=1}^{m_2 -1} a_b}\right) +k}\right)!}\right) \left({\frac{\upsilon\omega}{a_0 m_2 \upsilon + m_1 \omega}}\right)^{\left({\sum_{b=1}^{m_2 -1} a_b}\right) +k +1}\\ &\times \frac{\upsilon\omega}{a_0 m_2 \upsilon + m_1 \omega +\upsilon\omega} e^{-\frac{\lambda}{2}\, \frac{a_0 m_2 \upsilon +m_1 \omega}{a_0 m_2 \upsilon + m_1 \omega +\upsilon\omega}} \sum_{i_1 =0}^{\left({\sum_{b=1}^{m_2 -1} a_b}\right) +k} \left\{\varepsilon_{i_1}\right.\\ &\left.\times {\left(\frac{a_0 m_2 \upsilon +m_1 \omega}{a_0 m_2 \upsilon +m_1 \omega + \upsilon\omega}\right)}^{i_1} L_{i_1}\left(-\frac{\lambda}{2}\frac{\upsilon\omega}{a_0 m_2 \upsilon +m_1 \omega +\upsilon\omega}\right)\right\} \end{aligned}$$

(30)

where $\varepsilon_{i_1} = \left\{{\begin{array}{ll} 1,& i_1 <\left({\sum_{b=1}^{m_2 -1}a_b}\right) +k\\ 1+\frac{a_0 m_2 \upsilon + m_1 \omega}{\upsilon\omega}, & i_1 = \left({\sum_{b=1}^{m_2 -1}a_b}\right) +k \end{array}}\right.$, and $L_k(\cdot)$ denotes the Laguerre polynomial.

Substituting (30) into (29) yields the closed-form expression of $\Upphi_1$. Similarly, we can obtain closed-form expressions of $\Upphi_2$ and $\Upphi_3$. By substituting $\Upphi_1, \Upphi_2$ and $\Upphi_3$ into (28), we get the closed-form lower bound of $\bar{P}_{m,Nak}$ as shown in Theorem 1. The proof is completed.