Skip to main content
SpringerLink
Log in

Existence and sum decomposition of vertex polyhedral convex envelopes

  • Original Paper
  • Published:
Optimization Letters Aims and scope Submit manuscript

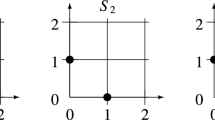
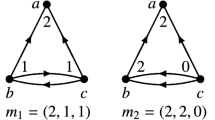
Abstract

Convex envelopes are a very useful tool in global optimization. However finding the exact convex envelope of a function is a difficult task in general. This task becomes considerably simpler in the case where the domain is a polyhedron and the convex envelope is vertex polyhedral, i.e., has a polyhedral epigraph whose vertices correspond to the vertices of the domain. A further simplification is possible when the convex envelope is sum decomposable, i.e., the convex envelope of a sum of functions coincides with the sum of the convex envelopes of the summands. In this paper we provide characterizations and sufficient conditions for the existence of a vertex polyhedral convex envelope. Our results extend and unify several results previously obtained for special cases of this problem. We then characterize sum decomposability of vertex polyhedral convex envelopes, and we show, among else, that the vertex polyhedral convex envelope of a sum of functions coincides with the sum of the vertex polyhedral convex envelopes of the summands if and only if the latter sum is vertex polyhedral.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adjiman C.S., Dallwig S., Floudas C.A., Neumaier A. (1998). A global optimization method, αBB, for general twice-differentiable NLPs I. Theor. Adv. Comput. Chem. Eng. 22: 1137–1158

    Google Scholar 

  2. Androulakis I.P., Maranas C.D., Floudas C.A. (1995). αBB: A global optimization method for general constrained nonconvex problems. J. Glob. Optim. 7: 337–363

    Article  MATH  MathSciNet  Google Scholar 

  3. Al-Khayyal F.A., Falk J.E. (1983). Jointly constrained biconvex programming. Math. Oper. Res. 8: 273–286

    MATH  MathSciNet  Google Scholar 

  4. Benson H.P. (2002). Using concave envelopes to globally solve the nonlinear sum of ratios problem. J. Glob. Optim. 22: 343–364

    Article  MATH  MathSciNet  Google Scholar 

  5. Benson H.P. (2004). Concave envelopes of monomial functions over rectangles. J. Naval Res. Logist. 51: 467–476

    Article  MATH  MathSciNet  Google Scholar 

  6. Benson H.P. (2004). On the construction of convex and concave envelope formulas for bilinear and fractional functions on quadrilaterals. Comput. Optim. Appl. 27: 5–22

    Article  MATH  MathSciNet  Google Scholar 

  7. Benson H.P., Erenguc S.S. (1998). Using convex envelopes to solve the interactive fixed-charge linear programming problem. J. Optim. Theory Appl. 59: 223–246

    MathSciNet  Google Scholar 

  8. Crama Y. (1993). Concave extensions for nonlinear 0-1 maximization problems. Math. Programming 61: 53–60

    Article  MathSciNet  Google Scholar 

  9. Falk J.E. (1969). Lagrange multipliers and nonconvex programs. SIAM J. Control 7: 534–545

    Article  MATH  MathSciNet  Google Scholar 

  10. Falk J.E. (1974). Sharper bounds on nonconvex programs. Oper. Res. 22: 410–413

    MATH  MathSciNet  Google Scholar 

  11. Falk J.E., Hoffman K.R. (1976). A successive underestimation method for concave minimization problems. Math. Oper. Res. 1: 251–259

    Article  MATH  Google Scholar 

  12. Falk J.E., Soland R.M. (1969). An algorithm for separable nonconvex programming problems. Manage. Sci. 15: 550–569

    MathSciNet  MATH  Google Scholar 

  13. Horst R. (1984). On the convexification of nonlinear programming problems: an applications-oriented survey. Eur. J. Oper. Res. 15: 382–392

    Article  MATH  MathSciNet  Google Scholar 

  14. Horst R., Tuy H. (1993). Global Optimization: Deterministic Approaches, 2nd edn. Springer, Berlin

    Google Scholar 

  15. Giannessi, F, Tardella, F.: Connections between nonlinear programming and discrete optimization. In: Handbook of Combinatorial Optimization, vol. 1, pp. 149–188. Kluwer, Boston (1998)

  16. Grotzinger S.J. (1985). Supports and convex envelopes. Math. Programming 31: 339–347

    Article  MATH  MathSciNet  Google Scholar 

  17. Kalantari B., Rosen J.B. (1987). An algorithm for global minimization of linearly constrained concave quadratic functions. Math. Oper. Res. 12: 544–561

    MATH  MathSciNet  Google Scholar 

  18. Kleibohm K. (1967). Bemerkungen zum Problem der nichtkonvexen Programmierung. Unternehmensforschung 11: 49–60

    Article  MATH  MathSciNet  Google Scholar 

  19. McCormick G.P. (1976). Computability of global solutions to factorable nonconvex programs. I. Convex underestimating problems. Math. Programming 10: 147–175

    Article  MATH  MathSciNet  Google Scholar 

  20. Meyer C.A., Floudas C.A. (2003). Convex Hull of Trilinear Monomials with Positive or Negative Domains: Facets of the Convex and Concave Envelopes. In: Floudas, C.A., Pardalos, P.M. (eds) Frontiers In Global Optimization, pp 327–352. Kluwer, Boston

    Google Scholar 

  21. Meyer C.A., Floudas C.A. (2004). Convex Hull of Trilinear Monomials with Mixed Sign Domains. J. Glob. Optim. 29: 125–155

    Article  MATH  MathSciNet  Google Scholar 

  22. Meyer C.A., Floudas C.A. (2005). Convex envelopes for edge-concave functions. Math. Programming 103: 207–224

    Article  MATH  MathSciNet  Google Scholar 

  23. Rockafellar RT (1970). Convex Analysis, Princeton Mathematical Series, No. 28. Princeton University Press, Princeton

    Google Scholar 

  24. Rikun A.D. (1997). A convex envelope formula for multilinear functions. J. Glob. Optim. 10: 425–437

    Article  MATH  MathSciNet  Google Scholar 

  25. Ryoo H.S., Sahinidis N.V. (2001). Analysis of bounds for multilinear functions. J. Glob. Optim. 19: 403–424

    Article  MATH  MathSciNet  Google Scholar 

  26. Sherali H.D. (1997). Convex envelopes of multilinear functions over a unit hypercube and over special discrete sets. Acta Math. Vietnam 22: 245–270

    MATH  MathSciNet  Google Scholar 

  27. Sherali H.D., Alameddine A. (1990). An explicit characterization of the convex envelope of a bivariate bilinear function over special polytopes. Ann. Oper. Res. 25: 197–209

    Article  MATH  MathSciNet  Google Scholar 

  28. Tardella F. (1988). On a class of functions attaining their maximum at the vertices of a polyhedron. Discrete Appl. Math. 22: 191–195

    Article  MathSciNet  Google Scholar 

  29. Tardella F. (1990). On the equivalence between some discrete and continuous optimization problems. Ann. Oper. Res. 25: 291–300

    Article  MATH  MathSciNet  Google Scholar 

  30. Tardella F. (2003). On the existence of polyhedral convex envelopes. In: Floudas, C.A., Pardalos, P.M. (eds) Frontiers In Global Optimization, pp 149–188. Kluwer, Boston

    Google Scholar 

  31. Tardella, F.: Constructing vertex polyhedral convex envelopes, forthcoming

  32. Tawarmalani M., Sahinidis N.V. (2001). Semidefinite relaxations of fractional programs via novel convexification techniques. J. Glob. Optim. 20: 137–158

    Article  MATH  MathSciNet  Google Scholar 

  33. Tawarmalani M., Sahinidis N.V. (2002). Convex extensions and envelopes of lower semi-continuous functions. Math. Programming 93: 247–263

    Article  MATH  MathSciNet  Google Scholar 

  34. Tawarmalani, M., Sahinidis, N.V.: Convexification and global optimization in continuous and mixed-integer nonlinear programming. In: Theory, Algorithms, Software, and Applications, Nonconvex Optimization and its Applications, Vol. 65. Kluwer, Dordrecht (2002)

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica per le Decisioni, Economiche Finanziarie e Assicurative, Facoltà di Economia, Università di Roma “La Sapienza”, Via del Castro Laurenziano, 9, 00161, Rome, Italy

    Fabio Tardella

Authors
  1. Fabio Tardella
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Fabio Tardella.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Tardella, F. Existence and sum decomposition of vertex polyhedral convex envelopes. Optimization Letters 2, 363–375 (2008). https://doi.org/10.1007/s11590-007-0065-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11590-007-0065-2

Keywords

Search

Navigation