Modelling and optimal control for Chikungunya disease

Abstract

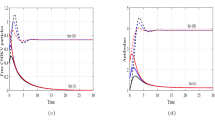
A generalized model of intra-host CHIKV infection with two routes of infection has been proposed. In a first step, the basic reproduction number $\mathscr {R}_0$ was calculated using the next-generation matrix method and the local and global stability analyses of the steady states are carried out using the Lyapunov method. It is proven that the CHIKV-free steady state $\bar{E}$ is globally asymptotically stable when $\mathscr {R}_0\le 1,$ and the infected steady state $E^*$ is globally asymptotically stable when $\mathscr {R}_0>1$. In a second step, we applied an optimal strategy via the antibodies’ flow rate in order to optimize infected compartment and to maximize the uninfected one. For this, we formulated a nonlinear optimal control problem. Existence of the optimal solution was discussed and characterized using an adjoint variables. Thus, an algorithm based on competitive Gauss–Seidel-like implicit difference method was applied in order to resolve the optimality system. The theoretical results are confirmed by some numerical simulations.

Appendix: Appropriated scheme for the control problem

Consider a subdivision of the time interval [0, T] as follows: $[0,T] =\displaystyle \bigcup\nolimits_{n=0}^{N-1} [t_n, t_{n+1}], \quad t_n = n \mathrm{d}t, \quad \mathrm{d}t = T/N$. Let $S^n, I^n, P^n, A^n, \lambda ^n_1, \lambda ^n_2, \lambda ^n_3, \lambda ^n_4$ and $\Lambda _2^n$ be an approximation of S(t), I(t), P(t), A(t), $\lambda _1(t)$, $\lambda _2(t), \lambda _3(t), \lambda _4(t)$ and the control $\Lambda _2(t)$ at the time $t_n$. $S^0, I^0, P^0, A^0$, $\lambda ^0_1, \lambda ^0_2, \lambda ^0_3, \lambda ^0_4$ and $\Lambda _2^0$ as the state and adjoint variables and the controls at initial time. $S^N, I^N, P^N, A^N$, $\lambda ^N_1, \lambda ^N_2, \lambda ^N_3, \lambda ^N_4$ and $\Lambda _2^N$ as the state and adjoint variables and the control at final time T. In order to resolve the state system, a created improving the Gauss–Seidel-like implicit finite-difference method was applied. For the adjoint system, a first-order backward difference is applied and then the following appropriated scheme was adapted:

$$\begin{aligned} \left\{ \begin{array}{lll} \displaystyle \dfrac{S^{n+1}-S^n}{\mathrm{d}t} \displaystyle =\Lambda _1-m S^{n+1}-S^{n+1}\dfrac{\bar{\mu }_1 P^n}{k_1+P^n}-S^{n+1}\dfrac{\bar{\mu }_2 I^n}{k_2+I^n},\\ \\ \displaystyle \dfrac{I^{n+1}-I^n}{\mathrm{d}t} \displaystyle =S^{n+1}\dfrac{\bar{\mu }_1 P^n}{k_1+P^n}+S^{n+1}\dfrac{\bar{\mu }_2 I^{n+1}}{k_2+I^{n}}-m I^{n+1},\\ \\ \displaystyle \dfrac{P^{n+1}-P^n}{\mathrm{d}t} \displaystyle =\pi I^{n+1}-m P^{n+1}-r A^nP^{n+1},\\ \\ \displaystyle \dfrac{A^{n+1}-A^n}{\mathrm{d}t} \displaystyle =\Lambda _2^n+r A^{n+1}P^{n+1}-mA^{n+1},\\ \\ \displaystyle \dfrac{\lambda _1^{N-n}-\lambda _1^{N-n-1}}{\mathrm{d}t} \displaystyle = \alpha _1 + \lambda _1^{N-n-1} \Big (m +\mu _1(P^{n+1})+\mu _2(I^{n+1})\Big ) - \lambda _2^{N-n} \Big (\mu _1(P^{n+1})+\mu _2(I^{n+1})\Big ) \\ \\ \displaystyle \dfrac{\lambda _2^{N-n}-\lambda _2^{N-n-1}}{\mathrm{d}t} \displaystyle = -\alpha _2 +\lambda _1^{N-n-1} S^{n+1} \mu _2'(I^{n+1}) + \lambda _2^{N-n-1} \Big (m-S^{n+1} \mu _2'(I^{n+1})\Big ) - \lambda _3^{N-n} \pi , \\ \\ \displaystyle \dfrac{\lambda _3^{N-n}-\lambda _3^{N-n-1}}{\mathrm{d}t} \displaystyle = \lambda _1^{N-n-1} S^{n+1} \mu _1'(P^{n+1})-\lambda _2^{N-n-1} S^{n+1} \mu _1'(P^{n+1})+\lambda _3^{N-n-1}(m+r A^{n+1}) - \lambda _4^{N-n} r A^{n+1} \\ \\ \displaystyle \dfrac{\lambda _4^{N-n}-\lambda _4^{N-n-1}}{\mathrm{d}t} \displaystyle = \lambda _3^{N-n-1} r P^{n+1} + \lambda _4^{N-n-1} (m-r P^{n+1}) \end{array} \right. \end{aligned}$$

Hence, the following algorithm will be applied under MATLAB software to solve the optimality system and then one deduces the optimal control.