Intelligent Chaos Synchronization of Fractional Order Systems via Mean-Based Slide Mode Controller

Abstract

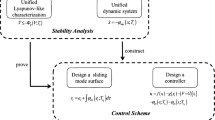
In this paper, a new mean-based adaptive fuzzy neural network sliding mode control is developed to perform the chaos synchronization among the master–slave fractional order uncertain systems. The mean-based expansion is adopted to replace the traditional Taylor expansion to transform a nonlinear function into a partially linear form for the linearization of nonlinear systems. In comparison with the traditional Taylor method, the proposed mean-based method can estimate the first-order derivative term on the identifier model, which will somehow alleviate the computational burden. Based on the learning algorithms, the adaptive laws and control laws can be tuned on-line to synchronize the master–slave fractional order uncertain systems. Furthermore, the stability of the closed-loop system can not only be assured but the synchronization deviation of external perturbation can also be alleviated. Finally, simulation examples are illustrated to demonstrate the feasibility and the synchronization performance of this new approach.

Appendix

Consider the following fractional differential equation:

$$ \begin{aligned} D_{t}^{\alpha } y(t) & = f(y(t),t),\quad 0 \le t \le T \\ y^{(k)} (0) & = y_{0}^{(k)} ,\quad k = 0,1,2, \ldots ,m - 1 \\ \end{aligned}, $$

(51)

which is equivalent to the Volterra integral equation [38] described as

$$ y(t) = \sum\limits_{k = 0}^{m - 1} {y_{0}^{(k)} \frac{{t^{k} }}{k!} + \frac{1}{\Gamma (\alpha )}} \int_{0}^{\text{T}} {(t - \lambda )}^{\alpha - 1} f(y(\lambda ),\lambda ){d}\lambda $$

(52)

Setting $h = {\raise0.7ex\hbox{$T$} \!\mathord{\left/ {\vphantom {T N}}\right.\kern-0pt} \!\lower0.7ex\hbox{$N$}}$, t _n  = nh, n = 0, 1, 2,…, N. Thus, the above Eq. (52) can be discretized as

$$ y_{h} \left( {t_{n + 1} } \right) = \sum\limits_{k = 0}^{m - 1} {y_{0}^{(k)} \frac{{t_{n + 1}^{k} }}{k!} + \frac{{h^{\alpha } }}{\Gamma (\alpha + 2)}} f\left( {y_{h}^{p} (t_{n + 1} ),t_{n + 1} } \right) + \frac{{h^{\alpha } }}{\Gamma (\alpha + 2)}\sum\limits_{j = 0}^{n} {a_{j,n + 1} f\left( {y_{h} (t_{j} ),t_{j} } \right)}, $$

(53)

where

$$ a_{j,n + 1} = \left\{ \begin{array}{ll} n^{\alpha + 1} - (n - \alpha )(n + 1)^{\alpha }, & j = 0 \\ (n - j + 2)^{\alpha + 1} + (n - j)^{\alpha + 1} - 2(n - j + 1)^{\alpha + 1} , & 1 \le j \le n \\ 1, & j = n + 1 \end{array} \right. $$

(54)

and the predicted value $y_{h}^{p} (t_{n = 1})$ is determined by

$$ y_{h}^{p} (t_{n + 1} ) = \sum\limits_{k = 0}^{m - 1} {y_{0}^{(k)} \frac{{t_{n + 1}^{k} }}{k!} + \frac{1}{\Gamma (\alpha )}} \sum\limits_{j = 0}^{n} {b_{j,n + 1} f\left( {y_{h} (t_{j} ),t_{j} } \right)}. $$

(55)

$$ b_{j,n + 1} = \frac{{h^{\alpha } }}{\alpha }\left( {\left( {n + 1 - j} \right)^{\alpha } + (n - j)^{\alpha } } \right). $$

(56)

The approximation error is given as

$$ \hbox{max} \left| {y\left( {t_{j} } \right) - y_{h} \left( {t_{j} } \right)} \right| = O(h^{p} ), \quad j = 1,2, \ldots ,N, $$

(57)

where p = min (2, 1 + α).