Skip to main content
SpringerLink
Log in

Why Gaussian quadrature in the complex plane?

  • Published:
Numerical Algorithms Aims and scope Submit manuscript

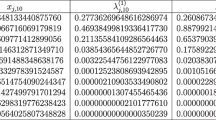
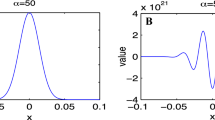
Abstract

This paper synthesizes formally orthogonal polynomials, Gaussian quadrature in the complex plane and the bi-conjugate gradient method together with an application. Classical Gaussian quadrature approximates an integral over (a region of) the real line. We present an extension of Gaussian quadrature over an arc in the complex plane, which we call complex Gaussian quadrature. Since there has not been any particular interest in the numerical evaluation of integrals over the long history of complex function theory, complex Gaussian quadrature is in need of motivation. Gaussian quadrature in the complex plane yields approximations of certain sums connected with the bi-conjugate gradient method. The scattering amplitude c T A −1 b is an example where A is a discretization of a differential–integral operator corresponding to the scattering problem and b and c are given vectors. The usual method to estimate this is to use c T x (k). A result of Warnick is that this is identically equal to the complex Gaussian quadrature estimate of 1/λ. Complex Gaussian quadrature thereby replaces this particular inner product in the estimate of the scattering amplitude.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. D. Arnett, Supernovae and Nucleosynthesis (Princeton Univ. Press, Princeton, 1996).

    Google Scholar 

  2. S.F. Ashby, T.A. Manteuffel and P.E. Saylor, A taxonomy for conjugate gradient methods, SIAM J. Numer. Anal. 27(6) (1990) 1542–1568.

    Google Scholar 

  3. O. Axelsson, Iterative Solution Methods (Cambridge Univ. Press, Cambridge, 1996).

    Google Scholar 

  4. T.L. Barth and T.A. Manteuffel, Conjugate gradient algorithms using multiple recursions, in: Linear and Nonlinear Conjugate Gradient Related Methods, eds. L. Adams and J.L. Nazareth (SIAM, Philadelphia, PA 1996).

    Google Scholar 

  5. W.E. Boyse, D.R. Lynch, K.D. Paulsen and G.N. Minerbo, Nodal based finite element modeling of Maxwell's equations in three dimensions, IEEE Trans. Antennas and Propagation 40(6) (1992) 642–651.

    Google Scholar 

  6. W.E. Boyse, D.R. Lynch, K.D. Paulsen and G.N. Minerbo, Scalar and vector potential formulation for finite element solutions to Maxwell's equations, in: Proc. IEEE AP–S International Symposium, Vol. 1 (Springer, Berlin, 1992) pp. 516–519.

    Google Scholar 

  7. W.E. Boyse, G.N. Minerbo, K.D. Paulsen and D.R. Lynch, Applications of potentials to finite element modeling of Maxwell's equations, IEEE Trans. Magn. 29 (1993) 1333–1336.

    Google Scholar 

  8. C. Brezinski, Padé-Type Approximation and General Orthogonal Polynomials (Birkhäuser, Basel, 1980).

    Google Scholar 

  9. C. Brezinski, Biorthogonality and Its Applications to Numerical Analysis (Marcel Dekker, New York, 1992).

    Google Scholar 

  10. D. Calvetti, G.H. Golub and L. Reichel, An adaptive Chebyshev iterative method for nonsymmetric linear systems based on modified moments, Numer. Math. 67 (1994) 21–40.

    Google Scholar 

  11. W.C. Chew, Waves and Fields in Inhomogeneous Media (Oxford, New York, 1996).

  12. G. Dahlquist and Å. Björck, Numerical Methods (Prentice-Hall, Englewood Cliffs, NJ, 1974).

    Google Scholar 

  13. G. Dahlquist, S.C. Eisenstat and G.H. Golub, Bounds for the error of linear systems of equations using the theory of moments, J. Math. Anal. Appl. 37 (1972) 151–166.

    Google Scholar 

  14. G. Dahlquist, G.H. Golub and S. Nash, Bounds for the error in linear systems, in: Proc. of the Workshop on Semi-Infinite Programming, ed. R. Hettich (Springer, New York) pp. 154–172.

  15. T.A. Driscoll, K.-C. Toh and L.N. Trefethen, From potential theory to matrix iterations in six steps, SIAM Rev. 40 (1998) 547–581.

    Google Scholar 

  16. B. Fischer and G.H. Golub, On the error computation for polynomial based iteration methods, in: Recent Advances in Iterative Methods (Springer, New York, 1993) pp. 59–69.

    Google Scholar 

  17. R. Freund, M. Gutknecht and N.M. Nachtigal, An implementation of the look-ahead Lanczos algorithm for non-Hermitian matrices, SIAM J. Sci. Statist. Comput. 14 (1993) 137–158.

    Google Scholar 

  18. R.W. Freund, The look-ahead lanczos process for large nonsymmetric matrices and related algorithms, in: Linear Algebra for Large Scale and Real Time Applications (Kluwer Academic, Dordrecht, 1993) pp. 137–163.

    Google Scholar 

  19. R.W. Freund and M. Hochbruck, Gauss quadrature associated with the Arnoldi process and the Lanczos algorithm, in: Linear Algebra for Large Scale and Real Time Applications (Kluwer Academic, Dordrecht, 1993) pp. 377–380.

    Google Scholar 

  20. W. Gander and J. Hřebíček, Solving Problems in Scientific Computing using Maple and Matlab (Springer, Berlin, 1995).

    Google Scholar 

  21. W. Gautschi, Orthogonal Polynomials: Applications and Computation, Vol. 5 (Cambridge Univ. Press, Cambridge, 1996) pp. 45–119.

    Google Scholar 

  22. W. Gautschi, Numerical Analysis: An Introduction (Birkhäuser, Boston, 1997).

    Google Scholar 

  23. G.H. Golub, Some modified matrix eigenvalue problems, SIAM Rev. 15(2) (1973) 318–334.

    Google Scholar 

  24. G.H. Golub, Private communication, 1996.

  25. G.H. Golub and G. Meurant, Matrices, moments and quadrature, in: Numerical Analysis 1993: Proc. of the 15th Dundee Conference, June–July 1993, eds. D.F. Griffiths and G.A. Watson, Essex, England, Pitman Research Notes in Mathematics Series, Vol. 303 (Longman, Harlow, 1994) pp. 105–156.

    Google Scholar 

  26. G.H. Golub and G. Meurant, Matrices, moments and quadrature II or how to compute the norm of the error in iterative methods, BIT 37(3) (1997) 687–705.

    Google Scholar 

  27. G.H. Golub and C.F. Van Loan, Matrix Computations, 3rd edn. (Johns Hopkins Univ. Press, Baltimore, MD, 1996).

    Google Scholar 

  28. G.H. Golub and J.H. Welsch, Calculation of Gauss quadrature rules, Math. Comp. 23 (1969) 221–230.

    Google Scholar 

  29. R.G. Gordon, Error bounds in equilibrium statistical mechanics, J. Math. Phys. 9(5) (1967) 655–663.

    Google Scholar 

  30. R.G. Gordon, Error bounds in spectroscopy and nonequilibrium statistical mechanics, J. Math. Phys. 9(7) (1967) 1087–1092.

    Google Scholar 

  31. W.B. Gragg, Positive definite Toeplitz matrices, the Arnoldi process for isometric operators, and gaussian quadrature on the unit circle, J. Comput. Appl. Math. 46 (1993) 183–198; English transl. of 1982 Russian article.

    Google Scholar 

  32. M. Gutknecht, Lanczos Type Solvers for Nonsymmetric Linear Systems of Equations (Cambridge Univ. Press, Cambridge, 1997) pp. 271–398.

    Google Scholar 

  33. M.T. Heath, Scientific Computing: An Introductory Survey (McGraw-Hill, New York, 1997).

    Google Scholar 

  34. P. Henrici, Applied and Computational Complex Analysis, Vol. 2 (Wiley, New York, 1977).

    Google Scholar 

  35. M.R. Hestenes and E. Stiefel, Methods of conjugate gradients for solving linear systems, J. Res. of NBS 49 (1952) 409–435.

    Google Scholar 

  36. C.T. Kelley, Iterative Methods for Linear and Nonlinear Equations, SIAM Frontiers Series, Vol. 16 (SIAM, Philadelphia, PA, 1995).

    Google Scholar 

  37. L.D. Landau and E.M. Lifshitz, Quantum Mechanics (Non-relativistic Theory), 3rd edn. (Pergamon, Oxford, 1977).

    Google Scholar 

  38. L. Lempert, Recursion for orthogonal polynomials on complex domains, Colloq. Math. Soc. János Bolyai 19 (1976) 481–494.

    Google Scholar 

  39. F.E. Low, Classical Field Theory, Electromagnetism and Gravitation (Wiley, New York, 1998).

    Google Scholar 

  40. G.N. Minerbo, G.H. Golub and P.E. Saylor, Nine ways to solve a scattering problem I, Technical Report, Stanford University (2001), in preparation.

  41. R.G. Newton, Scattering Theory of Waves and Particles, 2nd edn. (Springer, New York, 1982).

    Google Scholar 

  42. K.D. Paulsen, Finite element solution of Maxwell's equations with Helmholtz forms, Optical Society of America A: Special Issue on Scattering by Three-dimensional Objects 11 (1994) 1434–1444.

    Google Scholar 

  43. N.A. Pierce and M.B. Giles, Adjoint recovery of superconvergent functionals from pde approximations, SIREV 42(2) (2000) 247–264.

    Google Scholar 

  44. Y. Saad, Iterative Methods for Sparse Linear Systems (PWS, Boston, 1996).

    Google Scholar 

  45. H.S. Shapiro, The Schwarz Function and Its Generalization to Higher Dimensions (Wiley, New York, 1992).

    Google Scholar 

  46. D.C. Smolarski, Optimum semi-iterative methods of the solution of any linear algebraic system with a square matrix, Ph.D. thesis, Department of Computer Science, University of Illinois, Urbana (December 1981). Available as Technical Report 1077.

    Google Scholar 

  47. E. Stiefel, Kernel polynomials in linear algebra and their numerical applications, Nat. Bureau Standards Math. Ser. 49 (1958) 1–22.

    Google Scholar 

  48. G. Szegő, Orthogonal Polynomials, 4th edn. (Amer. Math. Soc., Providence, RI, 1975).

    Google Scholar 

  49. K.F. Warnick, Private communication (1998).

  50. K.F. Warnick, Continued fraction error bound for conjugate gradient method, Technical Report ECEN Department Report No. TR-L100-98.3, Department of Electrical and Computer Engineering, Brigham Young University, Provo, UT 84602 (9 June 1997).

    Google Scholar 

  51. K.F. Warnick, Gaussian quadrature and scattering amplitude (exact title tbd), Technical Report No. TBD, Department of Electrical and Computer Engineering, Center for Computational Electromagnetics, University of Illinois, Urbana–Champaign (2000).

    Google Scholar 

  52. H.S. Wilf, Mathematics for the Physical Sciences (Wiley, New York, 1962).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Computer Science, University of Illinois, Urbana, IL, 61801, USA

    Paul E. Saylor

  2. Department of Mathematics and Computer Science, Santa Clara University, Santa Clara, CA, 95053, USA

    Dennis C. Smolarski

Authors
  1. Paul E. Saylor
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dennis C. Smolarski
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Saylor, P.E., Smolarski, D.C. Why Gaussian quadrature in the complex plane?. Numerical Algorithms 26, 251–280 (2001). https://doi.org/10.1023/A:1016612909180

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1023/A:1016612909180

Search

Navigation