Abstract
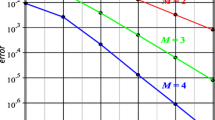
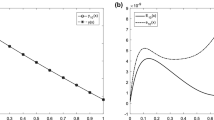
In solving integral equations with a logarithmic kernel, we combine the Galerkin approximation with periodic quasi-wavelet (PQW) [4]. We develop an algorithm for solving the integral equations with only O(N log N) arithmetic operations, where N is the number of knots. We also prove that the Galerkin approximation has a polynomial rate of convergence.
Similar content being viewed by others
References
K. Amaratunga, J.R. Williams, S. Qian and J. Weiss, Wavelet-Galerkin solutions for one-dimensional partial differential equations, Internat. J. Numer. Methods Engrg. 37 (1994) 2703–2716.
G. Beylkin, R.R. Coifman and V. Rokhlin, Fast wavelet trans forms and numerical algorithms I, Commun. Pure Appl. Math. 44 (1991).
M.E. Brewster and G. Beylkin, A multiresolution strategy for numerical homogenization, Appl. Comput. Anal. 2 (1995) 327–349.
H.L. Chen, Periodic orthonormal quasi-wavelet bases, Chinese Sci. Bull. 41(7) (1996).
H.L. Chen and S.L. Peng, Solving integral equations with logarithmic kernel by using periodic quasi-wavelet, J. Comput. Math., to appear.
Z. Chen, C.A. Micchelli and Y. Xu, The Petrov-Galerkin methods for second kind integral equations II: Multiwavelet scheme, Adv. Comput. Math. 7 (1997) 199–233.
S. Dahlke and I. Weinreich, Wavelet Galerkin methods: An adapted biorthogonal wavelet basis, Constr. Approx. 9 (1993) 237–262.
W. Dahmen, B. Kleemann, S. Prossdorf and R. Schneider, Multiscale methods for the solution of the Helmholtz and Laplace equations, Preprint (1997).
I. Daubechies, Ten Lectures on Wavelets, CBMS-NSF Regional Conf. Ser. in Appl. Math., Vol. 61 (SIAM, Philadelphia, PA, 1992).
C. de Boor and G.J. Fix, Spline approximation by quasiinterpolants, J. Approx. 8 (1973) 19–45.
D. Greenspan and P. Werner, A numerical method for the exterior Dirichlet problem for the reduced waved equation, Arch. Rational Mech. Anal. 23 (1966) 288–316.
M. Kamada, K. Toraichi and R. Mori, Periodic spline orthonormal bases, J. Approx. Theory 55 (1988) 27–34.
C.T. Kelley, A fast multilevel algorithm for integral equations, SIAM J. Numer. Anal. 32(2) (1995) 501–513.
Y.W. Koh, S.L. Lee and H.H. Tan, Periodic orthogonal splines and wavelets, Appl. Comput. Harmonic Anal. 2 (1995) 201–218.
R. Kress, Linear Integral Equations (Springer, Berlin/Heidelberg, 1989).
R. Kress and L.H. Sloan, On the numerical solution of a logarithmic integral equation of the first kind for the Helmholtz equation, Numer. Math. 66 (1993) 199–214.
R. Kress and W.T. Spassov, On the condition number of boundary integral operators for exterior Dirichlet problem for the Helmholtz equation, Numer. Math. 42 (1983) 77–95.
S. Mallat, Review of multifrequency channel decomposition of images and wavelet models, Technical Report 412, Robotics Report 178, New York University (1988).
Y. Meyer, Principe d'incertitude, bases hilbertiennes et algèbres d'opérateurs, in: Séminaire Bourbaki 662 (Astérisque, 1985–86).
C.A. Micchelli and Y. Xu, Weakly singular Fredholm integral equations I: Singularity preserving wavelet-Galerkin methods, in: Approximation Theory VIII, Vol. 2: Wavelets and Multilevel Approximation, eds. C.K. Chui and L.L. Schumaker (1995) pp. 283–300.
S.L. Peng, Wavelet transform for fast resolution of second kind integral equation with Calderón-Zygmund kernel, to appear.
R. Schinzinger and P.A.A. Laura, Conformal Mapping: Methods and Applications (Elsevier, Amsterdam, 1991).
L. Schumaker, Spline Functions, Basic Theory (Wiley, New York, 1981).
Z.W. Shen and Y. Xu, Degenerate kernel schemes by wavelets for nonlinear integral equations on the real line, Appl. Anal. 59 (1995) 163–184.
M. Tasche, Orthogonal periodic spline wavelets, in: Wavelets, Image and Surface Fitting, eds. P.J. Laurent, A. Le Méhauté and L.L. Schumaker (1994) pp. 475–484.
Y. Xu and Y. Zhao, An extrapolation method for a class of boundary integral equations, Math. Comp. 65 (1996) 587–610.
Y. Yan, A fast numerical solution for a second kind boundary integral equation with a logarithmic kernel, SIAM J. Numer. Anal. 31(2) (1994) 477–498.
Y. Yan, A fast boundary element method for the two dimensional Helmholtz equations, Comput. Methods Appl. Mech. Engrg., to appear.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Chen, HL., Peng, SL. A quasi-wavelet algorithm for second kind boundary integral equations. Advances in Computational Mathematics 11, 355–375 (1999). https://doi.org/10.1023/A:1018992413504
Issue Date:
DOI: https://doi.org/10.1023/A:1018992413504