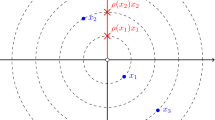
Abstract
We have been witnessing lately a convergence among mathematical morphology and other nonlinear fields, such as curve evolution, PDE-based geometrical image processing, and scale-spaces. An obvious benefit of such a convergence is a cross-fertilization of concepts and techniques among these fields. The concept of adjunction however, so fundamental in mathematical morphology, is not yet shared by other disciplines. The aim of this paper is to show that other areas in image processing can possibly benefit from the use of adjunctions. In particular, a strong relationship between pyramids and adjunctions is presented. We show how this relationship may help in analyzing existing pyramids, and construct new pyramids. Moreover, it will be explained that adjunctions based on a curve evolution scheme can provide idempotent shape filters. This idea is illustrated in this paper by means of a simple affine-invariant polygonal flow. Finally, the use of adjunctions in scale-space theory is also addressed.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Bruckstein, A.M., Sapiro, G., and Shaked, D. 1995. Evolutions of planar polygons. Intern. J. of Pattern Recognition and Artificial Intelligence, 9(6):991–1014.
Goutsias, J. and Heijmans, H.J.A.M. 2000. Nonlinear multiresolution signal decomposition schemes: Part I: morphological pyramids. IEEE Transactions on Image Processing, 9:1862–1876.
Heijmans, H.J.A.M. and Keshet (Kresch), R. 2002. Inf-semilattice approach to self-dual morphology. JVCIR, 13:269–301.
Heijmans, H.J.A.M. and Ronse, C. 1990. The algebraic basis of mathematical morphology. I. Dilations and erosions. Comput. Vision Graphics Image Process., 50:245–295.
Heijmans, H.J.A.M. and van den Boomgaard, R. 2000. Algebraic framework for linear and morphological scale-spaces. CWI Report PNA-R0003, Amsterdam. Also in JVCIR (to appear).
Kimmel, R. 1995. Curve evolution on surfaces. D.Sc. thesis, Technion-Israel Institute of Technology.
Kresch, R. 1998. Extension of morphological operations to complete semilattices and its applications to image and video processing. In Mathematical Morphology and its Applications to Image and Signal Processing (Proc. of ISMM'98), H.J.A.M. Heijmans and J.B.T.M. Roerdink (Eds.), pp. 35–42.
Keshet (Kresch), R. 2000.Amorphological viewon traditional signal processing. In Mathematical Morphology and its Applications to Image and Signal Processing V (Proc. of ISMM'2000), Palo Alto, California, USA.
Malladi, R. and Sethian, J.A. 1995. Image processing via level set curvature flow. Proc. of the National Academy of Sciences, 92(15):7046–7050.
Moisan, L. 1998. Affne plane curve evolution: A fully consistent scheme. IEEE Transactions on Image Processing, 7(3):411–420.
Pauwels, E.J., Van Gool, L.J., Fiddelaers, P., and Moons, T. 1995. An extended class of scale-invariant and recursive scale space filters. IEEE Trans. on PAMI, 17(7).
Rudin, L., Osher, S., and Fatemi, E. 1992. Nonlinear total variation based noise removal algorithms. Physica D, 60:259–268.
Sapiro, G. 1995. Geometric partial differential equations in image analysis: Past, present, and future. In Proc. ICIP'95, Vol. 3, pp. 1–4.
Sapiro, G. 2001. Geometric Partial Differential Equations and Image Analysis. Cambridge University Press.
Serra, J. (Ed.). 1988. Image Analysis and Mathematical Morphology, Volume 2: Theoretical Advances. Academic Press: New York.
Steiner, A., Kimmel, R., and Bruckstein, A.M. 1996. Planar shape enhancement and exaggeration. In Proc. ICPR 96', pp. 523–527.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
(Kresch), R.K., Heijmans, H.J. Adjunctions in Pyramids, Curve Evolution and Scale-Spaces. International Journal of Computer Vision 52, 139–151 (2003). https://doi.org/10.1023/A:1022952007509
Issue Date:
DOI: https://doi.org/10.1023/A:1022952007509