Abstract
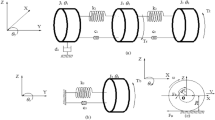
Consideration was given to the system of nonlinear differential equations describing a simplest power system with a unipolar installation, the electromagnetic brake (EMB). Behavior of the system was considered with and without EMB. By constructing a special Lyapunov function, local asymptotic stability of the system with EMB was proved.
Similar content being viewed by others
References
Sharov, Yu.V., Yankovich, I.D., and Kuznetsov, O.N., Method for Improving Dynamic Stability and Damping Oscillation of Electric-Power Systems and Device for Its Realisation, RFPatent no. 2 339 144, Buyll. Izobret., 2008, no. 32 www.fips.ru.
Antipova, N.A. and Kuznetsov, O.N., Determination of the Technical Characteristics of the Electromagnetic Brake for Improving Dynamic Stability of EPS, Vestn. MEI, 2012, no. 1, pp. 54–59.
Panin, A.V., Chumachenko, V.V., and Kuznetsov, O.N., Comparison of Efficiency of the Laws of Control of the Electromagnetic Brake, Izv. Vyssh. Uchebn. Zaved., Probl. Energ., 2014, nos. 5–6.
Tricomi, F., Integrazione di un’equazione differenziale presentatasi in electrotecnica, Annali della Scuola Normale Superiore di Pisa, Classe di Scienze 2, 1933, ser. 2, no. 1, pp. 1–20.
Andronov, A.A., Vitt, A.A., and Khaikin, S.E., Teoriya kolebanii (Oscillation Theory), Moscow: Nauka, 1981, 2nd ed.
Reissig, R., Sansone, G., and Conti, R., Qualitative Theorie nichtlinearer Differentialgleichungen, Roma: Edizioni Cremonese, 1963. Translated under the title Kachestvennaya teoriya nelineinykh differential’nykh uravnenii, Moscow: Nauka, 1974.
Malkin, I.G., Teoriya ustoichivosti dvizheniya (Motion Stability Theory), Moscow: Nauka, 1966, 2nd ed.
Gelig, A.Kh., Leonov, G.A., and Yakubovich, V.A., Ustoichivost’ nelineinykh sistem s needinstvennym sostoyaniem ravnovesiya (Stability of Nonlinear Controls with Nonunique Equilibrium State), Moscow: Nauka, 1978. English translation: World Scientific, 2004.
Leonov, G.A., Vvedenie v teoriyu upravleniya (Introduction to the Control Theory), St. Petersburg: S.-Peterburg. Gos. Univ., 2004.
Pai, M.A., Energy Function Analysis for Power System Stability, Boston: Kluwer, 1989.
Venikov, V.A., Perekhodnye elektromekhanicheskie protsessy v elektricheskikh sistemakh (Transient Processes in Electrical Systems), Moscow: Vysshaya Shkola, 1985, 4th ed.
Galaz, M., Ortega, R., Bazanella, A., and Stankovich, A., An Energy-shaping Approach to the Design of Excitation Control of Synchronous Generators, Automatica, 2003, vol. 39, no. 1, pp. 111–119.
Author information
Authors and Affiliations
Corresponding author
Additional information
Original Russian Text © B.T. Polyak, O.N. Kuznetsov, V.V. Chumachenko, 2016, published in Avtomatika i Telemekhanika, 2016, No. 9, pp. 58–69.
Rights and permissions
About this article
Cite this article
Polyak, B.T., Kuznetsov, O.N. & Chumachenko, V.V. Stability study of a power system with unipolar electromagnetic brake. Autom Remote Control 77, 1557–1566 (2016). https://doi.org/10.1134/S0005117916090046
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0005117916090046