Abstract
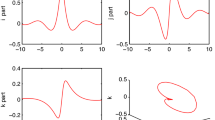
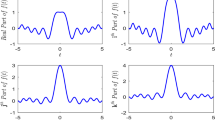
The main purpose of this paper is to study different types of sampling formulas of quaternionic functions, which are bandlimited under various quaternion Fourier and linear canonical transforms. We show that the quaternionic bandlimited functions can be reconstructed from their samples as well as the samples of their derivatives and Hilbert transforms. In addition, the relationships among different types of sampling formulas under various transforms are discussed. First, if the quaternionic function is bandlimited to a rectangle that is symmetric about the origin, then the sampling formulas under various quaternion Fourier transforms are identical. If this rectangle is not symmetric about the origin, then the sampling formulas under various quaternion Fourier transforms are different from each other. Second, using the relationship between the two-sided quaternion Fourier transform and the linear canonical transform, we derive sampling formulas under various quaternion linear canonical transforms. Third, truncation errors of these sampling formulas are estimated. Finally, some simulations are provided to show how the sampling formulas can be used in applications.
摘要
本文主要研究在不同形式四元数傅里叶变换和线性正则变换下有限带宽四元数函数的采样定理. 证明了有限带宽四元数函数可通过它们的直接采样或经过微分和希尔伯特变换后的采样重构. 此外, 讨论了不同形式变换下不同类型采样公式之间的关系. 首先, 如果四元数函数有限带宽区域是关于原点对称的矩形区域, 则不同形式四元数傅里叶变换下四元数采样公式具有相同形式; 否则, 采样公式是不同的. 其次, 利用双边四元数傅里叶变换和线性正则变换的关系, 得到不同形式四元数线性正则变换下有限带宽四元数函数采样定理. 再次, 分析了采样公式的截断误差. 最后, 通过仿真展示采样公式的应用.
Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
Alon G, Paran E, 2021. A quaternionic Nullstellensatz. J Pure Appl Algebr, 225(4):106572. https://doi.org/10.1016/j.jpaa.2020.106572
Bahia B, Sacchi MD, 2020. Widely linear denoising of multicomponent seismic data. Geophys Prospect, 68(2):431–445. https://doi.org/10.1111/1365-2478.12850
Bulow T, Sommer G, 2001. Hypercomplex signals—a novel extension of the analytic signal to the multidimensional case. IEEE Trans Signal Process, 49(11):2844–2852. https://doi.org/10.1109/78.960432
Chen LP, Kou KI, Liu MS, 2015. Pitt’s inequality and the uncertainty principle associated with the quaternion Fourier transform. J Math Anal Appl, 423(1):681–700. https://doi.org/10.1016/j.jmaa.2014.10.003
Cheng D, Kou KI, 2018. Generalized sampling expansions associated with quaternion Fourier transform. Math Methods Appl Sci, 41(11):4021–4032. https://doi.org/10.1002/mma.4423
Cheng D, Kou KI, 2019. FFT multichannel interpolation and application to image super-resolution. Signal Process, 162:21–34. https://doi.org/10.1016/j.sigpro.2019.03.025
Cheng D, Kou KI, 2020. Multichannel interpolation of nonuniform samples with application to image recovery. J Comput Appl Math, 367:112502. https://doi.org/10.1016/j.cam.2019.112502
Ell TA, Le Bihan N, Sangwine SJ, 2014. Quaternion Fourier Transforms for Signal and Image Processing. John Wiley & Sons, Hoboken, USA.
Hahn SL, Snopek KM, 2005. Wigner distributions and ambiguity functions of 2-D quaternionic and monogenic signals. IEEE Trans Signal Process, 53(8):3111–3128. https://doi.org/10.1109/TSP.2005.851134
Hitzer E, 2017. General two-sided quaternion Fourier transform, convolution and Mustard convolution. Adv Appl Clifford Algebr, 27(1):381–385. https://doi.org/10.1007/s00006-016-0684-8
Hitzer EMS, 2007. Quaternion Fourier transform on quaternion fields and generalizations. Adv Appl Clifford Algebr, 17(3):497–517. https://doi.org/10.1007/s00006-007-0037-8
Hu XX, Kou KI, 2017. Quaternion Fourier and linear canonical inversion theorems. Math Methods Appl Sci, 40(7):2421–2440. https://doi.org/10.1002/mma.4148
Hu XX, Kou KI, 2018. Phase-based edge detection algorithms. Math Methods Appl Sci, 41(11):4148–4169. https://doi.org/10.1002/mma.4567
Jagerman D, 1966. Bounds for truncation error of the sampling expansion. SIAM J Appl Math, 14(4):714–723. https://doi.org/10.1137/0114060
Jiang MD, Li Y, Liu W, 2016. Properties of a general quaternion-valued gradient operator and its applications to signal processing. Front Inform Technol Electron Eng, 17(2):83–95. https://doi.org/10.1631/FITEE.1500334
Kou KI, Morais J, 2014. Asymptotic behaviour of the quaternion linear canonical transform and the Bochner-Minlos theorem. Appl Math Comput, 247:675–688. https://doi.org/10.1016/j.amc.2014.08.090
Kou KI, Qian T, 2005a. Shannon sampling in the Clifford analysis setting. Z Anal Anwend, 24(4):853–870.
Kou KI, Qian T, 2005b. Shannon sampling and estimation of band-limited functions in the several complex variables setting. Acta Math Sci, 25(4):741–754. https://doi.org/10.1016/S0252-9602(17)30214-X
Kou KI, Ou JY, Morais J, 2013. Uncertainty principle for quaternionic linear canonical transform. Abstr Appl Anal, Article 725952.
Kou KI, Liu MS, Morais JP, et al., 2017. Envelope detection using generalized analytic signal in 2D QLCT domains. Multidim Syst Signal Process, 28(4):1343–1366. https://doi.org/10.1007/s11045-016-0410-7
Lian P, 2021. Quaternion and fractional Fourier transform in higher dimension. Appl Math Comput, 389:125585. https://doi.org/10.1016/j.amc.2020.125585
Marvasti F, 2001. Nonuniform Sampling: Theory and Practice. Springer Science & Business Media, New York, USA.
Pan WJ, 2000. Fourier Analysis and Its Applications. Peking University Press, China (in Chinease).
Pei SC, Ding JJ, Chang JH, 2001. Efficient implementation of quaternion Fourier transform, convolution, and correlation by 2-D complex FFT. IEEE Trans Signal Process, 49(11):2783–2797. https://doi.org/10.1109/78.960426
Splettstösser W, Stens RL, Wilmes G, 1981. On approximation by the interpolating series of G. Valiron. Funct Approx Comment Math, 11:39–56.
Yao K, Thomas JB, 1966. On truncation error bounds for sampling representations of band-limited signals. IEEE Trans Aerosp Electron Syst, AES-2(6):640–647.
Zayed AI, 1993. Advances in Shannon’s Sampling Theory. CRC Press, Boca Raton, USA.
Zou CM, Kou KI, Wang YL, 2016. Quaternion collaborative and sparse representation with application to color face recognition. IEEE Trans Image Process, 25(7):3287–3302. https://doi.org/10.1109/TIP.2016.2567077
Author information
Authors and Affiliations
Contributions
Xiaoxiao HU designed the research. Xiaoxiao HU and Dong CHENG processed the data. Xiaoxiao HU drafted the paper. Kit Ian KOU helped organize the paper. Xiaoxiao HU and Dong CHENG revised and finalized the paper.
Corresponding author
Ethics declarations
Xiaoxiao HU, Dong CHENG, and Kit Ian KOU declare that they have no conflict of interest.
Additional information
Project supported by the Research Development Foundation of Wenzhou Medical University, China (No. QTJ18012), the Wenzhou Science and Technology Bureau of China (No. G2020031), the Guangdong Basic and Applied Basic Research Foundation of China (No. 2019A1515111185), the Science and Technology Development Fund, Macau Special Administrative Region, China (No. FDCT/085/2018/A2), and the University of Macau, China (No. MYRG2019-00039-FST)
Supplementary materials for
11714_2022_1779_MOESM1_ESM.pdf
Sampling formulas for 2D quaternionic signals associated with various quaternion Fourier and linear canonical transforms
Rights and permissions
About this article
Cite this article
Hu, X., Cheng, D. & Kou, K.I. Sampling formulas for 2D quaternionic signals associated with various quaternion Fourier and linear canonical transforms. Front Inform Technol Electron Eng 23, 463–478 (2022). https://doi.org/10.1631/FITEE.2000499
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1631/FITEE.2000499
Key words
- Quaternion Fourier transforms
- Quaternion linear canonical transforms
- Sampling theorem
- Quaternion partial and total Hilbert transforms
- Generalized quaternion partial and total Hilbert transforms
- Truncation errors