Published online by Cambridge University Press: 12 March 2014
In this paper I am going to set forth a formal system based on five inter-class relations. These relations exist respectively between a class of a's and a class of b's.
(i) if and only if every a is a b and every b is an a,
(ii) if and only if every a is a b and not every b is an a,
(iii) if and only if it is not the case that either every a is a b or every b is an a or no a is a b,
(iv) if and only if every b is an a and not every a is a b,
(v) if and only if no a is a b.
These relations between classes, which correspond, as will be seen, to the five relations between two circles a and b shown in the well-known Eulerian diagrams,
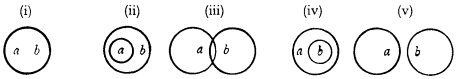
are of course connected in an intimate way with the four forms of proposition, A, E, I, O, of the traditional syllogistic logic. The French mathematician, J. D. Gergonne, seems to have been the first to recognize these relations explicitly and to understand their importance in syllogistic theory. It is therefore appropriate that they should be called by his name.
Gergonne first of all showed with reference to these relations what are the sufficient and necessary conditions of the truth of propositions of each of the four traditional forms: for example, an A proposition, ‘All a is b’ is true if and only if either the first or the second relation exists between the class of a's and the class of b's.
1 Gergonne, J. D., Essai de dialectique rationelle, Annales des mathématiques pures et appliquées, Vol. 7, (1817)Google Scholar.
2 Op.cit., p. 199.
3 See, however, comment on (32.3), p. 217.
4 Professor Łukasiewicz drew my attention to this point.
To send this article to your Kindle, first ensure no-reply@cambridge.org is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about sending to your Kindle. Find out more about saving to your Kindle.
Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.
Find out more about the Kindle Personal Document Service.
To save this article to your Dropbox account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Dropbox account. Find out more about saving content to Dropbox.
To save this article to your Google Drive account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Google Drive account. Find out more about saving content to Google Drive.