Published online by Cambridge University Press: 12 March 2014
We say that a set A of reals is recursive in a real y together with a set B of reals if one can imagine a computing machine 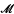 with an ability to perform a countably infinite sequence of program steps in finite time and with oracles for B and y so that
with an ability to perform a countably infinite sequence of program steps in finite time and with oracles for B and y so that 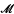 decides membership in A for any real x input to
decides membership in A for any real x input to 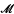 by way of an oracle for x. We write A ≤ yB. A precise definition of this notion of recursion was first considered in Kleene [9]. In the notation of that paper, A ≤yB if there is an integer e so that χA(x) = {e}(x y, χB, 2E). Here χA is the characteristic function of A. Thus Kleene would say that A is recursive in (y, B, 2E), where 2E is the existential integer quantifier.
by way of an oracle for x. We write A ≤ yB. A precise definition of this notion of recursion was first considered in Kleene [9]. In the notation of that paper, A ≤yB if there is an integer e so that χA(x) = {e}(x y, χB, 2E). Here χA is the characteristic function of A. Thus Kleene would say that A is recursive in (y, B, 2E), where 2E is the existential integer quantifier.
Gandy [5] observes that the halting problem for infinitary machines such as 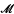 , as in the case of Turing machines, gives rise to a jump operator for higher type recursion. Thus given a set B of reals, the superjump B′ of B is defined to be the set of all triples 〈e, x, y〉 such that the eth machine with oracles for y and B eventually halts when given input x. A set A is said to be semirecursive in y together with B if for some integer e, A is the cross section {x: 〈e, x, y 〉 ∈ B′}. In Kleene [9] it is demonstrated that a set A is semirecursive in y alone if and only if it is
, as in the case of Turing machines, gives rise to a jump operator for higher type recursion. Thus given a set B of reals, the superjump B′ of B is defined to be the set of all triples 〈e, x, y〉 such that the eth machine with oracles for y and B eventually halts when given input x. A set A is said to be semirecursive in y together with B if for some integer e, A is the cross section {x: 〈e, x, y 〉 ∈ B′}. In Kleene [9] it is demonstrated that a set A is semirecursive in y alone if and only if it is 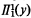
To send this article to your Kindle, first ensure no-reply@cambridge.org is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about sending to your Kindle. Find out more about saving to your Kindle.
Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.
Find out more about the Kindle Personal Document Service.
To save this article to your Dropbox account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Dropbox account. Find out more about saving content to Dropbox.
To save this article to your Google Drive account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Google Drive account. Find out more about saving content to Google Drive.